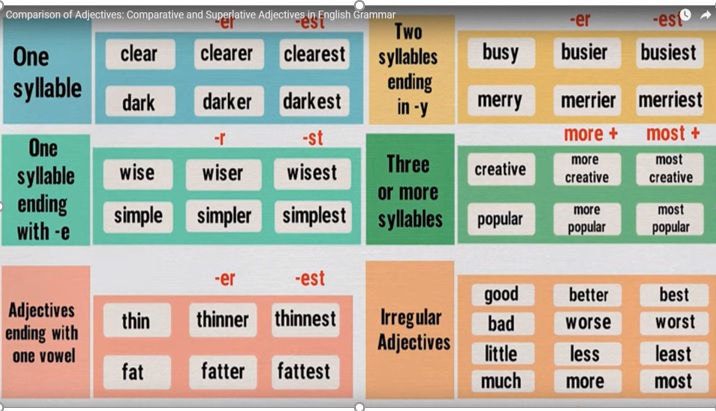
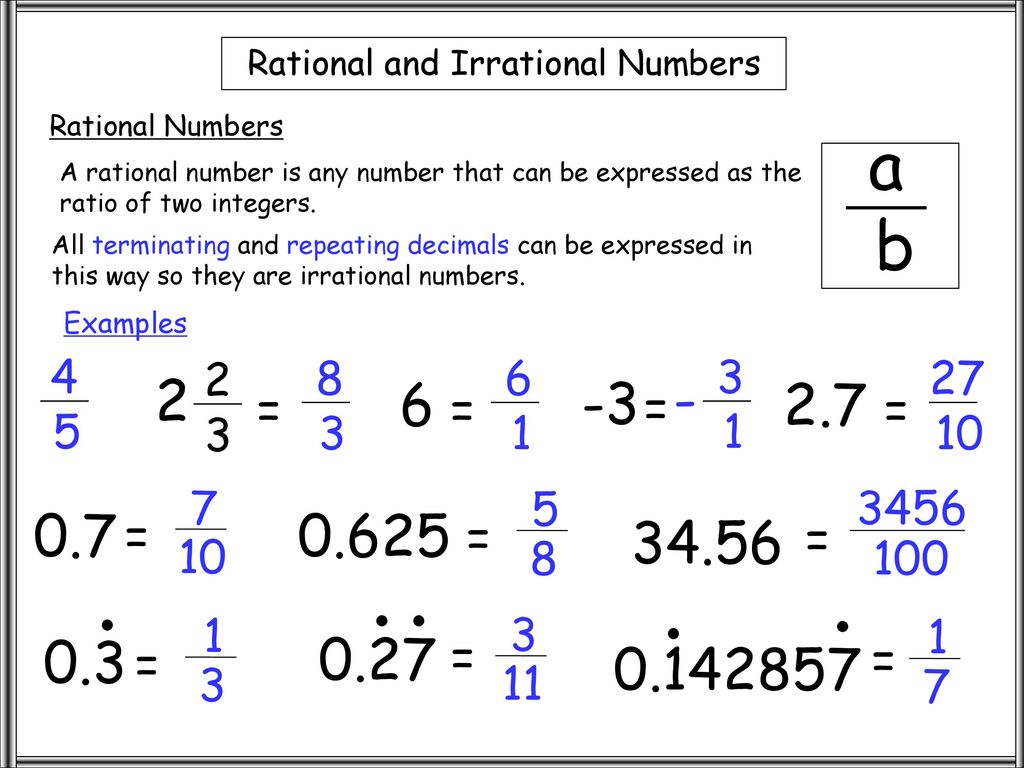
Example of number sense
The Ultimate Guide to Number Sense
Welcome to all things number sense. From recognizing numbers to ordering and comparing. Essential math information for teaching preschool math and beyond!
Number sense is a broad term that refers to a group or set of skills that are needed to perform basic math operations.
You might think that number sense is just simple counting and that young children will master number sense quickly. However, number sense refers to deep understanding of HOW numbers work. It will take some time for young children to master these skills!
Number sense is the critical foundation that ensures that students will be successful in mathematics for years down the road. It lines them up for success in addition and subtraction and understanding how these operations are related. In a nutshell, it is a critical part of preschool and kindergarten math development.
Number Sense Skills Include:
- Rote Counting (naming numbers in order)
- Number Recognition (recognizing numbers in print)
- Counting with One to One Correspondence (each number has a constant value)
- Comparing Quantities (understanding the concept of more/less/same)
- Cardinality of Number (recognizing that the last number said when counting is the number of total objects)
- Conservation of Number (understanding that the number of objects is always the same even when rearranged)
- Subitizing (the ability to recognize “how many” in a set without counting individually)
Let’s dive in! We’ll tell you a little more about each number sense skill, and give activities to practice it.
Plus, grab our free counting mats to get started on these skills right away!
Number Sense Skill: Rote Counting
Rote counting is like dipping the toe into the number sense pool. Young children begin rote-counting (with mistakes) at an early age, often around 2 years old.
To rote count is to count verbally in sequential order. Children mimic the pattern of numbers that they have heard, without understanding number value or quantity. Once children have mastered rote counting skills, they are able to progress to tagging – which is the prerequisite for one to one correspondence.
Rote Counting Activities
Rote counting is a breeze to practice – the important part is to make it fun and exciting! There are many fun, simple rote counting activities that can be added to your preschool math plans.
- Invite young children to count along with you using different voices (soft, loud, silly, grumbly, etc.).
- Sing along with counting songs like 5 Little Speckled Frogs and One, Two, Buckle My Shoe.
 You’ll find plenty of counting songs in our list of songs that teach.
You’ll find plenty of counting songs in our list of songs that teach. - Move and count! Incorporate counting as you clap, dance, jump, and hop.
- Use a puppet or stuffed animal to practice rote counting. Play a game that when the puppet gets to 10 it has to dance and start all over again!
Number Sense Skill: Number Recognition
Number identification is the ability to recognize a number in print and verbalize or write it. “At its most basic level, numeral identification is a form of shape recognition, which can result in a simple association of the word “two” with the symbol ‘2’ without a cardinal meaning” (Mix, Sandhofer, & Baroody, 2005). This means number recognition can develop at a different time than number value.
Number Recognition Activities
- Number bingo and number scavenger hunts are all creative and fun ways for young children to practice identifying the numeral.
- Textured numbers – sandpaper numbers and/or glitter/salt numbers are a great way to invite children to trace the number’s formation with their index finger.

- Using playdough snakes to form numbers is a great way to practice learning and making numbers!
- Stamping numbers is a kid favorite for practicing number recognition
- Give numbers a purpose – teach your students their phone number!
Number Sense Skill: One to One Correspondence
During the tagging stage, children will begin assigning numbers to objects. The numbers will be often out of order or random during this phase. For example, a student who has 6 apples placed in front of him may say, “1, 2, 4, 7, 8. There are 8 apples!” This student is making the connection that we use numbers to count objects, but his number sense is still too immature to understand that each number has a value. Tagging precedes one to one correspondence and children may not physically touch or attempt to manipulate the items, rather they just “count” by observation, which often results in incorrect counting or accidental re-counting.
During the one to one correspondence stage, children begin to understand the rule of counting. It’s the rule that each number translates to a specific quantity AND recognizes that numbers are a symbol to show a quantity. The student in the tagging example above has now begun to use correct number order to count the 6 apples placed in front of him.
It’s the rule that each number translates to a specific quantity AND recognizes that numbers are a symbol to show a quantity. The student in the tagging example above has now begun to use correct number order to count the 6 apples placed in front of him.
Check out our post about one to one correspondence for even more great information around this critical number sense skill.
One to One Correspondence Activities
- Use ten frame mats to help children count accurately. Show the child how to count to the desired number by slowly touching each object. Then, have the child do it after you so that you can assess any areas that they might need help with, whether it’s counting or following their finger. Check out our free dinosaur ten frame mats!
- Use counting at every natural opportunity. Count the grapes on your plate, count the butterflies in the book, and count the school buses in the parking lot.
- Use dice to help count out coins, pasta, stuffed animals, or books.
 Incorporate dice into an easy scavenger hunt at home or school. The options are endless!
Incorporate dice into an easy scavenger hunt at home or school. The options are endless!
We’ve got a set of counting cards in our store specifically to help with one to one correspondence! Each theme has a card with numbers 1-24. Switch out the seasonal counting cards to keep children’s interests and to follow along with your theme. These make a wonderful addition to your preschool and kindergarten math centers!
Number Sense Skill: Comparing Quantities
Learning to compare quantities helps preschoolers make sense of the relationships between numbers: three is one more than two, but it is one less than four. Most preschoolers naturally understand the concept of more or less, as they can quickly tell you if someone got more cookies than them!
Since many preschoolers understand more and less in a general sense, learning to compare with small numbers is a great place to start.
Comparing Quantities Activities
- Use a foam number puzzle as a creative way to count gems using one to one correspondence, and then compare the amounts in each number.

- Line up a small number of objects, perhaps 4 cars. Then line up 5 cars directly below each of the 4 cars. Ask children which row has more cars. Encourage them to share how they know. Use this same activity for less and same quantities.
- With connecting cubes, Legos, or building blocks invite the child to build two towers. Invite the children to make one tower more/less/or the same as another.
- Use real-life examples of more/less/same as they arise. Call attention to the two students who are wearing red and the four students who are wearing green. Invite the students to notice other ways to compare.
Number Sense Skill: Cardinality
Cardinality is recognizing that the last number said when counting is the number of total objects in the group. It’s the ability to count each object individually and correctly declare the total number of items in a set. This is the final and BIG step in one to one correspondence! During this stage, students may also begin to see patterns, such as five-frames and begin to know the total number of objects without counting (also known as subitizing).
Cardinality Activities
- Encourage children to be helpful! Invite them to count out items (snack, blocks, crayons, etc.) to share with other children.
- Invite children to count everything! Count the pencils on the table, the windows in the house, or the trucks in the sand box. Reinforce the total number of objects by confirming the child’s count. For example, “Yes, Maya! There are 5 trucks in the sandbox today. The total number is 5 trucks.”
Number Sense Skill: Conservation of Number
This important component of number sense is one that takes time for young children to master. Conservation of number shows that the child is able to understand that a number of objects remains the same even when rearranged, therefore there is no need to recount.
For example, Billy counted out 7 red fire trucks and declared the total proudly. You then moved the fire trucks all around and rearranged them and asked Billy, “How many fire trucks are there now?” If Billy recounts them each time the question is asked, he is still working on conservation of number and needs more time to conceptualize the value of numbers.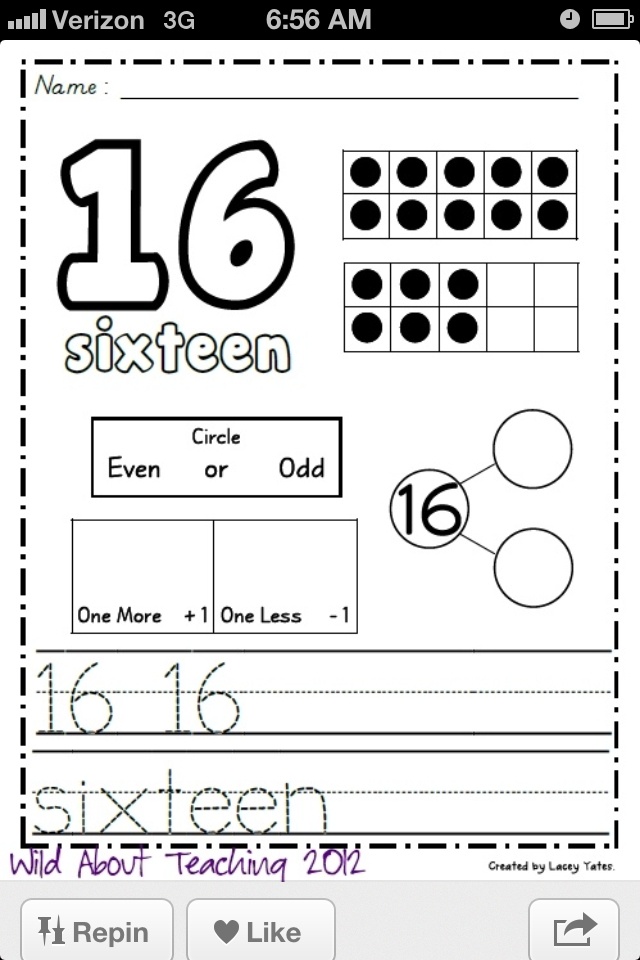 Repeated practice with this concept is crucial in preschool math.
Repeated practice with this concept is crucial in preschool math.
Conservation of Number Activities
- Invite a child to join you in a counting game. Place six items on the table and ask the student to count how many total items there are. Once it is counted correctly (if not, work on one to one correspondence), mix up the items and ask the student to share how many there are now.
- If the child needs to recount, tell the student that you did not add any more items and you did not take any items away. If the child continues to recount, model counting the group of items in lots of configurations and stating that it’s always 6 because I didn’t add any items or take any away.
- Invite one child to count 5 objects into a box. After counting the 5 objects, ask the child to tell you how many are inside the box. If needed, remind them that the number they put inside is still 5 because they did not add or take away any objects. Repeat with other numbers.

- Show multiple children a numeral, perhaps 4. Invite them to hunt the room and find 4 of the same items (crayons, books, blocks, counters, etc.). Invite the children to bring back the 4 items they found and share and count the items with their peers. Encourage them to discuss why some sets of the same number of objects look different.
Number Sense Skill: Subitizing
Subitizing is the ability to recognize “how many” in a set without counting individually. It’s the ability to know the number by “sight”. It’s also an ideal way to introduce simple addition and subtraction. Use dot cards, tally marks, fingers, and ten frames to help your littles becoming subitizing masters! Subitizing practice is a vital part of your preschool and kindergarten math curriculums.
For even more subitizing tips, tricks, and insight, check out our Subitizing 101 post!
Subitizing Activities
- Use music, movement, and Jack Hartmann to teach subitizing! These engaging videos, Subitize to 5 and Subitize to 10 are a great way to get some quick and fun subitizing practice.

- Subitize with dot cards, dice, or dominoes. Hold one up to the class for a few seconds and then ask the students to share how many they saw. Help students see the pattern inside the dots. For example, a 4 on a dice shows 2 rows of 2 which equals 4.
- Subitize with fingers! Hold up 3 fingers and ask students to share how many without counting. Then ask the children to model showing three fingers on their hand.
We hope this introduction to all things number sense has given you loads of ideas for your students and helped you feel more confident when teaching preschool and kindergarten math! Incorporate a variety of these activities into your lessons and watch the light bulbs illuminate in your little ones’ brains. Keep it fun, hands-on, and engaging and their number sense is sure to flourish.
- Author
- Recent Posts
Lauren Vaughan
I am an educator, book enthusiast, and a stay at home momma to a precious and long-awaited little boy and girl. My degree is in Early Childhood Education and Curriculum and Instruction and I have spent the last 15 years working with littles. I feel very fortunate to have this time to watch my babies grow and I can’t wait to share my passion for learning and reading with you!
My degree is in Early Childhood Education and Curriculum and Instruction and I have spent the last 15 years working with littles. I feel very fortunate to have this time to watch my babies grow and I can’t wait to share my passion for learning and reading with you!
Latest posts by Lauren Vaughan (see all)
What All Teachers Ought To Know
Math tips and strategies for building number sense to 20 in Kindergarten and first grade: An extensive list of number sense activities and resources are included: books, materials, math manipulatives, and FREE activities!
The math tips and strategies you need for building number sense to 20 are right here. You will have everything you need to quickly implement and bring a wealth of number sense activities, games, and centers into your classroom or home.
Building Number Sense to 20
The activities, books, resource suggestions (including a FREE one) cover a ton of number sense concepts and are perfect for Kindergarten and first grade. Plus, find valuable number sense materials and manipulative suggestions, as well as freebies you can access today! Warning: It’s LONG but filled with so much you won’t want to miss anything!
Plus, find valuable number sense materials and manipulative suggestions, as well as freebies you can access today! Warning: It’s LONG but filled with so much you won’t want to miss anything!
Why is Building Number Sense Important?
Children need opportunities to learn and experience numbers. Learning to count, identify numbers, and understand quantity are a few of the essential concepts. Repeated experiences build understanding and fluency with numbers. These experiences help expand knowledge and learn new ideas. A strong number sense is vital for future understanding of more complex math topics.
The activities below are ones that have been used successfully. Use them as they are described or adapt them to your own needs. Be flexible. Have conversations about numbers and encourage questions. When there is an opportunity to order, count, match, or describe with a child, go for it! They benefit from seeing numbers in natural, fluid ways.
Make time for fun and engaging number activities daily and watch fluency develop.
Number Sense Concepts & Activities
Build Number Sense Through Counting
It is crucial to count with children every day. Repeated oral counting helps them hear what numbers sound like and to learn their order. Using math manipulatives, like counting chips, can help build a connection to verbal counting and counting objects. This one-to-one counting is an important skill that needs to be practiced often.
Activities
- Count out loud as a class starting at one and building to higher and higher numbers.
- Sit with a partner or in a circle and take turns counting by 1’s.
- As counting develops, teach number patterns and counting by 2’s, 5’s, and 10’s.
- Use a number chart to see what numbers look like visually. Point to each number as it is said.
- Encourage kids to practice one-to-one counting with small objects. Have small groups of objects set up in a center for them to count.
- Provide number cards (like those seen below) for kids to represent with counting chips.

Build Number Sense by Matching Numbers to Quantities
Provide materials for kids to count and connect with objects and numbers. This encourages children to see that numbers are more than words. Teach children that numbers have many representations, such as dots, fingers, counters, numerals, objects, ten frames, etc.
Activities
- Match two different types of objects together, such as five counting bears and a domino that shows five dots.
- Use links to create a number chain for a number.
- Work up to showing quantity with more than two types of objects (as seen in the photo).
- Have many different items available for activities, such as dice, dominoes, number magnets, bears, counting chips, cubes, and number cards.
- Match number cards from a card deck or game of Uno with dots on dominoes. Find every number combination on the dominoes.
- Complete number puzzles that have a different number representation on each piece.
 They help kids see that numbers can be modeled in many ways. These number puzzles to 20 are available as a FREE download further on.
They help kids see that numbers can be modeled in many ways. These number puzzles to 20 are available as a FREE download further on.
- Encourage one-to-one counting with clip cards. Kids count groups of objects and clip the numeral that matches to the correct quantity.
- Ten frames are great for organizing numbers so that they can be easily counted. Matching numeral cards with ten frame representations is simple, yet effective at connecting quantity with numbers.
Build Number Sense with Number of the Day
When kids see numbers in many ways at once in a repeatedly, their number sense develops. Pick a number of the day starting at one and dissect it during your math block.
Activities
- Learn about a specific number for each day that you have been at school. Teach and dissect that number during calendar time. Represent that number on a number line, count to that number, show that number with straws or cubes, etc.

- Use a number of the day poster or anchor chart to break numbers down. Create your number of the day poster with the FREE poster templates.
- Encourage kids to participate and show their understanding on paper or mini whiteboards. Ask a question, such as “How do we show 5 with tally marks?” and allow them time to try on their own. This makes an excellent fluency building warm-up to math lessons.
- Read more tips on starting a number of the day routine in your classroom.
Build Number Sense by Ordering Numbers
Provide opportunities for kids to put numbers in order. While they put numbers or objects with numbers on them into the correct order, they are counting and building number sense.
Activities
- Provide opportunities with using a number line and number chart that puts numbers in order for us.
- Give sets of numbers to order correctly by finding them on the number line.
- Cover different numbers on a number chart with sticky notes and ask kids for the missing numbers.
- Build Lego stacks by putting the pieces together in an order written on the blocks.
- Work together with a partner to order number cards from 0 to 20. Create a number caterpillar like the one seen below.
- Number puzzles are a fun way to order numbers. Put the strips in the right order to reveal a picture.
- Puzzles are simple and easy to create. Take an old puzzle and write numbers on the back of each piece.
- Grab a piece of paper and write numbers in rows. Cut out pieces randomly and trade with a partner. Put the puzzle back together again for practice.
- Complete a number order maze starting at 0. This activity can be reused when put into a pocket protector.
Build Number Sense with Place Value Activities
Teaching kids about place value should begin with teaching them to see ten in many ways. Move on to illustrating numbers with base ten blocks.
Activities
- Practice making groups of ten things. Group straws, beans, counters, or any small objects.
- Teach counting by 10’s to count groups. Extend to teaching groups first and then singles.
- Make groups of ten to get to the 100th day.
- Show and examine a tens block and ones block. Discuss that a tens block has ten ones put together.
- Use base ten blocks to represent numbers. Do plenty of examples where kids must use and count blocks. Use a chart to help count the blocks.
- Say “Show (or draw) me 14 with place value blocks!”
- Represent the number of tens and ones on place value mats (seen below). Ask questions like “How many ones are there?” or “How many groups of ten?”. Make sure they understand what each number within the number stands for (i.e., the 1 in 18 is a ten and not a one).
- Represent a number in different ways: expanded form, standard form, word form, numeral, etc. Worksheets like the one below, can help build this connection.
Reading and Writing Numerals
Kids are constantly surrounded by numbers in their environment. They quickly learn to recognize the individual numerals 0 to 9 through different experiences. Continue to encourage this familiarity by having numbers on the wall. Posters, number lines, and calendars are helpful tools.
Activities
- Build numbers with playdough in a math center. Even something as simple as this playdough numbers activity here.
- Print numbers from 0 to 9 daily in different ways – in booklets, on whiteboards during math lessons, etc.
- Draw numbers on paper and give students do-a-dot markers or bingo dabbers to dot numbers.
- Use a Boogie Board (seen below) or whiteboard to practice numbers over and over quickly.
- Trace numbers frequently and repeatedly to build coordination and correct form. The mini-book below encourages this, as well as locating numbers.
- Encourage the correct formation of numbers by teaching it explicitly.
 The posters (seen below) are helpful tools to use and post on the wall.
The posters (seen below) are helpful tools to use and post on the wall. - Catchy number poems help students visualize and remember number formation. Use your whole body to make actions or draw in the air.
Reading and Writing Number Words
Teach kids how to read number words as they learn to read. Teach each number word one at a time and how to spell them phonetically (when applicable).
Activities
- Have number posters with both the numeral and number word on it.
- Include number words on spelling lists so that kids learn to read and spell number words.
- Use a number of the day routine to teach and focus on one word each day.
- Match numbers word cards with numeral cards (seen below).
- Play memory with a partner with number words and numeral cards. Look for pairs (twelve and 12).
- Build recognizing and speed with mental math flash cards. Flashcards to kids, and they race to call out what they see (tally, numerals, number words, etc.
 ).
).
Build Number Sense by Counting On and Back
Teach the strategy of counting on and back from a number to prepare kids for addition and subtraction.
Activities
- Give students a number and a group of small objects to count on. Ask, “How many more do I have?” given 7 to start and a group of 9 counters to count on with.
- Play games with small objects and decks of cards where counting on is needed.
- Play a group counting game called ‘Around the World.’ Say a number, and each person counts on and says the next number as you go around the circle.
- Use small objects to count on or back from a number given (cubes, counters, dice, etc.).
- Put numbers in backward order. Take the ordering activities seen above and reverse them.
Build Number Sense through Estimating
Provide lots of opportunities for kids to guess how many things they see in their day to day lives. Ask prompting questions to encourage this thinking. Make sure kids know that they are estimating and that these are just guesses. Stress that estimates do not need to be exact but that they should be thoughtful. With more practice, estimations should become closer to the actual number of objects.
Make sure kids know that they are estimating and that these are just guesses. Stress that estimates do not need to be exact but that they should be thoughtful. With more practice, estimations should become closer to the actual number of objects.
Activities
- Fill clear or open containers with different sized objects for kids to guess. Keep them simple and work up in difficulty and size of objects. Smaller objects and bigger containers are usually harder.
- Create an Estimation Station,” where containers are set up with objects inside. Kids can take a close look and make estimates.
- As a whole group activity, grab a pile of objects to show quickly. Cover them and have kids make guesses.
- Play ‘Show & Hide’ with a partner. Use a cup and put some small counting bears inside. Quickly show a partner, and then hide them. They make an estimate, and then you count together to check.
Build Number Sense by Comparing Numbers
As familiarity with numbers grow, so does an ability to compare numbers with each other.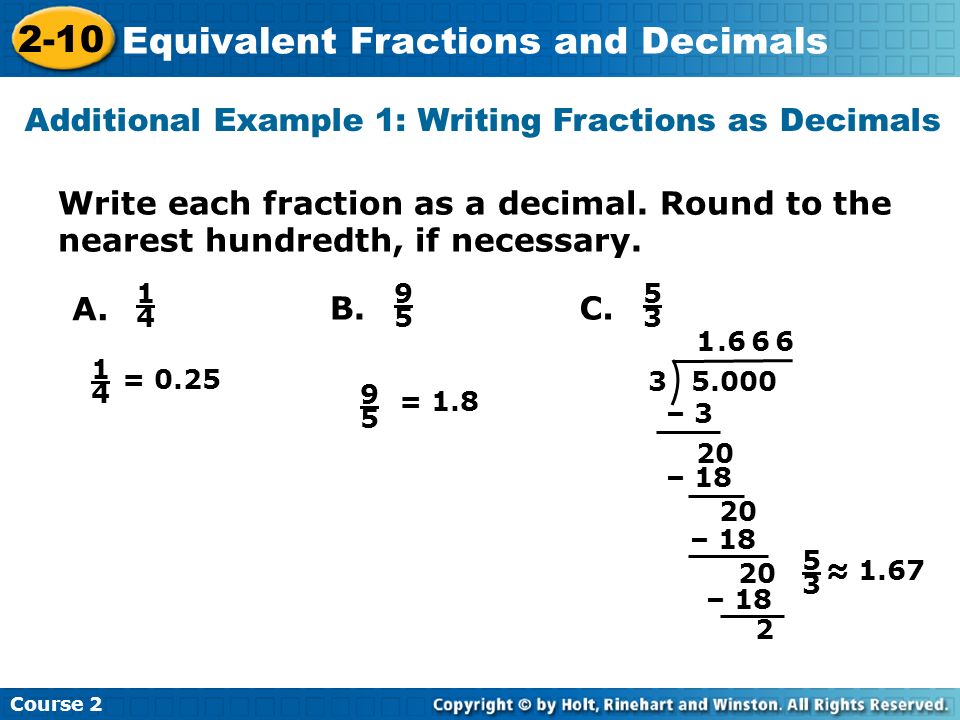 Provide opportunities to compare numbers daily. Ask questions about which number is bigger, smaller, or the same.
Provide opportunities to compare numbers daily. Ask questions about which number is bigger, smaller, or the same.
Activities
- Use a chart or number line to compare numbers.
- Use small objects to make comparing easier. Say “Show 11 and 15 with cubes”. Ask, “Which number is smaller?”.
- Teach the symbols < > and = and what they each mean. Use the alligator strategy (The alligator likes to eat the bigger number) to remember the signs and what they mean.
- Create number towers. Kids use cubes to form towers to represent numbers. Compare two towers to see which is the tallest and, therefore, the bigger number. The alligator eats the bigger tower.
- Compare numbers with math tools and indicate the correct symbol on clip cards (seen below). As kids become fluent, they should recognize the correct symbol without support.
The activities seen in this post can be found in the following resources found on Teachers Pay Teachers.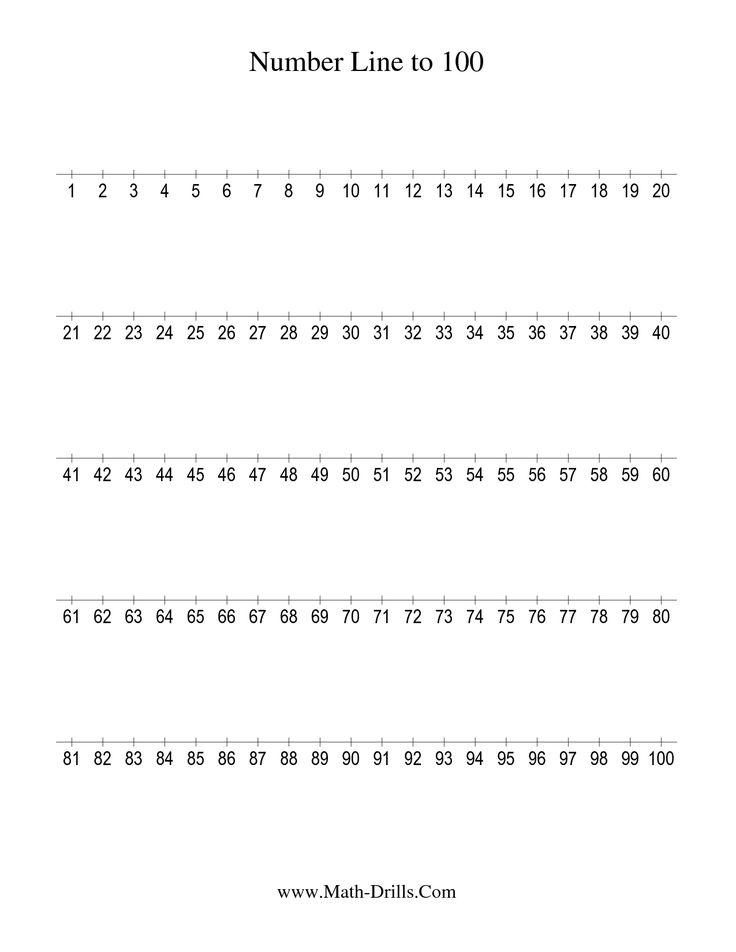
Numbers to 5 for Kindergarten
Numbers to 10 for Kindergarten
Numbers 11-20 for Kindergarten
Mindful Math Kindergarten Curriculum
Numbers to 10 for First Grade
Numbers to 20 for First Grade
Mindful Math First Grade Curriculum
Free Number Sense Puzzles to 20
Prep this fun set of number puzzles for your classroom centers and help kids build their number sense!
Grab 20 FREE number puzzles with different representations on each piece by clicking the image below.
Number Sense Books
I Spy Numbers by Jean Marzollo
1,2,3 Peas by Keith Baker
ChickaChicka 1,2,3 by Bill Martin Jr.
Splash! by Ann Jonas (counting)
The Very Hungry Caterpillar by Eric Carle (counting)
How Do Dinosaurs Count to 10? by Jane Yolen
Number Everywhere by Elliot Kaufman
10 Black Dots by Donald Crews
How Many Bugs in a Box? by David Carter
How Many Snails? by Paul Giganti, Jr.
Ten Sly Piranhas by William Wise
None the Number by Oliver Jeffers
Zero the Hero by Joan Holub
More or Less by Stuart Murphy
Tally O’Mally by Stuart Murphy
Place Value by David Adler
What’s the Place Value by Shirley Duke
Quack and Count by Keith Baker
Betcha! by Stuart J. Murphy
Number Sense Materials
Try the Mindful Math Comprehensive Program
Read about the Mindful Math program and how it can change your math block in positive ways! This comprehensive math curriculum is available for Kindergarten, first grade, and second grade.
Learn More about Teaching Number Sense
Building Number Sense free email series
Number of the Day Activities
5 Tips for Building Math Fluency
PIN
FREE Number Sense Email Series
Sign up for the building number sense email series filled with effective strategies, must try activities, and FREE resources to build routines in your classroom. Everything you need to help kids grow their number sense and have fun at the same time!
Everything you need to help kids grow their number sense and have fun at the same time!
First Name
Personal Email Address
We use this field to detect spam bots. If you fill this in, you will be marked as a spammer.
I'd like to receive the free email course.
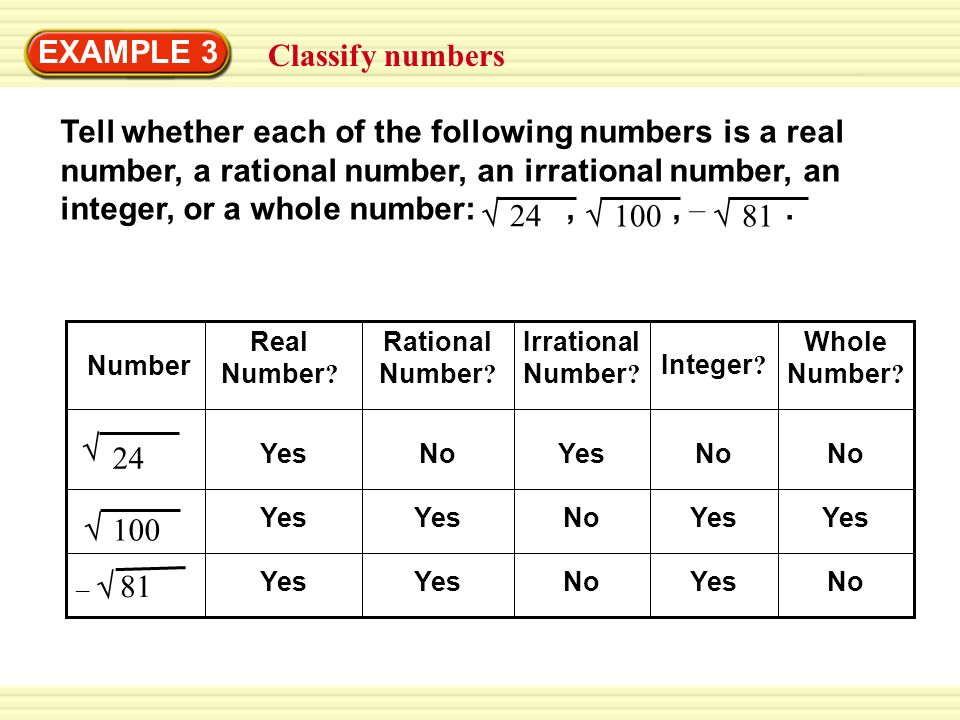
This form collects information I will use to send weekly emails with strategies, promotions, and resources. Unsubscribe at any time. Powered by ConvertKit| Main page CATEGORIES: Archeology TOP 10 on the site Preparation of disinfectant solutions of various concentrations Technique of the lower direct ball delivery. Franco-Prussian war (causes and consequences) Organization of the work of the treatment room Semantic and mechanical memorization, their place and role in the assimilation of knowledge Communication barriers and ways to overcome them Processing of reusable medical devices Samples of journalistic style text Four types of rebalancing Problems with answers for the All-Russian Olympiad in Law We will help you write your papers! DID YOU KNOW? The influence of society on a person Preparation of disinfectant solutions of various concentrations Practical work in geography for the 6th grade Organization of the work of the treatment room Changes in inanimate nature in autumn Treatment room cleaning Solfeggio. All rules for solfeggio Beam systems. | ⇐ PreviousPage 11 of 13Next ⇒ A numeric or arithmetic expression has no meaning if it cannot be evaluated.
122. Give an example of a numerical expression that makes sense and does not make sense. The expression makes sense (can be calculated) but the numeric expression does not make sense (cannot be calculated because the denominator is zero) What is an expression with variables or an algebraic expression? An expression with variables or an algebraic expression is a record consisting of numbers, letters, signs of arithmetic operations and brackets, composed in such a way that it has a computational meaning, if letters mean some numbers.
What is the value of an expression with variables or an algebraic expression given the values of the variables? The value of an expression with variables or an algebraic expression with given values of variables is the value of a numeric expression obtained after replacing variables with given numerical values.
What are the valid values of a variable expression or algebraic expression? Valid variable values in a variable expression are those variable values for which the corresponding numeric expression makes sense.
126. Give an example of an expression with variables that makes sense for any values of the variables The following expression with variables makes sense for any values of the variables: . It can be calculated for any values of a and b 127. Give an example of an expression with variables that does not make sense for any values of the variables. The expression cannot be evaluated for any values of the variables a and b. 128. Give an example of an expression with variables that does not make sense for all values of the variables. The expression can be evaluated for all values of the variables, except those for which a = b. ----------------------------------------------- -------------------------------------------------- ------
Which expressions with variables or algebraic expressions are called rational integers? An expression with variables is called an integer rational expression if it is composed of numbers, letters, brackets and all arithmetic operations except division by expressions containing variables (division by a number is allowed).
Which expressions with variables or algebraic expressions are called fractional rationals? Fractional rational expressions are such expressions with variables in which there is a division by an expression with variables that necessarily contains at least one variable.
⇐ Previous45678910111213Next ⇒ See also: Running long jump technique Organization of the procedure room Applications of synchronous machines Wiener and Kalman optimization |
| This page was last modified: 2016-12-11; views: 1639; Page copyright infringement; We will help you write your paper! infopedia. |
Numerical expressions - formulas, examples and solution algorithm in Grade 7 » Kupuk.net
Numerical expressions are the basis of mathematics. In grade 7, they are studied in more detail, since special techniques are applied to them, allowing them to perform simplification operations. The latter are used to optimize calculations in solving problems in various disciplines with a physical and mathematical bias. However, experts recommend studying the theory, which will help to avoid many errors in the calculation of quantities.
General information
Many novice mathematicians often confuse two concepts: numerical and algebraic expressions. There is a difference between them, which lies in the definitions. A numeric expression is a kind of mathematical identity consisting only of numbers, parentheses, and signs of arithmetic operations. For example, the identity "5+8/3−4*2" is a numeric expression. It means a sequence of mathematical signs combined into one logical chain.
For example, the identity "5+8/3−4*2" is a numeric expression. It means a sequence of mathematical signs combined into one logical chain.
Algebraic is a set of variables and numeric expressions that have a logical conclusion. The explanation of the meaning of the logic of the expression is as follows: a set of numbers and variables interconnected by the arithmetic operations of multiplication, division, addition and subtraction. For example, the expression "5t-2/3" is algebraic because it contains the variable "t".
A mathematical expression is not a set of characters that does not have a logical conclusion. For example, 234±4678++* is an ordinary series that can be made up of numbers and signs of arithmetic operations. The latter have the following designations:
A product is a type of arithmetic operation that allows you to multiply one value by another. It consists of three main elements. These include: I multiplier, II multiplier and product (result). Mathematicians say that multiplication is used to reduce addition, that is, 3+3+3+3+3+3=3*6=18. If both expressions are calculated, they will be equal to each other.
It consists of three main elements. These include: I multiplier, II multiplier and product (result). Mathematicians say that multiplication is used to reduce addition, that is, 3+3+3+3+3+3=3*6=18. If both expressions are calculated, they will be equal to each other.
Division is an arithmetic operation used to find the factors of the desired number. It consists of the following mandatory components: dividend, divisor and quotient. The first element is a composite value, the second is one of the factors of the first, and the quotient is the result of the division operation.
Addition is the simplest arithmetic operation, the compilation of which is carried out from at least three elements and allows you to increase the desired value by a certain value. The components have the following names: two terms and the result, which is called the sum.
Subtraction is an operation necessary to reduce the desired number by a given value. It has three components: minuend, subtrahend, and difference. The first is the numerical value from which the subtrahend is subtracted.
The first is the numerical value from which the subtrahend is subtracted.
However, each operation has a certain priority.
Operation precedence
When calculating mathematical expressions, there is a certain priority for arithmetic operations. Multiplication and division are performed first. They have the highest priority value. Sometimes, to optimize calculations, you can perform an operation on numbers or variables in any order, i.e. example "2*26/13" can be solved in two ways:
In the first case, the operation will take more resources: first, you need to multiply 2 by 26, calculate the result, and then divide it by 13. This is not very convenient. However, to optimize calculations, it is recommended to use the second method, since it is not difficult to divide 26 by 13, and then multiply the result with two.
Addition and subtraction also have the same priority level. You can first, for convenience, perform addition and then subtraction, or vice versa. Experts recommend following an important principle: calculations should be simplified as much as possible. To give priority to any mathematical action, you need to take part of the expression in brackets (group). As a result, the operation in parentheses will be executed first.
For example, you need to find the value of the expression: 2*2−2 (3−2)*7/14−25/5. Solve it correctly according to this method, taking into account the priority:
If you do not take into account the priority of operations, then you can find the value of a numeric expression using the following scheme:
 8.
8. If you compare two results, they do not match. Based on this, we can conclude that priority matters when performing calculations and cannot be violated, since the logic of the expression will disappear. However, not only brackets allow you to set the order of operations. There are some exceptions.
Special cases or exceptions
In algebra, as in all disciplines with a physical and mathematical bias, the speed of calculations is taken into account. This significantly affects the execution time of any task. In some cases, the expression can be simplified by using short multiplication formulas and performing mathematical transformations with identity elements. For these purposes, it is recommended to use the appropriate rules:

It should be noted that in the first case it is required to check if the denominator is equal to zero. To do this, specify the value of the variable, which should not turn the denominator into 0.
Calculation technique
Mathematicians have developed a special technique for finding the value of an expression. It boils down to breaking a numerical expression into parts. This approach was first used by Pythagoras. Its essence is as follows:
To demonstrate the algorithm, you need to solve the example: 9*7−21 (74/(43+31))/7−64-(27−3*9).

 Determination of support reactions and pinching moments
Determination of support reactions and pinching moments 

 su All materials presented on the site are for informational purposes only and do not pursue commercial purposes or copyright infringement. Feedback - 176.57.188.101 (0.004 s.)
su All materials presented on the site are for informational purposes only and do not pursue commercial purposes or copyright infringement. Feedback - 176.57.188.101 (0.004 s.)