Examples of mathematical concepts
Math Concept List, Uses & Examples | What is a Math Concept? - Video & Lesson Transcript
English Courses / PSAT Prep: Tutoring Solution Course / PSAT Math - Numbers and Operations: Tutoring Solution Chapter
Anita Dunn, Yuanxin (Amy) Yang Alcocer- Author Anita Dunn
Anita Dunn graduated from Saint Mary's College with a Bachelor's of Science in Mathematics, and graduated from Purdue University with a Master's of Science in Mathematics. She has been certified as a Developmental Education Specialist through the Kellogg Institute. She has more than 10 years of experience as a college professor.
View bio - Instructor Yuanxin (Amy) Yang Alcocer
Amy has a master's degree in secondary education and has been teaching math for over 9 years.
View bioAmy has worked with students at all levels from those with special needs to those that are gifted.
Learn about math concepts and understand how they are applied. Compare math concepts and math facts and study math concepts examples in addition and division. Updated: 07/15/2022
Table of Contents
- What is a Math Concept?
- What is a Math Fact?
- Math Concepts vs. Math Facts
- Using Math Concepts: Examples
- Lesson Summary
What is a Math Concept?
A concept is defined as an abstract idea. Therefore, a math concept is a generalized mathematical idea. Math concepts are things like the ideas of counting, addition, and multiplication.
Basic mathematical concepts have a long history. Counting was created long ago, and there are many examples of ancient groups of people keeping count of various things and keeping records. The numerical system used has varied over time, from pictographs being used by the Ancient Egyptians to roman numerals being used by the Ancient Romans. But our current, base 10 positional system called the Hindu-Arabic system makes basic mathematical concepts such as addition and multiplication much easier to figure out.
The numerical system used has varied over time, from pictographs being used by the Ancient Egyptians to roman numerals being used by the Ancient Romans. But our current, base 10 positional system called the Hindu-Arabic system makes basic mathematical concepts such as addition and multiplication much easier to figure out.
List of Math Concepts
Basic mathematical concepts include:
- Counting. Note that this can include both regular counting, such as 1, 2, 3, 4, 5..., and counting using different increments, such as 0.5, 1, 1.5, 2,.....
- Addition
- Subtraction
- Multiplication
- Division
An error occurred trying to load this video.
Try refreshing the page, or contact customer support.
You must cCreate an account to continue watching
Register to view this lesson
Are you a student or a teacher?
Create Your Account To Continue Watching
As a member, you'll also get unlimited access to over 84,000 lessons in math, English, science, history, and more. Plus, get practice tests, quizzes, and personalized coaching to help you succeed.
Plus, get practice tests, quizzes, and personalized coaching to help you succeed.
Get unlimited access to over 84,000 lessons.
Try it now
It only takes a few minutes to setup and you can cancel any time.
Already registered? Log in here for access
Back
Resources created by teachers for teachers
Over 30,000 video lessons & teaching resources‐all in one place.
Video lessons
Quizzes & Worksheets
Classroom Integration
Lesson Plans
I would definitely recommend Study.com to my colleagues. It’s like a teacher waved a magic wand and did the work for me. I feel like it’s a lifeline.
Jennifer B.
Teacher
Try it now
Back
Coming up next: What is a Mathematical Expression? - Definition & Examples
Take Quiz Watch Next Lesson
Replay
Just checking in.
 Are you still watching? Yes! Keep playing.
Are you still watching? Yes! Keep playing. Your next lesson will play in 10 seconds
- 0:00 Definition Of Math Concept
- 0:40 Math Fact
- 1:15 Math Concept VS Math Fact
- 2:35 Using Math Concepts
- 4:10 Lesson Summary
Save Save Save
Timeline
Autoplay
Autoplay
Speed
Speed
What is a Math Fact?
A math fact is a specific or group of specific facts. For example, {eq}2+2=4 {/eq} is a math fact. The multiplication table is made up of a group of math facts, and the fact that {eq}3*4=12 {/eq} is a math fact, where {eq}* {/eq} is a notation that is used for multiplication.
For example, {eq}2+2=4 {/eq} is a math fact. The multiplication table is made up of a group of math facts, and the fact that {eq}3*4=12 {/eq} is a math fact, where {eq}* {/eq} is a notation that is used for multiplication.
Math Concepts vs. Math Facts
While the terms math concepts and math fact are often used interchangeably, they are not exactly the same thing.
The idea that addition is the process of taking two quantities and putting them together is a math concept, while the fact that {eq}1+5=6 {/eq} is a math fact.
The idea that multiplication is the process of repeated addition of the same number over and over again is a math concept, while the fact that {eq}4*6=24 {/eq} is a math fact.
Using Math Concepts: Examples
Understanding mathematical concepts is important to learning and understanding mathematics. Memorizing a multiplication table is great, but understanding that multiplication is repeated addition, and that division is the inverse operation of multiplication, makes it easier to understand multiplication as a whole. It also helps when a math fact is forgotten. For example, if someone forgets that {eq}3*5=15 {/eq}, they could remember that this could be translated as {eq}3*5=3+3+3+3+3=15 {/eq}. Math concepts also build on one another. Understanding the concept of counting is going to be fundamental to understanding the concept of addition, and understanding the concept of addition will need to come before understanding multiplication.
It also helps when a math fact is forgotten. For example, if someone forgets that {eq}3*5=15 {/eq}, they could remember that this could be translated as {eq}3*5=3+3+3+3+3=15 {/eq}. Math concepts also build on one another. Understanding the concept of counting is going to be fundamental to understanding the concept of addition, and understanding the concept of addition will need to come before understanding multiplication.
Counting
Counting is one math concept that is taught to very young children. But, it also important for kids to go from just memorizing 1, 2, 3, 4... and recognizing that the number 3 can be connected to the idea that there are 3 of some sort of object.
Later, students can understand that to get from one number to the next, 1 is being added to the previous number. Thus, after counting 1, 2, 3, the next number is 3+1=4. But counting doesn't always have to be done in this traditional way. Perhaps a fabric store wants to sell fabric in half yard increments. Their website will then need to have quantities that represent this idea. So instead of 1, 2, 3.. being the choices a customer can choose from, the quantities would need to be 0.5, 1.0, 1.5, 2.0..... If the minimum fabric cut is 1 yard, then the choices could be 1.0, 1.5, 2.0.... Thus, the concept of counting can be used in various different real world situations, and can be done using different increments and starting at different starting numbers.
Their website will then need to have quantities that represent this idea. So instead of 1, 2, 3.. being the choices a customer can choose from, the quantities would need to be 0.5, 1.0, 1.5, 2.0..... If the minimum fabric cut is 1 yard, then the choices could be 1.0, 1.5, 2.0.... Thus, the concept of counting can be used in various different real world situations, and can be done using different increments and starting at different starting numbers.
Addition
Now that the concept of counting is understood, the concept of addition can be explored. As mentioned previously, a number can be thought of as a quantity of some object. Thinking about it in this way, consider someone counting blocks. They have two blocks, and then they add on three more blocks. Now, they have 5 blocks in front of them. Thus, {eq}2+3=5 {/eq}. Remember, {eq}2+3=5 {/eq} is a math fact, while the idea that addition is the process of taking two quantities and putting them together is a math concept.
Multiplication
Multiplication builds on the concept of addition.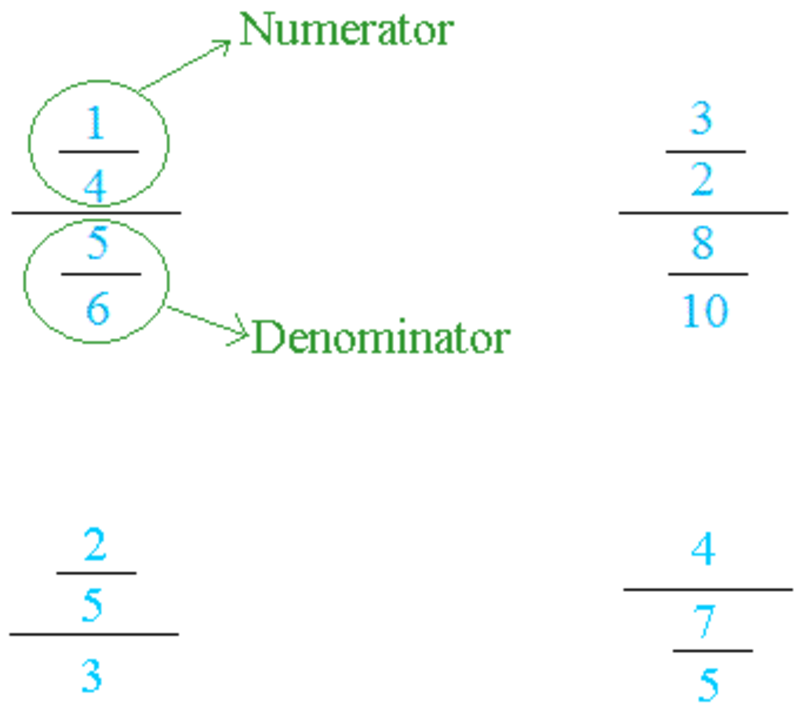 Sometimes, addition of the same number needs to be repeated. For example, if a parent has three kids, and wants to give each child $5 to go to the fair, then they could add {eq}5+5+5 {/eq} to find that they need to have a total of $15. But what if there is a teacher with 20 students, and the teacher wants to give each student 5 pencils? That teacher is not going to want to add 5 to itself over and over again 20 times. In this case, the concept of multiplication will save the teacher quite a bit of time, as adding 5 to itself 20 times is the same as {eq}5*20 {/eq}. If the teacher knows his or her math facts, then they will know that {eq}5*20=100 {/eq}, and so they will need 100 pencils to give to their students.
Sometimes, addition of the same number needs to be repeated. For example, if a parent has three kids, and wants to give each child $5 to go to the fair, then they could add {eq}5+5+5 {/eq} to find that they need to have a total of $15. But what if there is a teacher with 20 students, and the teacher wants to give each student 5 pencils? That teacher is not going to want to add 5 to itself over and over again 20 times. In this case, the concept of multiplication will save the teacher quite a bit of time, as adding 5 to itself 20 times is the same as {eq}5*20 {/eq}. If the teacher knows his or her math facts, then they will know that {eq}5*20=100 {/eq}, and so they will need 100 pencils to give to their students.
Division
Meanwhile, division is the idea that sometimes multiplication needs to be "undone". In other words, rather than putting a bunch of things together, division means splitting up a whole amount of something into equal bits. For example, if the teacher mentioned previously has 140 pencils and wants to evenly distribute them amongst their 20 students, what they need to know is what number times twenty yields one hundred and forty, or in math symbols: {eq}x*20=140 {/eq}, where x is the number of pencils each student will receive. Division allows this number to be found, because it shows that this situation can be rethought of as {eq}140 \div 20 = x {/eq}. If the teacher knows his or her math facts, they will know that {eq}140 \div 20 = 7 {/eq}, and thus each student can receive 7 pencils. In this case, one could say that 140 is divided evenly by 20 because there are no pencils remaining after they are evenly divided amongst the students. Thus, a number is evenly divided by another number if there is no remainder.
Division allows this number to be found, because it shows that this situation can be rethought of as {eq}140 \div 20 = x {/eq}. If the teacher knows his or her math facts, they will know that {eq}140 \div 20 = 7 {/eq}, and thus each student can receive 7 pencils. In this case, one could say that 140 is divided evenly by 20 because there are no pencils remaining after they are evenly divided amongst the students. Thus, a number is evenly divided by another number if there is no remainder.
Lesson Summary
A math concept is a general mathematical idea. In contrast, a math fact is a specific mathematical fact. Thus, a math concept is more general than a math fact. There are countless math concepts, but some basic ones include things like counting and addition. The mathematical concept of addition is the idea that addition is taking two numbers and combining them together. A math fact is a specific fact such as {eq}2+2=4 {/eq}.
To unlock this lesson you must be a Study. com Member.
com Member.
Create your account
What is an example of a concept in mathematics?
A concept is a general idea rather than a specific fact. So in mathematics, one example of a concept is the idea that addition is putting two sets of things together.
What are basic math concepts?
A math concept is an underlying mathematical idea. Things like addition, multiplication, counting, and equality are some basic math concepts.
Register to view this lesson
Are you a student or a teacher?
Unlock Your Education
See for yourself why 30 million people use Study.com
Become a Study.com member and start learning now.
Become a Member
Already a member? Log In
Back
Resources created by teachers for teachers
Over 30,000 video lessons & teaching resources‐all in one place.
Video lessons
Quizzes & Worksheets
Classroom Integration
Lesson Plans
I would definitely recommend Study.com to my colleagues. It’s like a teacher waved a magic wand and did the work for me. I feel like it’s a lifeline.
Jennifer B.
Teacher
Try it now
Back
Explore our library of over 84,000 lessons
Search
Browse
Browse by subject
Enrolling in a course lets you earn progress by passing quizzes and exams.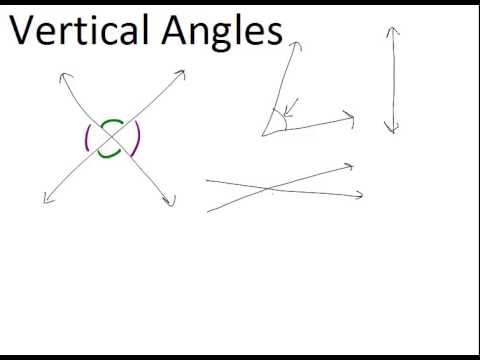
Track course progress
Take quizzes and exams
Earn certificates of completion
You will also be able to:
- Create a Goal
- Create custom courses
- Get your questions answered
Upgrade to Premium to add all these features to your account!
Upgrade Now
Upgrade to Premium to add all these features to your account!
What is a Math Concept? - Video & Lesson Transcript
English Courses / PSAT Prep: Tutoring Solution Course / PSAT Math - Numbers and Operations: Tutoring Solution Chapter
Instructor: Yuanxin (Amy) Yang AlcocerShow bio
Amy has a master's degree in secondary education and has been teaching math for over 9 years. Amy has worked with students at all levels from those with special needs to those that are gifted.
Amy has worked with students at all levels from those with special needs to those that are gifted.
Just like a concept in any other discipline, a math concept looks at the big idea, rather than something concrete to be memorized. Learn all about math concepts, including how they can be applied in practice and how they contrast with math facts. Updated: 10/12/2021
Definition of Math Concept
A math concept is the 'why' or 'big idea' of math. Knowing a math concept means you know the workings behind the answer. You know why you got the answer you got and you don't have to memorize answers or formulas to figure them out. Because you know why things work, you can figure out the answers and formulas yourself. You understand answers and formulas better and can tell when something isn't quite right.
When you understand a math concept, you have essentially reached an upper tier in math that allows you to think and process abstractly. This takes us to the difference between a math concept and a math fact.
This takes us to the difference between a math concept and a math fact.
An error occurred trying to load this video.
Try refreshing the page, or contact customer support.
You must cCreate an account to continue watching
Register to view this lesson
Are you a student or a teacher?
Create Your Account To Continue Watching
As a member, you'll also get unlimited access to over 84,000 lessons in math, English, science, history, and more. Plus, get practice tests, quizzes, and personalized coaching to help you succeed.
Get unlimited access to over 84,000 lessons.
Try it now
It only takes a few minutes to setup and you can cancel any time.
Already registered? Log in here for access
Back
Resources created by teachers for teachers
Over 30,000 video lessons & teaching resources‐all in one place.
Video lessons
Quizzes & Worksheets
Classroom Integration
Lesson Plans
I would definitely recommend Study.com to my colleagues. It’s like a teacher waved a magic wand and did the work for me. I feel like it’s a lifeline.
Jennifer B.
Teacher
Try it now
Back
Coming up next: What is a Mathematical Expression? - Definition & Examples
Take Quiz Watch Next Lesson
Replay
Just checking in. Are you still watching?
Yes! Keep playing.Your next lesson will play in 10 seconds
- 0:00 Definition Of Math Concept
- 0:40 Math Fact
- 1:15 Math Concept VS Math Fact
- 2:35 Using Math Concepts
- 4:10 Lesson Summary
Save Save Save
Timeline
Autoplay
Autoplay
Speed
Speed
Math Fact
A math fact is something that needs to be memorized or written down. For example, the multiplication and addition tables are math facts because they tell you that 1 + 1 = 2 and that 2 * 2 = 4. There are no ifs, ands, or buts about them.
For example, the multiplication and addition tables are math facts because they tell you that 1 + 1 = 2 and that 2 * 2 = 4. There are no ifs, ands, or buts about them.
Knowing a math fact allows you to recall the information when you need it, like for a test. However, if you were given a problem that is similar but uses different numbers or arrangements, then you wouldn't be able to do the problem because you only know the fact and not the concept behind it. You don't know how the problem works so you can't solve it because the facts you know don't include that specific problem.
Math Concept vs. Math Fact
Let's compare a few math concepts and math facts.
- Counting. The math concept for counting tells you that you start at a number and incrementally go up. You can choose your increment to suit your needs. The math fact for counting gives you the number line of 1, 2, 3, 4, etc.
- Addition. The math concept of addition tells you to gather 2 quantities or numbers together and get their total.
 The math fact is the additional table that tells you 1 + 1 = 2, 1 + 2 = 3, etc.
The math fact is the additional table that tells you 1 + 1 = 2, 1 + 2 = 3, etc. - Multiplication. The math concept of multiplication tells you to get the total of a certain number or quantity that has been copied or cloned so many times. The math fact is the multiplication table.
- Division. The math concept tells you that to divide means to split fairly. The math fact is the division table or the reverse multiplication table.
The math concept is more general than the math fact. Do you see how the math concept can be applied in so many more areas than the math fact? The concept allows you to solve so many more problems than just the math fact. One math fact lets you solve one specific type of problem, but one math concept covers a whole range of like problems.
Using Math Concepts
Let's see how these math concepts can be used.
- Counting. If you know the math concept of counting, you can count anything. You can count whole numbers, decimals, by twos, by fives, by tens, etc.
 Using this math concept, you can count 1.1, 1.2, 1.3, 1.4, or 5, 10, 15, 20, etc.
Using this math concept, you can count 1.1, 1.2, 1.3, 1.4, or 5, 10, 15, 20, etc. - Addition. You can use this math concept to add any 2 quantities together. You could add 236 + 2 together to get 238 and you can add 2.1 + 3.5 to get 5.6. You understand that adding means combining the two quantities to get the total.
- Multiplication. Just knowing the multiplication table limits you to just those numbers. Understanding the math concept allows you to do problems such as 21 * 3 to get 63. You understand that this problem is telling you to get the total of 3 bunches of 21.
- Division. Because you understand the sharing principle behind division, you can divide anything. You can divide problems that don't split nicely, such as 1 divided by 4. You understand the problem to mean dividing a whole of something. If you only had one pie, how would you split it among 4 people? You would realize that 1 divided by 4 gives a result of a quarter, or 0.
 25.
25.
Lesson Summary
Math concepts give you the 'big idea' of math. They are general and can be applied to a range of problems. Math facts, on the other hand, limit you to very specific problems. Applying math concepts makes your problem solving easier.
Vocabulary & Definitions
Math concepts: Math concepts are how math works, in general.
Math facts: Math facts need to be memorized or written down.
Learning Outcome
After viewing this lesson, you should be able to summarize the meaning and general application of math concepts.
To unlock this lesson you must be a Study.com Member.
Create your account
Register to view this lesson
Are you a student or a teacher?
Unlock Your Education
See for yourself why 30 million people use Study.
 com
comBecome a Study.com member and start learning now.
Become a Member
Already a member? Log In
Back
Resources created by teachers for teachers
Over 30,000 video lessons & teaching resources‐all in one place.
Video lessons
Quizzes & Worksheets
Classroom Integration
Lesson Plans
I would definitely recommend Study.com to my colleagues. It’s like a teacher waved a magic wand and did the work for me. I feel like it’s a lifeline.
Jennifer B.
Teacher
Try it now
Back
Mathematical concepts | Educational and methodological material on mathematics on the topic:
Slide 1
Methods of mathematics Primary school teacher: Anikina Tatyana Yurievna
Slide 2
Mathematical concepts Concepts, their scope and content. The relationship of genus and species between concepts. Explicit and implicit definitions of concepts. Algorithm for solving the problem of recognition using the definition through the genus and specific difference. Examples of explicit and implicit definitions of concepts.
The relationship of genus and species between concepts. Explicit and implicit definitions of concepts. Algorithm for solving the problem of recognition using the definition through the genus and specific difference. Examples of explicit and implicit definitions of concepts.
Slide 3
Concepts, their scope and content Concepts related to numbers and operations on them: number; addition; term; more. Algebraic concepts: expression; the equation; equality. Geometric concepts: segment; straight; triangle. Quantities and their measurement: centimeter; meter; kilometer.
Slide 4
The scope of a concept is the set of all objects denoted by one term. is the set of all essential properties of the object reflected in this concept. Content of concept
Slide 5
Relationship Scope of a concept Content of a concept sets. ! If A with B (A=/ B), then a is specific in relation to the concept b, and the concept b is generic in relation to the concept a. If A = B, then the concepts a and b are identical. If the sets A and B are not connected by an inclusion relation, the concepts a and b are not in relation to the genus and species and are not identical.
If the sets A and B are not connected by an inclusion relation, the concepts a and b are not in relation to the genus and species and are not identical.
Slide 7
Properties: 1) The concepts of genus and species are relative. 2) For a given concept, it is often possible to specify several generic concepts. 3) A species concept has all the properties of a generic concept. and implicit definitions of concepts Defined concept Generic concept Specific difference Defining concept + A definition is usually a sentence that explains the essence of a new term (or designation). !
Slide 10
Remember!!! 3. The definition should be clear 2. There should be no vicious circle in the definition (or their system) 4. The same concept can be defined in different ways. 1. The definition must be proportionate
Slide 11
Algorithm: Name the concept (term) being defined. 2. Indicate the closest generic (in relation to the defined) concept. 3. List the properties that distinguish the defined objects from the scope of the generic, i. e. state the difference. 4. Check whether the rules for defining the concept are met (is it proportionate, is there a vicious circle, etc.)
e. state the difference. 4. Check whether the rules for defining the concept are met (is it proportionate, is there a vicious circle, etc.)
Slide 12
Explicit definitions Have the form of equality, coincidence of two concepts. The general scheme of such definitions is: "A is (by definition) B". Here A and B are two concepts, and it does not matter whether each of them is expressed by one word or a combination of words. Geometry is the science of the properties of geometric shapes. A molecule is the smallest particle of a substance that retains all the chemical properties of this substance. This definition is by display. They are used to introduce terms, by demonstrating the objects that these terms designate.
Slide 14
X + 6 = 15 is an equation An example of a contextual definition can be the definition of an equation and its solution given in a mathematics textbook for grade II (Moro M.I., Bantova M.A. Mathematics: Textbook for 2 class of a four-year elementary school. ) Here, after writing + 6 \u003d 15 and a list of numbers 0,5,9,10, there is a text: “To what number must 6 be added to get 15? We denote the unknown number with the Latin letter X (x): To solve an equation means to find an unknown number. In this equation, the unknown number is 9, since 9+6=15. "Explain why the numbers 0.5 and 10 are not appropriate."
) Here, after writing + 6 \u003d 15 and a list of numbers 0,5,9,10, there is a text: “To what number must 6 be added to get 15? We denote the unknown number with the Latin letter X (x): To solve an equation means to find an unknown number. In this equation, the unknown number is 9, since 9+6=15. "Explain why the numbers 0.5 and 10 are not appropriate."
Slide 15
2*7 >2*6 is inequality 17-5=8+4 is equality inequalities) from other sentences, it does not indicate the properties characteristic of these concepts. They only associate terms with the objects they define.
Slide 16
Geometric shapes Definition of a segment, ray, angle, polyline. The main properties of these figures. The content of these concepts in elementary education in mathematics; types of definitions. Task examples.
Slide 17
Definition of a segment, ray, angle, polyline A segment is a straight line passing through two points. is a system of two points A and B belonging to the line a. The points located between A and B are called the points lying inside the segment AB, the points A and B are called the ends of the segment AB. - A B is a part of a straight line, which consists of all points of this straight line lying between two given points of it. These points are called the ends of the segment.
The points located between A and B are called the points lying inside the segment AB, the points A and B are called the ends of the segment AB. - A B is a part of a straight line, which consists of all points of this straight line lying between two given points of it. These points are called the ends of the segment.
Slide 18
Main property: Of the three points on a line, one and only one lies between the other two. Two points are enough to draw a straight line.
Slide 19
A ray is a part of a straight line that has a beginning but no end. - The beam is limited on one side and can be extended in a straight line only in one direction, as far as desired. - A ray with origin O is a set of all points of a straight line lying on one side of O.
Slide 20
О A ray is a part of a straight line, which consists of all points of this straight line, lying on one side of its given point. This point is called the starting point of the beam.
Slide 21
An angle is a figure formed by two rays with the same origin. A B The rays forming an angle are called the sides of the angle, and their common beginning is the apex of the angle. An acute angle is an angle that is less than a right angle. An obtuse angle is an angle that is greater than a right angle. A right angle equal to 90 is a set of two rays with a common origin, lying on different lines. - - From
A B The rays forming an angle are called the sides of the angle, and their common beginning is the apex of the angle. An acute angle is an angle that is less than a right angle. An obtuse angle is an angle that is greater than a right angle. A right angle equal to 90 is a set of two rays with a common origin, lying on different lines. - - From
Slide 22
Basic properties: Each angle has a certain degree measure greater than zero. A straight angle is 180. The degree measure of an angle is equal to the sum of the degree measures of the angles into which it is divided by any ray passing between its sides.
Slide 23
Polyline - B A C D E Polyline ABCDEG . Points A, B, C, D, E, G are the vertices of the polyline. The segments AB, BC, CD, DE, EG are the links of the broken line. G is a figure that consists of points A1, A2,……A n and segments A1A2, A2A3,…..A n A n connecting them.
Slide 24
Main property: The length of the polyline is not less than the length of the segment connecting its ends. The length of a broken line is the sum of the lengths of its links.
The length of a broken line is the sum of the lengths of its links.
Slide 25
Examples of tasks What is the broken line that has the most links? Less links? Which polyline has 3 vertices? 4 peaks? How to find out which segment is larger?
Slide 26
How can you draw a right angle on unlined paper? How to make 4 right angles with just two segments? And now 2 sharp and 2 blunt?
Slide 27
Rectangle A quadrilateral with all right angles. - is a parallelogram with all right angles. B A C D
Slide 28
Diagonals of a rectangle are equal Opposite sides are equal Sides do not intersect Diagonals intersect and the point of intersection is bisected
Slide 29
A square - - - - is a rectangle whose adjacent sides are equal. is a rectangle whose diagonals are mutually perpendicular and equal. This is a rhombus that has a right angle. It is a parallelogram in which all sides are equal and the angles are right. B A C D
Slide 30
After drawing the diagonals, we get equal triangles. The diagonals are equal. The diagonals intersect and the intersection point is bisected. The sides do not intersect. Diagonals intersect at right angles.
The diagonals are equal. The diagonals intersect and the intersection point is bisected. The sides do not intersect. Diagonals intersect at right angles.
Slide 31
Example tasks Is this figure a rectangle? Why? How to shift the sticks so that you get 3 identical squares? Which figure has the largest area? Why? Do the calculations.
Formation of mathematical concepts in elementary school
Author: Grebennikova Anna Andreevna
Formation of mathematical concepts in elementary school
Abstract: The article discusses various ideas on the formation of scientific concepts and their influence on modern ideas about the formation of the concept of number in primary school students.
Keywords: concept, number concept, generalization, various methodological approaches.
When acquiring scientific knowledge, elementary school students encounter different types of concepts. The student's inability to differentiate concepts leads to their inadequate assimilation.
Logic in concepts distinguishes scope and content. The volume is understood as the class of objects that belong to this concept, are united by it. So, the scope of the concept of a triangle includes the entire set of triangles, regardless of their specific characteristics (types of angles, size of sides, etc.).
The content of concepts includes that system of important properties due to which the process of uniting these objects into one class takes place. In order to reveal the essence of a concept, it is necessary, by means of comparison, to identify what signs will be sufficient to highlight its relationship to other objects. Until the moment when the signs are established, as well as the content, we will not be clear about the essence of the subject that is reflected by this concept. It will definitely be very difficult to separate such an object from adjacent objects, there will be some confusion.
If, for example, we talk about the concept of a triangle, then the properties can be attributed to such as a closed figure, consisting of three line segments. The set of properties, according to which objects are combined into one class, is usually called sufficient features or necessary features. In some concepts, these features can complement each other, thereby forming a content that allows you to combine objects into one class. An example of such concepts is a triangle, an angle, a bisector, and many others.
The set of properties, according to which objects are combined into one class, is usually called sufficient features or necessary features. In some concepts, these features can complement each other, thereby forming a content that allows you to combine objects into one class. An example of such concepts is a triangle, an angle, a bisector, and many others.
The set of such objects to which this concept will apply is a logical class of objects, which, in turn, is a collection of objects that have common features, and therefore are defined by a common concept. Concepts can be divided into types according to content, volume. It all depends on the nature and number of objects to which they are distributed. If we take the scope of a concept as a criterion, then it should be said here that concepts are divided into general and singular. For example, if the scope of a concept includes one object, then such a concept is called a single one. If we talk about the content, then the concepts can be divided into conjunctive and disjunctive, absolute and concrete, irrelative and relative. Conjunctive should be understood as such concepts that are interconnected by their features, that is, it is not possible to recognize an object of this class by individual features. For example, objects related to the concept of a triangle must necessarily consist of three line segments and be closed.
Conjunctive should be understood as such concepts that are interconnected by their features, that is, it is not possible to recognize an object of this class by individual features. For example, objects related to the concept of a triangle must necessarily consist of three line segments and be closed.
In other concepts, the relationship between necessary and sufficient features is different: they do not complement each other, but replace. This means that one feature is equivalent to the other. Such a connection of attributes is called disjunction, and the concepts are respectively called disjunctive. It is also important to take into account the division of concepts into absolute and relative. Absolute concepts can unite objects into classes, according to some features that characterize the essence of these objects. Relative concepts, on the other hand, set themselves the task of uniting them into classes according to properties that characterize their relationship to other objects.
Relative concepts cause more serious difficulties for students than absolute concepts. The essence of the difficulties lies precisely in the fact that schoolchildren do not take into account the relativity of concepts and operate with them as with absolute concepts.
In elementary school, each concept is initially introduced through visualization, through observation of specific objects, or through practical operation. In this case, the teacher is trying to build on the knowledge and experience of the children that they received early, perhaps even at preschool age. The teacher fixes acquaintance with the concepts of mathematics with the help of terms or symbols. But this does not mean that various types of definitions are not used at this stage of the work. The definition of a concept is a listing of all the important features of objects that are components of this concept. The definition of a concept by a word will be called a term, for example, “circle”, triangle. The tasks of the definition are very important: to single out and separate a certain concept from all the others, to indicate those essential features without which this concept cannot exist, on which all other features will depend. In the process of teaching children in elementary school, contextual and ostensive definitions are of particular interest among implicit definitions.
In the process of teaching children in elementary school, contextual and ostensive definitions are of particular interest among implicit definitions.
Any passage from the text, whatever the context, in which the concept that interests us occurs, is, in some sense, its implicit definition. Context allows you to put the concept in connection with other concepts, revealing their content.
For example, when working with children such expressions as “find the values of the expression”, “compare the value of the expressions 5 + a and (a - 3) 2, if a = 7”, “read expressions that are sums”, “ read expressions, and then read equations”, we reveal the concept of “mathematical expression” as a record that consists of numbers or variables and signs of actions. It should be noted that almost all definitions that we encounter in life will be contextual definitions, since if we hear an unfamiliar word, we try to figure out its meaning ourselves, based on the general meaning of what was said. Such a phenomenon is present in the system of teaching younger students, since some mathematical concepts are defined in elementary school through the context.
Such a phenomenon is present in the system of teaching younger students, since some mathematical concepts are defined in elementary school through the context.
These are, for example, such concepts as “big - small”, “any”, “any”, “one”, “many”, etc.
Contextual definitions remain largely incomplete and incomplete. They are used in connection with the unpreparedness of the younger student to assimilate the full and, all the more so, the scientific definition.
Ostensive definitions are definitions by demonstration. They resemble ordinary contextual definitions, but the context here is not a passage of some text, but the situation in which the object denoted by the concept finds itself. For example, the teacher shows a square (drawing or paper model) and says "Look - it's a square." This is a typical ostensive definition.
In elementary grades, ostensive definitions are used when considering such concepts as “red (white, black, etc.) color”, “left - right”, “left to right”, “number”, “preceding and following number”, "signs of arithmetic operations", "comparison signs", "triangle", "quadrilateral", "cube", etc.
Thanks to the assimilation of the meanings of words in an ostensive way, it is possible to introduce into the child's vocabulary the already verbal meaning of new, previously unfamiliar words, as well as entire phrases. It is ostensive definitions that can connect a concept with objects. In elementary school, there are usually some acceptable definitions, like "We'll use the word 'pentagon' to mean a polygon with five sides." This, one might say, would be a nominal definition. Various explicit definitions are used in mathematics. The most common of them is the definition through the nearest genus and species character. The generic definition is also called the classical one.
Examples of definitions in terms of genus and species: “A parallelogram is a quadrilateral whose opposite sides are parallel”, “A rhombus is a parallelogram whose sides are equal”, “A rectangle is a parallelogram whose angles are right”, “A square is a rectangle, in which the sides are equal”, “A square is a rhombus with right angles”.
Let's analyze such concept as a square. Speaking about the first definition, it should be said about the nearest generic concept - "rectangle", where the species attribute will be - "all sides are equal." In the second definition, the closest genus is "rhombus", and the specific feature is "right angles". And if we take a genus that will not be the closest, then there will be two specific signs of a square, for example: "A parallelogram is called a square, in which all sides are equal and all angles are right." Some definitions allow us to consider concepts according to the method of its formation, as well as according to the method of its occurrence. Such definitions are usually called genetic
Examples of genetic definitions: "Angle is the rays that come out from one point", "The diagonal of a rectangle is a segment that connects the opposite vertices of the rectangle." In elementary grades, genetic definitions are used for such concepts as "segment", "broken line", "right angle", "circle".