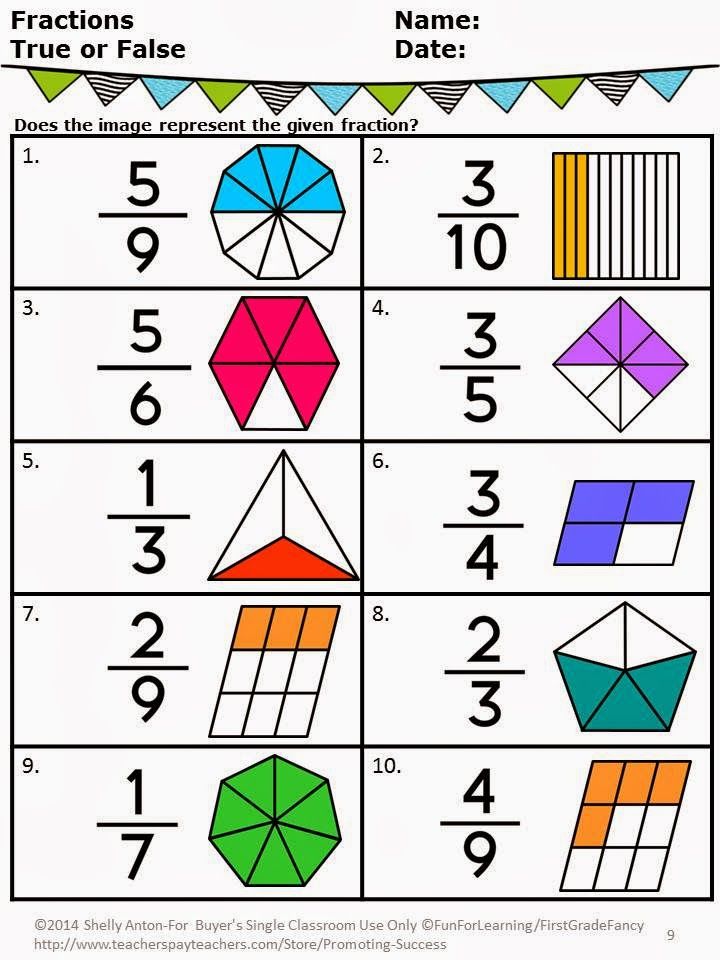
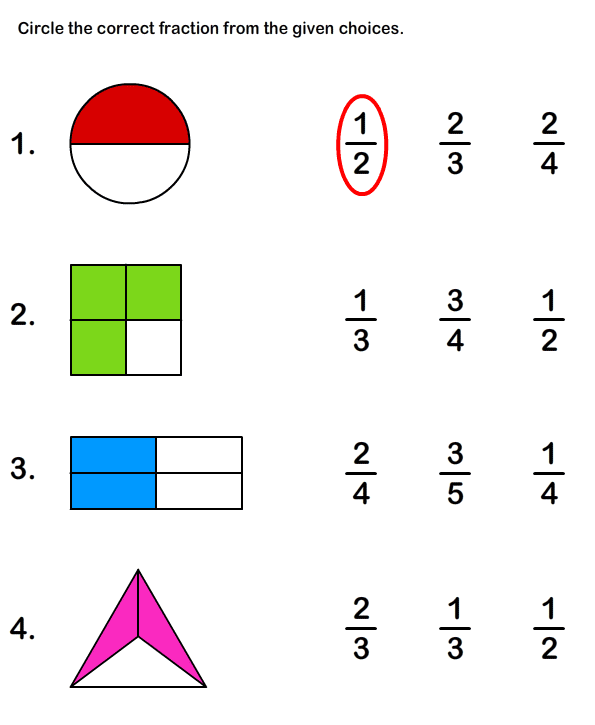
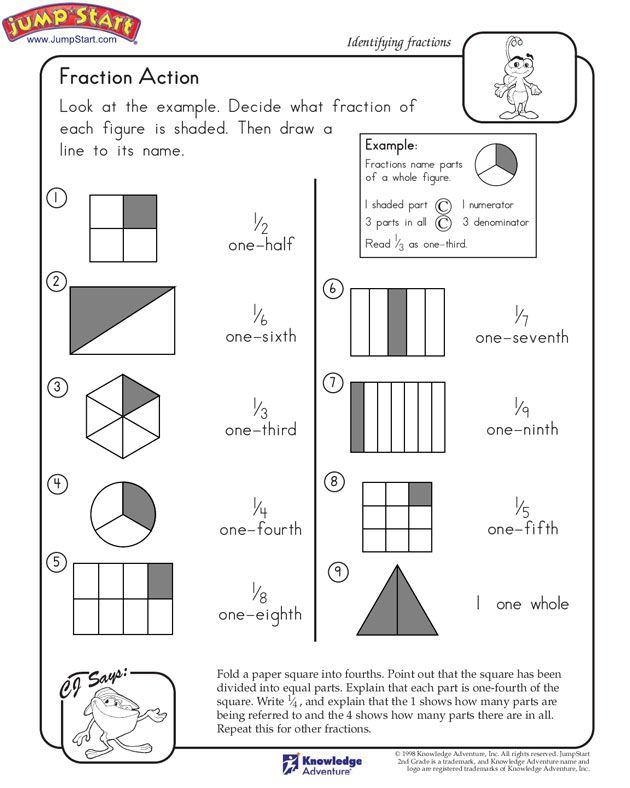
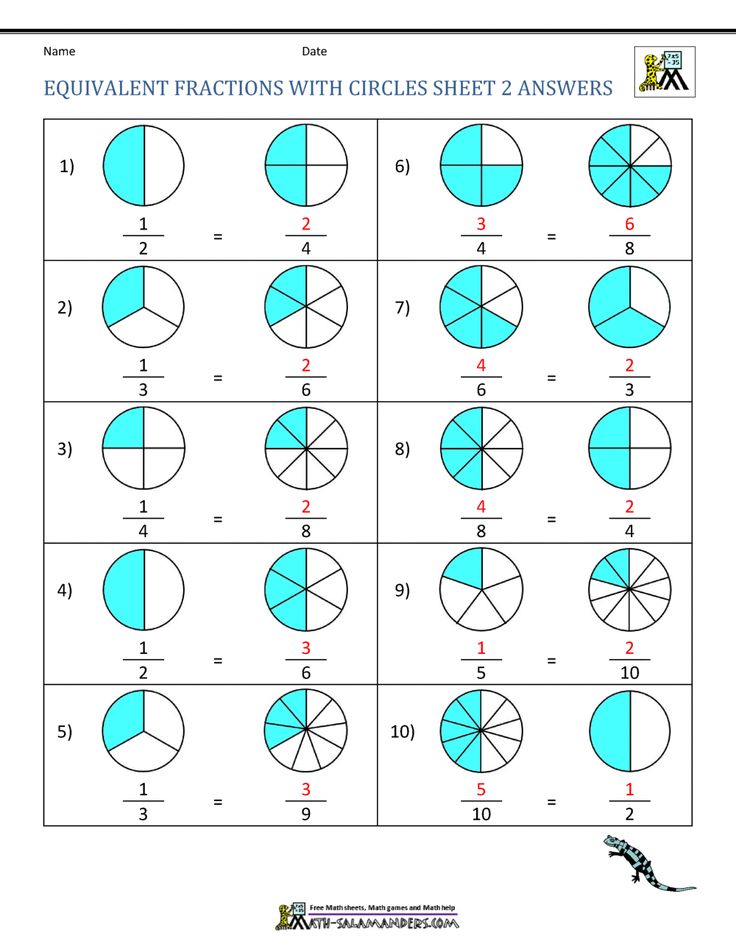
1St grade fractions activities
Fraction activities, games, and read alouds for 1st and 2nd grade
Happy Tuesday! I know many of you are on Spring Break this week but I wanted to share several fraction activities and games that are great for 1st grade and 2nd grade. There are lots of fun ones that will help amp up the engagement during your fraction unit. Our state standard focuses on fractions of a whole – not of a set.
Alrighty, let’s start planning!
FRACTION ACTIVITIES AND GAMES
For years now I’ve used sticky notes to introduce fractions and partitioning shapes in 1st grade. Over the years I collected sticky notes in various shapes and sizes…anytime I’m out and about and see unique shapes, I throw ’em in the cart. Amazon is also a great place to find lots of sticky notes. I have this pack and they’re great.
I always start off introducing equal and unequal when partitioning the different shapes. These anchor charts are easy to make and kids love them. I gave each student a sticky note and let them add it to the chart under the correct category – this is a great way to get lots of discussion and thinking going. Of course relating this to sharing food is a great way to connect to the real world.
Sticky notes are also great for students to use to fold, draw, and cut the shapes into various fractions. This Book of Fractions is a great activity to hold all their sticky note shapes.
Here are a few other fraction activities and games for partitioning shapes to create different fractions.
Spin and Make can be used in partners, independently, stations, or as a whole group. If using it as a whole group, simply project the activity onto your white board and use a magnetic spinner on the board. These are my favorite; I’ve used them for years. To play, students spin the spinner, create the shape they land on and partition it into the corresponding amount of pieces.
For Fraction Wreaths, students color in the circles to create their own fractions.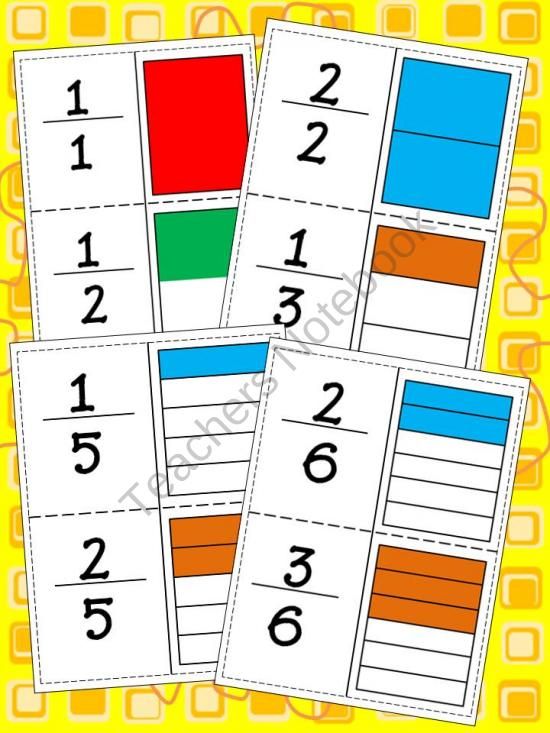 Afterwards, they write the fraction in word form and/or number form. The colors of the wreath can be changed out to match the season….Christmas could be red bow and green strips…fall could be brown bow and yellow strips, etc. The wreath is a paper plate with the middle cut out. Easy peasy!
Afterwards, they write the fraction in word form and/or number form. The colors of the wreath can be changed out to match the season….Christmas could be red bow and green strips…fall could be brown bow and yellow strips, etc. The wreath is a paper plate with the middle cut out. Easy peasy!
Next up is a class favorite from a few years ago. We played this with place value (see here) and lemme’ just tell ya’…it was a HIT! Loads of fun, meaningful, easy prep, peer collaboration…talk about engagement!
To play: group your students in table groups (this could be played independently but peer collaboration is my favorite!) and give each group a fraction card and several sticky notes. I like to give each table group a different color – this makes it easy to quickly see which table group is having trouble with the skill, etc.
Hang the different fraction posters around the room (square model, circle model, rectangle model, word form, numerator, denominator)…
Students will work together as a table group to represent the fraction on their card in the various forms that are on the posters.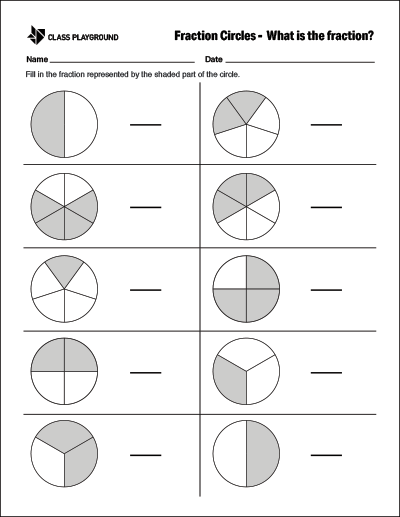 After they have it the form of their fraction on their sticky note, they stick it to the correct poster. Y’all! They love it! It’s such a fun fraction game! Plus, it helps the different forms of fractions and ways that fractions are represented, STICK.
After they have it the form of their fraction on their sticky note, they stick it to the correct poster. Y’all! They love it! It’s such a fun fraction game! Plus, it helps the different forms of fractions and ways that fractions are represented, STICK.
Spin It is another fraction game that is a blast. This is projected onto your whiteboard and used with a magnetic spinner. There are several different Spin It slides available here. To play: students (or you) spin the spinner, and students write the word form on their desks with an expo marker….or they could verbally tell you the fraction. You can play in table teams and make it a race to write the correct fraction first. My class always liked to play Spin It in table groups.
Flip and Share is a partner game where students take turns flipping over two cards and comparing them.
Tic-Tac-Find is another fun partner game to practice reading and identifying fractions.
Fraction Walk is a “Write the Room” activity and Read, Match, Scoot is played like the classic game of Scoot. I love using these type of activities for assessments.
I love using these type of activities for assessments.
I love this craftivity and hativitiy to wrap up the fraction unit!
And because you gotta get those grades in…Fraction Match Up sort and these Flip Books work well for informal assessments!!
There are several other flip book options here.
Whew. That was a lot, but I hope these activities will create engagement in your classroom and make learning about fraction fun for you and your students!
You can find all these activities here or Pin the picture below to your Pinterest board.
Oh and here are few old fraction freebies.
Before I go, I’ve rounded up some fraction read alouds for you. These are available on Amazon (affiliate links included), but you could definitely check your school or local library to see if they have them.
A Fraction’s Goal – Parts of a Whole
Fraction Fun
Full House: An Invitation to Fractions
Fractions in Disguise
Fraction Action
Give Me Half
If I were a Fraction
Twinderlla, A Fractioned Fairy Tale
Peg and Cat: The Pizza Problem
Miss Giraffe's Class: Fractions in First Grade
I know I seem to say this about every math concept I blog about but I LOVE FRACTIONS.
Well, the first grade version of fractions :)
It's weird to even hear myself say it because I struggled, and I mean struggled, with fractions in school. I was a good test taker though so I always flew under the radar with my teachers but I remember just looking at them like What is this?!
SO I think it is really important to build those fraction foundations so your students don't end up staring at fractions the way I did!
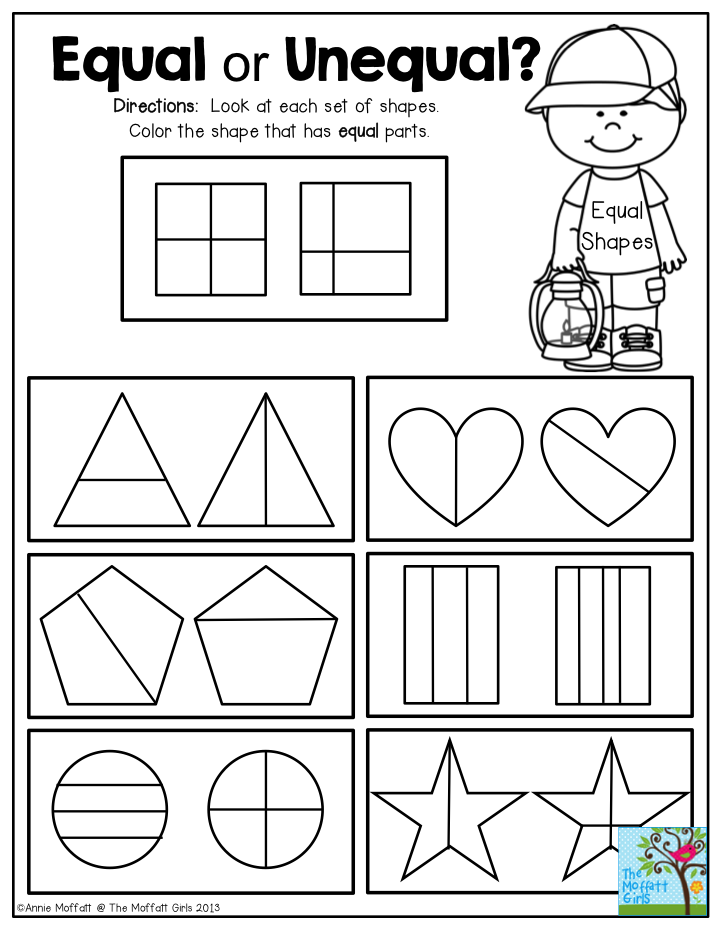
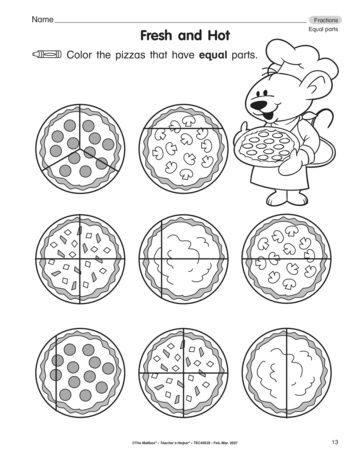
I think the first concept in fractions to teach is EQUAL PARTS, or EQUAL SHARES.
See those funky shapes with unequal parts on the right?? We call those "nutty shapes"!
Our job is to help our squirrel friend find the nutty shapes with unequal parts so we sorted the shapes by whether they had equal parts or unequal parts.
Then some practice!
Once your students understand that fractions have equal parts, I recommend having them partition fractions themselves! The most fun way to do this, in my opinion, is with dough!
In small groups, give each student a ball of dough.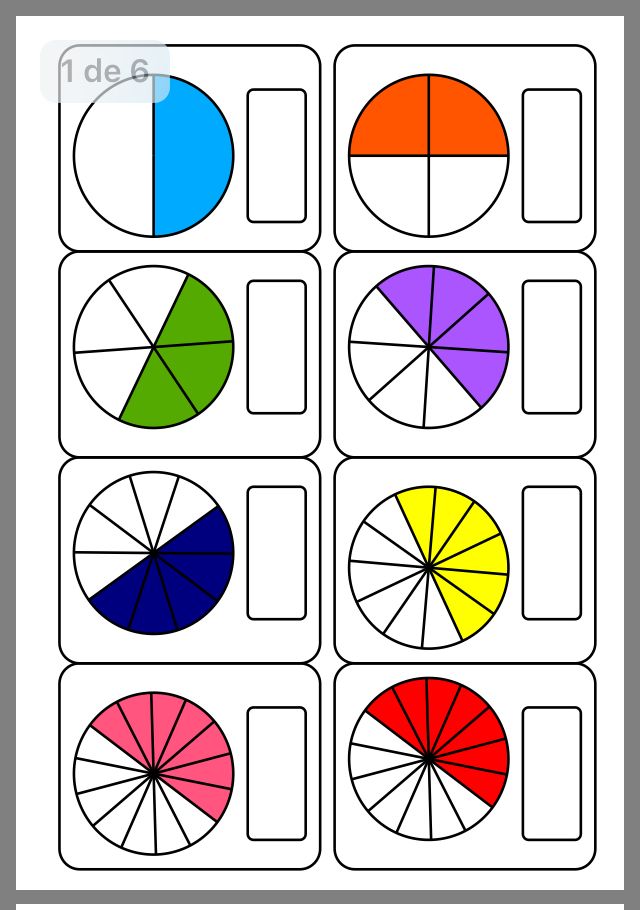 Provide a rolling pin for them to roll their balls flat and some circle cookie cutters to cut out circles. If you don't have rolling pins, you can use pretty much anything - even a highlighter - or they can just smash it down with their hands. If you don't have circle cookie cutters, you can use anything with a circle base. Turn a plastic cup upside down and press it in. Can you tell I improvise a lot?? :)
Provide a rolling pin for them to roll their balls flat and some circle cookie cutters to cut out circles. If you don't have rolling pins, you can use pretty much anything - even a highlighter - or they can just smash it down with their hands. If you don't have circle cookie cutters, you can use anything with a circle base. Turn a plastic cup upside down and press it in. Can you tell I improvise a lot?? :)
This activity is not only super fun but it promotes SO much good conversation and thinking.
Guide your students by having them cut one of their circles in half. Talk about how each of the 2 shares is a half and together they're called halves. Then have them cut their other circle into half.. and then half again. Let them know these are called fourths because there are four equal parts. As they're looking at their halves and fourths side by side, ask them if they notice anything. Discuss how the more shares you cut it into, the smaller the shares get. You could also cut your circle in an unequal way and ask if it's cut into halves/fourths/whatever and help them see that the shares must be equal to be called halves/fourths/etc.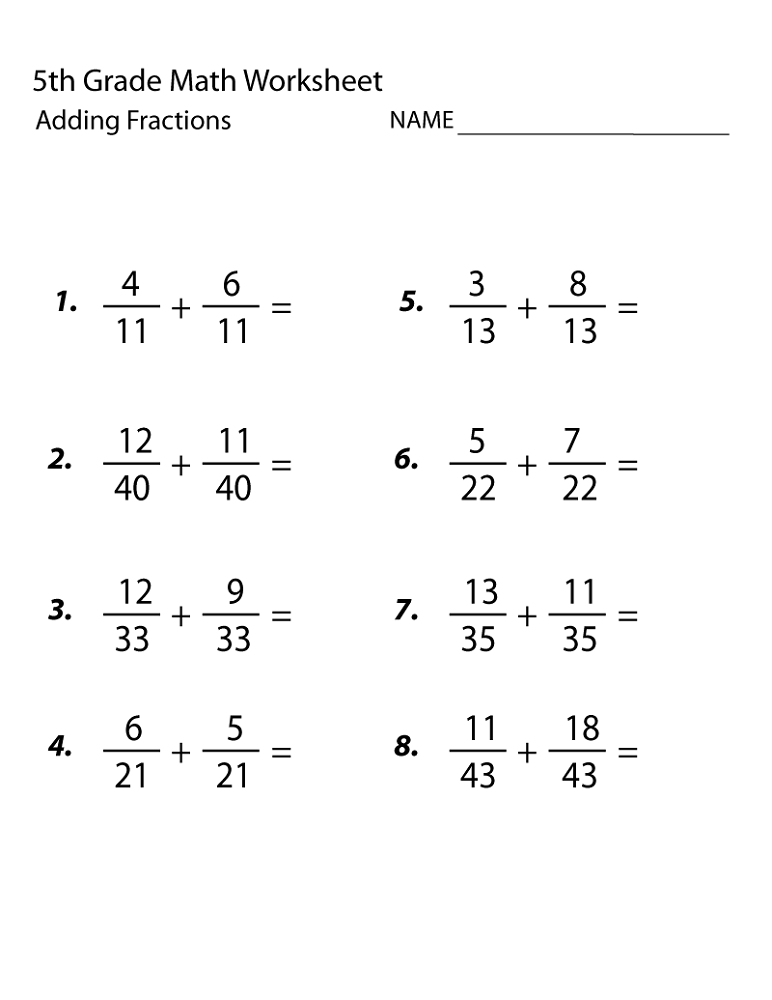 There are so many great concepts you can teach with the dough out! I also made a cute little recording sheet to go along with it so they can write about what they learned during/after their exploration.
There are so many great concepts you can teach with the dough out! I also made a cute little recording sheet to go along with it so they can write about what they learned during/after their exploration.
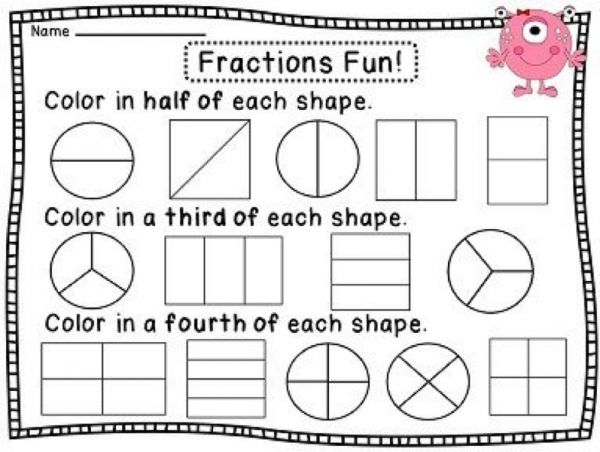
For more halves, fourths, etc. practice, I made practice sheets in different difficulty levels.
One of the types of worksheets I made were these sheets that focus on a specific type of fraction (cut into halves, thirds, fourths, or eighths).
I love these because they practice several skills at once but for each type of fraction so it's not too confusing or overwhelming.
If you need something a little easier, this is a great worksheet to do first to review what halves, thirds, and fourths are:
And of course some cutting and pasting action because I love having them for every math concept ever because kids love it and it is great, self-checking practice.
I've said this before but...
According to 5 and 6 years olds:
Math Worksheet = Math.
Math Worksheet + Cutting/Gluing = Party.
:)
and a little coloring action too because who doesn't love coloring?!
Once you think they've got it down, I recommend busting out the Fraction Flowers!!
I included this activity in a bunch of different ways because I love it so much. You can use it as a craft, a math puzzle/center, or hang the flowers up as a poster or visual aid.
The craft makes a super duper cute bulletin board!
I love the way ours turned out! Sorry for the #notsohumblebrag but I can't help it - I love, love them and they were a lot of fun to make!
Can someone help me think of a cute bulletin board title? "Come see what we're growing in our Fraction Garden!" "Our Fraction Garden is Blooming" ... I can't figure out exactly what I want it to say :)
For this math craft, we sorted the HALF OF fractions and FOURTH OF fractions. I also include WHOLE, THIRD OF, and EIGHTH OF.
It's differentiated as well so you can choose how much to challenge your students. Each flower comes in both full color and black & white. Each of those options also comes in pre-made (where the flower is already put together as a pretty flower like you see above) OR mixed up in pieces for students to sort and build their own flowers.
Each of those options also comes in pre-made (where the flower is already put together as a pretty flower like you see above) OR mixed up in pieces for students to sort and build their own flowers.
For the craft, I just printed the premade black and white flowers so all you had to do is color in the fraction either half of or fourth of, color the rest of your flower, and then cut and glue it to the construction paper.
What I recommend if your students are up for the challenge (or you have a select few that are) and you have the time, have them sort the petals to the right flower first! It's an awesome sorting activity that adds even more learning to the craft. Print the 'mixed up in pieces' black and white version for students to SORT FIRST to build their flowers. They'll put the petals with the right flowers and arrange them to build their flowers. It might not be as "perfect" looking for your bulletin board as the above example but it'll be authentic and awesome! (I used to always want bulletin boards to be perfect examples of perfect work and I finally realized that was silly and it was the imperfections and differences that made them authentic and beautiful examples of work - random weird philosophical thought, sorry! :))
The mixed up pieces color version is a review center for next week!
Students sort the petals to the right fraction and then copy what they match onto their recording sheet.
For the premade color ones, I printed and laminated them to use as a visual.
Right now, they're on my table as a reference tool but I'm going to cut them out and hang them up as a classroom poster display soon.
Being able to partition the fractions into halves and fourths themselves is a big concept in first grade so that's important to practice! I made worksheets to practice but this one (the easy level - A) is good to throw in a pocket protector with dry erase markers to practice in small groups when introducing it. Encourage your kiddos to find multiple ways to divide the circle/square into halves/fourths/thirds/whatever.
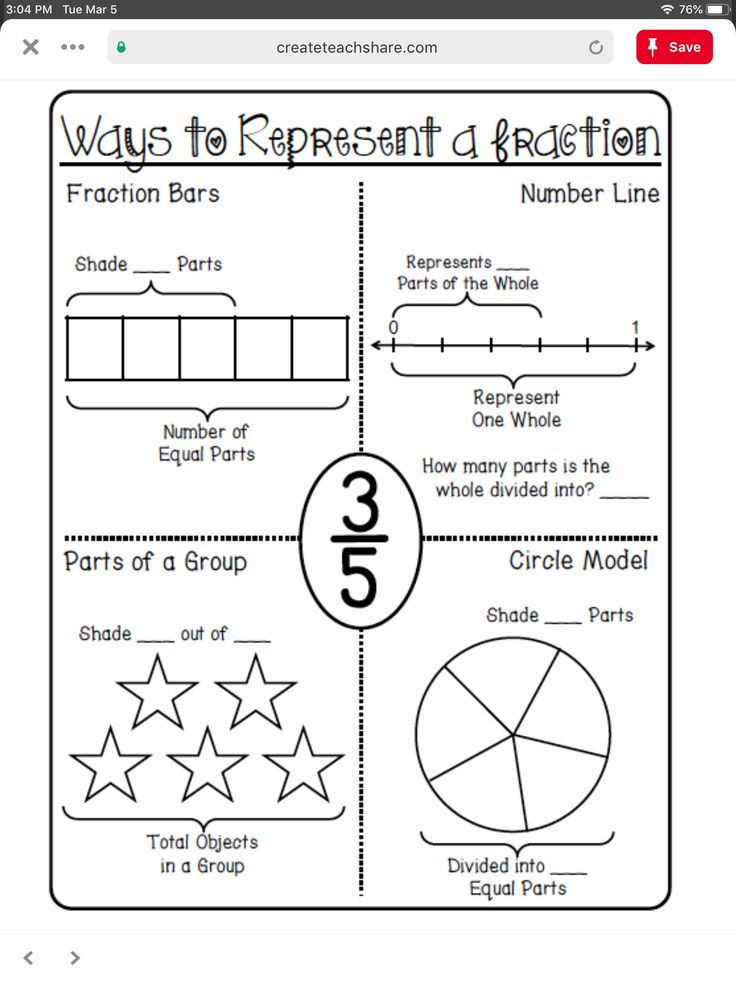
Once you feel like they really get the fundamental concepts, you can move into the number representation of fractions. I know a lot of first grade teachers are told not to do it but I personally think that once you lay down the basics, they're very capable. If you're saying "1 out of 3" shares, they might as well know the representation. BUT if you disagree, that's fine! I created some very simple straightforward worksheets and centers to practice it for those who want to venture into the fraction abyss.
BUT if you disagree, that's fine! I created some very simple straightforward worksheets and centers to practice it for those who want to venture into the fraction abyss.
I think a great way to help kids understand it is to write "out of" on the line that separates the numerator and denominator and teach them to read down to "read" the fraction. It really helps them get what the fraction means, but most importantly, they won't mix up the numerator and denominator if they know what the written expression for the fraction actually means. For example, the circle has 3 out of 4 pieces colored so that is how they would read it starting at the 3 and going down: "3 out of 4". Here is what I mean:
Puzzles obviously because I would make puzzles of puzzles if I could :)
And what else?? Cut and pasting fun! Always <3
Thank you for listening to me share some of my ideas for teaching fractions. I hope you got some new ideas!
If you want the activities from this post, they're ALL included in my:
First Grade Math Unit 18
along with A LOT more worksheets, centers, cutting and pasting activities, and assessments!
Unit 18 is also included in the First Grade Math Units 10-18 Bundle if you want all of the second half of my first grade math units :)
Okay one more thing I want to share with you that I love. ....
....
FRACTION BOWLING!!!
I scored this adorable little bowling set at Target for only $7 over the weekend and I'm so excited to use it. You can find 10-piece bowling sets in a lot of places so if you can't find this at Target, I'm sure you can find one online pretty easily. It comes with 10 plastic bowling pins and 2 little plastic balls.
So the way to play Fraction Bowling is to set up all the pins. (It comes with a mat that shows exactly where to set the pins to make it easy for kids to set up but I set it up without it because it's cuter for the picture, not gonna lie :))
Have students take turns rolling the ball to the pins to try and knock them down. I recommend letting them roll it twice just like with regular bowling so they don't completely miss on their turn and get upset. There are 2 balls.. an orange ball and a purple ball so they could get both balls to start and roll each.
At the end of their turn, they go to their recording sheet and color in how many pins they knocked down out of the 10 pins on the worksheet. Then, they write the fraction they made. So if they knocked down 4 pins, they'd color in 4 of the pins on their recording sheet and write 4/10 next to it. You can skip the writing the fraction part if your kids aren't ready for it. Just the 4 out of 10 is great practice anyway! Or if you want to make it harder for your super high kids, make them make a fraction of ALL the pins once they're all done (ex: 36/60 pins total).
Then, they write the fraction they made. So if they knocked down 4 pins, they'd color in 4 of the pins on their recording sheet and write 4/10 next to it. You can skip the writing the fraction part if your kids aren't ready for it. Just the 4 out of 10 is great practice anyway! Or if you want to make it harder for your super high kids, make them make a fraction of ALL the pins once they're all done (ex: 36/60 pins total).
I made a simple little recording sheet to go with it that you can download for free by clicking here:
Fractions Bowling
:)
If you want to save these ideas for later on Pinterest, feel free to pin this or any other picture from this post:
Lesson on "Comparing fractions with the same denominators"
"Comparing fractions with the same denominators" 4 class according to the School 2100 OS program
Purpose: Formation and development of value relationship to joint learning activities to determine and apply the method comparing fractions with the same denominators.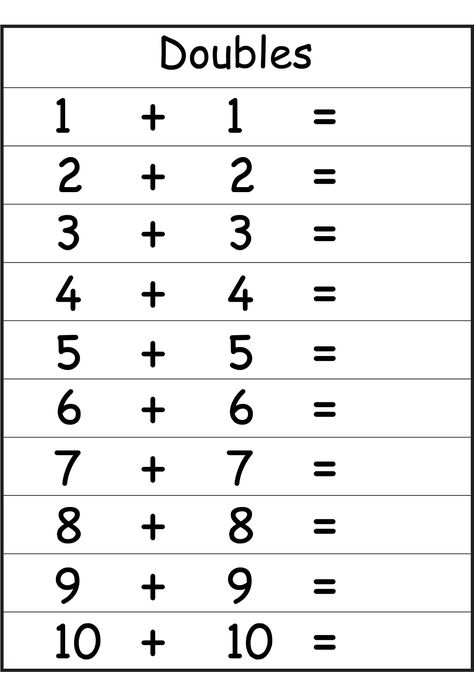
Ability to self-assessment based on the criterion of the success of educational activities;
Control (correlation of the mode of action and its result with a given standard), evaluation (awareness of the quality and level of assimilation)
Reflection of methods and conditions of action, control and evaluation of the process and results of activities.
Building an algorithm for activities to comparing fractions with different numerators and the same denominators .
Negotiate with people: playing various roles in group, to cooperate in the joint solution of the problem (task).
Ability to express with sufficient completeness and accuracy their thoughts in accordance with the tasks and conditions of communication. nine0003
to use when solving different task names and sequence of digits in number records,
to use when solving various problems and justifying their actions knowledge of how many units of each digit are contained in the notation of the number.
methods problem-dialogical learning (statement of the problem and search for its solution, leading or inciting dialogue),
Educational and methodological support. nine0032
Program: "Mathematics". Authors: S.A. Kozlova, A.G. Rubin, T.E. Demidova, A.P. Thin (Moscow "Balass" 2011)
"Mathematics" Textbook for grade 4 in 3 parts. Authors: T.E. Demidova, S.A. Kozlova, A.P. Thin (Moscow "Balass" 2013 d.)
“Mathematics. Grade 4 ”(Methodological recommendations for the teacher). Authors: S.A. Kozlova, A.G. Rubin, A.V. Goryachev (Moscow "Balass" 2013 g.)
|
Technology Stage |
Results: |
Evaluation of results |
Target of step |
Interaction in the educational process | |||||
|
personal |
metasubject | nine0079
Teacher actions |
Student activities | ||||||
| 1.
| Inclusion in educational activities at a personally significant level. | nine0133 Subject readiness for upcoming activities | OS for the ability to assess readiness for the upcoming activities | Formation and development of the AC to the ability to assess readiness for the upcoming activities (at the lesson) | Organizes the situation self-assessment by students of readiness for the upcoming activity (in the lesson). nine0004 Manages the situation self-esteem.
- Assess readiness to the lesson. (using color signal) Today we have an unusual lesson. Ready for him, I see Each of you. Mood and confidence, Well, keep it up. Sit down at your desks, It's time to start. Today we have an unusual lesson , because We are once again embarking on a mathematical journey. nine0003 For this we Divide into groups and each will receive their own guide sheet. | Perform self-assessment readiness criteria: · the correct choice of educational supplies, · correctness and neatness of the arrangement of objects on the desk, · self-training, · set up for the lesson.
nine0050 | |||
| 2. Presentation tasks.
| AC to the ability to perceive the task.
U educational interest in new learning material.
| Perception exercise.
| Understanding the subject content of the task. | nine0004 OS for the ability to perceive the task. | Formation and development of the AC to the ability to perceive the task.
Motivation for learning activities, formation of cognitive interest.
| Invites children to read the first task.
Compare given numbers and values: 1) area of lake Saltaim —146 km², Ik lakes - 1190 km² 2). Length of the Volga River 3,531 km, and the Indus River - 3,181 km 3) 2/10 Omsk lakes areas with salt water, and 8/10 with fresh water
Understand what says every sentence | Read (listen) exercise.
| ||
| 3. Understanding the task.
| AC to the ability to independently determine the goal, result, way of doing things. nine0003
| Skill predict, determine result , goal and method activity. Ability to target (at the level of acceptance of a practical task). | Representation of targets , result of (subject ZUN), which is required get for the successful completion of the task and the method of obtaining it. nine0050 | OS for independent determination of the result, purpose and mode of action.
| Formation and development of the AC to the ability to independently determine result, goal, method of activity.
Checking children's understanding of the task, the inclusion of everyone in the activity for the formation of cognitive interest.
| nine0004 Organizes the situation understanding of the task: its purpose, result and method of activity.
-How did you understand the task? - What will you get as a result?
| Represent the result, define the purpose and method activities through understanding the task. Agree - about the result task completion, - about plan and method actions to achieve result - about the form of expression and presentation of the result.
Suggested dialogue: A1: I understand the task like this: We will read the records, understand what they are about they say, compare the numbers A2: We will write down the numbers separately, discuss group which number is greater and why, put a comparison sign A3 : As a result of notebooks will be written in a column of numbers, between them there will be a sign comparisons. | nine0077 ||
| 4. Job in progress | CO for an independent practical activities
| The ability to independently apply methods of action to achieve the planned result, the ability to control and evaluate. | Completed (or not completed) task.
| OS for independent practical activities. nine0003
| Formation and development of the central heating to independent practical activity.
Organization of performance activities tasks.
| Includes students in practical activities, aimed at achieving results.
Observes children's activities, selects various outcomes.
Report group readiness checkbox. | Perform the task individually, in advance in the stipulated way.
Receive planned result.
Analyze what and how they did, evaluate the results.
| ||
|
| 5. Presentation of the result.
| AC for skill present and evaluate the results activities | Ability to present and evaluate results (retrospective self-assessment)
| Success in the application of ZUNs studied earlier. Error in using unexplored material. | OS per skill present and evaluate the results of their activities. nine0003
| Formation and development of the AC to the ability to present and evaluate results its activities. | 4. Organizes the situation presentation of results.
- Please introduce their results to group members.
| Present the results of the completed task (various) :
146 km² < 1190 km² 3,531 km > 3,181 km 2/10 > 8/10 2/10 < 8/10
| |
| 6. comparison performance results.
| AC for skills compare, evaluate the results of their activities and recognize the fallacy result. | nine0004 Ability to evaluate results (retro-spective self-esteem). | OS on the ability to independently monitor and evaluate their results, ways to achieve them, their achievements in the study of the topic. | OS on the ability to compare, evaluate the results of their activities and accept the error of the result.
| Formation and development of AC to the ability to compare, evaluate results their activities and admit the erroneousness of the result. nine0003 | 5. Organizes comparison situation. Draws attention to every result. - Are there groups with a different result?
(recording)
| 5. Describe results. They try to justify the correctness of the result. Match their results with suggested options. (The results are different, hence the doubt, difficulty, dissatisfaction with the result) | ||
| 7. Comparison of methods of activity.
| AC for the ability to describe, compare and evaluate the ways of one's own activities and recognize the fallacy of their choice.
| Ability to describe, compare and evaluate methods their activities and recognize the fallacy of their choice. Training action: description of the method of activity. nine0003
| OS on skill by yourself analyze and reflect on achievements | OS for the ability to compare, evaluate and recognize the fallacy of the methods of action on its achievement.
| Formation and development of the AC to the skill describe, compare and evaluate the way they operate and recognize the error of their choice.
nine0004
| Organizes the situation comparisons, finding out who acted in the same way as the disciples, presenting their results to all class. Offers to one a representative from each group to present the reasoning. nine0003
-Let's pay attention to the task again. The numbers are the same, but the two groups of guys got different results. - What guided the comparison?
HOW DID YOU CONSIDER?
At this stage happening team division into groups according to the unity of ways
nine0004
| Describe methods activities. Trying justify the correctness of the chosen method. Describe the actions Answer questions from teachers and classmates. 1) In the first case the units of measurement were compared first. They are one and the same. Then- the number of digits in each number. It is different. Therefore, more than the number which uses more digits. nine0004 2) In the second case the units of measurement were compared first. They are one and the same. Then- the number of digits in each number. They are one and the same. Then- the number of digits in each number. It is the same therefore, the digit of each digit was compared, starting with the highest. First digit the same, and the second is different, which means that the number where the digit of the discharge is greater is greater.
3)A 1: Compared denominators are the same. Compare numerators: more that fraction, y whose numerator is greater. nine0004 A 2: I presented the cake, divided it into 2 parts and 8 parts. Came to the conclusion that half of the cake is larger than an eighth. group with me agreed.
The appearance of doubt about the correctness of the method that everyone has chosen. | ||
| 8. Abstraction
| AC for the ability to identify the main principle used to obtain the result of each group students. nine0003 | Ability to highlight the main principle used to obtain the result of each group students. | Presented to each group the principle underlying their way of solving (concept, law, rule, etc.), dissatisfaction with its insufficiency, inexplicability or absence. | OS for the ability to allocate basic principle used to get a result for each group of students. nine0003 | Formation and development of the AC to the skill highlight the basic principle used to get the result of each a group of students. | The teacher offers name the rule, law, concept, etc., which formed the basis of actions each group. Helps you understand what the named principle is either insufficient, or inaccurate, or there is no such thing.
| They call the rule law, concept or do not find such. nine0003
Formulate their own difficulty in substantiating the results and corresponding actions.
| ||
| 9. Problem-tization
Goal setting
| AC to the ability to independently define and formulate a problem, a cognitive goal and a topic for study and, in accordance, assume one's actions. nine0003 | The ability to independently define and formulate a problem, cognitive purpose and topic to study and, accordingly, to assume their actions. Goal-setting at the level of redefining practice tasks in the theoretical | Representation of not studied subject content and methods its application. Definition of the area of "ignorance".
| nine0133 Formation and development of the AC to the skill independently define and formulate a problem, a cognitive goal and topic to study.
| Creates a situation of redefining a practical task into a cognitive one. Organizes the situation formulating a problem, goal and topic for study through finding out what knowledge is not enough to solve the problem. nine0003
- Can you name a method or a rule that will help us all equally How to compare fractions with different numerator and same denominator? - What problem did you encounter? - What is the goal we set? - What is the topic of the lesson? | Speak problem, separating knowledge from ignorance. Independently formulate the problem, goal and topic to be studied and, accordingly, anticipate their actions.
Issue: - We don't know how compare fractions with different numerator and same denominator
Target: - Find out how correctly compare fractions with different numerator and same denominator
Subject: - We can write down the topic in a notebook "Comparing fractions with different denominators and the same numerator".
| |||
| 10. Training solution tasks
10.1 Deproblematization | AC to the ability to independently determine ways to achieve the goal.
| Skill independently determine ways to achieve the goal. Learning activities: research, criticism, building a new "norm": concepts, way, algorithm. nine0003 Ability to search and highlight the necessary information from the textbook
| Knowledge and understanding of new way of activity.
| OS on skill by yourself determine ways to achieve the goal. | Formation and development of CO to independent educational and cognitive activity,
| nine0004 Organizes the situation solving a learning problem, searching for a theoretical foundation. To achieve the goal, we will perform practical task.
For its implementation it is necessary be able to identify numerator and the denominator of a fraction, to know what these numbers show in the notation of the fraction.
F/MINUTE
Carry out squats as many times as the numerator of 4/5. nine0004Do so much left-right slopes, what is the denominator of the fraction 2/8.
Jump so much times, what is the sum of the two numerators
Station 2. Textbook p.28 №2 (а,b)
The teacher organizes an introductory dialogue. - What is the length of both strips? - Into how many parts each was divided stripe? - How many parts were painted over? nine0003 - What part stripes painted over in each picture? - Write down fractions, name the numerator and denominator of each. - What do these have in common fractions? - Than they different? - What conclusion can be to do by looking at the pictures?
- Comparable to textbook rule page 28 - Does it match your conclusion?
| Group (frontal) work: solve the problem by discussing and putting forward hypotheses; looking for theoretical the basis for constructing an activity algorithm. nine0003
Children pronounce the rule.
- Length of each strips 16 cm. - Divided by 8 parts. nine0003 - Painted over 3/8 and 5/8 parts
- These fractions have different numerators and same denominators
Output: The greater is the fraction with the greater numerator. | ||
| 10.2. Simulation
| AC for skill visualize a concept (mode of action). nine0003
| Ability to create a visual image of a concept (mode of activity)
| Concept model (model new mode of activity)
| OS on the ability to create a visual image of a concept.
| nine0004 Formation and development of CO to the ability to create visual image of the concept,
| 2. Organizes the situation of building a model. Asks to speak theoretical formulation using model.
| Build a model of the fraction comparison method. Schematic portray her, They speak.
| nine0077 ||
|
|
|
|
|
| - Let's build model of our activity in comparison of fractions. Let's denote the same denominators the same letter, and different numerators with different letters.
a/v > s/v, if a > c | ||||
| 10.
| AC to the ability to build activity algorithm CO for the ability to apply significant additions, refine the algorithm, redesign. | Training activities on the level of adequate transfer of knowledge. Ability to build an algorithm activities Ability to make significant additions, refine the algorithm, redesign. nine0003 Training action: research, criticism, re-normalization.
. |
| OS on the ability to build an activity algorithm. | - to the ability to build a new algorithm of activity (or make significant additions, refine the previous algorithm, redesign). |
Comparison algorithm: 1. 2. I find different numerators with the same denominators. 3. I conclude that the fraction with which numerator greater than |
| ||
| 11. Primary fastening (Application of the rhythm algorithm in practice)
| AC for skill perform the task according to the algorithm. nine0050 | Ability to perform task according to the algorithm |
| OS for the ability to perform a task according to the algorithm. | Formation and development of CO to the ability to perform algorithm task. | Offers to return to the very first task and complete it according to the new algorithm, pronouncing him in external speech. | Perform original task with pronunciation of the algorithm in external speech.
| ||
|
12. Training
| AC for the ability to complete assignments for reinforcement knowledge and ways of doing things. | Ability to perform reinforcement tasks knowledge and ways of doing things. Learning activities - work on algorithm, - self-control, - self-assessment | Strong knowledge of the topic . Skill compare fractions by built algorithm.
| OS for the ability to perform tasks to consolidate knowledge and methods of action. | Formation and DH development towards consolidation of knowledge and methods of action. nine0004 | Organizes a decision situation typical tasks for a new method of action with pronunciation of the algorithm during external speech. Offers to execute system of training tasks.
Station 3. A) Work in pairs. Compare fractions, put them in order magnification and find out the name of the fish, write its name on the card, tell others that I have learned.
nine0004 Organizes self-assessment of children learning a new way of doing things.
| Perform tasks textbook (teacher) in pairs, first pronouncing the algorithm externally, and then on their own. Implement self-control and self-assessment of their work.
| ||
|
8/8 (k) 2/8 (y) 7/8 (a) 3/8 (d) 1/8 (s) _________ is a very common fish of the Volga.
13/16 (c) 7/16 (k) 2/16 (k) 5/16 (o) 9/16 (u) 15/16 (a)
Honey ____________ lives in the basins of the rivers Indus, Ganges and Brahmaputra (India), well sunlit ponds, this fish prefers ponds with clean, oxygenated water, but describes cases of finding it on rice paddies fields
7/21 (n) 1/21 (e) 20/21 (o) 5/21 (a) 14/21 (u)
______________ is widespread in aquarium culture. Fish of the zebrafish genus can be kept in a common aquarium, spacious and closed at the top.
B) Independent work. Textbook page 29 №4, 5
| |||||||||
| 13. Lesson summary. Reflection activities | AC to the ability to analyze and comprehend one's progress. | Educational and cognitive interest Goal setting nine0004 Learning activitiesSelf-monitoring Self assessment | Strong knowledge and skills on the topic. | OS on skill by yourself analyze and reflect on progress. | Formation and development of the AC to the ability to analyze and comprehend one's achievements. | Organizes discussion, dialogue, allowing students to comprehend the results obtained by the study of the topic, ways to achieve them, personal contribution to the collective work ...
- What was the topic of the lesson? Purpose? -Have you achieved your goal? (What rule did you get acquainted with?) -What skills did you develop? -What have you learned? - Where in life they can useful knowledge gained? - What's interesting was in class? | Participate in a dialogue with the teacher (organized discussion). Analyze and reflect on their achievements. nine0003
| ||
Pike perch is a very common fish in Akhtuba.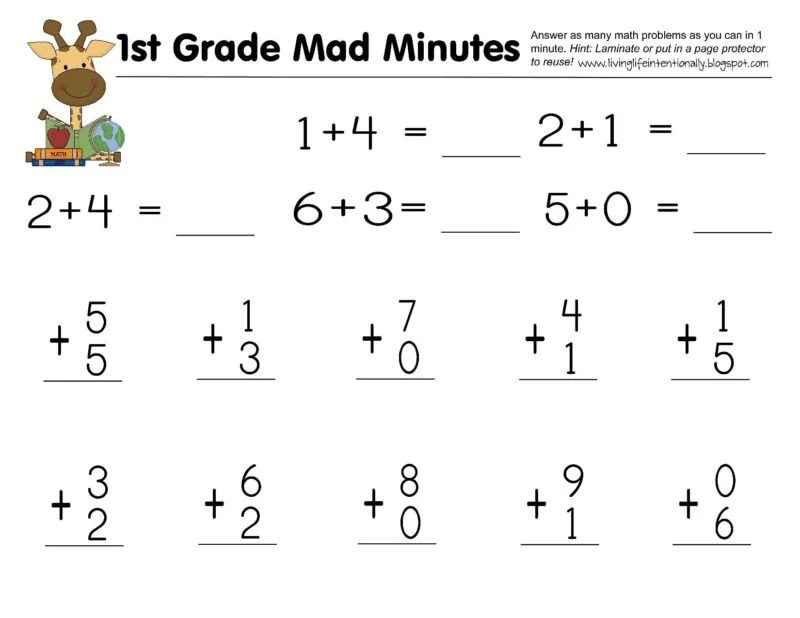 Has a distinctive greenish-gray in color, and its belly is white. In addition, on the sides are up to 12 dark brown transverse stripes. Pike perch can be up to 1 meters with a weight of up to 13 kg, but the main catch is individuals no longer than 50 cm and weighing no more than 3 kg. Lives in open water and rarely enters coastal zone. Adult zander feed on various types of young (perch, rudd and others). During the spawning season, the male guards his nests (located at a depth of no more than 30 cm), and also monitors fry
Has a distinctive greenish-gray in color, and its belly is white. In addition, on the sides are up to 12 dark brown transverse stripes. Pike perch can be up to 1 meters with a weight of up to 13 kg, but the main catch is individuals no longer than 50 cm and weighing no more than 3 kg. Lives in open water and rarely enters coastal zone. Adult zander feed on various types of young (perch, rudd and others). During the spawning season, the male guards his nests (located at a depth of no more than 30 cm), and also monitors fry
The honey colise lives in basins of the Ganges and Brahmaputra rivers (India and Bangladesh), in water bodies with low water level and high temperature. Colisa striped is found in the southern and southwestern part of front (western) India and in the basins of the rivers Indus, Ganges, Godavari, Mahapadi, Narmanda, mostly in small, well-lit sun reservoirs. Labiosa lives in the reservoirs of the Ayeyarwaddy River and in small rivers. East India and Burma. Lyalius is an inhabitant of the rivers Indus, Ganges, Brahmaputra, his the range reaches northern India, this fish prefers reservoirs with clean, oxygenated water, but cases of finding lalius and rice paddies are described fields
Some of these fish are widely distributed in aquarium culture (zebrafish, pink zebrafish).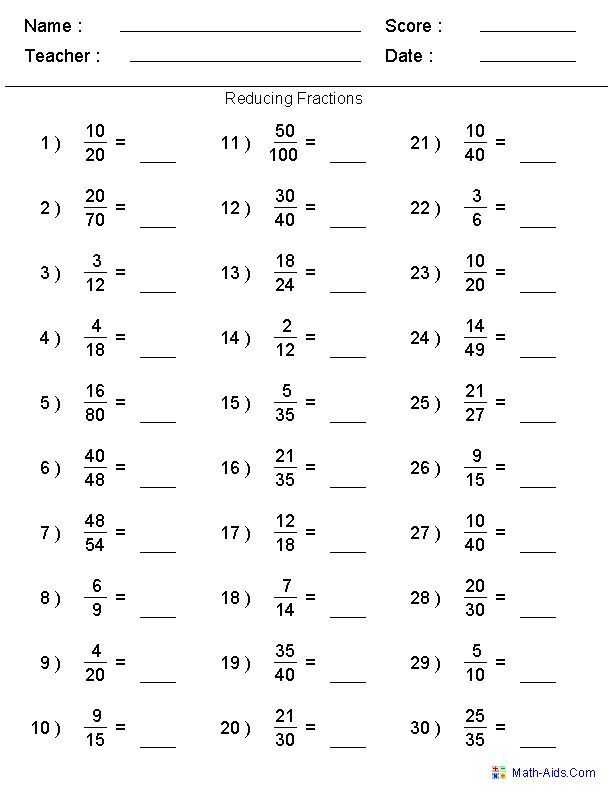 Danio rerio is used in biology as a model object for research. Danio fish can be kept in a common aquarium, spacious and closed at the top. Mandatory replacement 1/3-1/5 parts of the volume of water once a week for fresh. Recommended conditions: temperature 20-25 °C (4 degrees higher in a spawning tank), hardness 3-15`, acidity 6-7.5. The food is live and dry.
Danio rerio is used in biology as a model object for research. Danio fish can be kept in a common aquarium, spacious and closed at the top. Mandatory replacement 1/3-1/5 parts of the volume of water once a week for fresh. Recommended conditions: temperature 20-25 °C (4 degrees higher in a spawning tank), hardness 3-15`, acidity 6-7.5. The food is live and dry.

 Self-determination for activities
Self-determination for activities 

 nine0003
nine0003 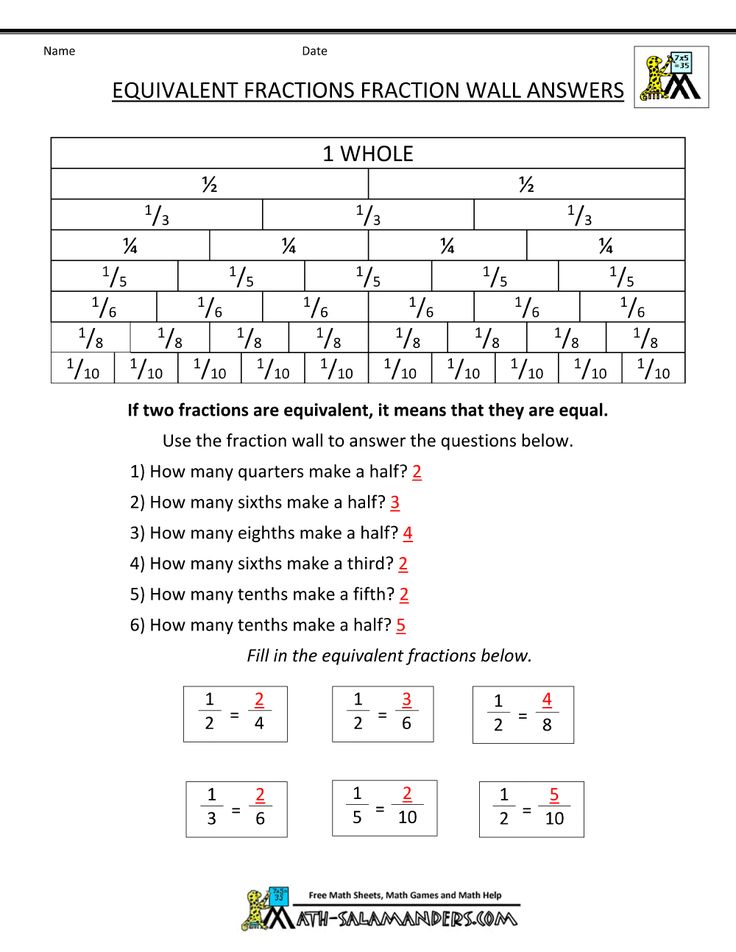
 Solving the problem. We conclude
Solving the problem. We conclude 

 3.
3.  I read, write down fractions.
I read, write down fractions. 
 Has a distinctive greenish-gray in color, and its belly is white. Pike perch can be up to 1 meters with a weight of up to 13 kg. Can be found along the coast. adult zander feed on various types of young animals (perch, rudd and other ). Male caring dad, guarding his nest (located on depth no more than 30 cm), also monitors fry
Has a distinctive greenish-gray in color, and its belly is white. Pike perch can be up to 1 meters with a weight of up to 13 kg. Can be found along the coast. adult zander feed on various types of young animals (perch, rudd and other ). Male caring dad, guarding his nest (located on depth no more than 30 cm), also monitors fry  Likes fresh water, so you need to week change 1/3 of the water . Recommended conditions: temperature 20-25 °C .Korm live and dry.
Likes fresh water, so you need to week change 1/3 of the water . Recommended conditions: temperature 20-25 °C .Korm live and dry. 
 nine0003
nine0003 
 What are shares?
What are shares? 
 nine0003
nine0003  nine0003
nine0003 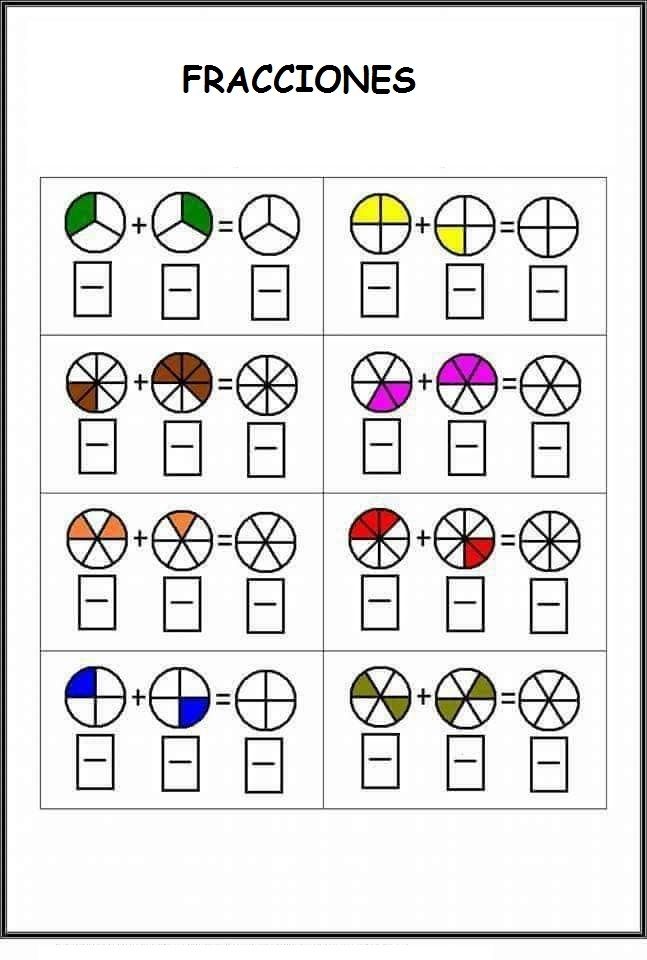
 nine0003
nine0003