Concept of math
How Math Concepts and Math Skills Work Together and Why You Should Care
Sometimes people use the words “math skills” and “math concepts” interchangeably. They are not the same, but they do go hand and hand. Understanding how new math skills and concepts work together will give you a new perspective on math education and math challenges.
Definition of Skills
Skills are actions. People do them. People master math skills the same way they master musical skills or sports skills. They practice the actions and steps intentionally, correctly and frequently. They may even go to a special place to practice the skill.
Definition of Concepts
Concepts are ideas. People understand them. People learn concepts by thinking, discussing, reading, listening and/or writing about them.
Similarities Between Skills and Concepts
Both can be learned and they are each the point of education.
Both have a natural progression, or order, in which they should be taught.
Both are a huge component of math success.
1. Education
Most of education focuses on skills. Skills are easier to observe and grade because a person can demonstrate skills. Proving that you understand a concept is difficult. Teachers try to measure a student’s understanding by asking them to write about, discuss or draw concepts. Even then, students are still limited in showing their understanding by their language, writing or artistic skills. For example, most adults in the U.S. understand the concept of basic nutrition. How many adults in the U.S. could explain basic nutrition in a foreign language? Our limited skill set in the foreign language would limit how well we could prove our understanding.
Retaining concepts is easier than retaining skills. What happens when we don’t practice a skill? We lose the skill. Most adults can think of a skill that they frequently practiced as child, but don’t practice now (piano for example). Chances are that their younger selves were much better at that skill than their current self.
If you understand a concept, you have that understanding forever. You may need to think about it before you remember all the details, but the underlying concept will always be there. You will always be able to build upon the concepts you understand and your previous understanding makes learning easier and faster.
2. Progression
Skills build on top of each other. When learning to swim, most children learn to float around the same time as learning to flutter kick. They are both beginning level skills. They must master all the beginning level skills before attempting an advanced skill, like swimming in open water or attempting the butterfly stroke.
Concepts are also best taught progressively. Our brains use neural pathways to make connections. Our understanding of underlying concepts pave the way for understanding new concepts. The deeper and broader our knowledge base is, the easier it is for us to connect it to new concepts.
3. Math Success
Skills are the “how-to” parts of math.
Children should master adding and subtracting before starting multiplication. They must know how to multiply and divide before attempting percentages.
Concepts are the underlying ideas of math. Concepts are ideas like equality and symbolic representation. Many math concepts build upon each other. A child who has a solid understanding of the relationship of quantity and numbers, or “number sense”, will find the concept of “wholes and parts”, naturally makes sense.
How Skills and Concepts Work Together
Understanding concepts makes learning skills easier. Mastering skills, especially thinking skills, makes learning concepts easier. Each prepares the brain differently.
People might be able to do a skill without understanding “why” it works. Understanding why a skill works in sports is helpful, but not critical. Can you imagine swim lessons at the neighborhood pool to 5 year-olds including a study of fluid dynamics and propulsion?
People might also have a concept about something, without the skill to execute it.
Many adults understand the concept of bacteria and wound maintenance, but they still go to a skilled professional to get stitches. Some people call this “book smarts” versus “street smarts.” Understanding the why helps you build your knowledge base and your retention of skills.
Most people in life don’t care about what others understand. They care about what others do. A conceptual understanding helps build skill sets faster.
How Skills and Concepts Work Together in Math
Math skills and concepts should be taught simultaneously. Children who learn math skills without learning the underlying concepts, will find math can get very confusing. Their lack of understanding will limit their ability to apply math in a variety of problem solving situations. Without understanding a concept, we are forced to rely on remembering and practicing a skill. For example, let’s say a 5th grader learned how to divide fractions using the "copy, dot, flip" method but never understood the concept behind why that process worked.
Then the 5th grader progresses to 6th grade and stops practicing dividing fractions because they are learning new skills. When that child stops practicing, he will then likely forget how to divide fractions. This will be a problem when dividing fractions comes up again in algebra and advanced math. The child who has both the skills and the concepts will be much better prepared.
Children who learn math concepts without learning the skills, will struggle to apply what they know. Knowing the difference between concepts and skills helps to identify the problems that arise in math.
How we Approach Skills and Concepts at Mathnasium
At Mathnasium of Littleton we focus on teaching foundational math skills like Counting, Numerical Fluency (adding and subtracting and multiplication facts), time and money and the order of operations while also teaching concepts such as Number Sense, Wholes and Parts, and Proportional Thinking. These latter are concepts that also involve skills.
Additionally, thinking is a skill that must be practiced. Thinking skills enable a child to learn the concepts and we never neglect to teach concepts. We encourage our students to ask us questions and we ask them questions to promote deep thinking and conceptual understanding.
The progression of skills and concepts and how they work together is exactly why at Mathnasium of Littleton, we work hard to close math gaps.
For more information about our methods, click here. The result is stronger math skills with a deeper understanding of mathematical concepts. Our motto is: “We Make Math Make Sense!”
Give us a call today! 303-979-9077
Articles referenced in this post:
Practice Makes Perfect so Perfect Practice is Essential
Multiple Practice Sessions Provide Better Learning than Cramming
Where Does your Child Go To Practice Math?
Is Mathematical Understanding Really Necessary?
Worried Your Child has Not Memorized the Multiplication Tables?
4 Tips for Figuring Out Percentages the Easy Way
Learning Math Symbols in Elementary School Takes Time
Prepare Your Child for Calculus Starting in Second Grade
Is Your Child Dependent on Algorithms and is that a Bad Thing?
Why Does Mathnasium of Littleton Focus on Foundational Math Skills?
Counting … Its More Complex Than You Imagine
Teaching Number Sense
Focus on Math: Wholes and Parts
Focus on Math: Proportional Thinking
What is Numerical Fluency?
Multiplication Fluency is Coming to Mathnasium of Littleton
Focus on Math: Learning About Money
Does Your Child Ask Questions in Math Class?
Focus on Math: Order of Operations
Understanding Mathematical Reasoning
Understanding Math Learning Gaps
4 Crucial Basic Math Concepts
Search my site:
Your child's success in learning Math depends on mastering these five crucial concepts.
They are essential for building a strong foundation in Math. They help your child understand and make sense of numbers and problem-solving; they strengthen his or her number sense.
The good news is that it is very easy to help your child understand these concepts. The key is to practice a few minutes several times a day. Make it a family affair so your child does not feel overly stressed. Your child or children will remember the lessons better if they had fun with it.
1. Understanding Numbers
The first concept your child must master is knowing how to read, write and understand numbers. Print out 2 sets of the number chart here. Use one set as a reference. Cut out the individual numbers from the second set.
Some ideas for you:
- read and write numbers
- recite a list of numbers forwards and backwards
- what number comes before or next
- arrange numbers in order (start with consecutive numbers)
- even and odd numbers
Print out this set of place numbers for older children.
2. More, Less or Equal
The next important concept in Math is to know how 2 numbers compare; whether one number is more, less or equal to another number.
Numbers represent quantity. Your child must first understand these types of questions.
- Amanda has 12 stickers. Benjamin has 9 stickers. Who has more stickers?
- Jack has 35 stamps. Alan has 19 stamps. Who has fewer stamps?
- Sam has 107 balloons. Jodie has 90 balloons. Who has more balloons? How many more?
- Charlie is 30-years-old. Mary is 17-years-old. Who is younger? How many years younger?
Followed by these types of questions:
- Rodney has $3 more than Janice. How much money does Rodney have if Janice has $26?
- I have 7 fewer pencils than books.
How many books do I have if I have 20 pencils? - John spent $11 less than Mark. How much did they spend altogether if Mark spent $15?
And these types of questions:
- 5 pencils cost the same as 2 books. Which is more expensive, the pen or the book?
- 6 apples cost the same as 10 oranges. What is the cost of 1 apple if 1 orange costs $0.60?
- Mary and James have the same amount of money. Mary spent half of her money. James spent one-third of his money. Who spent more money?
No matter how difficult the questions get, they can all be broken down into simple comparisons of more, less or equal.
3. Knowing Which Operation to Use
In Basic Maths there are actually only 4 operations your child needs to know: addition, subtraction, multiplication and division. Here are some clues to help your child decide which of these to use. Print the following graphic for your child to use as a guide.
4. Different Ways to Get to the Answer
When it comes to Math, many children assume there is only one way or method to use to come up with the correct answer. If your child does not understand the teacher's method, he or she assumes that it is because he or she too stupid to understand.
You must help your child realize that there can be different ways of understanding a problem and different strategies can be used to find the answer. However, though the strategies may differ, the answer to the question must always be the same.
Play this family game: Write out simple word problems on cards. Read out a problem, then each person explains how he or she comes up with the answer.
Example
Mary has 9 sweets. She bought a few more sweets and now she has 13 sweets. How many sweets did Mary buy?
Some methods of solving:
- Say '9' then count '10, 11, 12, 13' on your fingers. Since you used 4 fingers to count, the answer is 4.
- Draw 9 circles in a row. Draw 13 circles in a second row below the first, making sure you line them up exactly. Compare the two rows and point out there are 4 extra circles in the second row. The answer is 4.
- Write the numbers 1 to 13 on a number line. Point out that after the number 9, there are 4 more numbers to reach the number 13. The answer is 4.
Play this game often and your child will become more flexible and logical in his or her thinking. He or she will automatically look for different ways to solve a hard problem. This will surely help in tackling complicated Math problems later on in school. Teach your child to ask "Is there another way of solving?" when he or she cannot understand one explanation.
This will help them realize that failure to understand does not mean they are cannot learn Math.
After your child has mastered this Math concept, introduce deliberate mistakes in your reasoning to see if your child can spot and correct them.
But do this only after your child has thoroughly understood the different strategies or you'll only end up confusing your child.
For older children, you can help them understand the properties of the different operations of addition, subtraction, multiplication and division.
Sometimes the order that these operations are used does not matter. Sometimes it does. Discuss the different strategies to find out when order matters, and when it doesn't.
Example
Max bought 3 boxes of pencils. Each box contains 12 pencils. He gave 5 pencils to his brother and 8 pencils to his sister. How many pencils does Max have left?
Methods of solving:
- To find the total number of pencils at first, you can multiple (3 x 12) or add (12 + 12 + 12).
- To find the number of pencils left, you can add first (5 + 8) and then subtract
(36 - 13). - Or you can subtract only (36 - 5 - 8).
I hope these strategies help your child excel in Maths.
Share them with other parents whose children struggle with Maths.
The Concept for the Development of Mathematics Education in the Russian Federation
1. Approve the attached Concept for the Development of Mathematics Education in the Russian Federation.
2. The Ministry of Education and Science of Russia to approve within 3 months the action plan for the implementation of the Concept for the Development of Mathematical Education in the Russian Federation.
Chairman of the Government
of the Russian Federation
D.Medvedev
red.: the text of the order is published on the official Internet portal of legal information http://www.pravo.gov.ru, 27.12.2013.
The Concept for the Development of Mathematics Education in the Russian Federation
This Concept is a system of views on the basic principles, goals, objectives and main directions for the development of mathematics education in the Russian Federation.
I. The importance of mathematics in the modern world and in Russia
Mathematics occupies a special place in science, culture and social life, being one of the most important components of world scientific and technological progress. The study of mathematics plays a system-forming role in education, developing a person's cognitive abilities, including logical thinking, influencing the teaching of other disciplines. A high-quality mathematical education is necessary for everyone for his successful life in modern society. The success of our country in the 21st century, the efficiency of the use of natural resources, the development of the economy, the defense capability, the creation of modern technologies depend on the level of mathematical science, mathematical education and mathematical literacy of the entire population, on the effective use of modern mathematical methods. Without a high level of mathematical education, it is impossible to fulfill the task of creating an innovative economy, the implementation of long-term goals and objectives of the socio-economic development of the Russian Federation, and the modernization of 25 million high-performance jobs by 2020.
Developed countries and countries that are currently making a technological breakthrough are investing significant resources in the development of mathematics and mathematical education.
Russia has considerable experience in mathematics education and science, accumulated in 1950-1980. The accelerated development of mathematical education and science, providing a breakthrough in such capacious strategic areas as information technology, modeling in mechanical engineering, energy and economics, forecasting natural and man-made disasters, biomedicine, will help to improve the position and increase the prestige of Russia in the world. The system of mathematical education that has developed in Russia is a direct successor to the Soviet system. It is necessary to preserve its advantages and overcome serious shortcomings. Raising the level of mathematical education will make the life of Russians in modern society more fulfilling, and will provide the need for qualified specialists for science-intensive and high-tech production.
II. Problems of the development of mathematical education
In the process of social changes, the problems of the development of mathematical education and science have become more acute, which can be combined into the following main groups.
1. Problems of a motivational nature
Low educational motivation of schoolchildren and students is associated with public underestimation of the importance of mathematical education, overload of educational programs of general education, vocational education, as well as assessment and methodological materials with technical elements and outdated content, with the lack of curricula that meet the needs of students and the actual level of their training. All this leads to a discrepancy between the tasks of the intermediate and state final certification and the actual level of training of a significant part of students.
2. Content problems
The choice of the content of mathematical education at all levels of education continues to become obsolete and remains formal and out of touch with life, its continuity between levels of education is broken.
The needs of future specialists in mathematical knowledge and methods are not sufficiently taken into account. The actual absence of differences in curricula, assessment and methodological materials, in the requirements of intermediate and state final certification for different groups of students leads to a low efficiency of the educational process, the substitution of training by "training" for an exam, ignoring the actual abilities and characteristics of students' preparation. Mathematical education in educational institutions of higher education is divorced from modern science and practice, its level is falling, which is due to the lack of a mechanism for timely updating the content of mathematical education, insufficient integration of Russian science into the world.
3. Personnel problems
The Russian Federation lacks teachers and professors of higher educational institutions who can teach mathematics in a quality manner, taking into account, developing and shaping the educational and vital interests of various groups of students.
The existing system of training, professional retraining and advanced training of pedagogical workers does not meet modern needs. Graduates of educational institutions of higher education with a pedagogical orientation, for the most part, do not meet the qualification requirements, professional standards, have little experience in teaching and experience in the application of pedagogical knowledge. The training received by the overwhelming majority of students in areas of mathematical and pedagogical specialties does not contribute to either intellectual growth or the requirements of pedagogical activity in general education organizations. Teachers of educational organizations of higher education, for the most part, are cut off both from modern areas of mathematical research, including applied ones, and from the applications of mathematics in scientific research and applied developments of their educational organization of higher education. The system of additional professional education for teachers is not effective enough and is often just formal in terms of improving mathematical education.
III. Goals and objectives of the Concept
The purpose of this Concept is to bring Russian mathematical education to a leading position in the world. Mathematics in Russia should become an advanced and attractive field of knowledge and activity, the acquisition of mathematical knowledge should be a conscious and internally motivated process.
The study and teaching of mathematics, on the one hand, ensure the readiness of students to apply mathematics in other areas, on the other hand, they have a backbone function, significantly affect the intellectual readiness of schoolchildren and students for learning, as well as the content and teaching of other subjects.
The objectives of the development of mathematical education in the Russian Federation are: high achievements of science and practice;
ensuring that there are no gaps in basic knowledge for each student, forming among the participants of educational relations the attitude “there are no children incapable of mathematics”, ensuring confidence in an honest and adequate state final certification, providing teachers with diagnostic tools (including automated ones) and overcoming individual difficulties;
ensuring the availability of publicly available information resources necessary for the implementation of curricula for mathematical education, including in electronic format, tools for the activities of students and teachers, the use of modern technologies in the educational process;
Improving the quality of the work of mathematics teachers (from pedagogical workers of general education organizations to scientific and pedagogical workers of educational institutions of higher education), strengthening the mechanisms for their material and social support, providing them with the opportunity to refer to the best examples of Russian and world mathematical education, the achievements of pedagogical science and modern educational technologies, creation and implementation of their own pedagogical approaches and copyright programs;
support for leaders in mathematics education (organizations and individual teachers and scientists, as well as structures formed around leaders), identifying new active leaders;
providing highly motivated students with outstanding mathematical abilities with all the conditions for the development and application of these abilities;
popularization of mathematical knowledge and mathematical education.
IV. Main directions of implementation of the Concept
1. Preschool and primary general education
The system of curricula for mathematical education in preschool and primary education with the participation of the family must provide:
child support) for pupils to master the forms of activity, primary mathematical concepts and images used in life;
in primary general education - a wide range of mathematical activity (classes) of students both in the classroom and in extracurricular activities (primarily solving logical and arithmetic problems, building algorithms in a visual and gaming environment), material, informational and personnel conditions for development students using mathematics.
2. Basic general and secondary general education
Mathematics education should:
provide each student with the opportunity to achieve the level of mathematical knowledge necessary for further successful life in society;
to provide each student with developing intellectual activity at an accessible level, using the beauty and fascination inherent in mathematics;
ensure the number of graduates necessary for the country, whose mathematical training is sufficient to continue their education in various areas and for practical activities, including teaching mathematics, mathematical research, work in the field of information technology, etc.
students in accordance with their requests for the level of training in the field of mathematical education.
Each student, regardless of location and living conditions, must be given the opportunity to achieve any level of training, taking into account his individual needs and abilities. The possibility of achieving the required level of mathematical education should be supported by the individualization of education, the use of e-learning and distance learning technologies. The possibility of achieving a high level of training should be ensured by the development of a system of specialized general education organizations and specialized classes, a system of additional education for children in the field of mathematics, a system of mathematical competitions (olympiads, etc.). Relevant programs can also be implemented by higher education organizations (including within the framework of existing and created specialized educational and scientific centers of universities, as well as network forms for the implementation of educational programs).
Achieving any of the levels of training should not prevent the individualization of education and close the possibility of continuing education at a higher level or changing the profile.
It is necessary to stimulate an individual approach and individual forms of work with lagging students, primarily involving teachers with extensive experience.
Improving the content of mathematics education should be ensured primarily through advanced training and additional professional education of teachers based on the leadership practices of mathematics education, formed in general education organizations.
3. Vocational education
The system of vocational education should provide the necessary level of mathematical training for the needs of mathematical science, economics, scientific and technological progress, security and medicine. To do this, it is necessary to develop modern programs, to include the main mathematical areas in the corresponding priority areas for the modernization and technological development of the Russian economy.
Students studying mathematics, including information technology, and their teachers should be involved in mathematical research and projects. Teachers of mathematical faculties of classical universities need to conduct fundamental research recognized by the professional community, and their students should devote much more time than at present to solving creative educational and research problems. Teachers of mathematical departments of technical universities should conduct research in fundamental mathematics or in applied specialized areas, carry out work commissioned by organizations in which students also take part (similarly for economic and other educational organizations of higher education), teachers of mathematical departments of pedagogical universities should work with schoolchildren , participate in the development of certification materials, teaching aids for schoolchildren. Students (including those preparing to become teachers and educators in organizations engaged in educational activities) need to solve problems of elementary mathematics in their zone of proximal development, to a much greater extent than today, to practice at school, using this activity as a basis and motivating factor for obtaining psychological and pedagogical knowledge.
The interaction of the bodies exercising management in the field of education, educational organizations of higher education and general educational organizations should be focused on supporting the arrival of the best graduates of mathematical faculties of pedagogical educational organizations of higher education, graduates of specialized specialties of classical universities. It is necessary to provide the best graduates who studied under the programs of mathematical orientation of educational institutions of higher education and who have inclinations and abilities for pedagogical work with the opportunity to teach in an educational institution of higher education.
4. Additional professional education, training of scientific and pedagogical workers of educational organizations of higher education and scientific workers of scientific organizations, mathematical science , including internships in organizations - leaders in fundamental and applied research in the field of mathematics and mathematics education.
It is important to support world organizations in Russia that solve the problem of training researchers and teachers of the highest level, including the creation of world-class scientific and educational centers inviting scientists to conduct research and participate in the development of educational programs.
Higher education institutions and research centers should ensure the advanced level of fundamental and applied research in mathematics and its use in mathematics education. It is necessary to strengthen the integration of Russian mathematical research into world science, to ensure that the mathematical faculties of leading Russian universities achieve high positions in world rankings, as well as to increase the quality, quantity and citation of the works of Russian mathematicians, and the attractiveness of Russian mathematical education for the best foreign students and professors. The mobility of students, graduate students and young candidates of sciences should increase, cooperation between educational institutions of higher education and research institutes should be developed.
To solve the problems of this Concept, it is planned to finalize the system of labor assessment, taking into account the specifics of the activities and international practice of assessing the work of mathematics teachers, scientific and pedagogical workers of educational institutions of higher education and scientists of scientific organizations employed in the profile of mathematics.
Educational organizations of higher education and research centers should participate in the work on mathematical education and popularization of mathematical knowledge among the population of Russia.
5. Mathematical education and popularization of mathematics, additional education
For mathematical education and popularization of mathematics, it is planned to:
provide state support for the accessibility of mathematics for all age groups of the population;
creation of a public atmosphere of a positive attitude towards the achievements of mathematical science and work in this area, understanding the importance of mathematical education for the future of the country, the formation of pride in the achievements of Russian scientists;
providing continuous support and increasing the level of mathematical knowledge to meet the curiosity of a person, his general cultural needs, the acquisition of knowledge and skills used in everyday life and professional activities.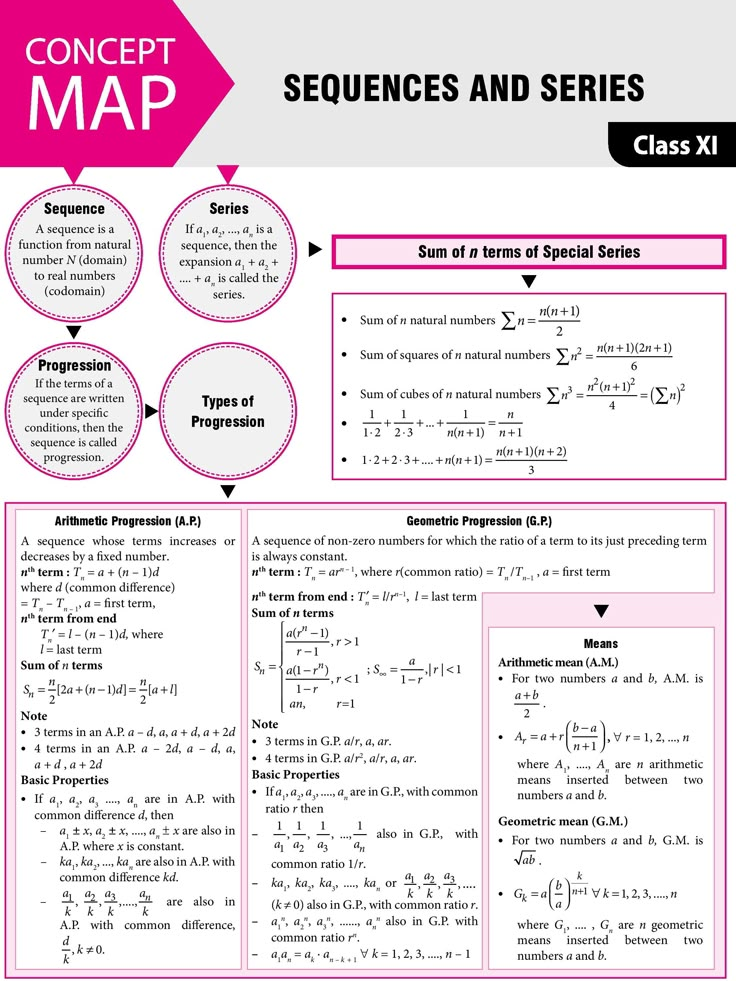
The system of additional education, including mathematical circles and competitions, is an important part of the Russian tradition of mathematical education and should be provided with state support. At the same time, such new forms as obtaining mathematical education in a distance form, interactive museums of mathematics, mathematical projects on Internet portals and social networks, and professional mathematical Internet communities should be developed.
V. Implementation of the Concept
The implementation of this Concept will provide a new level of mathematical education, which will improve the teaching of other subjects and accelerate the development of not only mathematics, but also other sciences and technologies. This will allow Russia to achieve its strategic goal and take a leading position in world science, technology and economics.
The implementation of this Concept will contribute to the development and testing of mechanisms for the development of education applicable in other areas.
| Catalog Book search Electronic applications Authorization Newsletter subscription Poems about us B wealth These difficulties have already turned into meanings. Feedback Send a message from the site Partners
|
Decree of the Government of the Russian Federation of December 24, 2013 N 2506-r approved the Concept for the Development of Mathematical Education in the Russian Federation. Decree of the Government of the Russian Federation N 2506-r dated December 24, 2013 The Concept notes the role of mathematics in the modern world and in Russia. It is said that “mathematics occupies a special place in science, culture and public life, being one of the most important components of world scientific and technological progress. The study of mathematics plays a system-forming role in education, developing a person's cognitive abilities, including logical thinking, influencing the teaching of other disciplines. A high-quality mathematical education is necessary for everyone for his successful life in modern society. Raising the level of mathematical education will make the life of Russians in modern society more fulfilling, and will provide the need for qualified specialists for science-intensive and high-tech production. There are three groups of problems in the development of mathematics education:
The training received by the overwhelming majority of students in the areas of mathematics and pedagogy does not contribute to either intellectual growth or the requirements of pedagogical activity in general education organizations. The third section of the Concept proclaims the objectives of the development of mathematical education in the Russian Federation:
The fourth section reflects the main directions of the development of mathematical education in basic and high school. In particular, it is said that mathematical education should:
Each student, regardless of location and living conditions, must be given the opportunity to achieve any level of training, taking into account his individual needs and abilities. The possibility of achieving the required level of mathematical education should be supported by the individualization of education, the use of e-learning and distance learning technologies. The possibility of achieving a high level of training should be ensured by the development of a system of specialized general educational organizations and specialized classes, a system of additional education for children in the field of mathematics, a system of mathematical competitions (olympiads, etc. 
|
It is a system of views on the basic principles, goals, objectives and main directions for the development of mathematical education in the Russian Federation: 
” 
The actual absence of differences in curricula, assessment and methodological materials, in the requirements of intermediate and state final certification for different groups of students leads to a low efficiency of the educational process, the substitution of training by "training" for an exam, ignoring the actual abilities and characteristics of students' preparation. Mathematical education in educational institutions of higher education is divorced from modern science and practice, its level is falling, which is due to the lack of a mechanism for timely updating the content of mathematical education, insufficient integration of Russian science into the world. 
Graduates of educational institutions of higher education with a pedagogical orientation, for the most part, do not meet the qualification requirements, professional standards, have little experience in teaching and experience in the application of pedagogical knowledge. 
