Maths concepts list
Branches, Fundamentals, Important Topics, Preparation Tips
Math is the science of quantity, pattern, order, structure and relation that has continuously evolved from basic practices of counting, measurements and symmetric study of shapes. It primarily involves applying logical reasoning and quantitative computation to find optimal solutions to problems. It has been worldwide recognized as an indispensable computational tool in the field of engineering, biology, medicine and natural sciences.
Math As A Subject
Math as a subject is an important part of the curriculum that plays a significant role in shaping a child’s future. It involves studying many useful concepts and topics relevant to practical life. Although math is an interesting subject, students often find it boring and complex due to the way it is taught conventionally. Cuemath helps students to explore and understand fundamental concepts in a fun and intuitive manner.
Fundamentals of Math
Fundamentals of math are the basic building blocks that help students form a solid mathematical foundation. Math learning entirely relies on the understanding of these fundamental concepts. If children lack the basic understanding of division or subtraction, then algebra automatically becomes confusing for them. Therefore, it is imperative that children must have a crystal clear knowledge of all fundamentals of math.
- Addition and Subtraction of Whole Numbers
- Multiplication and Division of Whole Numbers
- Exponents, Roots, and Factorization of Whole Numbers
- Introduction to Fractions and Multiplication and Division of Fractions
- Addition and Subtraction of Fractions, Comparing Fractions, and Complex Fractions
- Decimals and Fractions
- Ratios and Rates
- Techniques of Estimation
- Measurement and Geometry
- Signed Numbers
- Algebraic Expressions and Equations
Branches of Mathematics
Mathematics involves complex studies of interlinked topics and several concepts that overlap each other.
Generally, it can be categorized into the following branches:
Arithmetic
Arithmetic is the most basic branch of mathematics that deals with the elementary aspects of numbers, mensuration and numerical computations. This term is derived from the Greek word ‘arithmos’ which means number. It generally involves studying numbers and their relationships to solve problems that include the operations of addition, multiplication, subtraction, division, extraction of roots and raise to power.
Algebra
Algebra is an important and ancient branch of math that covers basic operations and symbols to represent numbers in formulas and equations. The word Algebra means the science of restoring and balancing. Learning algebra enables students to understand many real-life phenomena around them. It is a symbolic representation of numbers and how they work together to provide structure to equations. It forms the basis for advanced study in many fields like science, medicine, engineering, etc.
It allows mathematicians to write formulas and solve maths problems more efficiently.
Geometry
Geometry is the branch of math which deals with computation of various dimensions of solid shapes including height, width, area, volume, perimeter and angles. It has several useful applications from the construction of homes to interior designs.
Trigonometry
Trigonometry is an important branch of math that involves studying the relationship between angles, lengths, heights and distance. The applications of trigonometry can be found in many spheres including architecture, physics, surveying, electronics, satellite navigation, astronomy and engineering.
List of Branches of Maths
Important Math Topics
A thorough understanding of all important math topics will benefit students throughout their lifetime. Some of the essential math concepts that students must have an in-depth understanding of are based on the topics listed below.
- BODMAS Rule
- Prime and Composite numbers
- HCF and LCM
- Basic Menstruation
- Divisibility Rules
- Decimal and Fractions
- Ratio and Proportion
- Three-dimensional shapes
- Geometry
- Probability
Math Calculators
Students often find math challenging due to complex mathematical calculations. Math Calculators are handy tools to resolve all such problems. It makes calculations simple and quick. With the use of math calculators, calculations ranging from elementary arithmetic operations to complicated equations can be solved within a few seconds.
List of important calculators for students to solve problems quickly and get accurate solutions.
Important Math Formulas
Math formulas are expressions created after several years of research to help solve problems easily. Performing simple numerical operations like addition, subtraction and division are easy.
However, to solve algebraic expressions, and other complex operations we use mathematical formulas. These are quite useful in obtaining the answers precisely. Cuemath provides formulas for each math topic along with the illustrated steps of equations for students to understand them logically.
List of important formulas that students must learn and memorize.
Tips And Tricks To Learn Math Fast
Although math is a vast subject there are some tips and tricks to learn math fast. These tips and tricks will help students improve along their math journey.
Clear All Basic: The first and foremost step in learning mathematics is to clearly understand all basics. It will not only allow you to learn math faster but will also help in establishing links between various math topics.
Set Objectives: After clearing all basics, set goals for what you need to focus on. Once you understand your objective, start working on it. Explore various resources that can help you improve and get well versed in those topics.
Practice Daily: Math requires daily practice, implementing a proper study routine will help in grasping concepts better.
Take Guidance: Heading in the right direction is necessary as it will ensure good results. Consider taking help from your teacher or a math tutor if you feel doubtful about topics and concepts.
FAQs on Math
What Exactly is Math?
Math is the science involving numbers, shapes and patterns which is present in almost everything around us. It helps us to derive analytical solutions to practical problems. It is applied in various fields such as engineering, finance, physical science, etc. It has a great impact in every domain of our life and we can find many mathematics applications around us.
How to Get Better at Math?
Getting better at math requires enforcing a study routine and analyzing mistakes. Students must try to understand and rectify their mistakes through daily practice. Doing so will also help them clear all their doubts.
Students with better mathematical abilities achieve higher academic success. Thus, it is crucial to cultivate math interest in children at an early age. Cuemath’s visually-enriched math concepts enhance a child’s interest in mathematics and make it easier to learn the subject.
Why is Math Important in Our Daily Life?
Math is highly important in our daily life as there are several applications of mathematics in real-world situations. Statistics or probability theory are examples of applied maths.
What are the Fundamentals of Mathematics?
Fundamentals of mathematics are the building blocks for a solid math foundation. Students must possess a clear knowledge of all the fundamentals of mathematics to study advanced mathematical concepts. These fundamentals of mathematics are given below.
- Addition and Subtraction of Whole Numbers
- Multiplication and Division of Whole Numbers
- Exponents, Roots, and Factorization of Whole Numbers
- Introduction to Fractions and Multiplication and Division of Fractions
- Addition and Subtraction of Fractions, Comparing Fractions, and Complex Fractions
- Decimals and Fractions
- Ratios and Rates
- Techniques of Estimation
- Measurement and Geometry
- Signed Numbers
- Algebraic Expressions and Equations
How many Branches of Mathematics do we Have?
The branches of mathematics can be broadly categorized as:
- Arithmetic: Arithmetic involves studying numbers and their relationships to solve problems that include the operations of addition, multiplication, subtraction, division, extraction of roots and raise to power.
- Algebra: Algebra is the symbolic representation of numbers that provides structure to equations. It forms the basis for advanced study in many fields like science, medicine, engineering, etc.
- Geometry: Geometry is the calculation of various dimensions of solids including height, width, areas, volumes, perimeter and angles. It has many practical applications in architecture and other fields.
- Trigonometry: trigonometry deals with the study of the relationship between angles, lengths, and heights. The applications of trigonometry can be found in many spheres including architecture, physics, surveying, electronics, satellite navigation, astronomy and engineering.
What are the Most Important Math Topics?
Some of the most important math topics are prime numbers, composite numbers, BODMAS rule, geometry, probability, divisibility rules, HCF, LCM, three-dimensional shapes, basic menstruation, decimal, fractions, ratio and proportion.
An in-depth understanding of all important math topics will enable students to score well in exams.
How Math is Used in Sports?
Math is used in sports to accumulate data, study conditions and generate performance statistics which are considered for planning and optimizing the training session. The data collected in these mathematical calculations is also helpful for taking strategic decisions based on the team’s performance.
How Maths is Related to Other Subjects?
Math is related to other subjects, especially chemistry, physics, computer science and engineering. In chemistry, mathematics is used to write and balance equations. In physics. It is applied to calculate mass, velocity and acceleration. In computer science, math is used to build algorithms and solve problems.
The 4 Major Math Concepts Your Kids Learn in PreK & Kindergarten
There are so many different topics our children learn throughout the year, just keeping up with each night’s new piece of homework and its latest ideas can feel very overwhelming.
As parents, we don’t get to see the major idea behind the “everyday” work and it can be frustrating to understand where each skill is going. In this first blog post of a continuing series, I will be highlighting the major math concepts that are taught at the different grade levels so we, as parents, can help to build and support these ideas at home.
Here are the four major math concepts taught in pre-kindergarten and kindergarten, along with exercises you can practice with your children to help reinforce their learning.
1. Counting. Students are beginning their experience with numbers through counting, number names and written numerals. Students are learning to count objects and understand a one-to-one correspondence. They are also starting to compare different sets of objects and use appropriate language.
Encourage your child to:
- Touch different objects and count out loud.
- Move objects from one group to another.
- Count a set of objects and “see” or “write” that corresponding number.
- Start to use comparing words: more than, less than, the same as.
2. Addition & Subtraction. This is the very early stage of adding and subtracting. The focus should be on developing an understanding of addition as “putting together and adding to,” and subtracting as “taking apart and taking from.” Students do not need to write equations at this young an age, but are encouraged to begin using them.
Encourage your child to:
- Tell stories about adding and subtracting. For example, for addition: Two bunnies sat on the grass. Three more bunnies hopped there. How many bunnies are on the grass now? For subtraction: Five apples were on the table. I ate two apples. How many apples are on the table now? Draw pictures about putting together and taking apart.
- Count to 10 and break apart numbers (decompose – math’s fancy word for “breaking apart”) into different combinations. For example, 5 can be seen as:
Image Credit: http://kindercraze.
com
3. Measurement & Data. Young children are beginning to describe and compare their physical world. They are starting to classify, sort and group objects into categories.
Encourage your children to:
- Compare two different objects using appropriate language. For example:
“John is taller than Sarah.”
“This tree is shorter than that tree.”
“My bag is heavier than your bag.” - Sort objects by color, size, material, etc.
- Describe their physical world with directional words: in front of, behind, on top of, next to, below, etc.
4. Geometry. Students are starting to look at and compare two-dimensional (flat) and three-dimensional (solid) shapes. They are using appropriate language to recognize different shapes and talk about their attributes.
Encourage your child to:
- Find 2-D shapes in the world: squares, circles, triangles, rectangles, and hexagons.
- Find 3-D shapes in the world: cubes, cones, cylinders, and spheres.
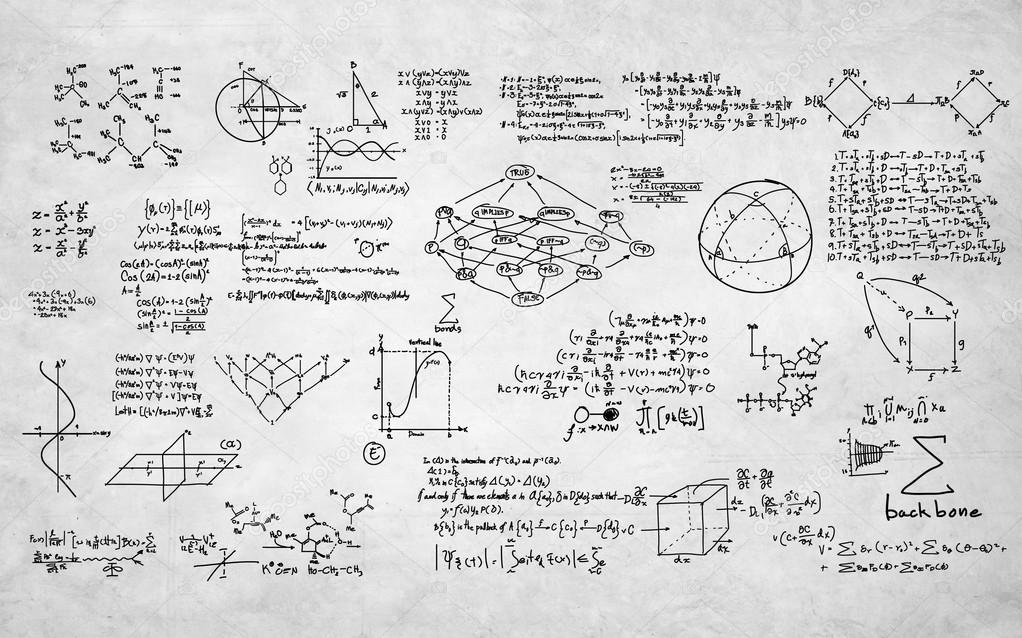
- Count the different number of sides, vertices, angles, etc.Model different shapes using clay, sticks, pipe-cleaners, etc.
See all blog posts in this series for information on what your child will learn in math class from preschool all the up through 8th grade.
Dictionary of terms in mathematics from A to Z - POCHEMUKHA.RU answers to questions.
Axiom is a statement accepted without proof.
The algebraic expression is a number of numbers, denoted by letters or numbers, and connected using the operations of addition, subtraction, multiplication, division, raising to a power, and extracting a root.
Abcissa (French word). One of the Cartesian coordinate points. Is the first. It is usually denoted by the symbol "X". First used by G. Leibniz in 1675 (German scientist).
Additivity. Some property of quantities. He speaks about the following: the value of a certain quantity corresponding to a full-fledged object is equal to the sum of the values of such a quantity that correspond to its parts in any division of a full-fledged object into parts.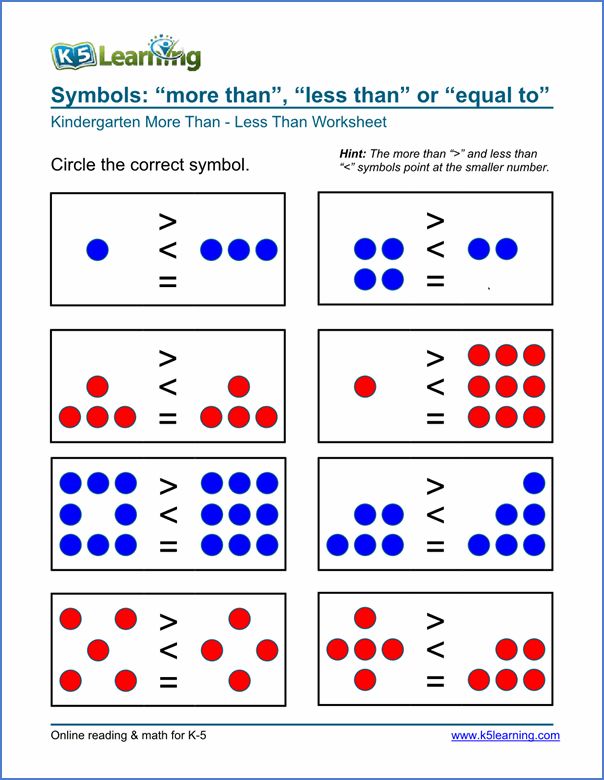
Adjunct. Fully corresponds to algebraic addition.
Axonometric. One of the ways to represent spatial figures on a plane.
Algebra. Part of mathematics that studies problems and solutions of algebraic equations. The term was first seen in the 11th century. Applied Muhammed ben-Musa al-Khwarizmi (mathematician and astronomer).
Argument (function). Variable value (independent) with which the value of the function is determined.
Arithmetic. The science that studies operations on numbers. Originated in Babylon, India, China, Egypt.
Asymmetric. Absence or violation of symmetry (inverse of symmetry).
Infinitely large value is greater than any given number.
An infinitely small value is less than any finite value.
Billion. One thousand million (one followed by nine zeros).
Bisector. A ray starting at the corner vertex (divides the corner into two parts).
Vector. Directed straight line segment. One end is the beginning of the vector; the other is the end of the vector. For the first time the term was used by W. Hamilton (an Irish scientist).
Vertical corners. A pair of corners that has a common vertex (formed by the intersection of two lines in such a way that the side of one corner is a direct continuation of the second).
Vector is a quantity characterized not only by its numerical value, but also by its direction.
Graph - a drawing that clearly depicts the dependence of one value on another, a line that gives a visual representation of the nature of the change in the function.
Hexahedron. Hexagon. The term was first used by Pappus of Alexandria (Ancient Greek scholar).
Geometry.
Part of mathematics that studies spatial forms and relationships. The term was first used in Babylon/Egypt (5th century BC).
Hyperbole. Open curve (consists of two unrestricted branches). The term appeared thanks to Apollonius of Perm (ancient Greek scientist).
Hypocycloid. This is the curve that the point of the circle describes.
Homothety. An arrangement between figures (similar), in which the lines connecting the points of these figures intersect at the same point (this is called the center of the homothety).
Degree. Unit for flat corner. Equal to 1/90 of a right angle. Measuring angles in degrees began more than 3 centuries ago. For the first time such measurements were used in Babylon.
Deduction. Form of thinking. With its help, any statement is deduced logically (based on the rules of the modern science of "logic").
Diagonal.
A line segment that connects the vertices of a triangle (they do not lie on the same side). First used the term Euclid (3rd century BC).
Discriminant. An expression made up of the values that define the function.
Fraction is a number made up of a whole number of fractions of one. It is expressed as the ratio of two integers m/n, where m is the numerator showing how many parts of the unit are in the fraction, and n is the denominator showing how many parts the unit is divided into.
Denominator. Numbers that make up a fraction.
The golden ratio is the division of a segment into two parts so that the larger part is related to the smaller one, as the entire segment is to the larger part. Approximately equal to 1.618. The criterion of beauty, used in architecture, etc. The term was introduced by Leonardo da Vinci.
Index. Alphabetic or numeric index.
With its help, mathematical expressions are supplied (this is done in order to distinguish from each other).
Induction. Method for proving a mathematical equation.
Int. Basic concept of mathematical analysis. It arose due to the fact that it took to measure volumes and areas.
Irrational number. A number that is not rational.
Leg. One of the sides of a right triangle that is adjacent to a right angle.
Square Regular quadrilateral (or rhombus). Each corner of the square is a straight line. All angles in a square are equal (by 90 degrees).
Mathematical constant. A value that never changes in its value. A constant is the opposite of a variable.
Cone. A body that is bounded by a single cavity by means of a conical surface. It intersects a plane (the plane is perpendicular to its axis).
Cosine.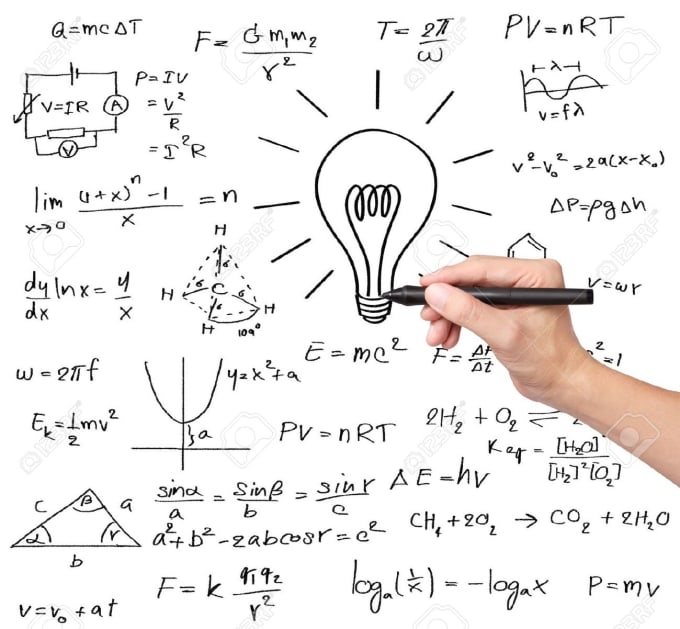
Is one of the trigonometric functions. The designation in mathematics/higher mathematics is cos.
Equation root - solution, the value of the unknown, found through known coefficients.
The constant is a constant value.
Coordinates are numbers that define the position of a point on a plane, surface, or in space.
Logarithm. Exponent "m". It must be raised to the "a" power in order to get some NT. For the first time, the logarithm was proposed by J. Napier.
Line is the common part of two adjacent surface areas.
Max. Highest value of the function.
Scale. The ratio of two linear dimensions to each other. Used in many modern industries. The main - cartography, geodesy.
Matrix. Rectangular table. It is formed using the set of a number (definite). Includes columns and rows (matrix structure).
For the first time, the term "matrix" appeared with the scientist J. Sylvester.
Median. A segment that connects the vertex of a triangle and its midpoint on the opposite side.
Minimum. Smallest value of the function.
Polygon. Geometric figure. The definition is a closed broken line.
Module. Absolute value (real number).
The set is a collection of elements united by some attribute.
Norm. The absolute value of the number.
The inequality is two numbers or expressions connected by signs (greater than) or (less than).
Oval. Convex, closed figure (flat).
Circle. Numerous points located on a plane.
Ordinate. One of the Cartesian coordinates. It is usually designated as the second.
Octahedron. Geometric figure.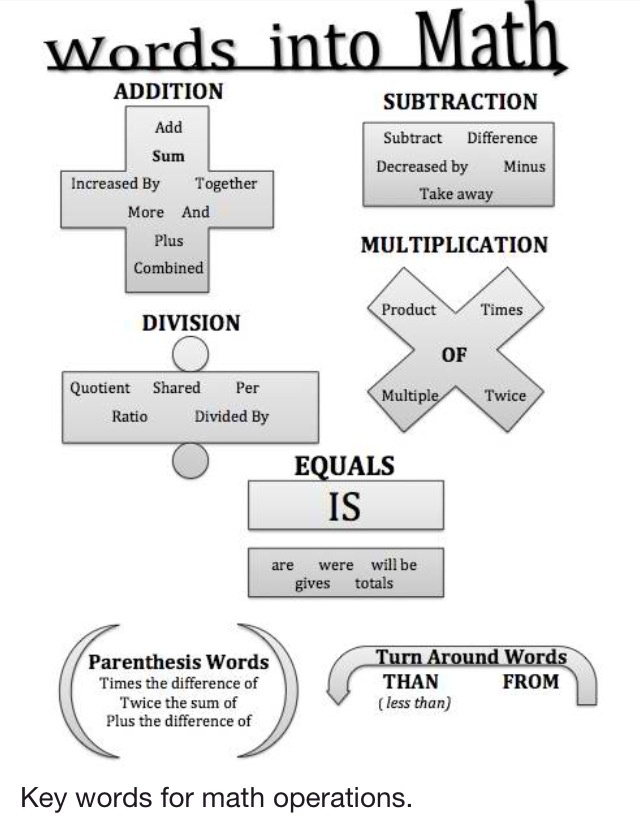
One of the five polyhedra (regular). The octahedron includes 8 faces (regular), 6 vertices and 12 edges.
Parallelepiped. Prism. The base is a parallelogram or a polyhedron (equivalent concepts). Has 6 edges. Each face is a parallelogram.
Parallelogram. Quadrilateral. Its opposite sides are parallel (in pairs). At the moment, there are 2 special cases of a parallelogram: a rhombus and a square. The main property of this geometric figure:
• Opposite sides are equal;
• Opposite angles are equal.
Perimeter. The sum of all sides of a geometric figure. For the first time it was possible to meet at Archimedes and Heron (ancient Greek scientists).
Perpendicular. A straight line that intersects a plane (any) at a right angle.
Pyramid. Polyhedron. Its base is a polygon. Any other face is a triangle (these faces have a common vertex). At the moment, pyramids can be of various types: triangular, quadrangular, and so on (they are distinguished by determining the number of corners).
Planimetry. One of the most important parts of elementary (simple) geometry. Planimetry studies the properties of figures that are on a plane. For the first time, the term was designated by Eculid (an ancient Greek scientist).
Plus. A sign that denotes a mathematical operation - addition. In addition, positive numbers are denoted with a plus. For the first time, the sign was introduced by J. Vidman (a famous Czech scientist).
Limit. The basic concept of mathematics. Denotes: a variable value approaches a constant value (defined) indefinitely. The term was first used by the famous scientist Newton.
Prism. Polyhedron. The first 2 faces are equal angles (these are the bases of the prism). The rest is the side faces.
Projection. One of the ways to depict spatial and flat figures.
Variable is a value whose numerical value changes according to a certain, known or unknown law.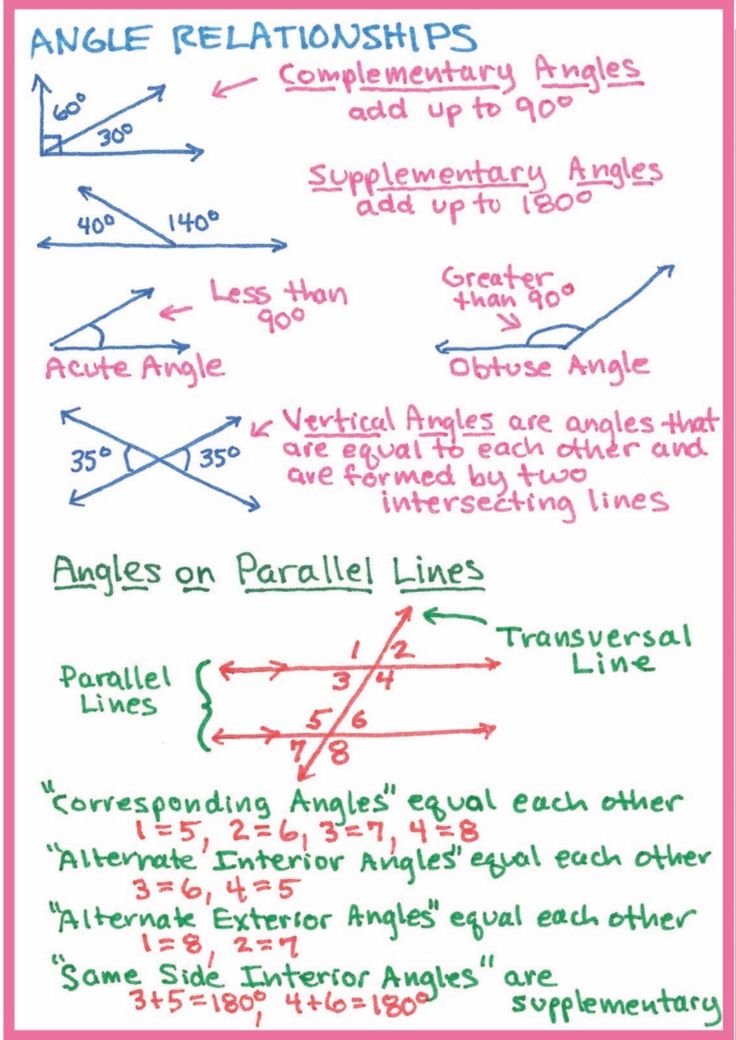
Plane is the simplest surface. Any line connecting two of its points belongs entirely to it.
Line is a set of points common to two intersecting planes.
Percentage is a hundredth of a number.
Radian. Angle unit.
Rhombus. Parallelogram. All sides of this figure are equal. A rhombus having right angles has the term "square".
Segment. Part of a circle (limited by a chord that connects the ends of the arc).
Secant. Trigonometric function. The designation in mathematics/higher mathematics is sec.
Sector. Part of a circle. Constrained by a circle + two radii (connects the ends of one arc to the center of the circle).
Symmetry - match.
Sinus. Trigonometric function. The designation in mathematics / higher mathematics is sin.
Stereometry. Part of elementary geometry. Engaged in the study of full-fledged spatial figures.
Tangent. Trigonometric function. The designation in mathematics/higher mathematics is tg.
Tetrahedron. Polyhedron, includes 4 triangular faces. Each vertex has 3 faces (converge at the vertices). A tetrahedron has 4 faces + 6 edges + 4 vertices.
Dot. Has no definite and final concept. Any point is denoted by letters A, B, C.
Triangle. Polygon (simple). Includes 3 tops + 3 sides;
Theorem is a statement to be proved based on axioms and previously proven theorems.
Identity is an equality valid for all values of the coefficients included in it.
Topology is a branch of mathematics that studies the properties of figures that do not change under any deformations carried out without ruptures and gluing.
An equation is a mathematical notation of the problem of finding the values of unknowns under which the values of two given functions are equal.
Angle. Geometric figure (flat). It is formed by two rays that come out of one point (the points are the corner vertices).
Factorial is the product of natural numbers from 1 to any given natural number n. Denoted n!. Factorial of zero o! = 1.
The formula is a combination of mathematical symbols that expresses a sentence.
Function is a numerical relationship between elements of two sets, in which one element of one set corresponds to a certain element of another set. Can be given by formula or graph.
Chord. A segment that connects 2 points on a circle.
Digits are symbols for numbers.
Central The middle of something (for example: a circle).
Cyl.
A body bounded by a cylindrical surface + parallel planes (two). For the first time, the concept of "cylinder" could be found in Euclid and Aristarchus.
Compass. Special instrument designed for drawing arcs, linear measurements and circles.
Numerator. A specific number with which a fraction is composed. The term was first used by Maxim Planuda (Byzantine scholar).
The number is one of the basic concepts of mathematics that arose in connection with the counting of individual objects.
Ball. Geometric body. It is the total set of all points in a certain space.
Exhibitor. Same as exponential function. The term was first introduced by G. Leibniz (a German scientist).
Ellipse. Oval curve. For the first time this term was introduced by Apollonius of Perga (an ancient Greek scientist).
50 "math" English words worth knowing
If you are going to study at an English-speaking school, college or university, you will have to take the international SAT or GMAT exams, which include a test of knowledge in mathematics.
In this video lesson you will find the 50 most necessary basic "math" words in English that will definitely come in handy.
Enjoy watching!
Symbols
- + add - plus
- - subtract - minus
- ÷ divide - division sign
- × multiply - sign of multiplication
- (>) – is greater than – greater than
- (<) - is less than - less than
- (≥) - is more than or equal to - greater than or equal to
- (≤) – is less than or equal to – less than or equal to
- (≠) – is not equal to – does not equal
Math
- + plus - addition
- - minus - subtraction
- ÷ divided by - division
- × times / multiplied by
- = equals / is - equals
Related article:
How to write dates in English: the difference between American and British versions
Geometric shapes
- Circle - a circle
- Triangle - triangle
- Square - square
- Rectangle
- Pentagon - Pentagon
- Hexagon
- Octagon
- Oval
- Cube - cube
- Cone
- Cylinder - cylinder
- Pyramid
- Sphere
Mathematics
- Algebra
- Geometry - geometry
- Trigonometry
- Statistics - statistics
- Probability theory
- Combinatorics
Article in the topic:
6 unusual sites for practicing and learning English online
General mathematical concepts
- Prime number - a prime number
- Odd number - odd number
- Even number - even number
- Equation - Equation
- Square root - square root
- Angle - angle
- Fractions
- Area - area
- Volume - volume
- Perimeter - perimeter
- Diameter - diameter
- Radius - radius
- Width - width
- Height - height
- Length - length
Next article
- English
