Algebra for 1st grade
Algebra in First Grade?! | mscastillosmath
Posted in Math by Catherine Castillo
One of my favorite sites for promoting mathematical discourse and allowing students to see patterns and connections in mathematics is Fawn Nguyen’s ww.visualpatterns.org.
I first learned of this website from a Twitter chat that I regularly participate in and couldn’t wait to try it out with my students. I decided to try it out in our small groups and simply ask students the following questions:
- What do you notice?
- What do you wonder?
- What do you think will come next in the pattern? Can you draw it?
- Explain your thinking.
It didn’t take long for the room to be full of chatter around the task. The website quickly became a favorite and students would ask me throughout the day if we would be using it in small group.
In my opinion, the greatest challenge in teaching today is student motivation…and if you can find that magical tool that combines deep conversation, connections to mathematical concepts AND your students are excited about participating…you run with it for as far and long as you can!
One thing that I have noticed about students over the years is when they understand, when they TRULY understand a mathematical concept, they are eager to share their learning with others if given the chance to do so in a creative way.
The interesting part is that many times my students who are reluctant to share at first aren’t students who struggle with a math concept, they are students who understand the concept, but don’t want to be singled out by a teacher who always calls on them. They have come from classrooms that value answers and they have already lost their excitement for learning.
However, if we stop making mathematics about answers and start making it about problems that can have creative solutions, those students are just as excited to share as others because they get to show what they really care about…who they are and what they have to offer the group; Not a one word answer to someone else’s problem.
One of the many great things about Fawn’s website is that the tasks are low floor, high ceiling. Every student in the classroom can enter the task and begin talking about the mathematics they see. Look at this example. Each student in my classroom could count the number of objects, tell me what shapes they were, notice that there were more each time, etc.
and the students who needed a challenge could easily set to work figuring out what the 7th image would look like while the rest of the students continued their conversations. It really is beautiful to see. Everyone working on the same task and every conversation a little different.
During this particular task I heard:
“No the fourth shape would have four on the bottom row because each time they added a row to the bottom with one more”
“They just added one more to each row.”
“There is 1 triangle in the first one and 3 in the second one…”
And students couldn’t wait to get up and explain their thinking to their peers. They even established ways to label their thinking to make it more clear to each other.
The amazing thing about these tasks is that they are giving students the opportunity to see patterns and relationships between numbers in a visual way so that they can create connections between concepts.
Math classrooms should be creative spaces for students to explore, connect, argue and explain.
Places where students can be engaged in problems that are exciting and stimulating. When I find a resource that can support that goal, it’s a win!
Like this:
Like Loading...
Leave a commentFirst Grade Arithmetic - ThinkAlgebra
Starting in the 1st grade, students should learn the math facts and the Standard Algorithms first, not a hodgepodge of reform math strategies or alternatives.
First Grade Algebra (TKA)
x - 3 is an expression.
19 is an expression.
Equation Structure:
Expression = Expression
Some smart 1st and 2nd grade students found x, not by guess-and-check, but by adding 3 to 19. Left side must balance the right side: L = R.
Click: Keep up with the latest.
Zig Engelmann's preschool. Factoring the expression 6A + 9b + 3C.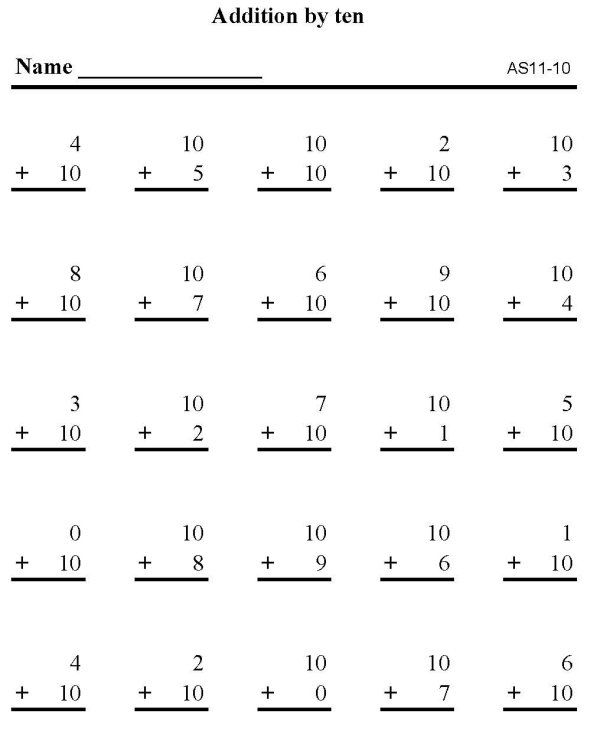
Zig Engelmann points out, "Without a firm foundation in number facts [automated in long-term memory], children are held back from further learning. Concepts are difficult to teach when students have mastered only some of the facts some of the time." Exactly!
In the 1960s, using old-fashioned repetitive drills, Engelmann's pre-1st-grade-students memorized number facts and multiples to do arithmetic, find areas of rectangles, solve equations, calculate fractions, and factor expressions.
"Education policymakers have a model of how things should be, but 'should be' is not reality. They believe that it is more important to preserve their flawed understanding of how kids learn than it is to provide effective instruction to kids." We should not limit students to so-called grade-level content. (Note. On a sad note, Zig Engelmann passed away on February 15, 2019. His wisdom will be missed.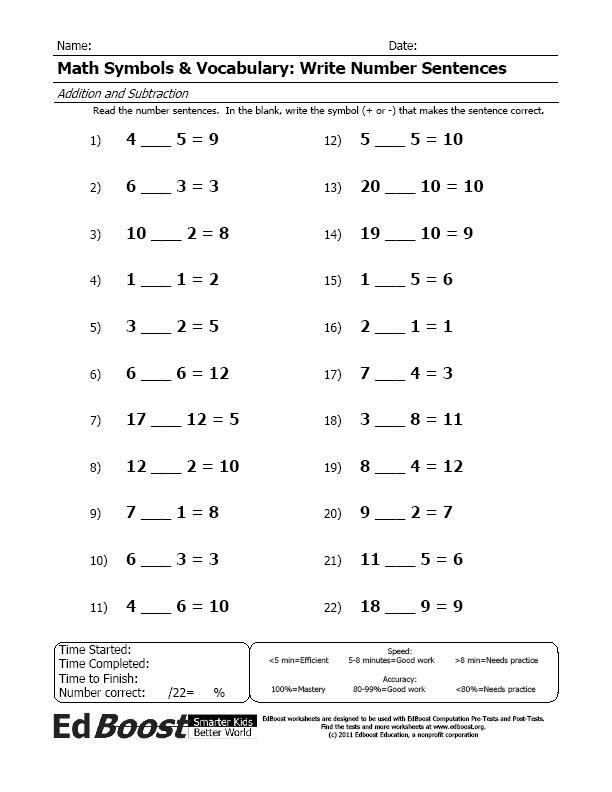
)
My 1st-Grade Algebra Program
We should focus on the mastery of fundamentals, not learning for a test. In the U.S., instruction is the opposite. It is geared toward learning for the state test via a reform math curriculum that had failed in the past. No wonder, our children can't do arithmetic or algebra well.
Starting in the 1st grade, students need to master basics, which involves memorization and practice-practice-practice.
Children need to do things that don't come easy for them.
They need to be persistent and complete tasks.
Click: Teach Kids Algebra
Practice does not cause talent; it improves performance.
3 + 5 = 10 - 2 is a true statement.
The logic behind the true statement is the transitive rule of equality: two things equal to the same thing {8} are equal to each other.
Left Side = Right Side or 8 = 8. Using guess and check, 1st-grade students can learn to solve equations such as 3 + 5 = x - 2. The letter x is an unknown number. If the left side is 8, then the right side must equal 8 to make a true statement. What number minus 2 is 8? {10 - 2= 8} The equal sign acts like the fulcrum of an equal-arm balance. Children need to think like a balance. Left Side = Right Side.
"You don't know anything until you have practiced."
Richard Feynman, Nobel Prize in Physics
Practice builds conceptual knowledge and math skills.
Thinking in math requires knowing math facts!
"You learn only through mastery!" Zig Engelmann
Being good at math is less about ability and more about hard work. Focus on the mastery of fundamentals, not state test proficiency scores.
"The only way to reach the more satisfying mastery stage [in math] is to first wade through that difficult and frustrating learning stage and then engage in substantial hours of practice." (Maya Thiagarajan)
The goal should be mastery of basics, not state test-based proficiency. Furthermore, the math skills are specific. Starting in 1st grade, some of the skills include memorizing the single-digit number facts, applying the place value system, using rules, and learning standard algorithms. These beginner skills are needed for doing arithmetic in 1st grade. So, why are our 1st-grade children not learning them?
The number line is key mathematics, but it is missing from most 1st-grade textbooks. Number lines start at ZERO, not 1.
A young student in my 1st-grade algebra class in the Spring of 2011. Some fundamental ideas of algebra are accessible to young children through arithmetic. 1st-grade students should memorize single-digit number facts and learn standard arithmetic and some algebra.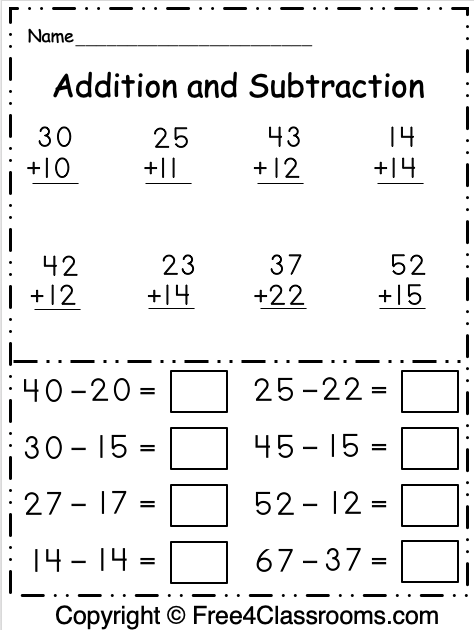
The Asian System
The way math is taught today in our schools (as reform math) rather than standard or traditional arithmetic is substandard compared to the Asian system. The Asian system is built on memorization, which forces students to store information in long-term memory where it is ready for use to solve problems, explains Arvin Vohra.The disparity starts in 1st-grade arithmetic. While Asian students excel at math and problem-solving, American students stumble over simple arithmetic, which is an unacceptable narrative! In contrast, Asian children are taught mechanics first with explanation later, and it works! We used to do that!
Children learn by repetition. The math skills and factual knowledge should come before teaching applications. Schools should teach the mastery of basic skills first, not a bunch of reform math fluff and its alternative algorithms.
Changes made on 6-22-19, 7-12-19, 12-14-2020
Most content is no longer available.
© 2004-2021 LT, ThinkAlgebra
Contact: [email protected]
Online math lessons • Skysmart.ru school 🏫
We will teach you how to count and solve problems with pleasure and develop mathematical thinking
We work with any program - Dorofeeva, Moro, Peterson and other authors
Anna Korchagina,
course methodologist
Elena Lisitsa,
methodologist of the course
“If a teenager has difficulty with mathematics, this does not mean at all that he has no abilities - we just need to find a clear explanation with a clear example from life.
”
Anastasia Oblakova,
course methodologist
“It's hard to love graphs and equations if you don't understand why they are needed. Therefore, we always explain in the lessons how each topic is useful in life.”
Anna Korchagina,
course methodologist
“It happens that a student is in the 7th grade, but his problems with mathematics remain in the 5th grade. Then we will re-analyze any topic so that the student understands everything connected with it.
Elena Lisitsa,
methodologist of the course
“If a teenager has difficulty with mathematics, this does not mean at all that he has no abilities - we just need to find a clear explanation with a clear example from life.”
One-on-one lessons on an interactive platform - where and when it suits you
The whole lesson in one window: video chat with the teacher, assignments and an online whiteboard
Raccoon Max and his friends will help you learn numbers, signs and shapes
Teachers will be interested in mathematics and at the same time keep their attention on lesson
In the school more than 90 teachers ready to help
Select the class format and package - the more lessons are in it, the lower the price of each
individuals
64 lesson
899 ₽ for lesson
32 lessons
999 ₽ for lesson
16 Lessons
1 099 ₽ for lesson
8 Lessons
1,299 ₽ for lesson
4 Lessons
1 499 ₽ for lesson
1 Lesson
Free
0003
Premium
64 Lesson
1 290 ₽ for lesson
32 Lesson
1 390 ₽ for lesson
16 Lessons
1 690 ₽ for lesson 9000 3 lessons
1 990 ₽ for lesson
4 lesson
2 290 ₽ per lesson
1 lesson
Free
Try it for free at the first lesson
We will agree on the first lesson by phone
0113
Sending a child to the first grade, parents always dream that their child will study well and receive only the highest marks in all subjects.
And if it is not at all difficult to teach a child to read, then it is not always easy for children to understand and solve mathematical problems. In order for a first-grader to succeed in mathematics at school, parents either hire a tutor, which is not always financially justified, or try to work with their children on their own. In this material, we will tell you how to pull up a first-grader in mathematics at home, talk about different types of problems and methods for solving them.
How to teach a child to count
Parents of first-graders should remember that at the age of 5–7 years, abstract thinking is still poorly developed in children. Remember the tale of Pinocchio, when he counted the apples that “Someone” allegedly took. Similarly, a child of 5–7 years old is not yet able to imagine the condition of the problem.
It is best to use visual aids that the child can see and touch. These can be counting sticks, cubes or pictures cut out of cardboard (for example, a set of cardboard hedgehogs, flowers, leaves, etc.
). Lay out in front of the child the entire condition of the task from visual materials: there was so much, so much was added or taken away. So it will be easier for him to understand the condition of the problem and easier to find its solution.
Another important point in teaching children is that the child must learn to distinguish tasks from each other by type. To do this, you can target it to some keywords. For example, if the task mentions the words "added", "brought", "arrived", "ran" and others denoting joining, then this is an addition task.
Understanding what type a particular problem belongs to, the child will learn to determine the required solution algorithm and successfully cope with the task.
Addition problems for first graders
As already mentioned, addition problems have a common feature - attachment. Another sign of addition tasks is the phrase “how much” in the task question.
The child must learn to clearly understand that if there is something added to the condition, then he needs to add up the available numbers. The child must understand what the first term, the second term and the sum are, and be able to find them in the task condition.
In order for a child to master mental counting well, he needs to regularly practice mental counting. If you are on vacation, at least once a day, ask him examples to develop memory. You can even study on the way to school or in the section.
Five to ten examples daily will not tire a first-grader too much, but will bring many benefits for his further studies.
Below are addition problems for first graders. For convenience, we have divided them into options, so that when studying at home, you can solve already completed tasks with your child.
Option 1
- Natasha read 5 books during the holidays, and Katya read 4 books. How many books did the children read together during the holidays?
- There were 6 apples on one branch of the apple tree, and 7 on the other. How many apples were there on both branches of the apple tree?
- There are potted flowers on the window in the classroom.
The first window has 2 flowers, the second has 3 flowers, and the third has 1 flower. How many flowers are in the class?
- Alyosha's family has 2 boys and 1 girl. Tanya's family has 1 girl and 1 boy. Serezha has 2 boys in the family, and Katya has only 1 girl. How many girls live in the families of all children? And how many boys?
- According to the results of assessments for the 1st quarter in the 1-A class, 10 excellent students, 14 good students and 2 three students. In the 1-B grade - 8 excellent students, 12 good students and 3 three students. And in 1-B - 11 excellent students, 11 good students and 4 three students. How many excellent students, good students and three students in the entire parallel of the first classes?
Option 2
- Natasha is 8 years old, how old will she be in 3 years? In 4 years, in 10 years?
- In the stationery store, Nastya liked the felt-tip pens for 18 rubles. She has 10 rubles, 5 rubles, 2 rubles and 1 ruble.
Will the girl have enough money to buy?
- 6 girls and 12 boys went for a walk. How many children went out for a walk?
- Sasha has a pack of counting sticks. Of these, 10 are red, 8 are blue and 12 are yellow. How many sticks are in the pack?
- 4 girlfriends and 5 friends came to Polina's birthday party. How many children will sit at the festive table? (here it is important that the child does not forget to count Polina herself, the answer in the problem is 10 children).
Variant 3
- Children came to the park and saw birds swimming on the lake: 8 swans and 12 ducks. How many birds were swimming in the lake?
- Children were planting saplings at school. Petya planted 2 seedlings, Anton planted 3, Natasha and Katya planted 2 seedlings. How many seedlings did the children plant in total?
- There were sweets in a box on the table. Masha ate 5 candies, Alena - 3 candies, Nastya - 6 candies, and Kolya ate 6 candies and the box was empty.
How many sweets were in the box from the very beginning?
- There are 20 postcards in Marina's collection. Yulia also has 20 postcards. How many postcards do girls have?
- Seva had 20 stamps, he was presented with 4 more stamps. How many stamps did Seva have in total?
Option 4
- Mom planted 20 cucumber bushes and 17 tomato bushes. How many plant bushes did mom plant in total? On Monday, 8 tables were brought to the canteen, on Tuesday - 7 tables, and on Thursday - 10 more. How many tables did the canteen receive in a week?
- Pasha and dad went camping. On the first day they walked 12 km. In the second - 10 km, in the third - 8, and in the fourth - 11. What path did dad and Pasha cover?
- The zoo has 12 monkeys, 8 tigers, 2 elephants, 6 bears and 4 raccoons. How many animals are in the zoo?
Important! If every day you solve one version of addition tasks with a child, then on the control tests he will show brilliant results.
- There are 13 boys and 12 girls in Grade 1-A. There are 12 boys and 15 girls in grade 1B and 10 boys and 12 girls in grade 1B. How many boys and how many girls are there in all first grades?
Subtraction tasks for first graders
Subtraction tasks also have their own characteristics and features. In the condition, you can always find some of the characteristic phrases: “how much is left”, “there were so many, of them ...”, “there were so many, so many left / flew away / ran away / deteriorated, etc.” and others. Here it is also important to understand what the first term, the second term and the sum are, to be able to find them in the task condition, because subtraction problems are the inverse of addition. And the concepts here are slightly different: reduced, subtracted, difference.
Below are subtraction problems for first graders. For convenience, we also divided them into options so that when doing homework, children can solve already completed tasks.
Here there are tasks both for finding the remainder (“how much is left”) and for decreasing (“by how much the number has changed”).
Option 1
- Andrei lives on the 7th floor, and Alena 3 floors below. What floor does Alena live on?
- Volodya has 17 cars, but Sasha has none. Volodya gave Sasha 8 cars. How much does he have left?
- Natasha is 12 years old, and her brother Seryozha is 7 years younger. How old is Seryozha?
- There were 10 apple trees in the garden, and 4 less pear trees. How many pears grew in the garden?
- On the first day Mila read 24 pages in the book, and on the second day she read 3 less. How many pages did Mila read on the second day?
Option 2
- Children receive books in the school library. Petya took 8 books, Alyosha took 2 books less than Petya, and Vanya took 3 books more than Alyosha. How many books did each boy take? How many books did they take together?
- There were 25 berries in a vase on the table.
Marina ate 4 berries, Alice ate 6 berries, Mila ate 3 berries, and Katya finished the rest of the berries. How many berries did Marina and Alice eat? Mila and Katya How many berries did Katya eat?
- There were 10 plates on the table and 6 less in the sink. How many dishes were in the sink?
- Serezha is 15 years old, his sister Larisa is 4 years younger. And the youngest brother Boris is 7 years younger than Larisa. How old are Larisa and Boris?
- Mom planted 30 cucumber bushes, 17 bushes sprouted. How many cucumber bushes were lost in total?
Option 3
- The children went to the forest for mushrooms. Dima found 10 russula and 7 white mushrooms. Tanya found 3 less russula and 2 less white ones. How many and what kind of mushrooms did Tanya find?
- The first house has 12 entrances, the second house has 4 entrances less than the first one. And in the third house there are 6 entrances less than in the first. How many entrances are in each of the houses?
- There are 23 apples in the first basket, and 11 less apples in the second.
How many apples are in both baskets?
- 12 girls took part in the performance, and 3 less boys. How many boys were in the play?
- There are 15 roses in one vase and 8 less in another. How many roses are in the second vase?
Option 4
- Sweets cost 30 rubles, and bread is 15 rubles cheaper. How much does bread cost?
- Grandmother baked pies. With potatoes 30 pieces, with jam 10 less than with potatoes, and with cabbage 5 less than with potatoes. How many and what kind of pies did grandma bake?
- There were 20 men on the bus. There were 5 fewer women than men, and 7 fewer children than women. How many people were on the bus in total?
- Children receive books in the school library. Sasha took 5 books, Misha took 2 books less than Sasha, and Serezha took 2 books more than Misha. How many books did each boy take? How many books did they take together?
- 20 buckets of water were used to water the garden.
12 buckets went to the beds with cabbage. How much went to the beds with carrots?
Comparison tasks for first graders
- Comparison tasks are aimed at finding a number that is less or more than the original. In principle, to some extent they can be attributed to addition or subtraction tasks, so we decided not to describe these tasks by options, but to give several similar examples:
- There were 10 cats sitting on the roof: 7 black and 3 grey. How many more black cats than gray ones?
- In the village, my grandmother has chickens and ducks. There are 18 chickens and 15 ducks. How many more chickens than ducks.
- Tanya has 3 dolls, and Dina has 4 more. How many dolls does Dina have? How many dolls does Tanya have less?
- Marina is 14 and Misha is 9. How many years is Marina older than Misha?
- There are 8 cars in the garage. Of these, 3 trucks and 5 cars. How many fewer trucks than cars?
- Dima was presented with gifts for his birthday.
First, mom and dad gave 2 gifts, then friends brought 5 gifts. How many more gifts did Dima have?
- On the first day of vacation Yura solved 5 problems, on the second - 7, and on the third - 2. How many more problems did Yura solve on the second day?
- How many tasks less than the first did Yura solve on the third day? And how many fewer problems did he solve on the third day than on the second?
- Sonya had 3 oranges and 10 apples. How many more apples than oranges?
- Olya has 3 hares and 2 squirrels. Mila has 5 dolls and 1 bear. Who has more toys and by how many?
- Cows were grazing in the meadow. 7 goats came to them and in total there were 15 animals in the meadow. How many cows were there?
Logic tasks for first graders
Articles with teachers' recommendations and lists of exercises and tasks have already been devoted to the development of logical thinking. Here we present several logical tasks that will allow not only to develop, but also to train the logic of first graders.
- Which is easier? A kilogram of cotton wool or a kilogram of nails? Tea, compote and cocoa were poured into a glass, mug and cup. There is no cocoa in the mug. There is no cocoa in the cup, and no compote. What was poured into what?
- How many fingers are on 3 hands?
- How many paws do 4 cats have?
- How many hands do 10 children have?
- Lena and Misha saw 2 ships in the sea. How many ships did each of the children see?
- Kitten tails sticking out from under the bed. How many kittens are there if 7 tails are visible?
- Dogs hid behind the fence. You can see 12 paws from under the fence, how many dogs are behind the fence?
- There are 5 peaches and 8 pears on the table. How many apples and plums will there be in total?
- There are 2 glasses of milk on the table. Petya drank the milk and put the camp on the table. How many glasses are on the table?
- Vanya left the school. 3 girls walked towards him.
How many children left school?
- Seven first graders went from home to school: Petya, Masha, Liza, Grisha, Tolya, Misha and Larisa, and 4 second graders: Seryozha, Tanya, Mila and Vanya. How many girls went to school?
- To get into the theater 2 daughters and 2 mothers needed 3 tickets. How could this happen?
- Misha is 2 years older than Lena. How much older will he be than Len in 5 years?
- Lena and Milana planted 10 flowers each and finished the work at the same time. Milana started work earlier. Which of the girls worked slower?
Instead of a conclusion
The mathematical development of first-graders is of great importance in their education. By solving examples and problems, the child acquires new experience, knowledge and skills. Learns to think logically and mathematically, find solutions from various situations, more successfully master related sciences in further studies.
Children's progress should not be left to chance, and every effort should be made to help them in this difficult task, like studying in the first grade.
