Concepts in math

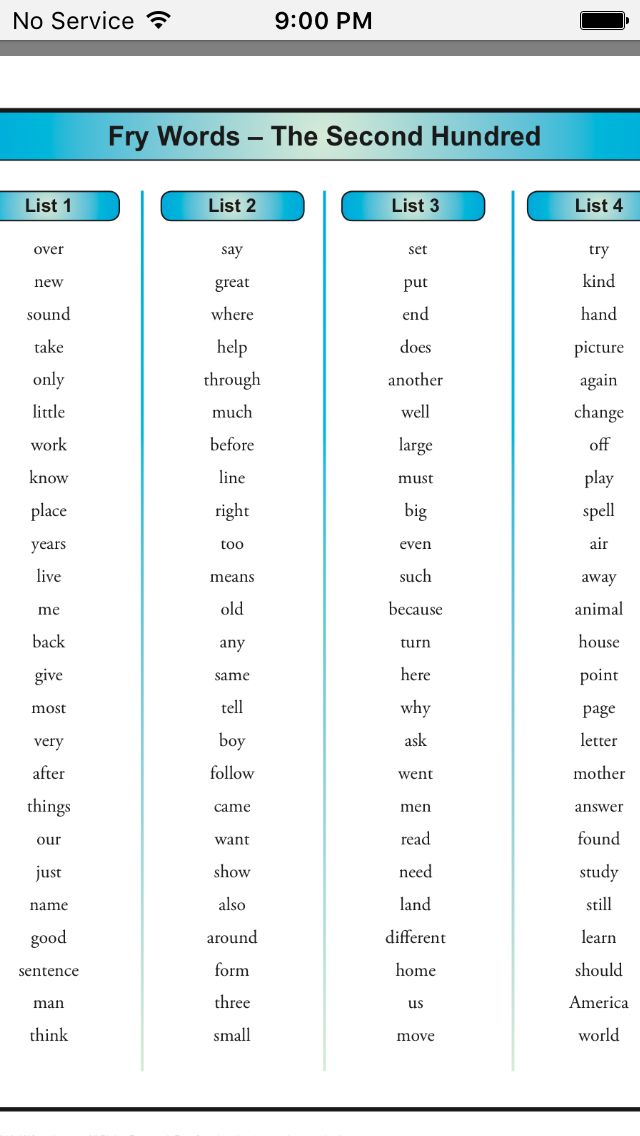
10 Math Concepts You Can't Ignore

Sets and set theory

A set is a collection of objects. The objects, called elements of the set, can be tangible (shoes, bobcats, people, jellybeans, and so forth) or intangible (fictional characters, ideas, numbers, and the like). Sets are such a simple and flexible way of organizing the world that you can define all of math in terms of them.

Mathematicians first define sets very carefully to avoid weird problems — for example, a set can include another set, but it can't include itself. After the whole concept of a set is well-defined, sets are used to define numbers and operations, such as addition and subtraction, which is the starting point for the math you already know and love.

Prime numbers go forever

A prime number is any counting number that has exactly two divisors (numbers that divide into it evenly) — 1 and the number itself. Prime numbers go on forever — that is, the list is infinite — but here are the first ten: 2 3 5 7 11 13 17 19 23 29 . . .

It may seem like nothing, but . . .

Zero may look like a big nothing, but it's actually one of the greatest inventions of all time. Like all inventions, it didn't exist until someone thought of it. (The Greeks and Romans, who knew so much about math and logic, knew nothing about zero.)

The concept of zero as a number arose independently in several different places. In South America, the number system that the Mayans used included a symbol for zero. And the Hindu-Arabic system used throughout most of the world today developed from an earlier Arabic system that used zero as a placeholder. In fact, zero isn't really nothing — it's simply a way to express nothing mathematically. And that's really something.

Have a big piece of pi

Pi (π): The symbol π (pronounced pie) is a Greek letter that stands for the ratio of the circumference of a circle to its diameter. Here's the approximate value of π:

π ≈ 3.1415926535…

Although π is just a number — or, in algebraic terms, a constant — it's important for several reasons:

Geometry just wouldn't be the same without it. Circles are one of the most basic shapes in geometry, and you need π to measure the area and the circumference of a circle.

Pi is an irrational number, which means that no fraction that equals it exactly exists. Beyond this, π is a transcendental number, which means that it's never the value of x in a polynomial equation (the most basic type of algebraic equation).

Pi is everywhere in math. It shows up constantly (no pun intended) where you least expect it. One example is trigonometry, the study of triangles. Triangles obviously aren't circles, but trig uses circles to measure the size of angles, and you can't swing a compass without hitting π.

Equality in mathematics

The humble equals sign (=) is so common in math that it goes virtually unnoticed. But it represents the concept of equality — when one thing is mathematically the same as another — which is one of the most important math concepts ever created. A mathematical statement with an equals sign is an equation. The equals sign links two mathematical expressions that have the same value and provides a powerful way to connect expressions.

Bringing algebra and geometry together

Before the xy-graph (also called the Cartesian coordinate system) was invented, algebra and geometry were studied for centuries as two separate and unrelated areas of math. Algebra was exclusively the study of equations, and geometry was solely the study of figures on the plane or in space.

The graph, invented by French philosopher and mathematician René Descartes, brought algebra and geometry together, enabling you to draw solutions to equations that include the variables x and y as points, lines, circles, and other geometric shapes on a graph.

The function: a mathematical machine

A function is a mathematical machine that takes in one number (called the input) and gives back exactly one other number (called the output). It's kind of like a blender because what you get out of it depends on what you put into it. Suppose you invent a function called PlusOne that adds 1 to any number. So when you input the number 2, the number that gets outputted is 3:

PlusOne(2) = 3

Similarly, when you input the number 100, the number that gets outputted is 101:

PlusOne(100) = 101

It goes on, and on, and on . . .

The very word infinity commands great power. So does the symbol for infinity (∞). Infinity is the very quality of endlessness. And yet mathematicians have tamed infinity to a great extent. In his invention of calculus, Sir Isaac Newton introduced the concept of a limit, which allows you to calculate what happens to numbers as they get very large and approach infinity.

Putting it all on the line

This pattern continues forever, with each value being halved, which means you can never get to the other side of the room. Obviously, in the real world, you can and do walk across rooms all the time. But from the standpoint of math, Zeno's Paradox and other similar paradoxes remained unanswered for about 2,000 years.

The basic problem was this one: All the fractions listed in the preceding sequence are between 0 and 1 on the number line. And there are an infinite number of them. But how can you have an infinite number of numbers in a finite space? Mathematicians of the 19th century — Augustin Cauchy, Richard Dedekind, Karl Weierstrass, and Georg Cantor foremost among them — solved this paradox. The result was real analysis, the advanced mathematics of the real number line.

Numbers for your imagination

The imaginary numbers (numbers that include the value i = √ - 1) are a set of numbers not found on the real number line. If that idea sounds unbelievable — where else would they be? — don't worry: For thousands of years, mathematicians didn't believe in them, either. But real-world applications in electronics, particle physics, and many other areas of science have turned skeptics into believers.

So, if your summer plans include wiring your secret underground lab or building a flux capacitor for your time machine — or maybe just studying to get a degree in electrical engineering — you'll find that imaginary numbers are too useful to be ignored.

Math Concepts | HowStuffWorks

How to Subtract Fractions

The very idea of trying to subtract one fraction from another may send you into convulsions of fear, but don't worry — we'll show you how.

By Jesslyn Shields

Advertisement

What Is the Formula for Distance?

You can find the distance between two points by using the distance formula. It's an application of the Pythagorean theorem. Remember that from high school algebra?

By Mark Mancini

You Know the Kilo. Now Say Hello to the Quetta!

When a peta and a tera won't do, you can now call on the quetta or the ronna prefixes. But just how big are these new metric systems of measurement?

By Alia Hoyt

What Is the Birthday Paradox?

How large does a random group of people have to be for a 50 percent chance to exist that at least two of the people will share a birthday?

By Laurie L. Dove

Advertisement

What's an Easy Way to Convert Meters to Feet?

Most of the world uses meters, apart from the U.S. and a few other countries. So what's an easy way to convert from meters to feet and vice versa?

By Mark Mancini

How to Find Range

Finding the range of a set of numbers is an easy subtraction problem!

By Jesslyn Shields

Advertisement

How to Convert Kilograms to Pounds

Converting kilogram measurements into pounds is not hard. We'll show you the textbook way plus two quick-and-dirty shortcuts.

By Mark Mancini

What Is the Formula for Velocity?

Many people get speed and velocity confused. It's no surprise because the terms are often used interchangeably. But they're not quite the same thing. So how do you find the velocity of an object?

By Mark Mancini

Math World Egg-static About New Egg-shape Equation

Scientists have come up with a new formula to describe the shape of every egg in the world, which will have applications in fields from art and technology to architecture and agriculture.

By Jesslyn Shields

Advertisement

What Are Real Numbers?

Real numbers are the opposite of imaginary numbers and include every number you can think of.

By Jesslyn Shields

What Is a Perfect Square?

A perfect square is a number, but it can also be explained using an actual square.

By Jesslyn Shields

How to Divide Fractions

Dividing fractions is easy once you learn a couple of rules and remember three words — keep, change and flip.

By Jesslyn Shields

How to Multiply Fractions

Multiplying fractions is easy — it just takes three simple steps!

By Jesslyn Shields

Advertisement

What Are Imaginary Numbers?

An imaginary number is a value that's the square root of a negative number. It can't exist on a one-dimensional number line. We'll explain.

By Patrick J. Kiger

How to Use the Unit Circle in Trig

A unit circle is an important part of trigonometry and can define right angle relationships known as sine, cosine and tangent.

By Nokware Knight

Advertisement

Bayes' Theorem Helps Us Nail Down Probabilities

Bayes' theorem describes the probability of an event, based on prior knowledge of conditions that might be related to the event. Sounds intimidating, but we'll walk you through it.

By Mark Mancini

1. Mathematical concepts

Lecture #2

in mathematics

Topic: "Mathematical concepts”

Plan:

1. Mathematical concepts

2. Scope and content concepts. Relations between concepts

3. Definition concepts

4. Requirements to definition of concepts

5. Some species definitions

concepts, which are studied in the initial course mathematics is usually represented in the form four groups. The first one includes concepts related to numbers and operations above them: number, addition, term, more, etc. The second includes algebraic concepts: expression, equality, equation and others. The third is geometric concepts: line, segment, triangle etc. The fourth group is formed by the concepts associated with quantities and their measurement.

How to study such an abundance of different concepts?

Before All you need to do is to understand the concept as a logical category and features mathematical concepts.

IN logic concepts are considered as a form thoughts reflecting objects (objects or phenomena) in their essential and general properties. The language form of the concept is a word or group of words.

Compose the concept of an object means to be able to to distinguish it from others similar to it objects. Mathematical concepts have a number of features. home is that the mathematical objects to be compiled concept does not exist in reality. Mathematical objects are created by the mind person. These are ideal objects reflecting real objects or phenomena. For example, in geometry one studies the shape and size of objects, without taking into account their other properties: color, mass, hardness, etc. From all this distracted, abstracted. That's why in geometry instead of the word "object" say "geometric figure".

result abstractions are and such mathematical concepts like "number" and "value".

At all mathematical objects exist only in the thinking of man and in those signs and symbols that form a mathematical language.

TO It can be added to what has been said that, by studying spatial forms and quantitative relations of the material world, mathematics not only uses different methods abstraction, but abstraction itself acts as a multi-step process. Mathematics considers not only concepts that emerged in the study real objects, but also concepts arising from the first. For example, the general concept of a function as a correspondence is a generalization of the concepts of specific functions, i.e. abstraction from abstractions.

To master general approaches to learning concepts in the elementary course of mathematics, The teacher needs knowledge about the volume and the content of the concept, about the relationship between concepts and about the types of definitions of concepts.

Any mathematical object has certain properties. For example, a square has four sides right angles equal to diagonals. Can specify other properties.

Among the properties object distinguish between essential and insignificant. Property feel essential to an object if it inherent in this object and without it, it is not may exist. For example, for square essential are all the properties mentioned above. Not essential for square ABCD property "side AD horizontal." If the square is turned then side AD will be located differently (Fig. 26).

That's why, to understand what is given mathematical object know its essential properties.

When talking about a mathematical concept, then usually means a set of objects, denoted by one term (word or group of words). So, speaking of a square, refers to all geometric shapes, which are squares. Think that the set of all squares is the scope of the concept of "square".

At all volume concepts are the set of all objects, denoted by one term.

Any concept has not only volume, but also content.

Contents concepts are the set of all essential object properties reflected in this concept .

Consider, For example, the concept of "rectangle".

Volume concepts are many different rectangles, and in its content includes such properties of rectangles, how "to have four right angles", "to have equal opposite sides", "to have equal diagonals”, etc.

Between scope of the concept and its content there is a relationship: if increases the scope of the concept, then it decreases content and vice versa. For example, the scope of the concept of "square" is part of volume of the concept of "rectangle", and in the content of the concept of "square" contains more properties than in the content of the concept "rectangle" ("all sides are equal", diagonals are mutually perpendicular and etc.).

Any the concept cannot be grasped without realizing it relationships with other concepts. That's why it is important to know in what respects to be concepts, and to be able to establish these connections.

Relations between concepts are closely related to relationships between their volumes, i. e. sets.

Let's agree concepts are capitalized Latin alphabet: a, b, c,...,z.

Let two concepts a and b are given. Their volumes will be denoted respectively by A and V.

If A B (A ≠ B), then they say that the concept of a - specific in relation to the concept b , a concept b - generic in relation to the concept a .

For example, if a is a "rectangle", b - "quadrilateral", then their volumes A and B are in relation to inclusion (A B and A ≠ B), since every rectangle is a quadrilateral. That's why it can be argued that the concept "rectangle" - specific in relation to to the concept of "quadrilateral", and the concept "quadrangle" - generic in relation to the concept of a rectangle.

If A = B, then they say that concepts a and b are identical.

For example, identical concept of "equilateral triangle" and "equiangular triangle" because their volumes match.

If sets A and B are not related inclusions, then we say that the concepts a and b are not related to genus and species and are not identical. For example, not related such relations of the concept of "triangle" and "rectangle".

Consider in more detail the relation of genus and species between concepts. First, the concepts of gender and species are relative: the same concept may be generic to one concept and species in relation to another. For example, the concept of "rectangle" - generic in relation to the concept of "square" and specific in relation to the concept "quadrilateral".

Secondly, for a given concept, one can often specify several generic terms. Yes, for the concepts of "rectangle" are generic are the concepts of "quadrilateral", parallelogram, polygon. Among them, you can specify the nearest. For the concept of "rectangle" to the nearest is the concept of "parallelogram".

Third, the species concept has all the properties generic concept. For example, a square being a species concept in relation to to the concept of "rectangle", has all the properties of a rectangle.

Since the volume concepts - set, convenient, setting relations between the volumes of concepts, depict them using Euler circles.

Set, for example, relationship between the following couples concepts a and b, if:

1) a - "rectangle", b - "rhombus";

2) a - "polygon", b - "parallelogram";

3) a - "straight", b - "line segment".

IN case 1) the volumes of concepts intersect, but not one set is a subset of another (Fig. 27).

Hence, It can be argued that these concepts and b are not related to genus and species.

IN case 2) the data volumes of the concept are regarding inclusion, but do not match - every parallelogram is polygon, but not vice versa (Fig. 28). Therefore, it can be argued that the concept of "parallelogram" - specific in relation to the concept of "polygon", and the concept of "polygon" is generic in relation to the concept of "parallelogram".

IN case 3) the volumes of concepts do not intersect, since not a single segment is possible say he is direct, and neither one line cannot be named segment (Fig. 29).

Hence, these terms are not related genus and species.

ABOUT concepts of "line" and "segment" can be say they are for whole and part: A segment is a part of a line, not a type of it. And if the species concept has all the properties generic concept, then the part is not necessarily has all the properties of the whole. For example, a segment does not have such property of the straight line, as its infinity.

Basic Concepts of Mathematics — Mathematical Structures Minor — National Research University Higher School of Economics

Course page

Reading: 1-2 module of the 2nd course
Prerequisites: none
Labor intensity: 5 credits

64 class hours:

• 32 hours of lectures;
• 32 hours of seminars.

Control forms:

• exam;
• 1 test;
• 10 homework assignments.

Teachers

Kirichenko Valentina Alekseevna

Department of Mathematics: Associate Professor

About the course

Purpose of the course:

Master the key mathematical concepts used in modern mathematics and its many applications. Starting from the concepts of school mathematics familiar to everyone, such as number, line, function, we will get their far-reaching generalizations, such as rings, fields, vector spaces, transformation groups, operators. Students will also learn about the aims, problems, and methods of mathematics, from set theory to the beginnings of algebra, geometry, and analysis.

The following topics will be discussed in the course:

1. What is a number? Natural numbers: Peano's axioms and the method of mathematical induction. Real numbers: Dedekind sections, Cauchy sequences. Complex numbers, quaternions, octaves, p-adic numbers. Generalizations: rings, fields, algebras.
2. What is planimetry? Hilbert's formal method: axiom systems of Euclidean geometry from Euclid to Hilbert and Kolmogorov. Groups of motions of the plane and space. Generalizations: linear space axioms, linear operators, bases, dimension, classical groups.
3. What is a set? Sets, functions and mappings. Combinatorics: Dirichlet principle and Newton's binomial. Binary relations, equivalence and order relations. Countable sets, uncountable sets. Cantor's Diagonal Method and the Paradoxes of Naive Set Theory.

Homework and seminar tasks:

• DZ1 Seminar1
• DZ2 Workshop2
• DZ3 Workshop3
• DZ4 Seminar4
• DZ5
• DZ6 Seminar5
• DZ7 Seminar6
• DZ8 Seminar7
• DZ9 Workshop8
• DZ10 Seminar9

Lecture notes (2nd module):

• Lectures_until_30_11

Literature:

1. V.I. Arnold, Huygens and Barrow, Newton and Hooke, the first steps in mathematical analysis and catastrophe theory, from involute to quasicrystals. M., Nauka, 1989
2. D. Hilbert, S. Cohn-Vossen, Visual geometry. M.-L., ONTI, 1936
3. G. Gindikin. Stories about physicists and mathematicians. M., MTSNMO, 2006
4. A.A. Kirillov.

   ---

   Learn more

   • 
     Winter games for kids
   • 
     E for elmo
   • 
     Kids spelling websites
   • 
     Learning rhyming words
   • 
     3 syllable compound words
   • 
     Easy reading kindergarten
   • 
     How to level reading
   • 
     Homer be more funny
   • 
     Uppercase and lowercase letter matching
   • 
     Fundamental math skills
   • 
     Getting ready for bedtime