Different math topics

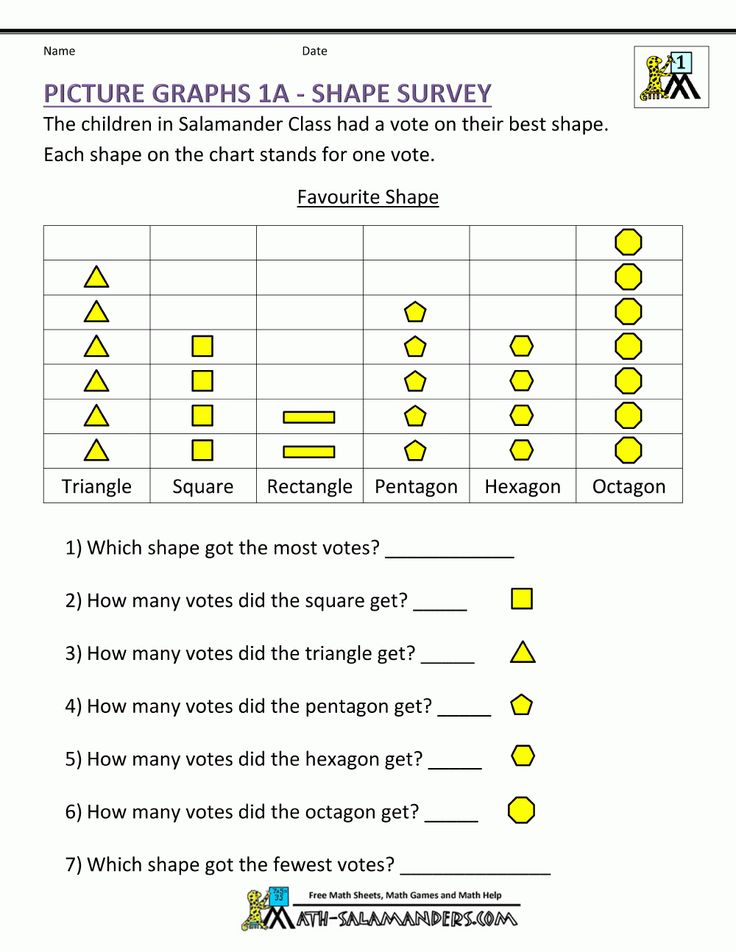
Main Branches of Mathematics Tree | PDF | Pure & Applied

Shakuntala Devi rightly said that without mathematics, there is nothing you can do. To explore more, let’s first know the 5 main branches of mathematics, i.e. Algebra, Number Theory, Arithmetic and Geometry. In the past 2 decades or so, our modern world has introduced more branches like Probability and Statistics, Topology, Matrix Algebra, Game Theory, Operations Research derived from these oldest branches of math. Breaking the myth of mathematics being called boring, we bring to you all about branches of mathematics with their list, tree and career options!

Check Out: Vedic Maths

This Blog Includes:

1. Tree of the Branches of Mathematics
2. MainBranches of Mathematics
3. Arithmetic
4. Algebra
5. Geometry
6. Trigonometry
7. Calculus
8. Probability and Statistics
9. Number Theory 
10. Topology 
11. Advanced Branches of Mathematics
12. Branches of Pure Mathematics
13. Branches of Applied Mathematics
14. Top Universities for Courses in Mathematics
15. Top Indian Colleges for Mathematics
16. Career Options in Mathematics
17. FAQs

Tree of the Branches of Mathematics

MainBranches of Mathematics

Mathematics is a complex area of study and comprises interlinked topics and overlapping concepts. Additionally, extensive analysis of the branches of mathematics helps students in organizing their concepts clearly and developing a strong foundation. Being aware of the differences and uniqueness of branches of mathematics helps in methodically studying various concepts of maths and also guides students in deciding the branch they would like to pursue as a career.

Here are the main branches of mathematics:

1. Foundations
2. Arithmetic
3. Algebra
4. Geometry
5. Trigonometry
6. Calculus
7. Probability and Statistics
8. Number Theory 
9. Topology

Below explained are the important branches of mathematics with their unique features and distinct concepts.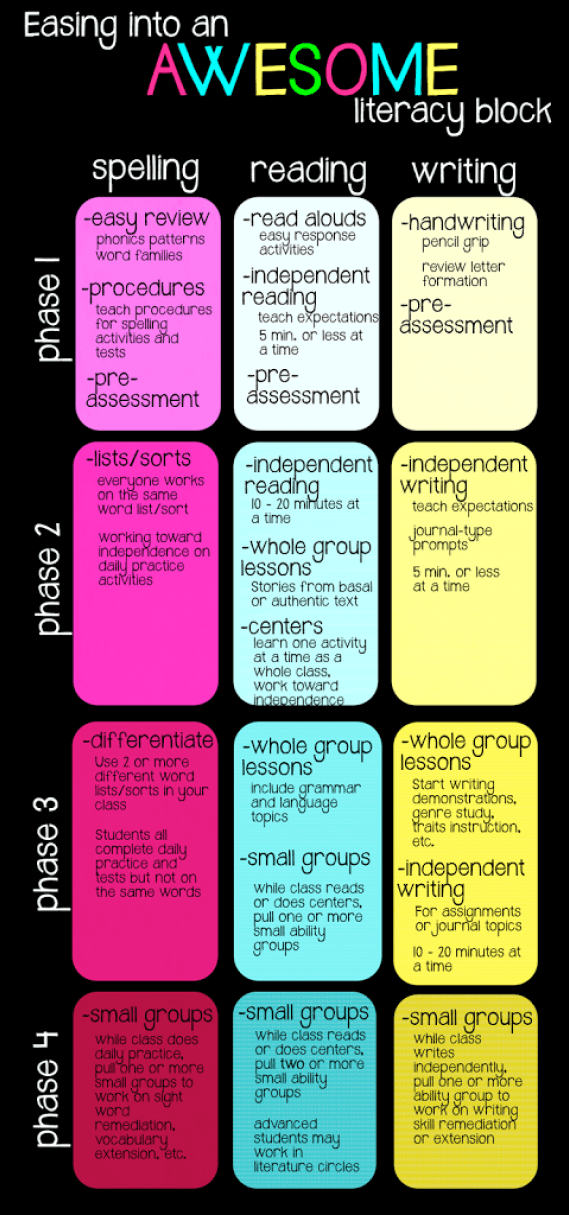 These branches are important for laying a strong foundation for mathematics. 

Fun Fact: -40 °C is equal to -40 °F.

Arithmetic

“Arithmetic must be discovered in just the same sense in which Columbus discovered the West Indies, and we no more create numbers than he created the Indians.”
– Bertrand Russell

Arithmetic is one of the most basic branches of mathematics and deals with numbers and their applications in many ways. Addition, subtraction, multiplication, and division are used as the basic groundwork to solve a large number of questions and progress into more complex concepts like exponents, limits, and many other types of calculations. This is one of the most important branches because its fundamentals are used in everyday life for a variety of reasons from simple calculations to profit and loss computation.

Word Problems on Arithmetic Operations

Arithmetic Reasoning

Algebra

“The algebraic sum of all the transformations occurring in a cyclical process can only be positive, or, as an extreme case, equal to nothing. ”
– Rudolf Clausius

A broad field of mathematics, algebra deals with solving generic algebraic expressions and manipulating them to arrive at results. Unknown quantities denoted by alphabets that form a part of an equation are solved for and the value of the variable is determined. A fascinating branch of mathematics involves complicated solutions and formulas to derive answers to the problems posed.

Algebra Questions

Algebra Formulas

Fun Fact: The word “mathematics” only appears in one Shakespearean play, “The Taming of the Shrew”.

Geometry

“The description of right lines and circles, upon which geometry is founded, belongs to mechanics. Geometry does not teach us to draw these lines, but requires them to be drawn.”
-Isaac Newton

Do you often wonder about the shapes and sizes of various objects? Then geometry is the branch you must explore. Dealing with the shape, sizes, and volumes of figures, geometry is a practical branch of mathematics that focuses on the study of polygons, shapes, and geometric objects in both two-dimensions and three-dimensions. Congruence of objects is studied at the same time focussing on their special properties and calculation of their area, volume, and perimeter. The importance of geometry lies in its actual usage while creating objects in practical life.

Coordinate Geometry Formulas

Trigonometry

Derived from Greek words “trigonon” meaning triangle and “metron” meaning “measure”, trigonometry focuses on studying angles and sides of triangles to measure the distance and length. Amongst the prominent branches of mathematics used in the world of technology and science to develop objects, trigonometry is a study of the correlation between the angles and sides of the triangle. It is all about different triangles and their properties!

Trigonometry Formulas

Calculus

“Calculus is the most powerful weapon of thought yet devised by the wit of man. ”
– Wallace B. Smith

It is one of the advanced branches of mathematics and studies the rate of change. With the advent of calculus, a revolutionary change was brought about in the study of maths. Earlier, maths could only work on static objects but with calculus, mathematical principles began to be applied to objects in motion. Used in a multitude of fields, the branch can be further categorized into the differential and integral calculus both starkly different from each other. A branch with mind-numbing questions, calculus is an interesting concept introduced to students at a later stage of their study in mathematics.

Fun Fact: “Forty” is the only number that is spelled with letters arranged in alphabetical order.

The abstract branch of mathematics, probability and statistics use mathematical concepts to predict events that are likely to happen and organize, analyze, and interpret a collection of data. Amongst the relatively newer branches of mathematics, it has become indispensable because of its use in both natural and social sciences. The scope of this branch involves studying the laws and principles governing numerical data and random events. Presenting an interesting study, statistics, and probability is a branch full of surprises.

Statistics Formulas for Competitive Exams

Number Theory 

“Mathematics is the queen of the sciences, and number theory is the queen of mathematics.”
–  Carl Friedrich Gauss

As the name suggests, Number theory is one of the oldest branches of Mathematics that established a relationship between numbers belonging to the set of real numbers. The basic level of Number Theory includes an introduction to properties of integers like addition, subtraction, multiplication, modulus and builds up to complex systems like cryptography, game theory and more. 

Topology 

“The basic ideas and simplest facts of set-theoretic topology are needed in the most diverse areas of mathematics; the concepts of topological and metric spaces, of compactness, the properties of continuous functions and the like are often indispensable. ”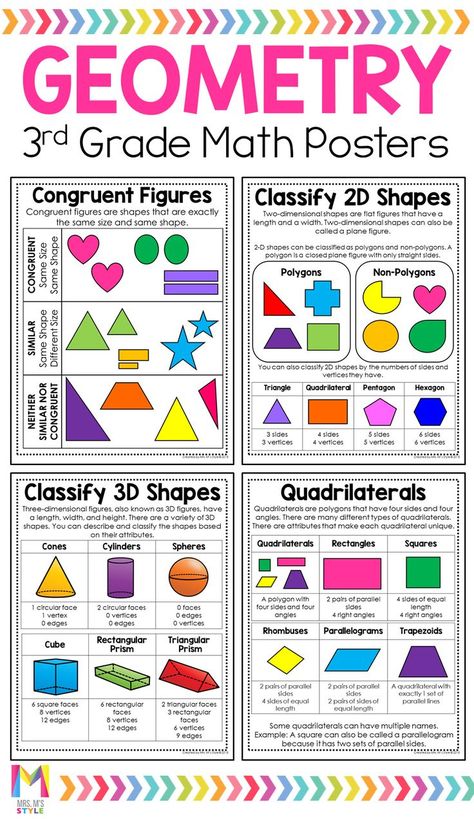
–  Pavel Sergeevich Aleksandrov

Topology is a much recent addition to the branches of Mathematics list. It is concerned with the deformations in different geometrical shapes under stretching, crumpling, twisting and bedding. Deformations like cutting and tearing are not included in topologies. Its application can be observed in differentiable equations, dynamical systems, knot theory, and Riemann surfaces in complex analysis.

Fun Fact: Every odd number has an “e” in it.

Advanced Branches of Mathematics

There are a vast number of advanced branches which are a part of the major branches mentioned above. These branches are studied at an advanced level and involve complex concepts that need strong computational skills. Such advanced branches are listed below.

Here are the 10 branches of mathematics:

1. Matrix Algebra
2. Numerical Analysis
3. Operations Research
4. Complex Numbers
5. Calculus
6. Set Theory
7. Game Theory
8. Analysis
9. Cartesian Geometry
10. Combinatorics

Applied Mathematics and Computation

Branches of Pure Mathematics

Pure mathematics can be simply described as the study of mathematical concepts purely based on maths and independent of any concept outside of mathematics. Here is the list of branches of Pure Mathematics:

• Algebra
• Number Theory
• Geometry
• Arithmetic
• Topology
• Combinatorics
• Mathematical Analysis

Branches of Applied Mathematics

Applied Mathematics uses the applications of varied other disciplines and combines it with mathematical concepts. It is simply the combined application of mathematics with specialised knowledge.

Here are the branches of applied mathematics:

• Statistics and Probability
• Set Theory
• Calculus
• Trigonometry

Fun Fact: Archimedes [287 – 212 BC] is the father of Mathematics

Top Universities for Courses in Mathematics

For aspirants wishing to establish a career in mathematics, the first step is to pursue a course in your chosen branch of Mathematics. Many renowned universities of the world offer lauded degrees in mathematics and its varied branches. Being aware of the major picks available to one helps in reducing the dilemma that comes with choosing the university. Tabulated below are some of the top class universities offering esteemed mathematics programs. 

Top Indian Colleges for Mathematics

1. Fergusson College
2. Mount Carmel College
3. Stella Maris College
4. St. Xavier’s College
5. Jadavpur University
6. St.Stephen’s College
7. Ramakrishna Mission Vivekananda College
8. Christ University

Career Options in Mathematics

If the number game attracts you and you can’t help but add or multiply any number you see, then you should pick up Mathematics as a career. You can become one of the following professionals if you choose a career in Mathematics:-

• Teacher/Lecturer– You can teach mathematics at the high school level and even university level if you are qualified enough and even explore research via postgraduation.
• Statistician- A statistician collects data, turns them into charts and graphs and makes interpretations which is an important aspect in all professions.  
• Data Analyst/Scientist– A data analyst/scientist is similar to a statistician but with certain differences. In Data Science, you will use scientific methods of data collection, comparison and give solutions to the organisation relatively.
• Mathematician– A mathematician is involved in all fields of profession. Whether it be inventing new business valuation methods or creating a formula that sends a rocket to the ISS, a mathematician does it all.
• Cryptographer– A cryptographer is a person who encodes and decodes various symbols or modes of communication to decipher what it means. Additionally, they also create safe codes for the military or other organisations. 

Every profession needs the Branches of Mathematics as the subject ties all the broken pieces and creates uniformity.

FAQs

What are the four branches of Mathematics?

Algebra, Geometry, Calculus and Statistics & Probability are considered to be the 4 main branches of Mathematics.

What is the hardest branch of Maths?

Algebra is the hardest branch of Maths. Abstract algebra particularly is the most difficult portion as it includes complex and infinite spaces.

Who is the father of Mathematics?

Archimedes [287 – 212 BC] is the father of Mathematics

Who invented Math?

It is difficult to trace back the exact origins of Maths. But it is estimated that in early 6th century, Pythagoreans invented Maths. After that Euclid introduced the axiomatic method consisting of the definition, axiom, theorem, and proof.

BSc Mathematics

MSc Mathematics

Are you intrigued by numbers and have a flair for doing difficult numeric calculations? Then, Mathematics is an all-encompassing science that deals with quantity, space and numbers and abstract concepts as well as applied maths in other fields. While at first glance it might be difficult to identify the branches of mathematics, it is imperative to possess knowledge about them for any student wishing to gain a holistic idea about the subject. We hope this blog has presented a comprehensive list of different areas of study and branches of mathematics. Planning to study a degree in Mathematics? Experts at Leverage Edu can help you find the best course and university as well as assist you throughout the admission process to ensure that you send a winning application! Sign up for a free session today!

Branches, Fundamentals, Important Topics, Preparation Tips

Math is the science of quantity, pattern, order, structure and relation that has continuously evolved from basic practices of counting, measurements and symmetric study of shapes. It primarily involves applying logical reasoning and quantitative computation to find optimal solutions to problems. It has been worldwide recognized as an indispensable computational tool in the field of engineering, biology, medicine and natural sciences.

Math As A Subject

Math as a subject is an important part of the curriculum that plays a significant role in shaping a child’s future. It involves studying many useful concepts and topics relevant to practical life. Although math is an interesting subject, students often find it boring and complex due to the way it is taught conventionally. Cuemath helps students to explore and understand fundamental concepts in a fun and intuitive manner.

Fundamentals of Math

Fundamentals of math are the basic building blocks that help students form a solid mathematical foundation. Math learning entirely relies on the understanding of these fundamental concepts. If children lack the basic understanding of division or subtraction, then algebra automatically becomes confusing for them. Therefore, it is imperative that children must have a crystal clear knowledge of all fundamentals of math.

• Addition and Subtraction of Whole Numbers
• Multiplication and Division of Whole Numbers
• Exponents, Roots, and Factorization of Whole Numbers
• Introduction to Fractions and Multiplication and Division of Fractions
• Addition and Subtraction of Fractions, Comparing Fractions, and Complex Fractions
• Decimals and Fractions
• Ratios and Rates
• Techniques of Estimation
• Measurement and Geometry
• Signed Numbers
• Algebraic Expressions and Equations

Branches of Mathematics

Mathematics involves complex studies of interlinked topics and several concepts that overlap each other.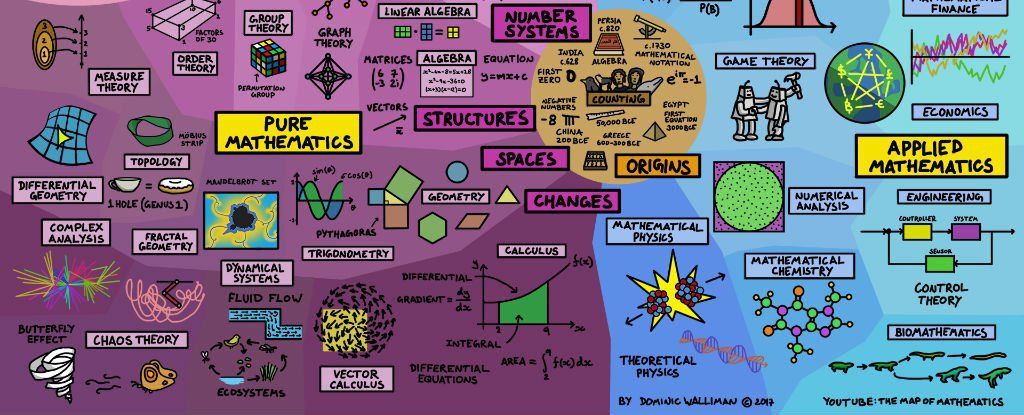 Generally, it can be categorized into the following branches:

Arithmetic

Arithmetic is the most basic branch of mathematics that deals with the elementary aspects of numbers, mensuration and numerical computations. This term is derived from the Greek word ‘arithmos’ which means number. It generally involves studying numbers and their relationships to solve problems that include the operations of addition, multiplication, subtraction, division, extraction of roots and raise to power.

Algebra

Algebra is an important and ancient branch of math that covers basic operations and symbols to represent numbers in formulas and equations. The word Algebra means the science of restoring and balancing. Learning algebra enables students to understand many real-life phenomena around them. It is a symbolic representation of numbers and how they work together to provide structure to equations. It forms the basis for advanced study in many fields like science, medicine, engineering, etc.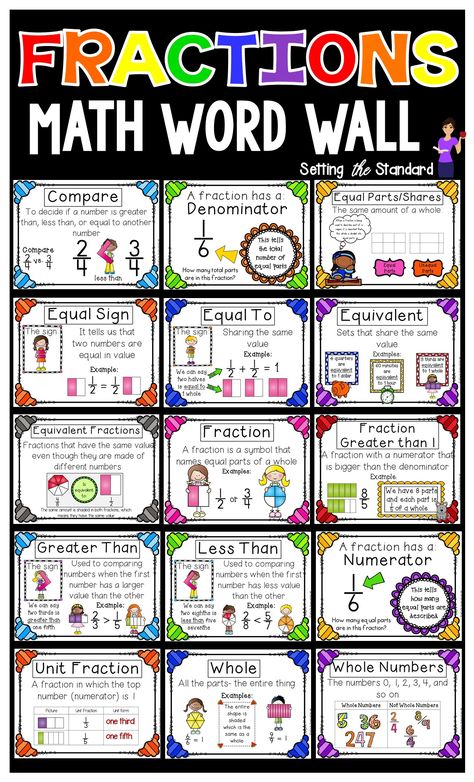 It allows mathematicians to write formulas and solve maths problems more efficiently.

Geometry

Geometry is the branch of math which deals with computation of various dimensions of solid shapes including height, width, area, volume, perimeter and angles. It has several useful applications from the construction of homes to interior designs.

Trigonometry

Trigonometry is an important branch of math that involves studying the relationship between angles, lengths, heights and distance. The applications of trigonometry can be found in many spheres including architecture, physics, surveying, electronics, satellite navigation, astronomy and engineering.

List of Branches of Maths

Important Math Topics

A thorough understanding of all important math topics will benefit students throughout their lifetime. Some of the essential math concepts that students must have an in-depth understanding of are based on the topics listed below.

• BODMAS Rule
• Prime and Composite numbers
• HCF and LCM
• Basic Menstruation
• Divisibility Rules
• Decimal and Fractions
• Ratio and Proportion
• Three-dimensional shapes
• Geometry
• Probability

Math Calculators

Students often find math challenging due to complex mathematical calculations. Math Calculators are handy tools to resolve all such problems. It makes calculations simple and quick. With the use of math calculators, calculations ranging from elementary arithmetic operations to complicated equations can be solved within a few seconds.

List of important calculators for students to solve problems quickly and get accurate solutions.

Important Math Formulas

Math formulas are expressions created after several years of research to help solve problems easily. Performing simple numerical operations like addition, subtraction and division are easy. However, to solve algebraic expressions, and other complex operations we use mathematical formulas. These are quite useful in obtaining the answers precisely. Cuemath provides formulas for each math topic along with the illustrated steps of equations for students to understand them logically.

List of important formulas that students must learn and memorize.

Tips And Tricks To Learn Math Fast

Although math is a vast subject there are some tips and tricks to learn math fast. These tips and tricks will help students improve along their math journey.

Clear All Basic: The first and foremost step in learning mathematics is to clearly understand all basics. It will not only allow you to learn math faster but will also help in establishing links between various math topics.

Set Objectives: After clearing all basics, set goals for what you need to focus on. Once you understand your objective, start working on it. Explore various resources that can help you improve and get well versed in those topics.

Practice Daily: Math requires daily practice, implementing a proper study routine will help in grasping concepts better.

Take Guidance: Heading in the right direction is necessary as it will ensure good results. Consider taking help from your teacher or a math tutor if you feel doubtful about topics and concepts.

FAQs on Math

What Exactly is Math?

Math is the science involving numbers, shapes and patterns which is present in almost everything around us. It helps us to derive analytical solutions to practical problems. It is applied in various fields such as engineering, finance, physical science, etc. It has a great impact in every domain of our life and we can find many mathematics applications around us.

How to Get Better at Math?

Getting better at math requires enforcing a study routine and analyzing mistakes. Students must try to understand and rectify their mistakes through daily practice. Doing so will also help them clear all their doubts. Students with better mathematical abilities achieve higher academic success. Thus, it is crucial to cultivate math interest in children at an early age. Cuemath’s visually-enriched math concepts enhance a child’s interest in mathematics and make it easier to learn the subject.

Why is Math Important in Our Daily Life?

Math is highly important in our daily life as there are several applications of mathematics in real-world situations. Statistics or probability theory are examples of applied maths.

What are the Fundamentals of Mathematics?

Fundamentals of mathematics are the building blocks for a solid math foundation. Students must possess a clear knowledge of all the fundamentals of mathematics to study advanced mathematical concepts. These fundamentals of mathematics are given below.

• Addition and Subtraction of Whole Numbers
• Multiplication and Division of Whole Numbers
• Exponents, Roots, and Factorization of Whole Numbers
• Introduction to Fractions and Multiplication and Division of Fractions
• Addition and Subtraction of Fractions, Comparing Fractions, and Complex Fractions
• Decimals and Fractions
• Ratios and Rates
• Techniques of Estimation
• Measurement and Geometry
• Signed Numbers
• Algebraic Expressions and Equations

How many Branches of Mathematics do we Have?

The branches of mathematics can be broadly categorized as:

• Arithmetic: Arithmetic involves studying numbers and their relationships to solve problems that include the operations of addition, multiplication, subtraction, division, extraction of roots and raise to power.
• Algebra: Algebra is the symbolic representation of numbers that provides structure to equations. It forms the basis for advanced study in many fields like science, medicine, engineering, etc.
• Geometry: Geometry is the calculation of various dimensions of solids including height, width, areas, volumes, perimeter and angles. It has many practical applications in architecture and other fields.
• Trigonometry: trigonometry deals with the study of the relationship between angles, lengths, and heights. The applications of trigonometry can be found in many spheres including architecture, physics, surveying, electronics, satellite navigation, astronomy and engineering.

What are the Most Important Math Topics?

Some of the most important math topics are prime numbers, composite numbers, BODMAS rule, geometry, probability, divisibility rules, HCF, LCM, three-dimensional shapes, basic menstruation, decimal, fractions, ratio and proportion. An in-depth understanding of all important math topics will enable students to score well in exams.

How Math is Used in Sports?

Math is used in sports to accumulate data, study conditions and generate performance statistics which are considered for planning and optimizing the training session. The data collected in these mathematical calculations is also helpful for taking strategic decisions based on the team’s performance.

How Maths is Related to Other Subjects?

Math is related to other subjects, especially chemistry, physics, computer science and engineering. In chemistry, mathematics is used to write and balance equations. In physics. It is applied to calculate mass, velocity and acceleration. In computer science, math is used to build algorithms and solve problems.

all classes, all formulas, all topics

Dear schoolchildren, students! On the site you will find topics on mathematics for grades 5-11 and lectures on higher mathematics. We not only study theoretical material, but also solve problems - we analyze them in detail. We also pay attention to the analysis of interesting USE problems in mathematics. Here you will find all the formulas in mathematics for grades 5-11, learn to reason and solve equations, inequalities, systems of equations and inequalities, analyze functions, take derivatives and integrals. We paid special attention to an important section of mathematics - "Geometry". Solving spatial problems, reasoning, diagrams and much more awaits you in this section.
Trigonometry is another important section in mathematics, it links together both geometry and algebra, helps to comprehend space. Trigonometric equations and inequalities are analyzed in detail in this section. All necessary formulas are given.

Grade 7. Algebra.

Linear function y=kx+b and its graph

086

If the function is given by the formula , where and are some numbers, it is called a linear function.

5th grade. Maths.

How to multiply by a column in grade 3 and grade 4

099

In this article, we will consider how to multiply different numbers in a column - two-digit, three-digit

USE in mathematics profile level

Find the smallest value of the function y=4/3x√x-3x+9 on the interval [0.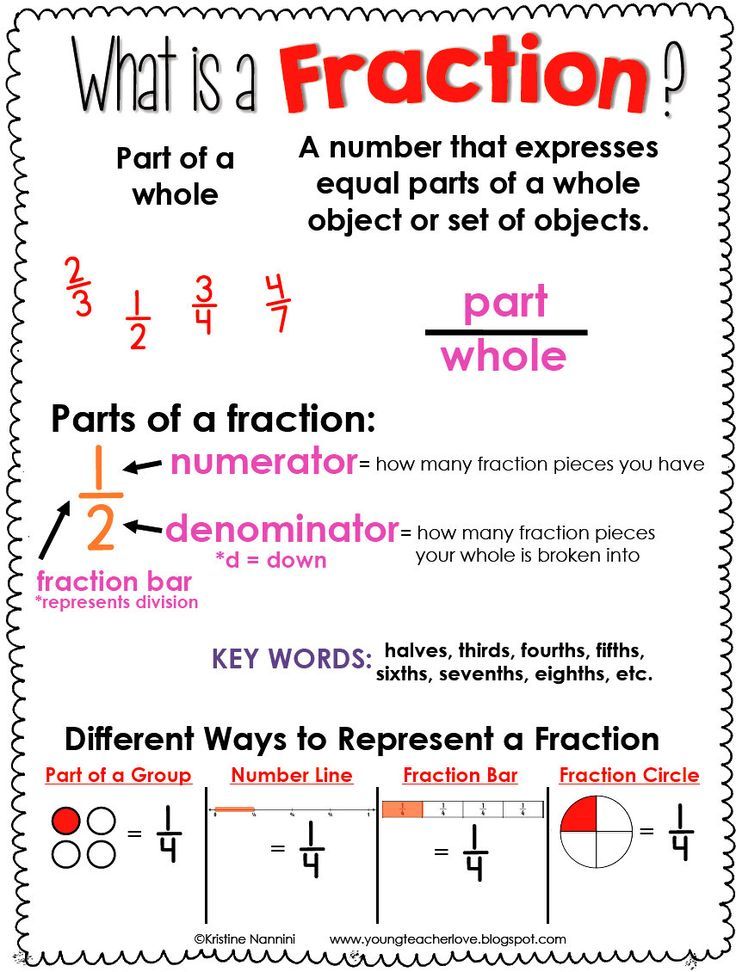 25; 30]

417.5k.

Find the smallest value of the function on the interval [0.25; thirty]. Solution: In order to find the smallest

USE in mathematics profile level

Two factories produce the same glass for car headlights

13.7k.

Two factories produce the same glass for car headlights. The first factory produces 30% of these glasses

5 class. Maths.

How to multiply by a column in the 3rd grade and in the 4th grade

099

Let's consider in this article how to multiply by a column various

5th grade. Maths.

Numeric expressions and letter expressions - rules

016.3k.

When solving examples and equations, it is necessary to clearly distinguish between

5th grade. Maths.

5.6.3. Solving problems with percentages. Part B

04.3k.

Problem 1. The first number is 80% of the second.

5th grade. Maths.

5.6.2. Solving problems with percentages. Part A

016.3k.

Problem 1. Water makes up 76% of potatoes. How many kilograms

Grade 5. Maths.

5.5.7. Rounding numbers

011.4k.

To round a number to any digit - underline

5th grade. Maths.

5.5.6. Decimal division

013.1k.

I. To divide a number by a decimal, you need

6th grade. Maths.

Numerical inequality, properties of numerical inequalities and examples of solutions

06.4k.

Consider what is the numerical inequality

Grade 6. Maths.

6.5.1. Linear equation with one variable

08.3k.

Very many schoolchildren have

6th grade. Maths.

6.4.2. Bracket opening. Reduction of similar terms

023.3k.

1. Opening brackets, before which

6th grade. Maths.

6.9.3. Solving systems of linear equations by the addition method.

023k.

To solve a system of linear equations

Grade 6. Maths.

6.9.2. Solving systems of linear equations by the substitution method

011.2k.

For solving a system of linear equations

6th grade. Maths.

6.4.1. Algebraic expression

18.3k.

I. Expressions in which along with

7th class. Algebra.

Linear function y=kx+b and its graph

086

If the function is given by the formula , where and

7 class. Algebra.

Identity - what is it in mathematics

08.7k.

Very often in mathematics there are such words «

Grade 7. Algebra.

7.2.3. Actions with monomials and polynomials

14.9k.

I. To multiply a monomial by a polynomial, you need to multiply

7th grade. Algebra.

7.2.2. Polynomial

03k.

I. The sum of monomials is called a polynomial.

Grade 7. Algebra.

7.2.1. Monomial.

02.4k.

I. Expressions that are made up of numbers, variables

Grade 7. Algebra.

7.1.2. Standard form of the number

210.2k.

Very large and very small numbers are usually written

Grade 8. Algebra.

8.2.1. Solving incomplete quadratic equations

07.3k.

I. ax2=0 - incomplete quadratic equation (b=0, c=0).

Grade 8. Algebra.

8.2.5. Decomposition of a square trinomial into linear factors

029.5k.

The square trinomial ax2+bx+c can be decomposed into linear ones

Grade 8. Algebra.

8.2.4. Application of Vieta's Theorem

07.9k.

Often you need to find the sum of squares (x12+x22) or

Grade 8 Geometry.

8.2.3.1. Rectangle. Problem solving 2

01.6k.

Task 1. Diagonal of a rectangle

Grade 8 Geometry.

8.2.3. Rectangle. Solving problems

08k.

Task 1. One side of a rectangle

Grade 8 Geometry.

8.2.5. Basic trigonometric identities. Part 2

01.8k.

Basic trigonometric identities.

Grade 8 Geometry.

8.2.4. Basic trigonometric identities. Part 1

08.2k.

Basic trigonometric identities.

Grade 9 Algebra.

9.3.3. Definition of an arithmetic progression. Examples

01.7k.

A numerical sequence, each member of which, starting from the second, is equal to the previous one, added with

9th grade. Algebra.

9.3.1. The numerical sequence is

017.4k.

The function an=f (n) of natural argument n (n=1; 2; 3; 4;...) is called a numeric sequence. Numbers a1;

Grade 9 Algebra.

9.3.2. Arithmetic progression. Theory

05.3k.

A numerical sequence, each member of which, starting from the second, is equal to the previous one, added with

10th class. Algebra.

Reduction formulas

113.8k.

The reduction formulas refer to the trigonometric function

Grade 10. Algebra.

10.3.0. Calculation of derivatives

012k.

In this lesson we will learn how to apply formulas

Grade 10. Algebra.

10.2.6. Solution of trigonometric inequalities. Part 6

02.2k.

In the previous lessons we solved trigonometric

Grade 11. Algebra.

11.1.9.3. Areas of curvilinear trapezoids enclosed between two curves

02k.

Problems of finding the area are often solved in algebra

Grade 11. Algebra.

11.1.9.2. The area of ​​a curvilinear trapezoid adjacent to the Oy axis

02.3k.

If a curved trapezoid adjacent to the OU axis (Fig.

grade 11. Algebra.

11.1.9.2. The area of ​​a curved trapezoid. Examples

030.4k.

Square of a curved trapezoid, limited from above

grade 11.

11.1.9.1. Definite integral. Area of ​​a curvilinear trapezoid

02.1k.

Time to get acquainted with the most powerful research tool

Geometry

Rectangle area

211k.

The area of ​​a rectangle is very often required to find

Geometry

How to calculate the area of ​​a circle - all formulas

113. 9k.

The area of ​​a circle is often required to be calculated in various

6th grade. Tests.

Test 6.6.1. Inequalities and their properties

02.4k.

Mathematics. 6th grade. Test 6.

Grade 6. Tests.

Test 6.5.1. Rounding decimals. Infinite periodic fraction

63.4k.

Mathematics. 6th grade. Test 5. Option 1.

Grade 6. Tests.

Test 6.4.1. All actions with rational numbers

02k.

Mathematics. 6th grade. Test 4.

Grade 6. Tests.

Test 6.3.1. Addition, multiplication of rational numbers

01.7k.

Mathematics. 6th grade. Test 3.

All main formulas in mathematics - Mathematics - Theory, tests, formulas and problems

Formulas of abbreviated multiplication

To the table of contents . ..

Square of the amount:

Square of the difference:

Squares:

Cuban difference:

Cubates: 9000

Cube of the sum:

Cube of the difference:

0004

Square equation and decomposition formula for a square three -member for multipliers

to table of contents ...

Let the square equation have the form:

Then Discriminant are found according to the formula:

If D > 0, then, then, then the quadratic equation has two roots, which are found by the formula :

0450 which is searched for by the formula :

If D < 0, then the quadratic equation has no roots. In the case when the quadratic equation has two roots, the corresponding square trinomial can be factored by the following formula :

Only if the quadratic equation has two roots (i. e. the discriminant is strictly greater than zero) is Vieta's theorem . According to , Vieta theorem , the sum of the roots of the square equation is:

The product of the roots of the square equation can be calculated by the formula:

Parabola

Parabox graphics are set the quadratic function:

, while the vertex of the top of the prarab may be a parabola. calculated by the following formulas. x vertex :

y vertex parabola:

The properties of degrees and roots

To the table of contents ...

The main properties of the degrees:

The last property is performed only at N > 0. Zero can only be raised to a positive power.

Basic properties of mathematical roots:

For arithmetic roots:

The latter is true: if n is odd, then for any a ; if n is even, then only if a is greater than or equal to zero. The following equation also holds for an odd root:

An even root has the following property:0368

The determination of the logarithm can be recorded in another way:

Logarithm properties:

Logarithm of the work:

Logarithm of fraction:

Other useful properties of logarithms:

0402

To the table of contents ...

Formulas N ARIPHMATIC Progress:

The ratio between three neighboring members of arithmetic progress:

Formula of the sum of arithmetic progress:

Athmetic progression

Geometric progression

To contents...0004

The ratio between three neighboring geometric progression members:

Formula of the Summer of Geometric Progress:

Formula of the amount of infinitely waning geometric progress:

9000 9000 9000 9000 9000 9000

To contents. ..

Let there be a right triangle:

Then, the definition of sine:

Cosinus Definition:

Tangens Definition:

Cotangens Definition:

The main trigonometric identity :

9,0007

Sine of double angle:

Cosine of double angle:

Tangent of double angle:

Double angle cattins:

Trigonometric addition formulas

Sinus summation:

Different Sila:

Difference tangent:

Sum cotangent:

Difference cotangent:

0368

Sinus amount:

Sinus difference:

The Summer Cosinus:

Cosinus difference:

Tangens amount:

Tangens difference:

Coats: 9000

Cotangent difference:

Trigonometric formulas for converting a product to a sum

Product of sines:

Product of sine and cosine:

Cosinus work:

Formulas of decrease degree

Formula of decrease the degree for the sinus:

Formula of lowering degree:

9000 cotangent:

Half angle formulas

Tangent half angle formula:

Cotangent half angle formula:

For other trigonometric functions, the notation is unique. For cosinus:

for tangens:

for cotoans:

Solution of trigonometric equations in some private cases:

9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 9000 on a plane (planimetry)

To contents...

Then, The amount of the angles of the triangle :

The area of ​​the triangle through two sides and the angle between them:

The area of ​​the triangle through the side and height lowered to it:

22 The semiperimeter of a triangle is found by the following formula:

Heron's formula for the area of ​​a triangle:

The area of ​​a triangle in terms of the radius of the circumscribed circle:

Median Formula:

Bessector Property:

Bisectress formulas:

The main property of the triangle heights:

Formula of height:

more useful property of the triangle heights

Cosine theorem :

Sine theorem :

Radius of a circle inscribed in a regular triangle:

The radius of the circle described near the correct triangle:

The area of ​​the correct triangle:

Pythagoras for a rectangular triangle ( C - A hypotenise, A B 9000 - Cathed