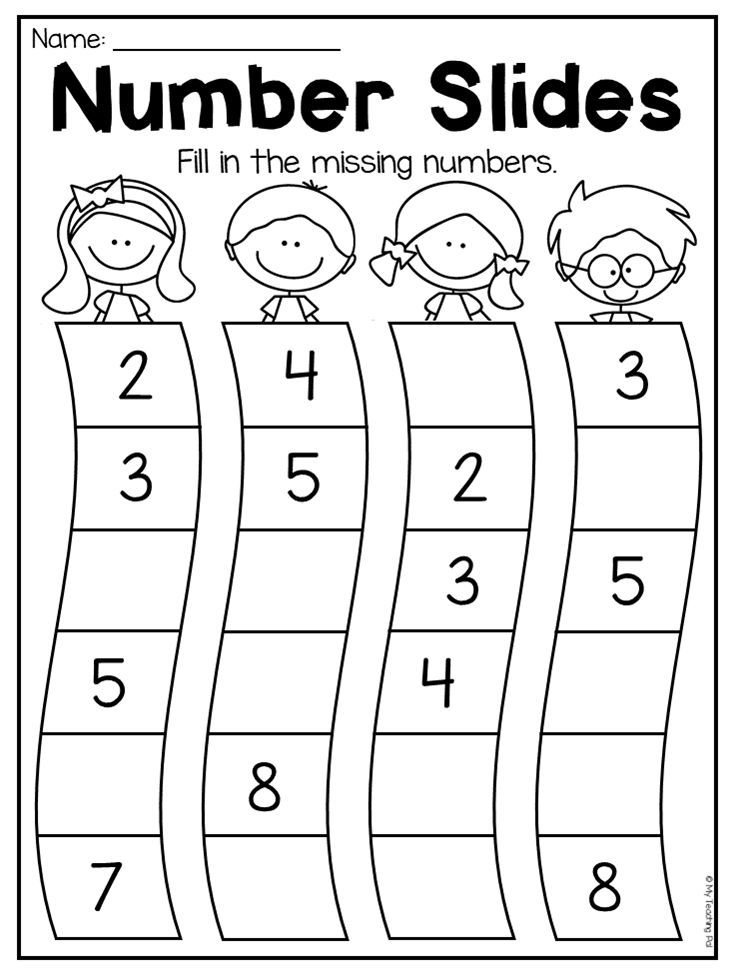
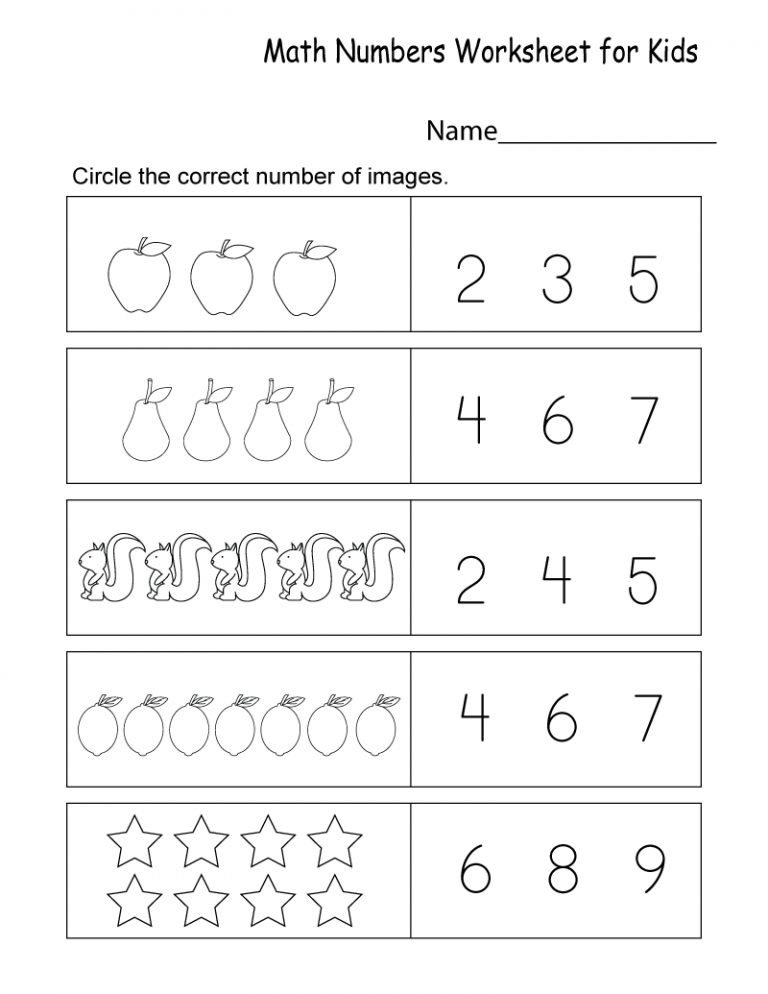
End of year math activity
10 Awesome End of Year Math Activities for Grades 1-8 — Mashup Math
Looking for Effective and Easy End of Year Math Activities?
The final weeks of the school year have arrived and keeping your students engaged in learning math is no easy task.
You can mix up your instruction and keep your students interested in learning math by incorporating a few awesome end of year math activities and projects into your upcoming lesson plans.
The following end of year math activity ideas are perfect for implementing during the final weeks of the school year, an extremely hectic time when sticking to your usual routine is nearly impossible.
And each end of year math activity is easy to modify to best meet the needs of your students! The activities can be modified for students at the elementary, middle, and high school levels.
So, which end of year math activity will you share with your students this spring?
(Tags: end of year math projects, end of year math activities, 1st grade, 2nd grade, 3rd grade, 4th grade, 5th grade, 6th grade, 7th grade, 8th grade, grade 1, grade 2, grade 3, grade 4, grade 5, grade 6, grade 7, grade 8)
1.Description: Standard math curriculums hardly ever make time for students to actually read about math. The end of the school year is the perfect time to give your students an opportunity choose a book about math and create and share a book report.
One option is to have your students research a famous mathematician. I also like having students choose a math-related fiction book, like any of the ones included on this list of 13 STEM Fiction Books Your Kids Will Love.
There are tons of excellent math-fiction books available for students of all grade levels.
by GeometryCoach.com
Description: This project blends elements of the history of math, geometry, and art/design into an engaging learning experience where students use mathematical thinking to manipulate shapes to create “Escher tiles” and use them to create geometric works of art!
Tessellation Monsters via www.
artwithmrsnguyen.com
Description: The Barbie Bungee data collection activity may be the most underrated math activity ever. Ideal for pre-algebra students, this activity gives students a fun and engaging opportunity to make predictions, collect real-world data, and then analyze the results within the context of their initial hypothesis. This activity is even endorsed by the National Council of Teachers of Mathematics (NCTM), who share free lesson resources for any teacher interested in trying the Barbie Bungee in their classroom.
Image via http://fawnnguyen.com
4.) Escape Room!Description: Classroom ‘escape room’ lessons are growing in popularity. Why? Because classroom escape rooms boost student excitement and engagement while helping your kids apply and improve their mathematical problem-solving skills.
If you are new to the idea of classroom escape rooms (sometimes called breakout challenges), check out this blog post So You Want to Build a Classroom Escape Room… by We Are Teachers.
Are you ready to turn your classroom into an escape room for a day?
5.) The Parachute Projectby Amy at www.allabout3rdgrade.com
Description: Students use their math skills to design and build a parachute that will stay in the air the longest. This STEM activity is a great opportunity for math and science teachers to collaborate—and it’s a great activity to do outdoors!
Image via www.allabout3rdgrade.com
Are YOU looking for some super fun daily math puzzles for your students in grades 3-8? Our best-selling 101 Daily Math Challenges workbook is now available as a PDF download!
6.
) Plan and Budget Your Dream Vacationby Jennifer at www.loveteachingkids.com
Description: Students are given a budget and have to work groups to plan all aspects of their determined “dream vacation.” This end of year math activity allows students to learn more about budgeting, personal finance, and real-world math applications. Aside from vacation planning, this activity can be modified to budgeting things like personal finances, events, starting a business, etc.
Image via www.loveteachingkids.com
7.) Build Fraction Kitsby Anthony at www.youtube.com/mashupmath
Description: Many students struggle with fractions and never really grasp the important concepts. Whether you want to help fortify your students’ understanding of fractions before the school year ends or you want to expose them to fractions a little early, this simple hands-on activity is highly effective and is definitely worth giving a shot!
Click here to get your Free Fraction Kit Lesson Guide!
Image via www.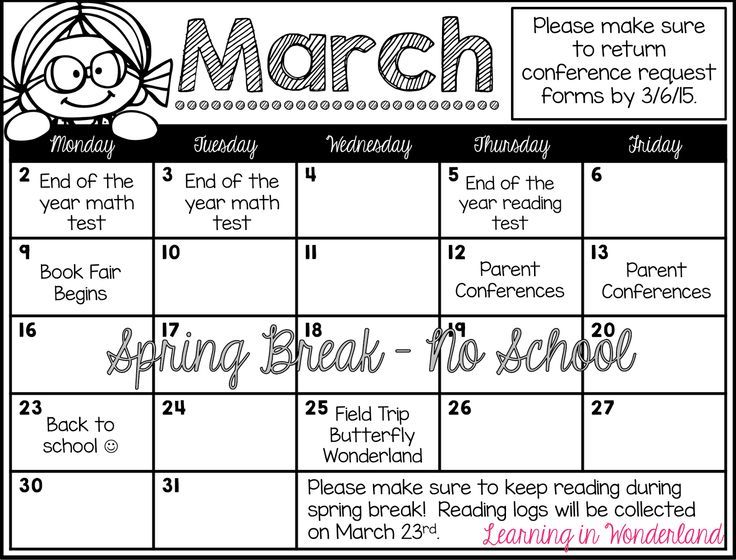
mashupmath.com
By Glimmersnaps Homeschool
Description: Students explore and apply the concepts of area and perimeter by building a city using LEGO bricks! This hands-on activity is another great example of how math teachers can use LEGO to teach challenging concepts.
If you don’t have access to Lego bricks, check out this free STEM: Area and Perimeter City activity guide by teachbesideme.com.
Image via www.glimmersnaps.com
“I always look forward to getting my Mashup Math newsletter email every week. I love the free activities!”
-Christina R., 5th Grade Math Teacher, Dallas, TX
Do YOU want free math resources, lesson activities, and puzzles and games for grades 1-8 in your inbox every week? Join our mailing list and start getting tons of free stuff (including a free PDF eBOOK)!
Sign-Up For FREE
9.
) Year End Reflection Activityby Brain Waves Instruction
Description: In this activity, students take time to reflect on what they learned in math this past school year, what challenges they overcome, and how they’ve grown as problem solvers. This activity is perfect for teachers who have students complete a math portfolio over the course of the school year.
Image via www.fouroclockfaculty.com
10.) Math Puzzles and Brain TeasersDescription: The end of the school year is a great time to let your students apply their mathematical problem-solving skills to fun puzzles, brain teasers, and challenge questions.
You can access dozens of free math puzzles for all grade levels by visiting our Free Math Puzzles page.
Shop now
Have any more ideas for awesome end-of-year math project ideas? Share your thoughts in the comments section below!
(Never miss a Mashup Math blog--click here to get our weekly newsletter!)
By Anthony Persico
Anthony is the content crafter and head educator for YouTube's MashUp Math and an advisor to Amazon Education's 'With Math I Can' Campaign.
You can often find me happily developing animated math lessons to share on my YouTube channel . Or spending way too much time at the gym or playing on my phone.
1 Comment
52 Fun End of Year Activities for Math Class
This blog post contains Amazon affiliate links. As an Amazon Associate, I earn a small commission from qualifying purchases. This comes at no cost to you. Thanks for your support of Math = Love!
Here in Oklahoma, the school year has come to an end. Given that some states still have a month or so left of school, I guess it’s not too late to share this round-up of end of year activities for math class.
The last few weeks of school are filled with awards assemblies, fun days, final exams, and technology turn-in days.
Though I try to continue teaching content as long as possible (much to my students’ dismay), I still find myself needing a fair number of one-off lessons to keep students engaged and occupied on those days when I only end up seeing one or two of six classes on a given day.
Over my last ten years in the classroom, I’ve put together quite a list of 52 different end-of-year math activities, so I decided it was time to put them together all in one place here on my blog.
STEM Building Challenges
Impossible Domino Tower
If you have access to a box of dominoes, you can definitely keep students busy for a while with the Impossible Domino Tower challenge from Ivan Moscovich. The challenge is definitely not impossible, but it will definitely appear that way to students at the beginning. I love watching students tackle this STEM building challenge as they try different approaches.
Impossible Domino Bridge
The Impossible Domino Bridge is similar to the Impossible Domino Tower challenge.
This challenge also comes from Ivan Moscovich, and it only requires a box of dominoes for students to complete.
Marble Roller Coasters
Years ago, when I was teaching physical science, I had my students build marble roller coasters using pipe insulation (super cheap at a hardware store like Lowe’s), marbles, and tape. This would make a great end of year activity as well. Students love the thrill of trying different designs and seeing what sort of crazy designs they can create.
Stellated Icosahedron Straw Art
If you want to keep students busy for an extended period of time, challenge them to build stellated icosahedrons from clear plastic straws and curling ribbon. Janelle Graham shares step-by-step directions and tons of tips for doing this fun hands-on project with your students.
Marshmallow Challenge
I typically use the Marshmallow Challenge with students as a team-building activity at the beginning of the year, but it would also make a great STEM building challenge for the end of the school year as well.
How tall of a tower can your students build using spaghetti and tape? Oh, did I mention that the tower needs to support a marshmallow at the very top?
Games
Train Game
The Train Game (20 Express) is one of my go-to activities for the first week of school. If you played this game with students at the beginning of the year, it would be a lot of fun to revisit the game again at the end of the school year. If you didn’t play the game with students, the end of the school year is the perfect time to introduce a new game. I use dry erase pockets with the game boards so that I only have to print a single class set of game boards.
If you don’t have a classroom set of dry erase pockets, you could also use heavy duty sheet protectors. But, I highly recommend investing in a classroom set of the pockets since they are so much more durable.
Make a Million Math Game
Julie Morgan’s Make a Million Game is a fun dice-based game that encourages critical thinking about place value.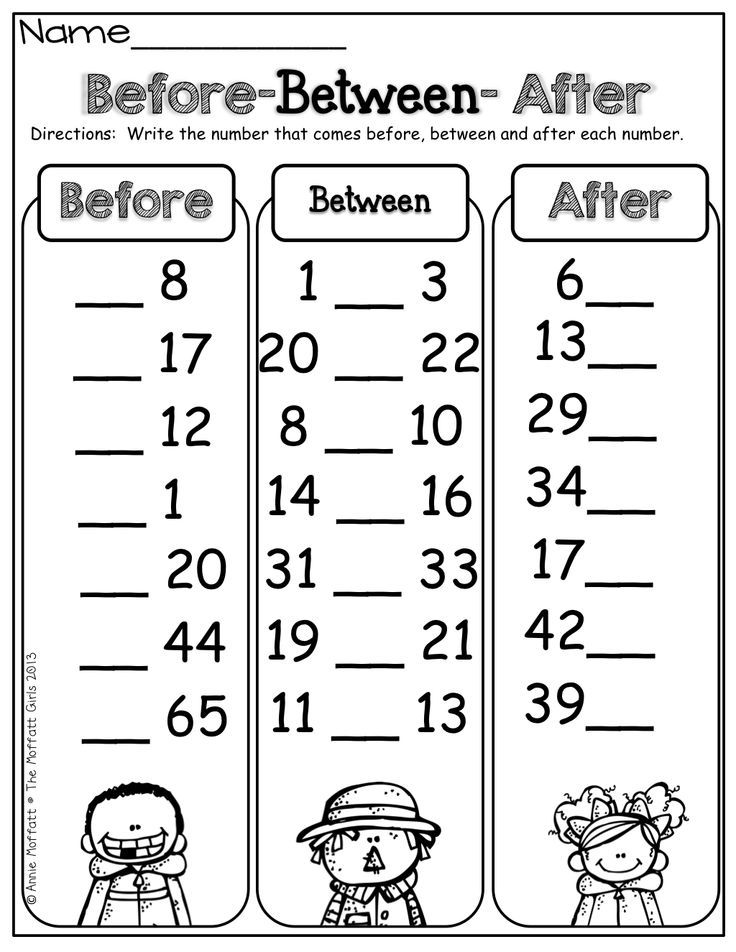
You will need a 10-sided die in order to generate the digits for students to place on their game boards. Like the Train Game, I print a class set of game boards and give them to students in a dry erase pocket.
Traffic Lights Game
Traffic Lights is a fun game from NRICH which is easy to teach students but hard to master. You will need red, yellow, and green bingo chips for students to play with. You could also cut up sheets of red, yellow, and green paper to make playing pieces if you need to.
The Game of SET
During the next to last week of school this year, I taught my statistics students to play the Game of SET. I originally learned how to play at a Math Teachers’ Circle event. The rules can be a bit tricky for students to wrap their minds around at first, but Greta Bergman’s Desmos Activity Builder introduction to the Game of SET is brilliant!
Sprouts
Sprouts is a fun paper-and-pencil math game, but I usually teach my students to play Sprouts with individual dry erase boards.
Students love competing against one another in this simple game that only involves drawing dots and lines.
Cover Up Game
Cover Up is a free printable dice-based game from Frank Tapson. Students play against one another in pairs. Each student gets a game board featuring the numbers from 1 to 12. They must race to roll the dice and be the first to cover up their entire game board with either bingo chips or two-colored counters. This game would make a great addition to a unit on probability or as a stand-alone game at the end of the school year.
Skittles Game
Skittles is another fun two-player game from Frank Tapson. Like Cover Up, the game of Skittles also involves rolling dice. However, the students must share a game board, and the goal of the game is to remove the most counters (I typically use bingo chips) from the game board.
Manifest Game
If dice games aren’t your thing, check out this card-based game from Frank Tapson called Manifest. This game is designed for two players, and students must compete to see who can build the largest numbers using the cards in their deck.
The thing I love about this game is that students must make all of their playing decisions at the beginning of the game in secret. Then, they are revealed one at a time to see who wins. This is a very fun strategy-based game that students will beg to play over and over!
Farkle
Farkle is one of my favorite dice games to play with family and friends, so it probably isn’t that much of a surprise that I love to teach the game of Farkle to my students each year. I love how few supplies the game requires. Each group of students will need six dice and a score sheet that I like to print and place in dry erase pockets for easy reusability. I offer a free printable farkle score sheet on my blog that features a summary of the rules/scoring guidelines at the bottom for easy reference.
Thirteen Game
If you are looking for a quick game that involves the entire class at once, look no further than the Thirteen Game! I learned about this game from Julie Morgan, and it has been a hit with my classes ever since.
Students must stand in a circle and strategize about how to not end up saying the number thirteen.
Warning: if you teach students to play this game, they will continue to start up games of it on their own whenever there are five random minutes left at the end of class.
5 x 5 Game
Sara VanDerWerf’s 5 x 5 Game is a ton of fun. It only requires a set of printable game boards for students and a deck of playing cards for the teacher. Students love competing to see who can come up with the highest score! This year, I used Kurt Salisbury’s Desmos Activity Builder version of the game, and I really liked how it made sure how students understood the game’s scoring system before moving onto the actual game!
Game of 24
If you are looking to fit in a bit more mental math practice before the end of the year, check out the Game of 24. Students must race to figure out how to combine the four given numbers to make a target number of 24.
Witzzle
Another favorite mental math game is Witzzle Pro! I love that this game allows you to randomly generate target numbers for students to create using all of the numbers in a row, column, or diagonal of the playing card.
When I offer candy for whoever figures out how to get the target number first, my students become super competitive. I also love that this game allows for negative target numbers!
Some years, I have built an interactive Witzzle bulletin board in my classroom to allow me to play with students anytime we have a few spare minutes. It also works great as a brain break activity!
Jumbo Tic Tac Toe
My students love to play tic-tac-toe, so I decided to make a jumbo set of tic-tac-toe pieces (magnetized with disc magnets on the back of each piece) so that students could easily play a game of tic-tac-toe on the dry erase board.
Wild Tic Tac Toe
We had so much fun playing with the jumbo tic-tac-toe pieces, that I decided to introduce some tic-tac-toe variants like Wild Tic Tac Toe to my students. They enjoyed trying some new strategies with this familiar game with slightly different rules.
Tic Tac Toe Dice Game
While searching for different tic tac toe variants, I also ran across this fun tic-tac-toe dice game to share with you all.
Numerical Tic Tac Toe
This last tic-tac-toe variant is perfect for math class since it involves numbers instead of X’s and O’s.
Brainteasers
Likes and Dislikes Brainteaser
I like jelly, but I don’t like jam. I like food, but I don’t like eating. I like soccer, but I don’t like sports. I like puzzles, but I don’t like brainteasers.
Can you and your students figure out the secret to what I like and dislike in this fun brainteaser?
Petals Around the Rose
One of my most favorite brainteasers is Petals Around the Rose. The only supplies you need to introduce students to this perplexing puzzle is a set of five dice. This is one of my go-to activities for the first week of school, but it is perfect for any time during the school year.
Camel Crossing the Desert Puzzle
I discovered this camel crossing the desert puzzle for the first time in the Discovering Algebra textbook. But it wasn’t until I later attended a math teaching workshop that I finally set down and played with the puzzle.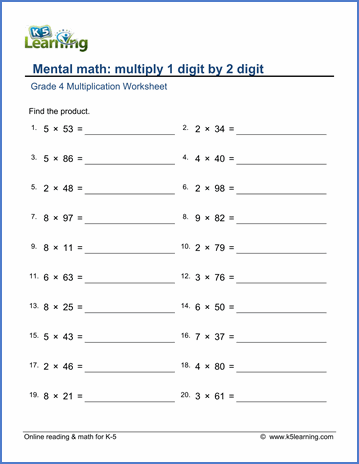
This brainteaser is easy to explain, but your it will drive your students crazy for possibly multiple days!
The Proof is in the Pudding Brainteaser
Another fun brainteaser is called “The Proof is in the Pudding.” Can your students decipher the clue given to the census taker to determine how old the man’s children are? This is one of those puzzles that seems like it doesn’t give you enough information, but I promise it does!
Paper and Pencil Puzzle Packets
Strimko Puzzle Booklet
I am a huge logic puzzle fan, and one of my favorite new logic puzzle finds are Strimko puzzles. Check out this free printable book of strimko puzzles from the Grabarchuk family. My students always say these puzzles remind them of “sudoku but more fun.”
Make Six Puzzle
The Make Six Puzzle looks simple, but it will definitely exercise your students’ brains as they try to find all of the solutions. I recommend printing a class set of this puzzle and giving it to students in dry erase pockets.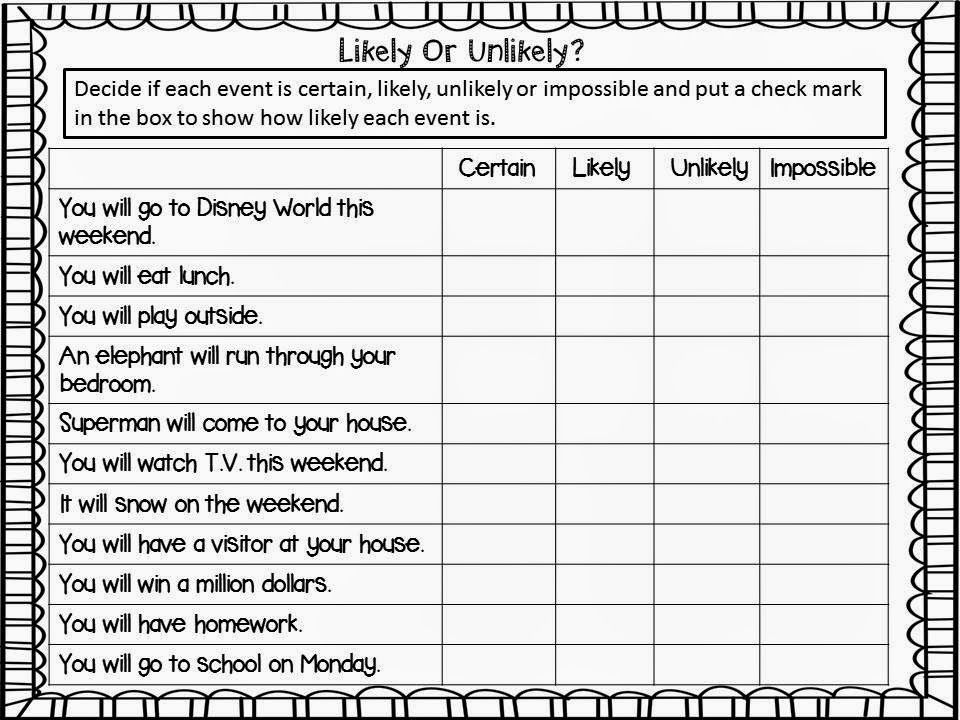
Can you make each equation equal six?
20 x 9 Challenge
The 20 x 9 Challenge asks students to create the number twenty in nine different ways. Some of the solutions are easy to find. Others will drive your students crazy!
How Far Can You Climb?
If your students are competitive, they will love this How Far Can You Climb? Puzzle from Frank Tapson. I give this puzzle to students in a dry erase pocket so they can erase their paths through the maze as they try to find the highest scoring route.
5-4-3-2-1 Challenge
This 5-4-3-2-1 Challenge is a fun review of the order of operations and an excellent reminder of the importance of parentheses! How many of the forty solutions will your students be able to find?
Hidato Puzzles
Hidato Puzzles (or Hidoku Puzzles) are a creation of Dr. Gyora M. Benedek, an Israeli mathematician. The Hebrew word “hida” means riddle. In a hidato puzzle, you are given a grid with a selection of the numbers already filled in.
Your task is to fill in the missing numbers so that each number connects to the next number either horizontally, vertically, or diagonally. 1 must connect to 2, 2 must connect to 3, and so on.
Shikaku Puzzles
Shikaku Puzzles are a geometric-based logic puzzle from Japan. The goal is to subdivide the grid into rectangles (and squares) so that the number in each rectangle refers to the area of that rectangle. Only one number may appear in each rectangle. Additionally, no rectangles may overlap.
Kazu Sagashi Puzzles
I prefer to call these Apple and Orange puzzles when I introduce them to students because they involve drawing boxes that include various numbers of apples and oranges. Sounds simple, right? I promise that these Kazu Sagashi puzzles from Naoki Inaba will keep your students entertained and puzzled for a good 30 minutes!
Hands-On Puzzles & Activities
Let’s Make Squares Activity
I used the Let’s Make Squares activity from Kagan’s Cooperative Learning book on the first day of school this year, but if you didn’t use it during the first week it will make a perfect cooperative group activity to wrap up the end of the school year.
Your students will need popsicle sticks or strips of colored paper to use to build the given number of squares. This activity is a great conversation starter, and it involves lots of critical thinking.
Ink Stain Matching Activity
I have used this ink stain matching activity both with students at the beginning of the school year and the end of the year. Students must pretend that a group of objects has been entirely covered in ink. They must match up a set of ink-stained hands with the objects to determine which hand handled which object.
Students really enjoy this fun challenge, and it is a definite conversation starter!
Lonesome Llama Activity
The Lonesome Llama activity requires students to work in a group and practice their communication skills as they try to determine which card in the deck does not have a match. If you tried this activity with students at the beginning of the year, there are several other versions featuring different objects at the bottom of the post that you could try with your students.
DIY Instant Insanity Puzzle
All you need to make your own DIY Instant Insanity Puzzle is a set of wooden cubes and some colored paint or dot stickers. Once the puzzle is built, will your students be able to build a tower using the blocks that does not repeat colors on any of the sides? As the name implies, this puzzle will drive your students insane!
Tangram Puzzles
I believe that every classroom needs a set of tangrams. Check out this free collection of printable puzzles for your students to attempt to build using their tangrams.
Panda Squares Puzzle
Panda Squares is a fun color-matching puzzle with tons of different solutions for students to find. If you have ever played Izzi before, Panda Squares is an easier printable version of that puzzle. It was originally created by Ivan Moscovich, but it has been renamed and popularized by David Butler.
Pentominoes Challenges
In addition to a set of tangrams, I also think each math classroom needs a set of plastic pentominoes.
There are so many different puzzles that you can do with students. I usually start them with this set of 13 free printable pentominoes challenges, then we venture off into the Star Pentominoes Puzzle, Elephant Puzzle, Terrier Puzzle, and Penguin Puzzle.
Skyscraper Puzzles
These last two hands-on puzzles involve linking cubes. These skyscraper puzzles are a latin-square type puzzle that require students to figure out where various skyscrapers must be built based on numbers written around the outside edge of a grid. These numbers tell how many skyscrapers can be seen from that vantage point.
These puzzles take a bit of explaining for students to wrap their minds around, but they can occupy students for an entire class period after the initial teaching process!
Build It Activity
Build It is a cooperative, team building activity from the book Get It Together: Math Problems for Groups that encourages students to work together, communicate, and think logically as they construct a geometric object from linking cubes to satisfy a set of given clues.
If you’re looking to work on vocabulary, this activity introduces/reinforces words such as “face” and “edge.”
Origami and Paper Folding Projects
Modular Origami with Sonobe Units
I love introducing students to modular origami. This is a great class-wide origami project since there is only one piece that students must learn to fold. After students learn to fold the sonobe unit, they can build as many pieces as they want and assemble them to build various different objects.
Hexaflexagons
Hexaflexagons are a fun paper-based toy that is created from a strip of equilateral triangles. Students will love discovering and coloring the different sides of the hexaflexagon. If your students love playing with fidget toys, think of the hexaflexagon as the ultimate build-it-yourself fidget toy.
Crazy Eight Paper Folding Puzzle
The crazy eight paper folding puzzle is actually eight different puzzles in one. It’s easy to build, but hard to solve! I love dividing my dry erase board up into eight different sections so students can add their name to each section as they solve each of the paper-folding puzzles.
Origami Columbus Cubes
An origami columbus cube tower is a fun origami project that creates an impressive and eye-catching final product. I have a columbus cube tower on my desk as a fun, mathematical decoration, and students beg all year to learn how to build one themselves. The end of the school year is the perfect time to let students build this fun origami project.
Eight Lettered Squares Puzzle
Similar to the Manifold Origami Puzzle, the eight lettered squares puzzle gives students a strip of paper featuring eight letters which they must fold so that the letters are in alphabetical order. It’s easy to explain, but hard to figure out!
Reflection Activities
Learning from A to Z Activity
Help students to reflect on everything they have learned over the course of the school year with this Learning from A to Z Activity. Students must come up with something they learned that starts with each and every letter of the alphabet.
Alphabetical Advice
I also like to have my students reflect on the year by having them create a set of advice for future students beginning with each letter of the alphabet.
I have each student write their own advice, then we compile a set of the best alphabetical advice for future students.
End of Year Concept Maps
Another way to encourage students to reflect on the past school year is to create a concept map summarizing the main ideas of the course and their connections. It always impresses me what students come up with!
End of Year Letters of Advice
My students always groan when I ask them to write letters of advice to future students at the end of the year. They are always a lot of fun for me to read, and they give me great feedback on what my students enjoyed and did not enjoy during the school year.
My Favorite Puzzle Books
100 Geometric Games
Buy Now →100 Numerical Games
Buy Now →Puzzle Box, Volume 1
Buy Now →Puzzle Box, Volume 2
Buy Now →Puzzle Box, Volume 3
Buy Now →The Big, Big, Big Book of Brainteasers
Buy Now →Strimko Book 1
Buy Now →The Original Area Mazes
Buy Now →The Big Book of Brain Games
Buy Now →More Printable Paper and Pencil Logic Puzzles
“At all times, mathematics has become a bridge between the islands of misunderstanding in the world,” Nikolai Andreev
, the creator of the project "Mathematical Etudes" Nikolai Andreev.
We asked him to talk about this event, as well as about the work of the Laboratory for the Popularization and Propaganda of Mathematics, which he heads at the Mathematical Institute. V. A. Steklov RAS (MIAN). nine0004
Nikolai Nikolaevich, your project "Mathematical Etudes" is widely known in the country, it has many awards and distinctions at the national level, and the Lilavati Prize also means its international recognition. How important is it?
Russia does not have many prizes of the International Mathematical Union, and this is the highest award in our community, and, of course, it is a great honor to receive it. But, in my opinion, this is not only recognition of our project, but in general recognition of the traditions and contribution of our country to the popularization of mathematics. The book “Mathematical Component” published within the framework of our project has a chapter “Bookshelf” with a list of the most interesting literature on mathematics. I had to carefully select books for her, because indeed in Russia at different times a lot of very high-quality popular science literature was published.
nine0005
And although in our project we have started using 3D computer graphics, we are trying to keep the main feature of the traditional Russian approach to popularization, which is that it is necessary not only to show some kind of trick, but also to teach something. At one time, we even had a slogan: new forms of tradition.
International recognition is an important step, since we mainly worked in Russia, for example, all lectures were held in Russian. It is all the more pleasant that this work was also appreciated abroad. Before the prizes are presented, the laureates are introduced, and we were represented, in my opinion, by the best popularizer of modern mathematics - Tadashi Tokieda from Stanford. I highly recommend everyone to find recordings of his performances on YouTube. His lectures are always very interesting, his education - both general cultural and mathematical - knows no bounds. nine0005
The main page of the site "Mathematical Etudes".
Are there any plans to develop the project abroad in connection with this success?
In fact, the site "Mathematical Etudes" is partially translated into English, and not only into English, but also into French and Italian, and will continue to be translated.
As for his foreign prospects, everything is simple: such high-quality material appears extremely rarely and, of course, it is valuable in all languages. nine0005
How much did the political situation influence the atmosphere during the congress?
Of course, if the congress were held in St. Petersburg, as originally planned, there would be much more attention both at home and abroad, however, I hope that after some time the situation will improve. Mathematicians are lucky, because the theorems, being proved, do not change from political views, therefore, at all times, mathematics has become a bridge between the islands of misunderstanding in the world. It is to be hoped that this will continue to be the case. nine0005
One of 71 animated films of the Mathematical Etudes project.
How did your project start and how did the Laboratory for Popularization and Propaganda of Mathematics at MIAN come about?
We started popularizing mathematics back in 2002, and the prize is, in a sense, a gift to us for our 20th anniversary.
The first computer films were made on the scientific problems that I was involved in. When it became clear that they were of great interest both in the scientific community and among teachers and schoolchildren, we decided to continue this work, and in 2010, on the initiative and with the support of our then director Valery Vasilievich Kozlov, a laboratory was created - a small , but important for two reasons. nine0005
First of all, only a person who understands science can tell well about science. You need to know much more than what you are talking about in order to be able to select from this knowledge what is useful for an unprepared person to know. And the second aspect is that in this room where our laboratory is located, many employees of the institute come and share their ideas about the interesting things they have learned in the world of science. And we try to use them for promotion.
It is important that in this way we introduce new ideas into the world of popularization.
They can draw beautiful pictures for the well-known "Entertaining Mathematics" by Yakov Isidorovich Perelman without us, although even this cannot be done without mathematical preparation. A separate merit of Roman Koksharov, the graphic designer of all our projects and, in particular, the book "Mathematical Component", that all his pictures are mathematically accurate. The same applies to the films on our Mathematical Etudes website. In them, everything is correctly added up, laid out and moved from the point of view of mathematics, and this is very important, because, firstly, it is not misleading, and secondly, everything must be honest in the popularization of science. Everything you say must be true. You may not be telling the whole truth, because some things are very hard to explain, but what is said must be true, including pictures. nine0005
One of the spreads of the book "Mathematical component".
You said that the first animations came from your mathematical work.
Was it part of them?
No, of course, this was not some kind of scientific mathematical research. It just seemed that the task that I was doing could be more understandable and accessible even to an unprepared person in the form of computer animation. And it soon became clear that this was indeed the case. nine0005
What were the problems that first gave you the idea of computer animation?
In fact, these tasks are very close to what Marina Vyazovskaya received the Fields Prize for this year. She solved the problem of packing balls in 8- and 24-dimensional space. I've been working on related issues. One of them is the Thompson problem about the arrangement of electrons on a sphere, when you throw several electrons that repel each other, but they are forbidden to leave the sphere, and you need to find a configuration of their arrangement with the minimum potential energy of the system. And the second problem is the problem of the contact number of balls.
You have a ball, and you need to understand in different dimensions how many of the same balls can touch the central one. This is actually a very practical problem about spherical error-correcting codes. The first films were shot as an explanation of these works, it was they that laid the foundation for our project, although now we are reviewing them with a smile, because since then we have advanced a lot technically. nine0005
One of the first 3D animations of the project was devoted to solving the Thompson problem.
How is this progress expressed?
After all, a lot of experience has already been accumulated, a presentation style has been developed. More than a thousand lectures were held in completely different audiences, most often among schoolchildren and teachers, and not only from some special schools. You look at their reaction, sometimes you understand that you need to show it differently here. We, as a rule, first test our films at lectures, somehow change them, and then we put them in open access on the Mathematical Etudes website.
Relatively recently, about a year and a half ago, they started a VKontakte page and introduced mathematical Tuesdays there, when something new appears every week on that day. nine0005
We make not only virtual things for the Internet, but also material visual aids. We dreamed - and continue to dream - to present our models to all schools, but this requires large finances. In the meantime, we have opened the project “Mathematical Model to School!”: if a student himself or with his parents makes some kind of model and gives it to the school, then we send him our book “Mathematical Component” as a gift. Apparently, this interested the children, and we send one or two books a week during the school year in response to sent photographs of models made for schools. I would like the math classrooms to be filled with visual aids. This, of course, will not revolutionize education, but it will help to take some small step towards making children understand mathematics better and make it more interesting for them to study, and when there is interest, this is the key to a good study of the subject.
nine0005
Nikolai Andreev dreams of giving his mathematical models to all schools.
Popularization is an urgent problem for any science, but I have not heard that laboratories for the popularization of physics, chemistry or electronics were created at other institutes of the Russian Academy of Sciences. Why did the management of the Mathematical Institute decide to create such a division?
You know, Valery Vasilievich Kozlov, now Academician-Secretary of the Department of Mathematical Sciences of the Russian Academy of Sciences, and in those years the director of the Moscow Institute of Astronomy, did a lot both for the development of the institute and for science. His intuition and vision of the problem also played a role in the creation of the Popularization Laboratory. The importance that he attached to the creation of the laboratory is emphasized by the fact that our institute is actually small compared to others, although in terms of the number of members of the Academy of Sciences who worked and are working within these walls, if I am not mistaken, it is in the lead: by 150 employees we have 15 academicians and 17 corresponding members of the RAS.
nine0005
In this sense, the award can be considered another confirmation that the idea of creating a laboratory has fully justified itself. Indeed, by and large, our project has no other awards, including the Prize of the President of the Russian Federation in the field of science and innovation, the Gold Medal of the Russian Academy of Sciences and now the Lilavati Prize. We hope that this example will show that it is possible and necessary to create similar structural units in other institutions. Of course, one should not compare the pursuit of science and the popularization of science, but this is also an important and necessary thing that requires a lot of effort and time, and I want those employees who know how to do this - both to read lectures and prepare materials - to go into some credit for work. nine0005
You said that only a scientist can speak well about science, but I had a case when one mathematician answered my request to popularize the essence of his work, which was noted by the Academy of Sciences, frankly replied that, unfortunately, this is absolutely impossible.
It turns out that only a mathematician can understand mathematics?
Indeed, there are areas that cannot be explained to the general public. Moreover, there are areas of mathematics that I myself, for example, do not understand and therefore do not undertake to explain them, because the entry threshold there is such that you need to work for many years before you can at least tell something about these areas. But I did not say that everything can be explained in mathematics. I said that only a mathematician can explain mathematics qualitatively. Moreover, it is always nice when the specialist himself talks about his work, otherwise it turns out to be a kind of secondariness, and even when journalists take up explanations, it turns out to be quite sad. And we, by the way, took this into account in our book "Mathematical Component", which has three parts: the simplest, about the manifestations of mathematics in everyday life, it was written by the laboratory staff Nikita Panyunin, Sergey Konovalov and me, and the other two parts contain the author's articles of the leading mathematicians of the country, and to get such an explanation first hand is very important and useful.
nine0005
What is the specificity of the popularization of mathematics?
Mathematics is distinguished by the fact that it deals mainly with abstract concepts. Physicists or chemists can make a visual demonstration of their experiments, show equipment, demonstrate the results of experiments - and the audience will be impressed, and mathematicians still have to figure out how, in addition to incomprehensible formulas, to explain abstract things. This, in fact, is the main difficulty, therefore one of the main activities of our laboratory is the creation of visual mathematical models in the form of computer animation or in physical form. nine0005
The second difficulty is to choose mathematical subjects that can be presented beautifully. After all, the standard Pythagorean theorem from the school curriculum can be presented, as we did, in the form of a puzzle, which, by the way, is very popular, but to do this, again, you need to know a lot of things besides the school curriculum.
Technology does not stand still. For example, computer games are becoming more and more popular. Are you going to develop the project in this direction? nine0008
We are always trying something new. An interesting experience is the creation of applications for mobile devices. At the end of 2011, we first released the Mind App, which can be found on the App Store. This is an interactive implementation of the problem book "1001 tasks for mental arithmetic" by the great Russian teacher of the 19th century Sergei Alexandrovich Rachinsky. Interestingly, in the century before last, village children could solve such problems in their minds, while a modern person needs to puff a lot to cope with them. nine0005
This program gained immense popularity, and at some point came out on top in the main App Store rating and stayed there for quite a long time. It has been downloaded by millions of users. And this is an example of how modern devices, when used correctly, can be a channel for traditional ways of popularizing science.
I will also mention the geometry programs "Euclidia" and "Pythagoria", made by colleagues from St. Petersburg and also popular.
But isn't it a problem that the attention of today's children needs to be captured by something more and more visually spectacular? After all, new gadgets appear all the time, like virtual reality glasses.
When we started using 3D computer graphics in 2002, it was really new and in itself became a powerful tool for attracting attention, but then some kind of art is required so that, having captured attention, you can tell something interesting and useful about mathematics, so that the matter is not reduced to entertainment alone. If we talk about virtual reality, then so far I don’t see anything interesting in it for mathematical teaching. If done well, it is not always necessary to chase fashion or external effects, and in support of this I will give two examples. nine0005
We started with computer graphics, and then we came to the book "Mathematical Component", but we made it very high quality, and it was sold in huge circulation despite the fact that it was posted for free on the Internet.
After all, it is a separate pleasure to sit and think about a book.
And the second example is Quantik magazine, a stunningly designed monthly magazine for the curious, and not just schoolchildren. I also find a lot of interesting things in it. This magazine is successful, it is subscribed. All the children you know, when you bring them Quantik, they freeze with it where they received it. And it still retains the traditional genre of correspondence with readers, namely, a monthly competition is held, tasks are published, children solve them, write to the editor, and the editorial board answers them what is right and what is wrong. This experience shows that content is more important than form. The issue of quality is much more important than being in the race for modern technology. nine0005
Quantik magazine covers.
Why is there no sound in your animated sketches?
Because they are designed for thoughtful viewing, which sometimes needs to be stopped to read an explanatory article.
Cognition requires full concentration and its own pace. Even the background music gets in the way. In addition, these films are designed to be shown to completely different audiences, someone just needs to be shown and superficially told, while someone can make a scientific report based on the film. Here the sound would only get in the way, because each lecturer can tell in his own way. nine0005
There are many tapes of my lectures on the Internet where I show films and very often stop them to take small - or large - digressions depending on the reaction of the audience. These films are a kind of universal thing, and, by the way, this is exactly what was noted in the presentation for the award, which was made by Tadashi Tokieda. He said that this is a material that everyone can use at their own discretion. Our films and our models provide a wide range of possibilities for their use in a variety of situations. nine0005
One of the interesting projects of the laboratory is the creation of models of Chebyshev mechanisms.
Computers are already beating people at chess, writing poetry, composing music, drawing pictures. It is possible that artificial intelligence will soon replace, for example, journalists. Can he ever replace mathematicians?
Journalists may be replaced, but certainly not mathematicians. Even a math teacher cannot be replaced, because when teaching, human communication with children is very important. You can’t just put a TV in the classroom and start some kind of talking head on the broadcast, although we, unfortunately, are moving towards this, but this will be the end of education. After all, it’s one thing when all these materials are just on the site, even if many people use them, and another thing is real lectures when I come to different cities and show all this. We manage to interest those schoolchildren who themselves would never have come to this site and have not looked at our book. Those who are keen on mathematics will look at our studies themselves, but it is our task to change the attitude of those who consider this science uninteresting.
nine0005
A teacher is human communication that a computer cannot replace, but why can't artificial intelligence replace mathematics?
It will not replace, because before solving a problem, it is necessary to choose and set it, and only a person can do this. Mathematics is not reduced to the mechanical derivation of one formula from another. Mathematics is the understanding of the world and how it works. And with this task, except for man, no one can cope, and humanity has to think about it all the time of its existence. nine0005
Do you use such factors as the beauty of mathematics, its philosophy in popularization?
Beauty and philosophy are two different things. In fact, music is based on mathematics, which is covered in a separate chapter in the "Mathematical component". A lot of mathematics in the engravings of Maurice Escher. As for the philosophy of mathematics, then, as I said, in the "Bookshelf" section in the same book "Mathematical Component" we have given a list of literature that would be useful in school mathematics classrooms, but in general there is a lot of interesting things to read and adults.
Among them are three books that appeared not so long ago, after Soviet times, glorious for the abundance of good books on mathematics. This is a book by our great scientist and popularizer Vladimir Igorevich Arnold, which is called "Mathematical Understanding of Nature", a book by Vladimir Andreevich Uspensky "Apology of Mathematics", and, in fact, why I remembered this list, it contains a book by our great mathematician Yuri Ivanovich Manin, titled "Mathematics as Metaphor". It is to this book that I refer those who are interested in the philosophy of mathematics. nine0005
I am very glad that Yuri Ivanovich took part in the creation of our book. His article in it is called "Mathematics - the language of description of possibilities." It ends with the following words: “Mathematics describes the phase spaces of the real world - the spaces of possibilities. It studies the laws that determine the possible trajectories in this phase space, as well as the conditions - the set of information that is necessary to select a particular phase trajectory.
That is, mathematics gives humanity not only specific tools, but also really in a sense tells about where it can move. nine0005
Interviewed by Leonid Sitnik, editorial board of the RAS website.
Photo by Elena Sitnik
Read also “Lev Beklemishev about the Lilavati Award to Nikolai Andreev”
Logic, Motivation and Solving problems, collective and individual work on complex issues, complex and individual work, and individual work on difficult issues, including those that go beyond the school curriculum: the course "Mathematical Laboratory" invites both children who are interested in mathematics, and those who have not yet tasted all the delights of this multifaceted science. nine0005
Teacher Boris has many years of experience in working in a German school and in various mathematical studios, including those for gifted children; he loves mathematics very much and brings amazing tasks to the lesson that do not leave any participant indifferent.
The main tasks of the "Mathematical Laboratory" are to consolidate and strengthen a positive attitude towards mathematics; to give children knowledge that will help them cope with the school curriculum and go beyond it; get acquainted with mathematics as an exciting, puzzling, fun and at the same time serious activity. nine0178 Math in a Suitcase is an intellectual adventure that brings results that are understandable and useful for children.
In the classroom, children will develop logical-analytical thinking and algorithmic skills.
Basic principles of work:
- exactingness through support: each student is offered tasks on the border of the level of mathematical development and motivation he has achieved
- understand, not imitate. Using understandable material, children will learn not only to correctly solve the problems set by the teacher, but to ask themselves and each other meaningful questions, independently compose puzzles and action plans, formulate assumptions, and find generalized solutions for several problems; study new mathematical problems worthy of attention and spending free time.
nine0194
The course consists of four modules "Regularities around us", "How to think correctly", "Fantasy in the service of mathematics" and "Culture of thought exchange".
At the end of the year, individual and group reporting project work is planned: both children and parents will be able to see the result of mathematical activity on the course.
If you are unable to pay by card or have problems registering, please contact the administrator to pay by cash or transfer. nine0005
Reviews
Lesson impressions
Dear Lena, Thank you very much once again for the opportunity to lift the curtain on the mystery of your little beautiful laboratory for teaching young mathematicians, although from the outside everything looks more like a good interesting game with a useful intent. I am completely delighted: what a wonderful atmosphere, what high involvement and interest from absolutely all participants (together with me 😁).
What wonderful smart kids and what a wonderful and exciting teacher you are. The atmosphere of a fun game, a lot of positive emotions, the lesson swept by in an instant, it was so interesting to me. Our children are very lucky to go to such a wonderful and interesting educational activity. Until now, before my eyes, hard-thinking cute children's faces and delight in shining eyes in case of a correct answer. I encourage all parents to take the opportunity to attend the class. nine0005
Mathematics for 7-8 years old
Hello, today we came to meet you. Daniel is our child, he was delighted, he liked everything, we would like to cooperate with you further with pleasure.
Developmental activities
I wanted to say thank you for the fairy tales.
Learn more
