First in math player at home
Pritchard, L / First in Math Player of the Day
Skip to Main Content
Select a School...
Select a School
- PreKindergarten & Early Childhood
- Clara Barton School No. 2
- Dr. Alice Holloway Young School of Excellence
- George Mather Forbes School No. 4
- John Williams School No. 5
- OACES Office of Adult & Career Education Services
- Virgil I. Grissom School No. 7
- Roberto Clemente School No. 8
- Dr. Martin Luther King Jr. School No. 9
- Dr. Walter Cooper Academy School 10
- Anna Murray-Douglass Academy School No. 12
- RECEC NE
- The Children's School of Rochester No. 15
- John Walton Spencer School No.
16
- Enrico Fermi School No. 17
- Dr. Charles T. Lunsford School No. 19
- Abraham Lincoln School No. 22
- Francis Parker School No. 23
- Nathaniel Hawthorne School No. 25
- Henry Hudson School No. 28
- Adlai E. Stevenson School No. 29
- Audubon School No. 33
- Dr. Louis A. Cerulli School No. 34
- Pinnacle School No. 35
- Andrew J. Townson School No. 39
- Community School No. 106
- Abelard Reynolds School No. 42
- Mary McLeod Bethune School No. 45
- Charles Carroll School No. 46
- Helen Barrett Montgomery School No. 50
- Frank Fowler Dow School No. 52
- Montessori Academy School No. 53
- The Flower City School No. 54
- World of Inquiry School No. 58
- All City High
- Early College International High School
- East Upper & Lower Schools
- Edison Career and Technology High School
- Franklin Campus
- James Monroe High School
- Joseph C.
Wilson Foundation Academy - Joseph C. Wilson Magnet High School
- Leadership Academy for Young Men
- LyncX Academy
- Northeast College Preparatory High School
- Northwest Junior HS at Douglass Campus
- P-TECH Rochester
- Rochester International Academy
- School of the Arts
- School Without Walls Commencement Academy
- NorthSTAR Social and Emotional Learning Center
- Home / Hospital Tutoring
- Youth and Justice Programs
- Annual Professional Performance Review
- Common Core
- Department of Bilingual Education & World Languages
- Special Education
- IM&T
- Professional Learning
- School Library System
- Student Support Services
- Virtual Academy of Rochester
- Summer Learning
-
About Us
- Contact Us
- School Based Planning Team
- School Profile
- Community Engagement Team
- School Events Gallery
-
Academics
- Academics Home
- Math PreK-6
-
Library
- Library
-
News
- "
- District News
- School News
-
Parents
- Parents
- Parent School Compact
-
Staff
- "
- Community School Coordinator
- Administration
- Main Office
- Nurse's Office
- Social Workers
-
SW-PBIS
- What is SWPBIS?
-
Teachers
- "
- Bolzner, E
- Brinson, K
- Brownlee, S.
- Brumaghim, V
- Buckley, J
- Burgess, J
- Dake, R
- Davison, L
- DeLorme, A
- Devine, R
- Falvo III, J
- Ferreira, S.
- Fisher, G
- Frenzel, D
- Gamble, J
- Gliwinski, E
- Grantham, J
- Guzman, C
- Hahn, T.
- Hansen, H
- Hashim, C
- Heisinger, M
- Hochadel, S
- Johnson, T
- Jones, T.
- Kelly, L
- Ketchum, M.
- Lanz, S
- LeGrett, J
- Leo, D
- Loewke, J.
- Lonadier, J.
- Mack, C
- Manengu, D
- Mercurio, J
- Meyers, D
- Mirrione, M
- Murty-Jackson, M
- Nagar, R
- O'Reilly, H.
- Ortiz, M.
- Osbourne, C.
- Pelliccia, M
- Persica, K
- Phinney, M
- Pickett, A
- Pritchard, L.
- Reyes, M
- Richardson, D
- Rongo, A
- Sampson, K
- Sasso, C.
- Sequeira, J
- Serrano, A
- Shepard, K.
- Smith, S
- Spencer, M
- Sperry, E.
- Tan, T
- Taylor, K
- Torres, K
- Torres-Hayes, C.
- Tsang, E
- Vaughn, A
- Vargas, L
- Pritchard, L
- Calendar
- Home
- Student Links
- Parent Connect
- First in Math Player of the Day
- Perfect Attendance/Asistencia Perfecta
- Math Curriculm/Curiculo de matematicas
5 Math Games Every Classroom Needs to Play
Guest post by Leigh Langton
Hey guys! It’s Leigh from The Applicious Teacher! I am super excited to be blogging at Corkboard Connections today.
I’m sharing a practice that I use to help increase my students’ engagement and number sense during my math block.
Do you play games in your classroom? Wait… what?! No time? Well… you should make time! Especially during your math time. To me, math and games go together like Nutella and pretzels. Delicious separate, but amazing together.
As a third grade teacher, I know how limited our time can be, so I am here to share with you 5 math games you should take the time to play this year! All of these games are fun, easy, and require little to no prep. They are math games that I’ve played for years with my second graders. When I moved up to third, I was able to easily modify these games for my new “big kids”.
First up… 100’s Game
This game can be played in a k-5 classroom. It is perfect for building number sense and it’s only prerequisite is that students can count. There’s no supplies needed to play and my kids loved playing this as a “brain-break” before math.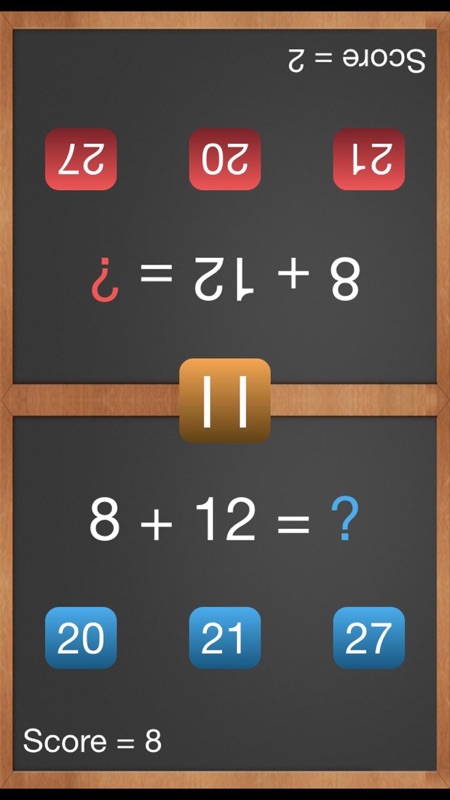
Here’s how to play…
Have your class stand in a circle. Moving in a clockwise direction, have the students count out loud until they get to a hundred. The person who says, “100” sits down. The last person standing, WINS!
The idea is simple, but can be modified for your students. In second grade we’d count by 5’s,10’s, and 25’s (to help with money later on in the year). For third, we count the multiples of numbers. For numbers that don’t have a multiple of 100, I choose the last number in the sequence of 12 as the “end number.”
Other Variations
Students sit down on a certain multiples (like the multiples of 7) Students don’t say the multiple. Students can count by ones to a hundred, but all the multiples of say, 4, are “off limits.” If a student says them, they sit down. You could also change it to student don’t say the divisors (perfect for those 4th/5th graders who need more practice with their facts!)
101 and Out…
This paper and pencil game works well in second to fifth grade classrooms and can be played by teams of students (like boys against girls) or in pairs.
To play you will need a sheet of paper, a pencil, and one dice. The object of the game is to score as close to 101 without going over or “out.”
To play, students take turns rolling the dice. As they roll, they can either take the number as a one or a ten. For example, if a student rolls a 5, they could take it as a 5 or a 50. Students keep a running record of their total as they play.
I love how the kids start to form a strategy for what numbers they want to roll next. It’s a great way to build mental math strategies. To introduce this game, I usually play it as, “The Teacher vs. The Class”. This allows time for modeling while keeping the kids in on the action. What class doesn’t love beating the teacher? They always want to play again if I win the round.
This game works best in longer stretches, so multiple rounds can be played. I usually like to use it at the beginning of the year as a class game before math centers. It then becomes an easy and fun game for the kiddos to play during math centers.
Back 2 Back
Seriously, hands down, my class’ favorite game to play! This game is perfect for inside recess as the whole class can play at once and everyone is excited for the game.
This game requires some “brain sweat”, so it works well for grades 2-5. There are two different versions of this game. Supplies needed are minimal: a writing surface, writing utensils, and someone who is quick with their math facts for a “caller.”
The object of the game is to guess the other player’s number before they guess yours. To play, two students come up to the board and stand back to back (hence the name). This allows for the students to write on the board, but blocks their view of the other person’s number.
The “Caller” states, “Numbers Up”. This signals the two students write a number of their choice on the board. I usually play with numbers 2-9 to keep kiddos from dwelling in the 0’s and 1’s easy train, but you can play with numbers as high or as low as needed for your group of kids.
The caller then states the sum (for younger students) or product (3rd-5th) of the two numbers. The students use their understanding of math facts to figure out what they other person’s number is when added or multiplied by their number. The first player to say the other person’s number wins the round. The “loser” gets to choose the next person to come to the board.
Please be warned… this game can get a little rowdy as students win and lose rounds and somehow the teacher always gets pulled up to “clear out” a player who’s been up a little too long… But it’s a lot of fun and well worth the 10-20 minutes! Beats the repetitious practice drills of flashcards!
Guess My Number
This next game is very versatile and can be modified in so many ways! It can be played in kindergarten all the way through 5th grade classrooms. To play, you need a number chart and a dry erase marker. This game can be played whole group, in pairs or in small groups of 3-4.
To begin, one student chooses a number.
The other players try to guess the number by asking a series of questions. The student crosses off numbers it can’t be and circles numbers it could. The person who guesses the right number, wins and gets to choose the next number.
The best part of this game is that it can be played with laminated personal hundreds charts in small groups.
It can also be played as a whole group game using a large chart.
For third grade, I encourage the use of question clues like “Is it a multiple of 5? Or greater than 70?” To introduce the game, I usually model crossing out numbers as students ask questions about the numbers and help link the clues to finding the right number.
For a kindergarten or first grade classroom, you may want to play with a number line with numbers 1-20. Then, students could ask if the number is bigger or smaller than numbers within that range. A 4th or 5th grade classroom can beef up the game with question clues like, “Is it divisible by 3?” or “Is it a multiple of 5?” The possibilities are endless! Time range to play can be from 5 minutes to 20 minutes and can be used as an inside recess game or a quick brain break before or after a lesson.
Math Fact Top It!
This last game works well in 1st through 5th grade classrooms and is best played in groups of 2-4 students. All that is needed to play are math fact flash cards. You can use addition, subtraction, multiplication or division cards. It just depends on where your students are in their math skills. I like to think of this game as “War for the classroom,” as the rules for the traditional card game apply to this math fact version.
To play, students divide the flash cards evenly among all players. Then, on the count of three, all students throw down a card. The card with the highest sum or product wins all the cards in play. This can be modified to lowest difference or quotient. If students have the same answer, then they play each other again, with the winner capturing all the cards in play. Students play until all the cards are won. The student depending on the flashcards you are using. with the most cards at the end wins. I find this game works best in math centers and is an easy way for students to practice their math facts in a new and unique way!
Download Freebie with Game Directions
So go forth and play! Get your students engaged and learning in the new year! If you’re not sure you’ll remember all these games I shared today, I’ve compiled all the directions in one file for you.
It’s available here at my TpT store!
Leigh is a wife, mother, and a second-grade- turned-third-grade teacher. She currently resides in Central Florida where she has been teaching for 7 years. When Leigh isn’t teaching or writing for her teacher blog, The Applicious Teacher, she enjoys snuggling up with a good book, running a few miles, or spending time with her family.
Start playing to love math
Sections: Mathematics, Extracurricular activities, Competition "Teaching with passion, or How to fall in love with mathematics?"
Many people often ask themselves why mathematics is needed? Often the very fact that this subject is included in the compulsory curriculum of schools, puts people at a loss. This bewilderment is expressed in the following: “Why do I, whose future profession will not be connected with making calculations and applying mathematical methods, need sines, cosines, functions?” All difficult topics raise similar questions.
A large number of people do not see any sense for themselves in the development of this science. “And my child is a humanist!” - like to add parents. Part of the problem is that no one in society has even a rough idea of what mathematicians do. The general understanding seems to be that mathematics is somehow related to the natural sciences: mathematicians help scientists with their formulas, or calculate huge numbers on computers for this or that scientific problem. Many may say that mathematics is a difficult science. But this is absolutely not true. It's just that mathematics requires a child to practice regularly. Math is interesting when there is creativity in it and the laws are clear and easy to use. Continuous cramming can discourage a student from studying mathematics, even if the child has a predisposition. This approach has been out of date for a long time.
Children, especially those aged 5-9, do not need to be taught the importance of learning math. It is much more important to give the child the opportunity to plunge into the world of entertaining interactive mathematics.
Studying mathematics and solving problems, the child learns to:
- generalize and highlight important things;
- analyze and systematize;
- find patterns and establish cause-and-effect relationships;
- reason and draw conclusions;
- think logically, strategically and abstractly.
Just as regular sports training "pumps" the body, makes it healthy, strong and resilient, so regular math exercises "pump" the brain - develop intellect and cognitive abilities, broaden one's horizons.
When you are doing interesting and useful things, mathematics often comes to you by itself. But no longer as a painful end in itself, but as a useful means to achieve your real goals .
Now many parents do not understand the role of the regime and rules in school life, the child's day is often not built in a certain order, household rituals are not always practiced. The redundancy of activities and entertainment, endlessly replacing each other, leads to the fact that they do not become events, are not deposited in the memory of the child.
But, these are the most important factors in the formation of the consciousness of the student. Everyday life, with its regimen and rules, streamlines the behavior and consciousness of the child, rituality rooted him in his own life, the repetition and predictability of events give a sense of security and understanding of what is happening to him.
Meanwhile, the leading activity of children of primary school age is child's play. In the game, the formation of the external sociality of the child and his self-development takes place. Mental neoplasms develop, which are necessary for the entire future life of the child, including imagination and fantasy. Any learning is associated with the need to represent something, imagine, operate with abstract images and concepts. All this is impossible to do without imagination and fantasy.
Increasing the mental load in mathematics lessons makes us think about how to maintain interest in the material being studied, their activity throughout the lesson.
Didactic games get along very well with "serious teaching". The inclusion of didactic games and gaming moments in the lesson makes the learning process interesting and entertaining, creates a good mood for students, and makes it easier to overcome difficulties in mastering educational material. It cannot be assumed that the use of game situations in the lesson makes it possible to master mathematics "easily and happily." There are no easy paths to science. A didactic game is not an end in itself in the lesson, but a means of education and upbringing. Play should not be confused with fun, should not be seen as an activity that gives pleasure for the sake of pleasure, but students do not need to know this. Who said that it is impossible to devote the last five minutes of the lesson to the game "Battleship", to hold a mini championship, and after a while to introduce the children to the Cartesian coordinate system. Before scaring the guys with such words as the abscissa and the ordinate, it is better to rely on what they already know, namely the geographical map.
With a degree network - a complex of parallels and meridians on globes and geographical maps, which serves to determine the geographical coordinates (latitude and longitude) of points on the earth's surface, students in geography lessons get acquainted earlier than with a system of mathematical coordinates. Therefore, you can safely take the card into service. Interdisciplinary connections increase the level of scientific education and its role in shaping the scientific worldview of students. Students have already worked with the map in math classes, when they studied the scale, a special case of direct proportionality, and learned how to solve more complex problems. And not just translate the numerical scale into a named one, using the rule: 1:25,000,000 - 1 cm - 250 km; 1:10 000 000 - 1 cm - 100 km; 1:20 000 - 1 cm-200 m and, if necessary, return the translation, add the same zeros, when converting kilometers to centimeters, add 5 zeros, meters to centimeters - 2 zeros. You can also tell the children about such mathematicians as Edward Wright, Dirk Rembrandts van Nierop and remember the octagonal Butterfly Map, which was invented by Bernard Joseph Stanislav Cahill.
Didactic play should be viewed as a form of transformative creative activity in close connection with other types of educational work.
Didactic games can be widely used as a means of education, upbringing and development. The main learning action belongs to the didactic material, game actions that, as it were, automatically lead the learning process, directing the activity of children in the right direction. The didactic game should be distinguished from the game in general and the game form of classes, although this division is conditional.
Here are some forgotten games that will help diversify your math lessons.
GAMES ON PAPER
Capturing territory. To play, you need 2 dice, a piece of squared paper and a pen or pencil. Roll 2 dice. Build a rectangle with sides whose lengths are equal to the rolled dice (a 2 * 3 cell rectangle is when 2 and 3 are rolled). Write down the area inside the rectangle.
The rectangle must be attached to your existing territory, that is, there must be a common line of at least one cell with already existing rectangles. The player's first rectangle is placed in the lower right corner, the opponent's rectangle is placed in the opposite one. If you cannot draw a new rectangle according to the rolled dice, then you miss a turn. The game is considered completed when all spaces are filled. The winner is the one with the largest territory, you can find out by simply summing the numbers inside your rectangles.
Naval battle. This game is for two players. Its goal is to sink all enemy ships. Ships are located on two square fields measuring 10 by 10 cells. Rows are denoted by numbers, columns by letters. You place ships on your field, and the enemy strikes at them. And on the other field, the enemy has his ships, which you "shoot" at. Each player has an equal number of ships - 10 pieces: one-deck (1x1 cell size) - 4 pieces; two-deck (1x2 cells) - 3 pcs; three-deck (1x3 cells) - 2 pcs; four-deck (1x4 cells) - 1 pc.
When arranging ships, keep in mind that there must be at least one empty cell between them, you can’t put ships close to each other.
Experienced players have their own strategy for placing ships. On his turn, the player selects a cell on the opponent's field and "shoots", naming its coordinates: "A1", for example. At the same time, he marks this move on his additional field.
If you sank the enemy ship, then the opponent must say “killed”, if you wounded the ship (that is, you hit a ship with more than one deck), then the opponent must say “wounded”. If you hit the enemy ship, you continue to "shoot". The game ends when all ships of one of the players are "sunk".
Points and lines. The conditions of this game on paper are simple: several dots are placed on the sheet (at least 8, and preferably at least 15). Two players play by alternately connecting any two points with segments. You cannot capture the third point. Each point can be the end of only one segment.
The segments must not intersect. The one who cannot make a move loses.
Barriers. A simple tactical game, the essence of which is the positional struggle for space. On an 8x8 field (i.e. the size of a chessboard), players draw small lines one after another that overlap any 2 cells in a row: i.e. for example, player 1 draws a vertical line. Player 2 does the same, but his line cannot intersect or touch existing "obstacles". As the field fills up, there is less and less free space, and at the end a sober calculation is needed to complete the game. A player who can no longer place his trait because everything is already blocked, losing.
Dots. Simplified paper version of the famous Japanese game "Go". The playing field is a regular sheet of paper in a box. Each player must have a pen or pencil of their own color. Players take turns putting dots in random places at the intersection of cells. The goal of the game is to capture as many paper possessions as possible.
A territory is considered captured if it is surrounded by dots of its color. The dots must be spaced one cell apart horizontally, vertically, or diagonally. The captured territory is painted over with its own color or a fortress wall is drawn around it (thick line). If you managed to encircle the territory or points of the enemy with dots, they are yours. After such a capture, the player is granted the right of an extraordinary move.
In some versions of the game, you can only capture territories where there are already enemy fortifications. In others, any, including free, lands are available to you. Choose what you like best. At the end of the game, the size of the occupied lands is calculated and the winner is declared.
Snake. On a sheet of paper, draw a square playing field 7x7 cells in size. Two adjacent sides of the square must be of the same color (for example, red), the remaining two - of another (for example, blue). On the playing field, put the red and blue dots in random places.
Now the players make moves in turn, starting to draw broken lines - snakes from the point of "their" color with "their" pencil. In one move, the line is extended by one cell in any direction (but not diagonally).
The lines must not intersect, they can be drawn along the side of the playing field, but these should not be sides of "their" color. The one who has nowhere else to extend his snake loses.
Headbands. A simple and rather fun game, built on the same principles as the parade of coins, but completely different in form. On a small field (it can be a square or a rectangle of arbitrary size, it's not particularly important), players place about 15-20 points in various places, although more or less evenly.
Then the first player draws a rounded but free-form rim that goes through at least 1 point. The maximum in the classic version is unlimited, although I would recommend giving a maximum of 4 dots per border.
The next player draws his own bezel, the only constraint is ? it cannot intersect with already drawn ones. The rims can be drawn inside the rims, or, conversely, surround existing ones, the main thing is that they do not intersect. After a while, there is very little space left, and the one who draws the last rim loses.
A variation of this game is the rule to draw circles covering only 1 or 2 points, no more.
Dots and squares. Martin Gardner, a well-known scientific researcher, author of books of entertaining mathematics, called this children's game a pearl in a huge number of logic games. It can be found under the name "Chests" and under the name "Embroidery".
The playing field consists of four rows with four dots. Players must connect horizontally or vertically adjacent points with lines. Moves should be done in sequence. If a player closes one or two squares with his move, then he continues to make his moves until he makes a move that does not close the square or until the end of the game.
When all the segments have been completed, the squares closed by each player are counted, and the player who has completed more squares is considered the winner. In total, nine squares can be closed. The field for the game is usually drawn either 5x5 or 7x7 squares, but even if the field is only 3x3, then the game is quite complicated. As in chess, in this game you can use combinational attacks, let the beginner close a few squares, so that he then makes an ill-conceived move and loses. The result of the combination is a gain in speed.
Sim . This game was invented in 1969 by the American graph theorist Gustav Simmons. Hence its name. The rules of the sim game are very simple. Several dots (at least five) are placed around the circle on a sheet of paper. Two players in turn, each with their own color, draw segments connecting any two previously unconnected points.
The player who is the first to be forced to draw a triangle of his color loses (with vertices at spaced points, of course; triangles that are randomly obtained due to the intersection of segments do not count).
Students involved in gaming activities learn and memorize new things, navigate in new and non-standard situations for them, develop creativity and originality, not noticing that they are performing educational activities. Even those students who are not active in the standard lesson get involved in the game with great enthusiasm and make every possible effort. Actions in the form of a game contribute to the activation of learning activities, students in the form of a manifestation of the individual abilities of students, by applying their knowledge, skills and abilities to achieve the results of the game.
Quiet games are a good means of transition from one mental activity to another. In mathematics lessons, using gaming technologies, students in a non-trivial form are drawn into the educational process and perform the necessary tasks, using all their arithmetic skills, also perform tasks of a comparative and analytical nature, apply the skill of setting and solving problems.
The game causes interest in learning and, of course, the desire to win, and for this you need to be creative, quick in finding the necessary information, as well as be able to complete tasks and clearly follow the rules of the game. Consequently, the cognitive activity of students is noticeably activated in the lesson where the game activity was used. In addition, such lessons create a positive working atmosphere, thereby activating the creative abilities of students, creating a situation of success, which in turn increases students' interest in the topic being studied.
Literature
- Vasiliev V.G. etc. Mathematical competitions. - M.: Nauka, 1994.
- Kovalenko N.G. Didactic games in mathematics lessons. - M .: Education, 1996.
- Kordemsky B.A. Engage students in mathematics. - M.: Education, 1991.
- Minkin E.M. From game to knowledge. - M .: Education, 1982.
- Perelman E.A. Live mathematics. - M.: AST: Astrel, 2008.
- Spivakovskaya A.S. The game is serious. - M .: Pedagogy, 1991.
List of sources used
- https://kubirubi.livejournal.com/135632.html
- https://www.belykrolik.ru/articles/igry_na_paper/
Mathematics for children grade 1.2
Description
Math can be fun! Fun Math is a funny and interesting game that helps to practice problem solving skills (addition, subtraction, multiplication, division) for students in grades 1, 2, 3 and 4.
One of the most important skills in elementary school mathematics is mental arithmetic (solving mathematical problems in your head). Mastering this skill takes a lot of time, effort and daily practice. Our game is designed to make this process more interesting and fun for kids.
In our game you can choose examples and arithmetic operations that you want to practice. However, we have options that are suitable for children of all ages:
• Preschoolers: addition and subtraction up to 10
• Grade 1: addition and subtraction up to 20
• Grade 2: addition and subtraction of two-digit numbers, multiplication table
• Grade 3: multiplication and division, addition and subtraction up to 100
• Grade 4: practicing skills of all arithmetic operations within 100 and 1000
In the game, you can choose the examples and arithmetic operations that you want to practice, as well as adjust the speed of the monsters and the way you enter the answer (select a number or enter it yourself).
A variety of levels, monsters, new weapons when defeating the Boss, the ability to buy clothes for the hero will not let the child get bored and will motivate him to make progress in learning.
The game is great for practicing problem solving for grades 1, 2, 3 and 4 of elementary school.
We would love to hear from you. If you have any questions or comments please email us at: [email protected]
Oct 14
Version 8.5.1
Minor fixes
Ratings and reviews
Ratings: 1.1k
Good graphics, interesting tasks
Great game. The child develops by playing.
👍🏻
Thank you for your feedback!😇
We are very glad that you and your child appreciated our approach to learning mathematics!
We will definitely pass on your feedback to our team!😊
Very cool game!
Thanks to this game, my son began to count well! Thanks a lot!!
Thank you for your feedback!🥰
We are very happy that you and your son are enjoying our app!😃
Excellent
You are the best
Thank you for your kind words! We are very pleased!🥰
We are very glad that you enjoy our app!😊
In-App Purchases
Mathematics (Full version)
Unlock all levels and features
449.
00 RUB
The developer of SpeedyMind LLC has indicated that, in accordance with the application's privacy policy, data may be processed as described below. Detailed information is available in the developer's privacy policy.
Related with user data
The following data may be collected that is related to the user's identity:
- Usage data
- Diagnostics
Sensitive data may be used differently depending on your age, features involved, or other factors. Read more
Information
- Provider
- Speedy Mind LLC
- Size
- 180.
Learn more
