How to learn counting

25 ideas to help children learn to count - Tips and tricks

1. Colourful abacus
   Purchase an abacus with large, colourful beads. Line all the beads up on the left side and show children how they can slide one bead at a time towards the right. Count the beads with them. Little ones will quickly grasp the concept. Later, you can invite children to slide one red bead, two blue beads, or three yellow beads and so on. Not only will children learn how to count with this activity, they will also work on their fine motor skills and practice color recognition.
2. Magnetic numbers
   At first, use several "1's", "2's", and "3's". Spread them out in no particular order on a table or the floor. Have fun arranging them in the correct order (1, 2, 3) on a cookie sheet or magnetic board while naming the numbers. Once children master these three numbers, you may choose to add one number at a time to increase the level of difficulty.
3. Bouncing balls
   Give each child an attractive ball or, if children are very young, manipulate a single ball yourself. The goal is to bounce the ball on the floor and count each bounce. This may represent quite a challenge for young children. This is a good thing; they will need to practice counting the first few numbers several times.
4. A tower to build, blocks to count
   Deposit a bin filled with Lego or Duplo blocks on the floor and invite each child to take "1" block. Next, encourage them to take a second block and place it on top of the first one. Count the number of blocks each child's tower contains. Continue this activity until each child's tower contains ten or more blocks, depending on their age. Once the maximum number of blocks is reached, let children take their tower apart, one block at a time, counting them as they work. With very young children, use plastic cubes and simply help them stack the cubes while counting them together. Keep going until their tower falls down. They will surely want to start over again and again!
5. Drinking straw pieces
   Purchase several large, colourful drinking straws (for thick smoothies). Have fun counting them with your group. If you have a baby bottle drying rack containing several branches, encourage children to slide the straws over them, counting them as they work. After a while, cut the drinking straws in two and repeat this exercise. You may continue to cut the drinking straws into smaller and smaller pieces. Children will have more to count!
6. Cut and re-cut cheese
   For each child, deposit a slice of cheese in a plate. Using a knife, draw a grid-like design on each child's slice of cheese, but let children separate the tiny cheese cubes independently. Count each piece with them as they put them in their mouth.
7. Adhesive numbers
   Purchase a large quantity of adhesive numbers. Hang large pieces of cardboard on a wall or set them on the floor, in a row. Using a marker, write a large number "1" on one piece of cardboard, a large number "2" on a second piece of cardboard, a large number "3" on another large piece of cardboard and so on. Invite children to associate the adhesive numbers to the corresponding board. Encourage them to name the numbers as they stick them on the correct boards. At the end of the activity, count with your group, pointing to the decorated pieces of cardboard as you go along.
8. One line, two lines, many lines!
   Purchase scented markers, bright highlighters, or any other type of marker or crayon you know the children in your group will be eager to manipulate. Invite children to use them to draw vertical lines at the bottom of a piece of paper. Of course, they must count the lines one by one. Next, depending on the ages of the children in your group, you may ask them use scissors to cut along the lines while counting them once more. Young children who are beginning to use safety scissors will particularly enjoy this activity that will help them develop both their fine motor and counting skills.
9. Counting coins
   Purchase a cute piggybank that is sure to attract children's attention as well as a large quantity of colourful coins. Make sure the coins can easily be inserted in the piggybank's opening. Let children take turns depositing one, two, or three coins in the piggybank while counting them. Once the piggybank is full, shake it until it is empty and start all over again! Children adore this very simple activity. You may choose to use several different piggybanks or even make your own. Simply decorate a metal coffee can and cut a slit in the lid. If you decide to make your own piggybank, decorate it with adhesive numbers!
10. One for you, one for me
    At snack time, help children find a partner. Give each team a bowl filled with a variety of berries. Encourage them to divide the berries among themselves, counting them as they deposit them in their individual plates. Help children if necessary. If the children in your group are very young, invite them to pick three berries each at a time so they only have to count up to three.
11. Planting flowers
    Purchase pretty fabric or plastic flowers at the dollar store as well as one or several oasis blocks (or simply use modeling dough). Let children prick the flower stems in the foam, counting each new addition. They will love repeating this activity over and over again and will therefore have several opportunities to practice counting. It's up to you to choose how many flowers you let them use for each "bouquet". You may also choose to count the flowers for the children in your group. Hearing you say the numbers in the correct order will help them remember them.
12. Birthday cake candles
    Cut a huge birthday cake shape out of white cardboard and display it on a wall. Cut narrow rectangles out of colourful paper to represent candles. Use adhesive putty to add one candle at a time. Count the candles as you add them.
13. Two, three, and four-legged friends
    You will need your animal figurine bin for this activity. Invite children to choose one animal figurine at a time and count how many paws/legs it has. This is a great exercise for learning to count from 1 to 4. Of course, some animals (snakes, fish, etc. ) have zero legs. Young children may not be familiar with this number (0)...after all, we always start at "1" when we teach children to count.
14. Hopscotch
    Have fun drawing unusual hopscotch grids. For example, your hopscotch grids may contain only numbers 1 to 3 or, instead of drawing boxes, you may choose to draw flowers, suns, stars, etc. Of course, what is important here is that you find a way to use this simple game to explore numbers with your group.
15. Bright sticks
    Empty a bag of colourful Popsicle sticks on a table or on the floor. Deposit pieces of construction paper (same colors) nearby. Ask children to associate the Popsicle sticks to the paper of the matching color one by one while counting them. You may also use the colourful sticks to represent the numbers children are familiar with. Once you are satisfied with the result, glue the Popsicle sticks together to create colourful numbers that can be used to decorate your daycare and to practice counting with your group. Simply display them on a wall, in the correct order.
16. Dance steps
    Invent simple dance steps and teach them to your group. Show them how to count their steps, "1, 2, 3...1, 2, 3...".
17. Light show
    Children love flashlights. Purchase several colourful flashlights. Turn the lights off and have fun turning the flashlights on and off repeatedly. Count every time you turn the flashlights on with your group. You may also use the flashlights as spotlights to light up large numbers displayed on a wall. Name the numbers you see.
18. Countable paperclips
    Purchase several large, colourful paperclips or paperclips with pretty designs. You can even find paperclips that have fun shapes glued to their tip. Hold a large piece of heavy paper and encourage children to slide paperclips on it one at a time while counting them. Help them if necessary. To push this activity even further, you could use hot glue to stick foam numbers on your paperclips and ask children to slide them on your paper in numerical order.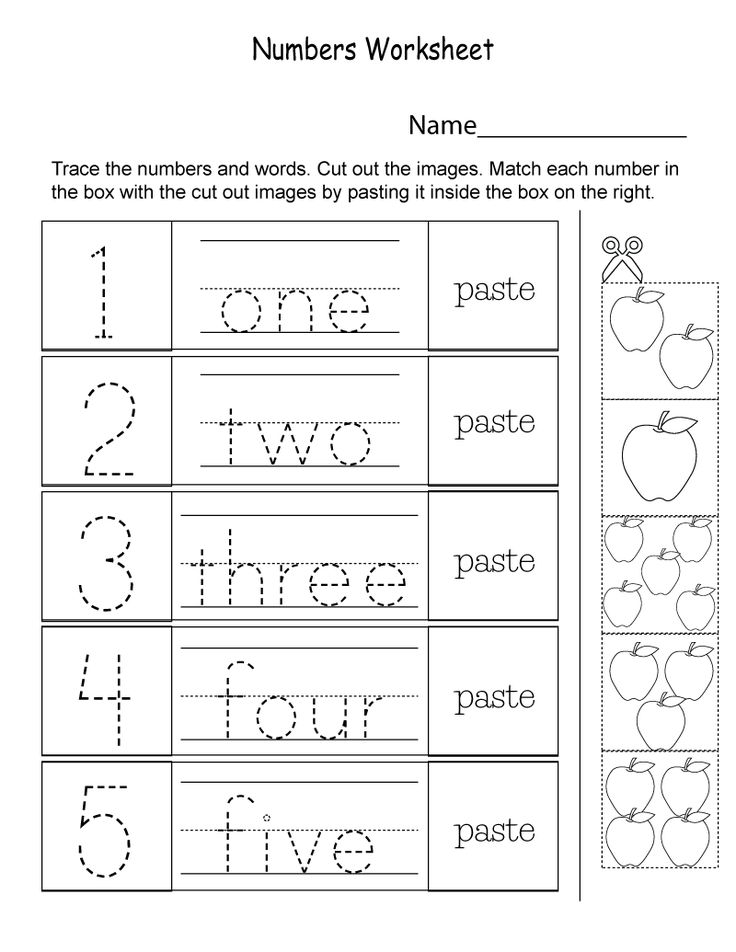
19. Race cars
    Purchase small toy cars and stick an adhesive number on each one. You will end up with car number 1, car number 2, car number 3, and so on. Invite children to roll the cars from a start line to a finish line in numerical order. Name the numbers appearing on each car with your group.
20. Animals in their pen
    Use wooden sticks (Popsicle sticks) to delimit small pens on the floor of your daycare. In each pen, draw a number (1 to 5). Ask children to place the corresponding number of animals in each pen. Count the animals together.
21. Let's punch holes!
    Young children are fascinated with hole-punches. Purchase hole-punches that will make it possible for children to create a variety of tiny shapes. Hold a piece of construction paper in your hands and invite children to use the hole-punches to make holes in your paper. Count the tiny shapes as they fall or, if they are collected in a small reservoir under the hole-punch, empty it from time to time to count the miniature shapes.
22. Foam numbers
    Glue foam numbers to the tips of long wooden skewers. Encourage children to prick them in an oasis block in numerical order. Count them together.
23. Circle stamping
    Let children press wine corks in paint and then on a large piece of paper. Encourage them to count the circles as they appear on the paper. Offer a different color of paint and repeat the activity as long as children are having fun to give them several opportunities to count.
24. Let's play store
    Set up a store area within your daycare. Collect a variety of empty food containers and write fictional prices on each one ($1, $2, or $3). Give each child several plastic coins and invite them to shop in your store. They will have to count the coins required to pay for each item they choose. Prepare for repeat customers!
25. Toothpick numbers
    At the dollar store, I found thick, colourful toothpicks, but regular toothpicks can work too. Draw large numbers on colourful construction paper. Children will enjoy exploring the numbers in a variety of ways with the toothpicks. You can, for example, ask them to deposit the correct number of toothpicks on each number or have them deposit toothpicks directly on the number outlines. This will help children learn to recognize the different numbers.

Patricia-Ann Morrison

Previous articles

ALL ARTICLES

Recommended products

PRINTABLE DOCUMENTS

LAMINATION

WIKKI STIX

MAGIC NUUDLES

How Children Learn to Count

• Education
• Maths Tips
• 5 and under

Counting is easily taken for granted but there's a lot of fascinating research into how we learn to count - and there's more to it than you may think.

The mathematical brain

It’s first worth considering where our capacity to do mathematics comes from.

Neuropsychologist Brian Butterworth in his book “The Mathematical Brain” suggests we’re born with an innate sense of number hard-wired into our brain and he attributes this to a small region of the brain behind the left ear he calls "the number module". He compares this idea to colour – in the same way we perceive the “greenness” of a leaf we can also perceive the “twoness” or “threeness” of a group of objects.

Take counting. Like times tables and algebra, we tend to think it's something kids have to be taught. Wrong, says Butterworth - it's an instinct. Sure, we have to learn the names and symbols of numbers to develop that instinct, but, because the number module is hardwired into the brain, basic counting comes naturally.

Remote tribes can count even when they have no words for numbers. In maths as in language he believes, "kids start off with little starter kits" And their maths starter kit is the number module.

There are other theories too - such as maths being an extension of our spatial awareness – but there’s something nice in the idea of a “little maths starter kit”.  

A Word of warning - All this doesn't mean a child is predestined to be either good at maths or not. Far from it, we’re all born ready to learn maths – and it’s what happens in the first 10 years or so that sets us up.

Counting with toddlers

Research suggest that toddlers - even as young as 12 months - have a sense of how many there are in a set - up to around three objects. This comes from their innate sense of number.

Counting is learned when the toddler starts making the connection between this innate sense of "how many there are" and the language we use to count "one, two, buckle my shoe". This is the first stage in learning maths and it's the building block for many early concepts.

Should parents count with their toddlers? Absolutely, using a variety of real objects. And since counting and language are interlinked reading to your toddlers is equally, if not more, important.

Counting - early learning milestones

Here are some stages of learning to count that you may notice your child going through at ages 3 to 5:

• Recognising how many objects are in a small set without counting. So if you show your child four apples they won't have to count them to tell you there's four.
• Knowing the "number words" from one to ten and their order.
• Know the sequence regardless of which number they start on. So if you say "start counting at four" they will count "four, five . ." as opposed to always counting from one.
• Conservation of quantity - This is where children realise that the number of objects in a set stays the same unless any are added or removed. So if they count six cans of beans in a straight line, then you rearrange the beans ( in front of their eyes ) into say two stacks of three - they will realise there's still six without recounting.
• Counting non-visible objects - your child will realise they can count things they can't touch or even see - such as sounds, members of someone else's family, or even ideas.
• Cardinality, not to be confused with carnality - This is knowing that the last number counted is equal to the quantity of the set. If your child counts six oranges 1,2,3,4,5,6 and then you ask "how many oranges are there"? and they count them again then they haven't grasped "cardinality".

Counting on - as a step towards adding

Learning to add comes as an extension of counting. Here are some stages a child goes through to make this connection:

• Counting all - For 3 + 5, children will count "one, two, three" and then "one, two, three, four, five"  to establish the quantity of the sets to be added – for example, three fingers on one hand and five fingers on the other. The child will then count all the objects "one, two, three, four, five, six, seven, eight"
• Counting on from the first number - Some children come to realise that it is not necessary to count the first number to add. They can start with three, and then count on another five to get the solution. Using finger counting, the child will no longer count out the first set, but start with the word ‘Three’, and then use a hand to count on the second added: ‘Four, five, six, seven, eight’.
• Counting on from the larger number -  It's more efficient when the smaller of the two numbers is counted. The child now selects the biggest number to start with which is "five", and then counts on "six, seven, eight".
• The final stage isn't really counting - it's where learners know their number facts and skip the time-consuming counting altogether.

Number lines are great visual tools for making this connection between "counting on" and addition or subtraction - we use them in Komodo a lot. Here's an earlier blog article all about number lines.

Beyond basic counting

Counting is the first mathematical pattern learners encounter. From here they soon begin to count backwards which is a step towards subtraction and they'll also count in twos, fives and tens which are a foundation for multiplication.

The next big step is the idea of place value and counting to base 10. Learners often make this leap simply because it's an obvious and efficient way to count large numbers. In Komodo, we use practice examples like this to help learners make the connection to counting in tens and ones.

It's easy to forget that counting is a key concept in maths with many stages before it's mastered. There's certainly a lot more to it than one, two, three!

I'm Ged, Co-founder of Komodo, ex-maths teacher and dad. If you have any questions please get in touch.

About Komodo - Komodo is a fun and effective way to boost primary maths skills. Designed for 5 to 11 year olds to use in the home, Komodo uses a little and often approach to learning maths (15 minutes, three to five times per week) that fits into the busy routine. Komodo users develop fluency and confidence in maths - without keeping them at the screen for long.

Find out more about Komodo and how it helps thousands of children each year do better at maths - you can even try Komodo for free.

Related Posts

Mindset - the path to mastery

People who have a growth mindset believe that they always have the potential to learn and improve. They are more motivated to persevere with difficult tasks, to take risks and to learn from failure.

Top tips to de-stress homework time

Homework can be a trigger for some of the worst fights and arguments that we have with our children. Here are some solutions that can help reduce tensions and get homework done without arguments.

How to learn to quickly count in your mind? - Meduza

1

Why in the mind when you can on a calculator or in a column?

Minimum counting skills, a sense of numbers are the same element of human culture as literate writing and speech, knowledge of a foreign language, a basic understanding of art and the world around.

In addition, when you easily count without improvised means, you feel a completely different level of reality control - you know in advance how much change you will be given in the store or whether it is worth cramming seven into an elevator with a carrying capacity of 400 kilograms. nine0003

Think about the fact that the calculator and the actions in a column are a kind of magic. Most likely, you do not understand how it works, and you are forced to simply trust them. And when you have a good understanding of how mathematical operations work and can reproduce them "by hand", your sense of control (and self-confidence) gets a serious bonus.

And finally, mental counting develops your mental abilities: attention, memory, concentration, switching between several streams of thought, and can also serve as a means for meditation or distraction from sad thoughts. nine0003

2

But where can I get tasks for training? Think of examples for yourself?

Of course not. The network is full of mobile applications that will offer you the training of math skills for every taste.

When choosing, keep in mind that a good application, at least, must have sufficiently flexible difficulty settings and keep statistics of the tasks you have solved.

Try these apps for iOS and Android, or look for alternatives on the App Store and Google Play. nine0003

3

How exactly should you train?

There are only four basic mathematical operations - addition, subtraction, multiplication and division. Each action has its own characteristics, but they are not complex. You need to figure it out once, and then train for 5-10 minutes a day, and very soon you will feel that you think better. Most likely, in two or three months you will reach a fairly decent level that can be maintained by episodic training.

4

Where do you start?

Start with the easiest level, single digit addition, and work it up to perfection: 99% correct, 1-2 seconds for each answer. To solve the "with the transition through 10" examples, try using the following technique - "Reliance on the ten".

Let's say you need to add 8 and 7.

1) Ask yourself how much 8 is missing from 10 (that's 2).

2) Imagine 7 as the sum of 2 and some second piece (that's 5). nine0003

3) First add to 8 that part of the number 7, which was missing to 10, and then that second piece - you get 10 and 5, and this, of course, is 15.

5

How to add multi-digit numbers?

Here the most important principle is the addition of identical digits to each other. Having broken both numbers into "bit parts", start adding from the highest digits - thousands with thousands, hundreds with hundreds, tens with tens, ones with ones. If necessary, enlarge what happens and count everything together again. nine0003

For example, how do you add 456 and 789?

1) 456 consists of three bit parts - 400, 50 and 6.

789 is also divided into three bit parts - these are 700, 80 and 9.

2) Add hundreds with hundreds: 400 + 700 = 1100, tens with tens: 50 + 80 = 130, ones with units: 6 + 9 = 15.

10+5, that is, 1200+40+5 = 1245.

Amendment . When adding the digits, we mixed up the units and added 8 instead of 9 to 6. As a result, the sum also turned out to be incorrect - 1244 instead of 1245. We apologize for the mistake, and do not repeat it - carefully follow the numbers, especially in the mental account!

6

What about subtraction?

And here you need to start from the basic level - subtracting a single digit from the numbers of the first and second ten - and bring this skill to perfection. As with addition, problems usually arise with "going through 10" subtraction. And here a similar method of "reliance on a dozen" will help. nine0003

Let's say we need to subtract 8 from 12.

1) Let's ask ourselves how much we need to subtract from 12 to get 10 (that's 2).

2) We will subtract 8 from 12 in parts - first we subtract this 2, and then everything else. And the rest is how much? (that's 6).

3) After subtracting 2 from 12, we got 10, and we need to subtract 6 more, we get 4. Done!

7

What about multi-digit numbers? Is everything difficult with them?

Not particularly. It is only important not to confuse the technique of subtraction with the technique of addition. When adding, it was convenient for us to break each of the numbers into digit parts, and here we break only the number that we subtract. nine0003

So let's say we need to subtract 512−259.

1) The number 259, which we subtract, consists of three digit parts - 200, 50 and 9. We will subtract them in turn.

2) 512−200 - subtracting hundreds does not affect the tens and units of the number 512 in any way, it only affects hundreds, so the result will be this - 312.

3) From what happened after subtracting hundreds, now subtract tens, 312 -50.

This is similar to subtracting by ten. First subtract 10 from 312 to whole hundreds (units will not be affected), we get 302. And then subtract everything else (50 had to be subtracted in total, 10 has already been subtracted, it remains to subtract 40), we get 262.

4) It remains to subtract the units: 262−9.

Clean transition through ten - first subtract 2, we get 260, and then subtract the rest, 7, we get 260−7 = 253. Here is the answer.

8

How does multiplication work?

Let's start with single-digit multiplication. First you need to remember that multiplication is when you add the same thing several times. For example, multiplying 4 by 7 means adding four sevens. Using the addition technique, we can easily calculate - two sevens, 7 and 7, will be 14, if we add the third 7, we get 21, and adding the last, fourth seven, we get 28 as a result.0003

Gradually, as a result of training, you will remember the reference multiplication values ​​that are convenient for you and with their help you will be able to quickly calculate neighboring ones. For example, if you need to multiply 6 by 7 (that is, add six sevens), and you remember that 5 times 7 (that is, add five sevens) will be 35, then to get the final result, you just need to add the sixth seven - you get 42.

The most difficult example in the multiplication table is 7∙8. To remember it, there is a good mnemonic rule “five six seven eight”, which means 56 = 7∙8. nine0003

9

How to multiply a multi-digit number by a single-digit number?

Let's look at an example. Let's say we need to multiply 468 by 6.

1) 468 consists of 400, 60 and 8, and all this needs to be multiplied by 6. Well, taken separately, these problems are no more difficult than multiplying single-digit numbers.

2) Going from the most significant digit to the least significant: 400∙6 = 2400 (since 400 is 100 times greater than 4, then the result of 400∙6 will be 100 times greater than the result of 4∙6).

Accordingly, 60∙6 = 360, and 8∙6 = 48.

3) And now, as in addition, we add it all together, grouping the same digits: 400+300)+(60+40)+8 = [add the same digits] =

= 2000+700+100+8 = [group and add the same digits] =

= 2000+800+8 = [further enlarge nothing, we get the answer] = 2808.

10

How to multiply two-digit numbers?

For an ordinary person, this is already aerobatics! If you have mastered double-digit multiplication, consider that you have been accepted into the world of the mental counting elite. But in fact, there is nothing fundamentally complicated here, just a higher load on short-term memory (at the same time we will train it). nine0003

So, for example, let's multiply 78 by 56. This means that we need to add (take) the number 78 56 times.

1) These 56 times can be divided into stages - first add 78 50 times, then 6 times, and then combine the results.

2) Adding the number 78 50 times is not difficult - it is 10 times more than adding it 5 times. 78∙5 = 70∙5+8∙5 = 350+40 = 390. So, 78∙50 = 3900, remember this number.

3) Now let's calculate 78∙6 = 70∙6+8∙6 = 420+48 = 468.

4) Now let's add both results together: 3900+468 = 3000+900+400+60+8 = 3000+1300+60+8 = 4368. Voila!

Amendment . At the final stage, when adding 3900 and 468, we incorrectly split the second number into digits - we forgot about 60. As a result, the total turned out to be 4308. We apologize for the mistake, and do not repeat it - you cannot lose terms in the mental calculation.

11

Wow, the last thing left is action, division?

Yes, we are at the finish line. And again, let's start from the simplest level: dividing by a single-digit number of those numbers that are familiar to us from multiplying single-digit numbers. nine0003

So what is division? In fact, this is the "reverse" operation to multiplication.

For example, dividing 56 by 7 means picking up such a number that if you multiply it by 7, you get 56. Since you are already well versed in the multiplication table by this point, you will surely remember that exactly 8 times 7 gives 56. So, the desired number is 8, 56:7 = 8.

And so always - remember which number, when multiplied, gives the desired result - this is the number that you need. nine0003

12

How do you divide multi-digit numbers by single-digit numbers?

Let's divide 6144 by 8. Our way is to "cut off" the maximum "round" parts from the original number, each of which is guaranteed to be divisible by 8 according to the multiplication table.

1) Select from 6144 as large a part as possible, which is divisible by 8 according to the multiplication table. This will be 5600, because 56 is divisible by 8, and the next number that is divisible by 8 is already 64, which does not suit us, since 6400 is greater than 6144. Great, 6144 is 5600 and 544 (here we needed the subtraction skill ). nine0003

We will divide along the way:

6144:8 = [select the maximum convenient round part] =

= (5600+544):8 = [divide the selected part by 8, and work on the second part in the next step] =

= 700+544:8. We will remember

700 as a partial result, and we ourselves will deal with the division 544:8.

2) Similarly, out of 544, the largest part that can be conveniently divided by 8 according to the multiplication table is 480 (after all, 48 is divisible by 8, and the next number - 56 - does not suit us, because 560\u003e 544) . So 544 = 480+64. nine0003

We continue the division:

544:8 = [select the maximum convenient round part] =

= (480+64):8 = [divide the selected part by 8, and work on the second part in the next step] =

= 60 +64:8.

60 add to 700, 700+60 = 760 - remember this as the second part of the result and move on to the last division, 64:8.

3) The remaining piece, 64, is also divisible by 8 according to the multiplication table, 64:8 = 8.

Accordingly, the total result of division is 760+8=768. Everybody! nine0003

13

How do you divide by a two-digit number?

The technique of dividing by a two-digit number is the most diverse, unlike anything, exquisite. Let's get acquainted with it on the example of 5148:66.

1) Guess what ten is our result. Recall that 5148:66 means: we are looking for a number that, when multiplied by 66, will give 5148. We will use the "shooting" technique.

Just randomly try the number 20 as a possible candidate. 20∙66 = 1320, which is 4 times less than 5148, which we need. nine0003

4 times more than 20 is 80, let's try it. 80∙66 = 5280, it turned out more than the desired 5148, but a little, most likely, this is the “top” ten.

Let's check for reliability 70, the previous before 80 tens. 70∙66 = 4620, which is just less than 5148, great! This means that the number we are looking for lies between 70 and 80.

2) Let's use the mathematical law about the last digit of the result of multiplying two numbers.

It turns out that it always coincides with the last digit of the result of multiplying the last digits of these numbers (try to think why this is so). For example, what number will 123 9 end with0061 4 ∙567 8 ? To the same as 4 ∙ 8 , that is, to 2 (4∙8 = 3 2 ).

Therefore, if we are looking for a number that, when multiplied by 6 6 , will give 5148, then to guarantee this 8 in last place, the desired number can only end in either 3 or 8 (3∙ 6 = 18, 8 6 = 48).

3) With these endings between 70 and 80, we have two total candidates - 73 and 78.

5148 is clearly closer to 5280, so let's check 78 first.

78∙66 = 78∙60+78∙6 = 4680+468 = 5000+148 = 5148, hooray!

(Well, if the result did not converge, then we would check the second number, and it would already fit exactly).

14

What are some final recommendations?

Here, in general, and all the methods that are enough to know to train confident counting within 10,000 (and the ability to work mentally with large numbers, perhaps, is already beyond the scope of the necessary general development).

Surely you will also come across other techniques, the so-called. "tricks" of quick counting, but do not rush to get carried away with them. In addition, remember that regularity is more important than intensity - try to exercise on the simulator every day for 5-10 minutes, no more is needed, otherwise there is a high risk of "burning out" and giving up. nine0003

Don't rush anywhere in the process - catch your rhythm, focus on correct answers, not speed, speed will come later.

Be sure to try to pronounce your actions out loud, especially at first - you will have a chance to feel how everything is like poetry, and it will be easier to decide this way.

And don't be upset if something doesn't work out - the walker will master the road, and sooner or later you will definitely succeed.

How to learn to quickly count any numbers in your head: mental counting techniques

Mental arithmetic is an activity that fewer and fewer people are bothering with nowadays. It is much easier to get a calculator on your phone and calculate any example.

But is it really so? In this article, we will present math hacks that will help you learn how to quickly add, subtract, multiply and divide numbers in your mind. Moreover, operating not with units and tens, but with at least two-digit and three-digit numbers.

After mastering the methods in this article, the idea of ​​reaching for a calculator on your phone doesn't seem so good anymore. After all, you can not waste time and calculate everything in your mind much faster, but at the same time stretch your brains and impress others (of the opposite sex). nine0003

So, welcome to the exciting world of computing! We have collected tips from our authors on how to improve mental arithmetic and become a math hero and genius. By the way, if you are interested in mathematics, you can read the article "Limits for dummies" in our blog.

Warning! If you are an ordinary person and not a child prodigy, then it will take training and practice, concentration and patience to develop mental numeracy. At first, everything can turn out slowly, but then things will go smoothly, and you can quickly count any numbers in your head. nine0003

Gauss and mental counting

One of the mathematicians with a phenomenal speed of mental counting was the famous Carl Friedrich Gauss (1777-1855). Yes, yes, the same Gauss who came up with the normal distribution.

In his own words, he learned to count before he could speak. When Gauss was 3 years old, the boy looked at his father's payroll and declared, "The calculations are wrong." After the adults checked everything, it turned out that little Gauss was right. nine0003

In the future, this mathematician reached considerable heights, and his works are still actively used in theoretical and applied sciences. Until his death, Gauss did most of his calculations in his head.

Here we will not deal with complex calculations, but start with the simplest.

Adding numbers in your head

To learn how to add large numbers in your head, you need to be able to accurately add numbers up to 10 . Ultimately, any complex task comes down to performing a few trivial actions. nine0003

Most often, problems and errors occur when adding numbers with "going through 10 ". When adding (and even when subtracting), it is convenient to use the technique of “reliance on a dozen”. What's this? First, we mentally ask ourselves how much one of the terms is missing up to 10 , and then we add to 10 the difference remaining to the second term.

For example, add the numbers 8 and 6 . To get 8 from 8 , 2 is missing. Then to 10 it remains to add 4=6-2 . As a result, we get: 8+6=(8+2)+4=10+4=14

The main trick with adding large numbers is to break them into bit parts, and then add these parts together.

Let's add two numbers: 356 and 728 . The number 356 can be represented as 300+50+6 . Similarly, 728 would be 700+20+8 . Now add up:

356+728=(300+700)+(50+20)+(8+6)=1000+70+14=1084

come easy. But unlike addition, where each number is divided into bit parts, when subtracting, you only need to “break” the number that we subtract. nine0003

For example, how much is 528-321 ? We split the number 321 into bit parts and get: 321=300+20+1 .

Now we calculate: 528-300-20-1=228-20-1=208-1=207

Try to visualize the processes of addition and subtraction. At school, everyone was taught to count in a column, that is, from top to bottom. One way to restructure thinking and speed up counting is not to count from top to bottom, but from left to right, breaking numbers into place parts. nine0312

Mental multiplication

Multiplication is the repeated repetition of a number. If you need to multiply 8 by 4 , this means that the number 8 needs to be repeated 4 times.

8*4=8+8+8+8=32

Since all complex problems are reduced to simpler ones, you need to be able to multiply all single-digit numbers. There is a great tool for this - multiplication table . If you do not know this table by heart, then we strongly recommend that you first learn it and only then take up the practice of mental counting. In addition, there is, in fact, nothing to learn there.

Multiplication of multi-digit numbers by single digits

First, practice multiplying multi-digit numbers by single-digit numbers. Let's multiply 528 by 6 . Breaking down the number 528 into digits and go from oldest to youngest. We multiply first and then add the results.

528=500+20+8

528*6=500*6+20*6+8*6=3000+120+48=3168

4 9002, by the way! For our readers, there is now a 10% discount on any kind of work