Important math concepts

10 Most Important Math Topics

Table of Contents

Math can be a scoring subject by focusing on the most important math topics. The math topics are the same entailing equal importance irrespective of the grade of the student.

Focusing on the math topics that carry more weightage can help students to secure more marks, and also prepare a strong foundation for other STEM fields.

10 Most Important Math Topics

These math topics are considered significant academically and also lays down the foundation to understand other math concepts in after-school grades.

1. Algebra

Algebra is one of the most important math topics a student must master as the equations, and variables like x,y, and z are all the roots of math topics like integration and differentiation encountered by the student in higher grades and while preparing for competitive exams.

Hence, algebra is one of the essential topics that require students’ focus and attention.

2. Geometry

Geometry is one of the most important math topics a student cannot afford to skip. Shapes are all around us and geometry helps students to understand and calculate the dimensions, positions, and angles of different shapes.

Learning how to calculate the length, breadth, and width is essential for any student aiming to opt for a career in architecture, interior design and even measuring the size of your study table in day-to-day life.

3. Heron’s Formula

Focusing on the calculation of the area, and perimeter of triangles, academically Heron’s formula is one of the important math topics. Questions based on triangles, calculating the areas, and perimeter carry more weightage in the syllabus and assure more marks in exams.

4. Pygaoethores Theorem

A well-known theorem by students, the derivation of the formula and diagram carries marks in itself. Henceforth, students must focus on this theorem from class 5 as one of the important math topics in class 6 math.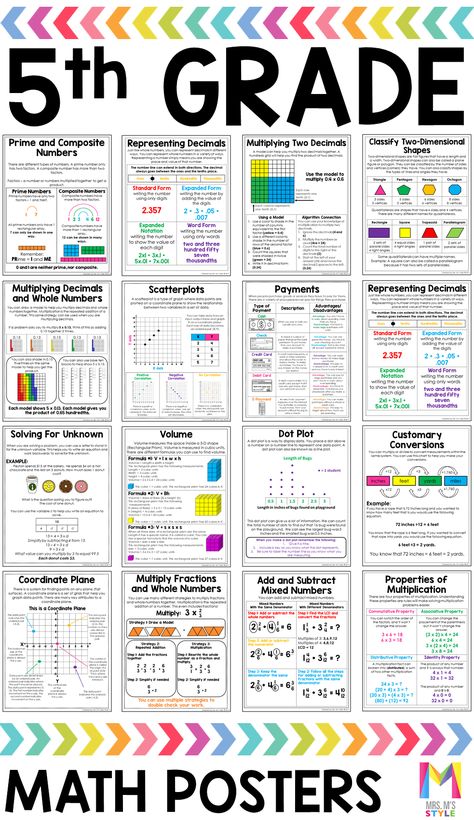

5. Trigonometry

One of the important math topics that study the relationship between the sides, and angles of the same or two different triangles.

6. Circles

Circles are one of the most important math topics in class 10 maths for students preparing for boards. As per the syllabus circles, the math topic entails a lot of weightage. Circles are one of the math topics that are formula-based and can help students to secure more marks in exams. 

7. Profit and Loss

Profit and loss are one of the most important math topics in life apart from academics. This math topic is essential for any student aiming for a career in entrepreneurship, finance, accounting, or in general calculating the gains and losses in assets.

8. Percentages

Percentages are one of the most prominent math topics as students compare their performance with their peers based on the percentage secured in exams. Also, percentages are considered one of the important math topics in the class 5 math syllabus.  

9. Statistics

Statistics is essential and important math topic in the class 9 math syllabus as per the CBSE board. This math topic is also used in Data Analytics and Quantitative Analysis.  

10. Probability Theory

Probability theory helps students to calculate the possibilities in cases like cards deck, heads, and tails in coins, probability of winning the match, and many more situations. This is considered one of the most important math topics in the class 9 math syllabus and for students preparing for other Competitive exams. 

Other Important Math Topics

Apart from the above-mentioned Math topics, there are other topics that are considered important for students from grade 1 math to grade 10 math and above.

• Number System
• Ratio Analysis
• Integration
• Quadratic Equations
• Mensuration

Conclusion

Foucing on the important math topics can help students to master the basics of math required for a better understanding of other math topics and additionally prepare them for other STEM fields in the future.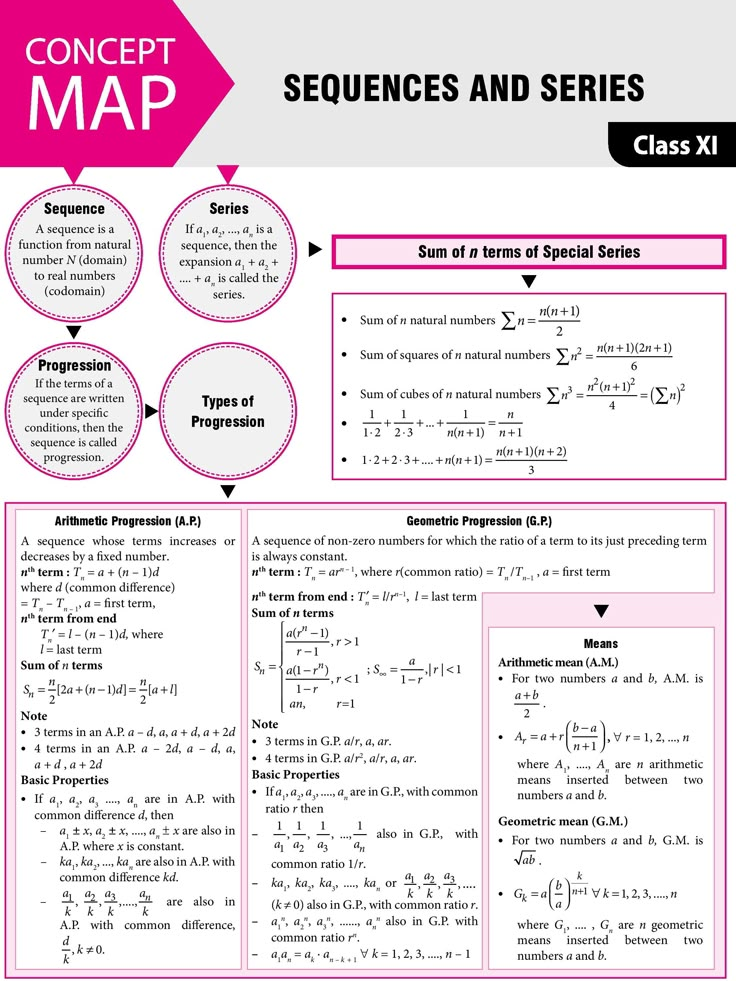

{igebra.ai} Live Online Math Classes make math learning fun and easy focusing on application-based math, and making students fall in love with math!

Learn Basic Math Online

10 Math Concepts You Can't Ignore

Sets and set theory

A set is a collection of objects. The objects, called elements of the set, can be tangible (shoes, bobcats, people, jellybeans, and so forth) or intangible (fictional characters, ideas, numbers, and the like). Sets are such a simple and flexible way of organizing the world that you can define all of math in terms of them.

Mathematicians first define sets very carefully to avoid weird problems — for example, a set can include another set, but it can't include itself. After the whole concept of a set is well-defined, sets are used to define numbers and operations, such as addition and subtraction, which is the starting point for the math you already know and love.

Prime numbers go forever

A prime number is any counting number that has exactly two divisors (numbers that divide into it evenly) — 1 and the number itself. Prime numbers go on forever — that is, the list is infinite — but here are the first ten: 2 3 5 7 11 13 17 19 23 29 . . .

It may seem like nothing, but . . .

Zero may look like a big nothing, but it's actually one of the greatest inventions of all time. Like all inventions, it didn't exist until someone thought of it. (The Greeks and Romans, who knew so much about math and logic, knew nothing about zero.)

The concept of zero as a number arose independently in several different places. In South America, the number system that the Mayans used included a symbol for zero. And the Hindu-Arabic system used throughout most of the world today developed from an earlier Arabic system that used zero as a placeholder. In fact, zero isn't really nothing — it's simply a way to express nothing mathematically. And that's really something.

Have a big piece of pi

Pi (π): The symbol π (pronounced pie) is a Greek letter that stands for the ratio of the circumference of a circle to its diameter. Here's the approximate value of π:

π ≈ 3.1415926535…

Although π is just a number — or, in algebraic terms, a constant — it's important for several reasons:

Geometry just wouldn't be the same without it. Circles are one of the most basic shapes in geometry, and you need π to measure the area and the circumference of a circle.

Pi is an irrational number, which means that no fraction that equals it exactly exists. Beyond this, π is a transcendental number, which means that it's never the value of x in a polynomial equation (the most basic type of algebraic equation).

Pi is everywhere in math. It shows up constantly (no pun intended) where you least expect it. One example is trigonometry, the study of triangles. Triangles obviously aren't circles, but trig uses circles to measure the size of angles, and you can't swing a compass without hitting π.

Equality in mathematics

The humble equals sign (=) is so common in math that it goes virtually unnoticed. But it represents the concept of equality — when one thing is mathematically the same as another — which is one of the most important math concepts ever created. A mathematical statement with an equals sign is an equation. The equals sign links two mathematical expressions that have the same value and provides a powerful way to connect expressions.

Bringing algebra and geometry together

Before the xy-graph (also called the Cartesian coordinate system) was invented, algebra and geometry were studied for centuries as two separate and unrelated areas of math. Algebra was exclusively the study of equations, and geometry was solely the study of figures on the plane or in space.

The graph, invented by French philosopher and mathematician René Descartes, brought algebra and geometry together, enabling you to draw solutions to equations that include the variables x and y as points, lines, circles, and other geometric shapes on a graph.

The function: a mathematical machine

A function is a mathematical machine that takes in one number (called the input) and gives back exactly one other number (called the output). It's kind of like a blender because what you get out of it depends on what you put into it. Suppose you invent a function called PlusOne that adds 1 to any number. So when you input the number 2, the number that gets outputted is 3:

PlusOne(2) = 3

Similarly, when you input the number 100, the number that gets outputted is 101:

PlusOne(100) = 101

It goes on, and on, and on . . .

The very word infinity commands great power. So does the symbol for infinity (∞). Infinity is the very quality of endlessness. And yet mathematicians have tamed infinity to a great extent. In his invention of calculus, Sir Isaac Newton introduced the concept of a limit, which allows you to calculate what happens to numbers as they get very large and approach infinity.

Putting it all on the line

This pattern continues forever, with each value being halved, which means you can never get to the other side of the room. Obviously, in the real world, you can and do walk across rooms all the time. But from the standpoint of math, Zeno's Paradox and other similar paradoxes remained unanswered for about 2,000 years.

The basic problem was this one: All the fractions listed in the preceding sequence are between 0 and 1 on the number line. And there are an infinite number of them. But how can you have an infinite number of numbers in a finite space? Mathematicians of the 19th century — Augustin Cauchy, Richard Dedekind, Karl Weierstrass, and Georg Cantor foremost among them — solved this paradox. The result was real analysis, the advanced mathematics of the real number line.

Numbers for your imagination

The imaginary numbers (numbers that include the value i = √ - 1) are a set of numbers not found on the real number line. If that idea sounds unbelievable — where else would they be? — don't worry: For thousands of years, mathematicians didn't believe in them, either. But real-world applications in electronics, particle physics, and many other areas of science have turned skeptics into believers.

So, if your summer plans include wiring your secret underground lab or building a flux capacitor for your time machine — or maybe just studying to get a degree in electrical engineering — you'll find that imaginary numbers are too useful to be ignored.

Basic mathematical concepts

The FEMP methodology in the system of pedagogical sciences is designed to help prepare preschool children for the perception and assimilation of mathematics - one of the most important subjects in school and the comprehensive development of the child.

The FEMP technique has a specific, purely mathematical terminology .

This is:

- set;

- number;

- counting and computing activities;

- value;

- geometric figures;

- time;

- space.

SET is a collection of objects that are considered as a whole . The world in which a person lives is represented by a variety of sets: a lot of stars in the sky, plants, animals around him, a lot of different sounds, parts of his own body.

Sets consist of elements. The elements of the set are the objects that make up the set. It could be real objects (things, toys, drawings), as well as sounds, movements, numbers, etc. .

The elements of a set can be not only individual objects, but also their combinations . For example, when counting in pairs, triplets, tens. In these cases, the elements of the set are not one object, but two, three, ten - a set.

Thus, the set is considered as a set, a set , a collection of any objects and objects, united by a common, for all characteristic property .

Any property can be considered as belonging to some objects.

Attention!

If you need help writing a paper, we recommend that you contact professionals. Over 70,000 authors are ready to help you right now. Free corrections and improvements. Find out the value of your work.

Calculation CostGuaranteesReviews

For example, has the property of being red for some flowers, berries, cars and other objects. The property of being round is possessed by the moon, the ball, the wheels of bicycles and cars, parts of various machines and machine tools, etc.

Thus, each property is associated with a set (objects) that have this property. It is also said that the set is characterized by the given property - or the set is given by specifying the characteristic property.

Under the characteristic property of a set is meant such a property, which all objects belonging to a given set (elements of this set) have, and not a single object that does not belong to it, i.e. this item is not its element.

If some set A is specified by specifying the characteristic property P , then this is written as follows:

A = { x | Р ( x )}

and reads like this: “ A is the set of all x such that x has the property P ”, or, in short, “A is the set of all 9003 having property R ". When they say: "the set of all objects that have the property P", they mean those and only those objects that have this property.

Thus, if the set A is given by the characteristic property P , this means that it consists of all objects that have this property, and only of them. If some a has the property P , then it belongs to the set A , and, conversely, if the object a belongs to the set A , then it has the property P .

An infinite set of objects can have some property, only a finite set can have another. Therefore, the sets are subdivided into end and end .

A finite set can be specified by direct enumeration of all its elements in an arbitrary order. For example, the set of children of a given group living on Sadovaya Street can be described using a characteristic property: { x | x - lives on Sadovaya Street) or listing all its elements in an arbitrary order: {Lena, Sasha, Vitya, Ira, Kolya}.

It is quite clear that an infinite set cannot be defined by listing all of its elements .

Mathematics mostly deals with infinite sets (numbers, points, figures and other objects), but basic mathematical ideas and logical structures can be modeled on finite sets.

Naturally, in pre-mathematical preparation usually deals with finite sets .

COUNT - first and main mathematical activity based on element-by-element comparison of finite sets.

NUMBER is the general invariant category of the set , which is an indicator of the cardinality of the set. This is just a sound designation.

Theoretical foundations for the formation of elementary mathematical representations in preschoolers include a detailed study of only the system of natural numbers . Therefore, when we say "numbers", we mean natural numbers.

NUMBERS - a system of characters (“letters”) for writing numbers (“words”) (numerical characters). The word “digit” without qualification usually means one of the following ten characters: 0 1 2 3 4 5 6 7 8 9 (so-called “Arabic numerals”). Combinations of these numbers generate two (or more) digit numbers.

The number has 2 values ​​: number and ordinal.

With a quantitative value of , we are interested in the number of elements in the set. We use the question HOW MUCH? and we start counting with the cardinal number ONE.

When the ordinal value is , we are interested in the place of the number among others or the ordinal number of the element in the set. The question is WHICH COUNTS? and set the direction of the account. Ordinal numbers are used, counting begins with the word FIRST.

When we talk about quantity, it does not matter the direction of the count, the object from which the count began. The final number does not change. With an ordinal count, the final number may change.

COUNTING is considered as an activity with specific elements of the set, in which a relationship is established between objects and numerals. The study of numerals and sets of objects leads to the assimilation of counting activities.

CALCULATION ACTIVITY - is an activity with abstract numbers, carried out through addition and subtraction. The simple naming of numerals will not be called counting activity. The system of computational actions is formed on the basis of quantitative knowledge.

VALUE is the quality and property of an object, with the help of which we compare objects with each other and establish a quantitative characteristic of the compared objects.

The concept , the value in mathematics is considered as the main one.

A direct answer to the question “what is a quantity?” no, since the general concept of magnitude is a direct generalization of more specific concepts: length, area, volume, mass, speed, etc.

The size of an object is its relative characteristic , emphasizing the length of individual parts and determining its place among homogeneous ones. The value is a property of an object perceived by various analyzers: visual, tactile and motor. In this case, the size of an object is most often perceived simultaneously by several analyzers: visual-motor, tactile-motor, etc.

Item size, i.e. item size , determined by only based on comparison . It is impossible to say whether it is a large or a small object, it can only be compared with another.

The perception of magnitude depends on the distance from which the object is perceived, and also on the magnitude of the object with which it is compared . The farther an object is from the one who perceives it, the smaller it seems, and vice versa, the closer it is, the larger it seems.

The characteristic of the size of the object also depends on its location in space . The same object can be characterized either as high (low), or as long (short). This depends on whether it is in a horizontal or vertical position. So, for example, in the figure, objects are located in a vertical position and are characterized as high and low, , and in another figure (in a horizontal position), these same objects are characterized as long and short.

The value of an item is always relative to , it is dependent on which item it is compared to . Comparing an object with a smaller one, we characterize it as a larger one, and comparing the same object with a larger one, we call it a smaller one.

So, the magnitude of a particular object is characterized by the following features : comparability, variability and relativity.

1) comparability carried out:

- overlay,

- application,

- measurement using a conditional measure,

- comparison by eye.

2) relativity - depends on the object with which we are comparing, on the distance at which we are comparing, on the location in space.

3) variability . Size is closely related to size. And size is a property of magnitude variability. Each item has its own generic purpose. It can change its dimensions without changing its essence.

GEOMETRIC FIGURE is an abstract concept with the help of which we personify all the objects around us in form.

A geometric figure is the presence of points on a plane, limited by space.

Figures are flat (circle, square, triangle, polygon…) and spatial (ball, cube, parallelepiped, cone...), which are also called geometric bodies.

GEOMETRIC BODY is a closed part of space, limited by flat and curved surfaces.

If the surface bounding the body consists of planes, then the body is called a polyhedron . These planes intersect along straight lines, which are called edges, and form the faces of the body. Each of the faces is a polygon whose sides are the edges of the polyhedron; the vertices of this polygon are called the vertices of the polyhedron.

Some polyhedra with a certain number of faces have special names : tetrahedron - tetrahedron, hexahedron - exahedron, octahedron - octahedron, dodecahedron - dodecahedron, twenty-sided - icosahedron.

What is a geometric FORM?

SHAPE is the outline, the outer appearance of the object.

Form (lat. forma - shape, appearance) – the mutual arrangement of the boundaries (contours) of the subject, object, as well as the relative position of the points of the line.

TIME is a philosophical concept that is characterized by the change of events and phenomena and the duration of their existence.

Time has properties :

- fluidity (time cannot be stopped)

- irreversibility and uniqueness

- duration .

SPACE is the quality by which relationships such as neighborhoods and distances are established.

Orientation in space implies orientation towards oneself, away from oneself, from other objects, orientation on the plane and orientation on the ground.

We will help you write any work for a similar one subject

• Abstract

  Basic mathematical concepts

  From 250 rubles

• Control job

  Basic mathematical concepts

  From 250 rubles

• Coursework

  Basic mathematical concepts

  From 700 rubles

Get a job done or expert advice on your educational project

Find out the cost

Glossary of terms in mathematics from A to Z - POCHEMUKHA.RU answers to questions.

Axiom is a statement accepted without proof.

Algebraic expression - a number of numbers, denoted by letters or numbers and connected using the operations of addition, subtraction, multiplication, division, raising to a power and extracting a root.

Abcissa (French word). One of the Cartesian coordinate points. Is the first. It is usually denoted by the symbol "X". First used by G. Leibniz in 1675 (German scientist).

Additivity. Some property of quantities. He speaks about the following: the value of a certain quantity corresponding to a full-fledged object is equal to the sum of the values ​​​​of such a quantity that correspond to its parts in any division of a full-fledged object into parts.

Adjunct. Fully corresponds to algebraic addition.

Axonometric. One of the ways to depict spatial figures on a plane.

Algebra. Part of mathematics that studies problems and solutions of algebraic equations. The term was first seen in the 11th century. Applied Muhammed ben-Musa al-Khwarizmi (mathematician and astronomer).

Argument (function). Variable value (independent) with which the value of the function is determined.

Arithmetic. Science that studies operations on numbers. Originated in Babylon, India, China, Egypt.

Asymmetric. Absence or violation of symmetry (inverse of symmetry).

Infinitely large value is greater than any given number.

The infinitely small value is less than any finite value.

Billion. One thousand million (one followed by nine zeros).

Bisector. A ray starting at the corner vertex (divides the corner into two parts).

Vector. Directed straight line segment. One end is the beginning of the vector; the other is the end of the vector. For the first time the term was used by W. Hamilton (an Irish scientist).

Vertical corners. A pair of corners that has a common vertex (formed by the intersection of two lines in such a way that the side of one corner is a direct continuation of the second).

Vector is a quantity characterized not only by its numerical value, but also by its direction.

Graph - a drawing that clearly depicts the dependence of one value on another, a line that gives a visual representation of the nature of the change in the function.

Hexahedron. Hexagon. The term was first used by Pappus of Alexandria (Ancient Greek scholar).

Geometry. Part of mathematics that studies spatial forms and relationships. The term was first used in Babylon/Egypt (5th century BC).

Hyperbole. Open curve (consists of two unbounded branches). The term appeared thanks to Apollonius of Perm (ancient Greek scientist).

Hypocycloid. This is the curve that the point of the circle describes.

Homothety. An arrangement between figures (similar), in which the lines connecting the points of these figures intersect at the same point (this is called the center of the homothety).

Degree. Unit for flat corner. Equal to 1/90 of a right angle. Measuring angles in degrees began more than 3 centuries ago. For the first time such measurements were used in Babylon.

Deduction. Form of thinking. With its help, any statement is deduced logically (based on the rules of the modern science of "logic").

Diagonal. A line segment that connects the vertices of a triangle (they do not lie on the same side). First used the term Euclid (3rd century BC).

Discriminant. An expression made up of the values ​​that define the function.

The fraction is a number made up of a whole number of fractions of one. It is expressed as the ratio of two integers m/n, where m is the numerator showing how many parts of the unit are in the fraction, and n is the denominator showing how many parts the unit is divided into.

Denominator. Numbers that make up a fraction.

The golden ratio is the division of a segment into two parts so that the larger part is related to the smaller one, as the entire segment is to the larger part. Approximately equal to 1.618. The criterion of beauty, used in architecture, etc. The term was introduced by Leonardo da Vinci.

Index. Alphabetic or numeric index. With its help, mathematical expressions are supplied (this is done in order to distinguish from each other).

Induction. A method for proving a mathematical equation.

Int. The basic concept of mathematical analysis. It arose due to the fact that it took to measure volumes and areas.

Irrational number. A number that is not rational.

Leg. One of the sides of a right triangle that is adjacent to a right angle.

Square Regular quadrilateral (or rhombus). Each corner of the square is a straight line. All angles in a square are equal (by 90 degrees).

Mathematical constant. A value that never changes in value. A constant is the opposite of a variable.

Cone. A body that is bounded by a single cavity by means of a conical surface. It intersects a plane (the plane is perpendicular to its axis).

Cosine. Is one of the trigonometric functions. The designation in mathematics/higher mathematics is cos.

Equation 9 root0386 - solution, the value of the unknown, found through known coefficients.