Learn about shape
What Children Know and Need to Learn about Shape and Space
Geometry encompasses two major components. One is reasoning about shape. We learn, for example, that triangles must have three straight sides and three angles, but the angles may be narrow or wide, and the triangles may be tall or short, red or blue, or tilted in any number of ways. The second component is thinking about space. We learn how objects relate to one another and to us in space: the ball is on top of the sofa, the sofa is under the ball, and we are in front of both.
Although children accurately perceive shape and space in their everyday environments, preschool children from about three to five years of age need to learn to think about these topics. Our main educational goal should be to promote understanding of basic geometry.
ShapeContext and overview
Perception of objects begins soon after birth. From their earliest days to about 18 months, babies can easily see the differences between common objects: they see that mother is different from father and that dog is different from cat. Babies can differentiate types of objects: they see that this is the plate and this is the cup, even if they don’t know the name for each and cannot articulate the key differences between them. Further, babies can identify objects even when they change location: this is mother regardless of whether we see her from one side or another, or whether she is close or far, lying down or standing up, or partially or fully visible.
By the end of infancy, object perception is relatively well developed, and children navigate the everyday world with relative ease. At the same time, they still have a great deal to learn, particularly the analysis of shapes, that is, understanding their essential features. Learning the shape names is easy. But analyzing them is much harder. Hence, the focus of early geometry education should be on analysis and understanding.
Early perceptions and ideas of shape
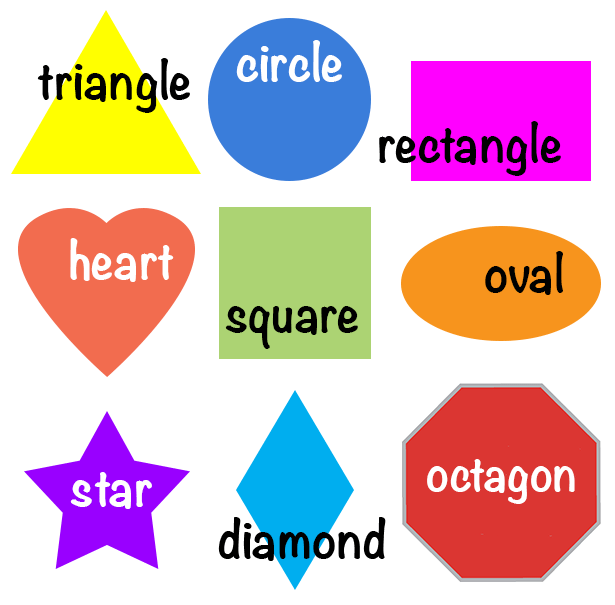
At roughly three and four years of age, children learn several aspects of shapes, both two-dimensional (2-D) and solid (3-D). The illustrations that follow mostly involve 2-D shapes, but the same points can be made about solids as well.
The illustrations that follow mostly involve 2-D shapes, but the same points can be made about solids as well.
Perception of difference and sameness
Young children can easily discriminate (see or perceive the differences) between various shapes. For example, asked whether the 2-D shapes in Figure 1 are different, children will quickly agree that they are.
They can also easily discriminate between 3-D shapes, for example, between a rectangular prism (like a book) and a sphere (like a ball), or between a sphere and a cube (like a block with six square faces).
Clearly young children can see differences between triangles and rectangles, and between books and balls. They may even know the names triangle and rectangle. But at the same time, they may not be able to analyze the basis for their discriminations. They may have no knowledge about the properties of triangles and rectangles. They may not understand, for example, that a triangle must have three sides, that it is a closed figure, or that both figures are polygons.
In brief, the ability to discriminate means only that the children see that the shapes look different. At the same time, the children may not know anything important about them. We need to distinguish between seeing and thinking, perception and thought.
What about the idea of same? Young children can see that two rectangles are perceptually the same or identical (congruent). They might even see the congruence if one of the rectangles were tilted a little to the side (but not too much!). Figure 2 shows an example.
Identifying sameness, in the sense of congruent shape, is not very difficult for young children, who are expert perceivers, at least of what is on the surface. Their perception is largely non-verbal and direct. Note that language is not essential for any of these judgments: children (or animals) can see that shapes are identical without being able to name them. Children can also give the shapes incorrect names but still accurately perceive sameness (and difference). For example, you can say that a pack of “dogs” are the same when you should have called them “elephants.” The objects are seen as the same, whatever you call them.
For example, you can say that a pack of “dogs” are the same when you should have called them “elephants.” The objects are seen as the same, whatever you call them.
Figure 3 illustrates an interesting complication. Sometimes children seem not to recognize a clear difference. For example, a three-year-old might say that the shapes in Figure 3 are the same because they both have “pointy tops.”
Does this mean that the child cannot see the difference between the shapes? Not necessarily. The child probably sees the differences, but thinks that the shapes are nevertheless the same. If the adult asks whether the shapes are at all different, the child might say that one has three sides and the other four, but they are the same because they each have that “pointy top.” So the child sees a perceptual difference, but thinks the shapes are the same because they each share a vertex on top. Actually, the child is quite right: even though the shapes are different, they are the same in the property the child describes. This is one reason to interview children in an attempt to uncover the thinking that underlies their overt responses. The child may say “same” but also understand that the shapes are different in another respect.
This is one reason to interview children in an attempt to uncover the thinking that underlies their overt responses. The child may say “same” but also understand that the shapes are different in another respect.
Classification. Young children need to go beyond perceiving sameness and difference. They must learn to classify objects that are similar (as opposed to congruent) in key respects. They need to learn that three-sided figures of different sizes are all triangles; that non-congruent but similar four-sided figures with equal length and right angles are all squares; that basketballs and globes are spheres; and that blocks varying in color can be cubes.
Some classifications are easier for young children than others. For example, they can see that squares of different sizes all go together. They can identify prototypical, that is standard common triangles, like those in Figure 4, regardless of size. Again, this can be done without knowing the names. Whatever the figures are called, some go together and others do not.
Whatever the figures are called, some go together and others do not.
Names. Of course, children need to learn the correct names. Names are useful in several ways: they allow you to communicate to others (“This is a triangle.”) and they also refer you to a category to analyze (“This is called a triangle and so are these. I wonder how they are the same.”). The English shape names are a bit odd, because many derive from Greek or Latin. For example, the word triangle derives from the Greek for “three angles.” By contrast, the Chinese shape names are transparent. In Chinese, the name for rectangle translates as "four-sided shape." Despite this, geometric names are not difficult for children to learn. Preschool children know thousands of names, including special names like Brontosaurus or esoteric names of cartoon characters or toys or action figures. Given their ability to absorb language, young children should experience little difficulty in learning names like rectangular prism or pentagon. But the adult should always keep in mind that names, while necessary, are superficial. Children need to learn to understand the properties of shapes, not just how to sort or name them.
But the adult should always keep in mind that names, while necessary, are superficial. Children need to learn to understand the properties of shapes, not just how to sort or name them.
Understanding. Understanding is multifaceted. Children need to learn to analyze shapes, identify their defining properties, and talk about them. They need to learn what makes a triangle a triangle and how a triangle is different from a square. They need to learn that a square is a sub-class of rectangles.
As mentioned above, children can easily learn to categorize prototypical shapes. They learn that equilateral, isosceles, and right triangles are all triangles. At the same time, children may not know that a long, thin, scalene triangle, like that in Figure 5, is also a legitimate member of the triangle family, and that all triangles of any color can be small or large, tipped to the side or lying on a horizontal base. Size, color and orientation do not matter when the goal is to identify shapes that are the same type.
The child's main challenge is to acquire explicit knowledge of the defining properties of shapes. Children need to understand that a triangle has certain defining properties and a square has others and that these forms are invariant over changes in size, orientation, and color. They also need to be able talk about the shapes; to explain why the triangle is a triangle even if it is not prototypical.
Children’s limited understanding of essential and non-essential properties may stem in part from the limited array of shapes that they see. Children are often exposed to prototypical shapes in books and toys. If a picture book introduces a triangle, it is likely to be equilateral or isosceles, and rarely scalene. Shape sorting toys also involve prototypes, in this case three dimensional, like an equilateral triangular prism.
Given that children are seldom presented with non-prototypical shapes, adults need to expose children to them and teach the basic properties of shapes, making explicit the reasons why one shape is a triangle and the other is a pentagon. As in other areas, adults need to help children mathematize their knowledge of shapes, that is, develop an explicit awareness of the formal mathematics. Children need to learn how to think and talk explicitly about mathematical properties such as the number of vertices and sides that define a figure.
As in other areas, adults need to help children mathematize their knowledge of shapes, that is, develop an explicit awareness of the formal mathematics. Children need to learn how to think and talk explicitly about mathematical properties such as the number of vertices and sides that define a figure.
Composing and decomposing shapes. Children also need to explore and learn about taking shapes apart and using shapes to construct other shapes. For example, if the goal is to create a square from two triangles, the child must pay attention to the interior angles and the lengths of the sides of the triangles. Composition and decomposition foster analysis.
Children can explore shapes using several activities of this type. As shown in Figure 6, children can compose shapes. When a child puts two squares of the same size together by aligning their widths, the result is a long rectangle. When a child places two identical semicircles together by aligning their diameters, the result is a full circle.
Children can also decompose shapes. As shown in Figure 7, when a child divides a rectangle along its diagonal or cuts an equilateral triangle down the middle, the child gets two right triangles.
Composing and decomposing can be a lot of fun, whether the shapes are physical forms or computer graphics, and whether the child is engaged in exploration or solving a problem created by the adult.
SpaceContext and overview
Humans (and animals too) require basic concepts of space if they are to function adequately in the everyday world. For this reason, often on their own, young children (even infants) begin to use or develop basic spatial concepts, including ideas about location, relative position, symmetry, and direction. Some spatial skills and ideas are built into the human perceptual system: even babies demonstrate that they can distinguish between near and far when they attempt to reach for the closer of two toys. Babies and toddlers further develop these abilities as they crawl or walk around, become aware of their surroundings, and think about where they are going. They know where they are in space and how to move from one location to another. In familiar places such as homes and schools, they generally know where things are and how to get to the things they want. They learn that the ball is close to the chair, that the doll is under the chair, and that the fastest route to the chair involves going under the table. They learn to use words to describe positions, locations, and directions. When they get older they use blocks and other objects to create symmetries that are sometimes beautiful, such as the creation shown in Figure 8.
They know where they are in space and how to move from one location to another. In familiar places such as homes and schools, they generally know where things are and how to get to the things they want. They learn that the ball is close to the chair, that the doll is under the chair, and that the fastest route to the chair involves going under the table. They learn to use words to describe positions, locations, and directions. When they get older they use blocks and other objects to create symmetries that are sometimes beautiful, such as the creation shown in Figure 8.
Although their everyday spatial ideas are often useful (as in the case of moving around familiar surroundings) and sometimes surprisingly powerful (as in the case of complex symmetries), young children still have a great deal to learn and need adults to help them move forward. Teachers and parents can build upon and extend what young children already know about space. Adults can help young children mathematize their everyday ideas of space. This involves using language and various representations to describe and understand spatial ideas.
Adults can help young children mathematize their everyday ideas of space. This involves using language and various representations to describe and understand spatial ideas.
The Importance of a Mathematical Understanding of Space
There are many reasons for learning about space, just as there are for shape and number.
Space is interesting in itself. Spatial ideas include the following:
- Simple location and position: the dog is on top of the elephant and at the same time the elephant is under the dog.
- Perspective: from where I sit I see the cat on the left and the whale on the right, but from where you sit, opposite me, you see the cat on the right and the whale on the left.
- The coordinates on the Cartesian plane: the poodle is in the fourth row and the fifth column.
- Directions: the child can get to the treasure chest by walking two steps forward, turning right, and then moving four steps forward, whereupon the child makes a half turn leftward and follows the diagonal for five paces.

- The aesthetic qualities of reflectional symmetry: this symmetrical design is really beautiful but this asymmetrical mess is not.
Each of these topics presents challenges: if the mouse is on top of the cat that is on top of the dog, then the cat is both on top of and underneath something else at the same time. Children find it difficult to coordinate the two different relations (such as on top of and underneath), but adults can help.
Spatial ideas underlie much of our mathematical understanding. To understand addition, a child might use ideas of merging two separate groups of objects or jumping to the right on a standard number line. To understand subtraction the child might think of monkeys jumping off a bed. To understand equivalence, the child might imagine balancing objects on a scale. To understand multiplication, the child might refer to areas or arrays of dots. Indeed, spatial metaphors and ideas permeate children’s and adults’ understanding of number.
Spatial understanding, language, and symbolism are of practical value. Children (and adults) live in space. Moving purposefully in it requires first an understanding of spatial relations in the everyday environment, as when the baby learns that the stuffed cow is behind the sofa. Later, the child learns and uses appropriate spatial language to get around in the world (for example, when mother says, “Go to the living room and look under the sofa for your toy raccoon.”). Later still, reading a highway map is necessary for getting to a destination. The adult uses ideas of space to build a bookcase or carpet a room. Language and symbolism allow us to surpass the everyday spatial knowledge of animals.
Spatial knowledge and language predict future academic performance. Those children who acquire a solid understanding of space and spatial language tend to demonstrate higher math achievement than students who do not achieve such mastery.
What Children Need To Learn About Space
Children have an informal knowledge about space on which early math education can build. They are quite capable of learning more about the following important topics.
They are quite capable of learning more about the following important topics.
Basic position. Children are skilled at locating things in their environments. They often use informal or vague language to describe where things are in relation to other things, including themselves. But they need to deepen their understanding of position and learn correct mathematical words for talking about it.
Consider how a child might specify the locations of objects and people in a room.
- I am standing on top of the desk.
- I put my gooey candy on the white sheet.
- I threw the apple core behind the tree.
- I put my underwear under the bed.
- I put the pickle in the middle of the sandwich.
- My chair is below the window.
- There is a spider in the bottom of the cup.
- I put the coin in my piggy bank.
All of the nouns in the examples refer to things, classes of objects that the child can easily identify. For example, there are many kinds of apples and the child can easily learn to identify them all as apples. But positions and locations are abstract ideas, and all are relative. For example, the pickle in the middle of the sandwich touches the bread immediately on top of and below it. The underwear underneath is relative to the bed above it.
For example, there are many kinds of apples and the child can easily learn to identify them all as apples. But positions and locations are abstract ideas, and all are relative. For example, the pickle in the middle of the sandwich touches the bread immediately on top of and below it. The underwear underneath is relative to the bed above it.
Another way of thinking about spatial relations is that objects serve as landmarks for the location in question. Landmark means to mark the land, or to specify an object that helps define the location. Even young infants can use landmarks to find the location of a hidden object. For example, if you place a toy behind a sofa as a child watches, she can locate the toy later: she knows that the toy is behind the sofa. In this case, the toy is the object, the sofa is a landmark, and the relation between the landmark and object is behind or in back of.
In all of these cases, children need to learn two things: the words and the concepts. They need to learn positional words such as above or next to and they need to know the concepts to which these words refer. For example, the words next to refer to a concept specifying that an object is adjacent to another in a variety of ways, either on its right or its left. You can help children learn to develop these words and concepts by modeling. For example, if a preschooler is asked, "Where are the picture books?" she may reply by saying, "Over there" and gesturing. You can reply, "You're right. They are on the shelf next to the coat closet."
They need to learn positional words such as above or next to and they need to know the concepts to which these words refer. For example, the words next to refer to a concept specifying that an object is adjacent to another in a variety of ways, either on its right or its left. You can help children learn to develop these words and concepts by modeling. For example, if a preschooler is asked, "Where are the picture books?" she may reply by saying, "Over there" and gesturing. You can reply, "You're right. They are on the shelf next to the coat closet."
Complex positions. Left and right are notoriously difficult for young children to learn, and they need a lot of practice with these ideas. First, children need to remember that one hand is on the right and the other is on the left. This idea is reinforced when children put a sticker on their right hand and then shake hands properly. Then children must apply the idea of right and left to external objects.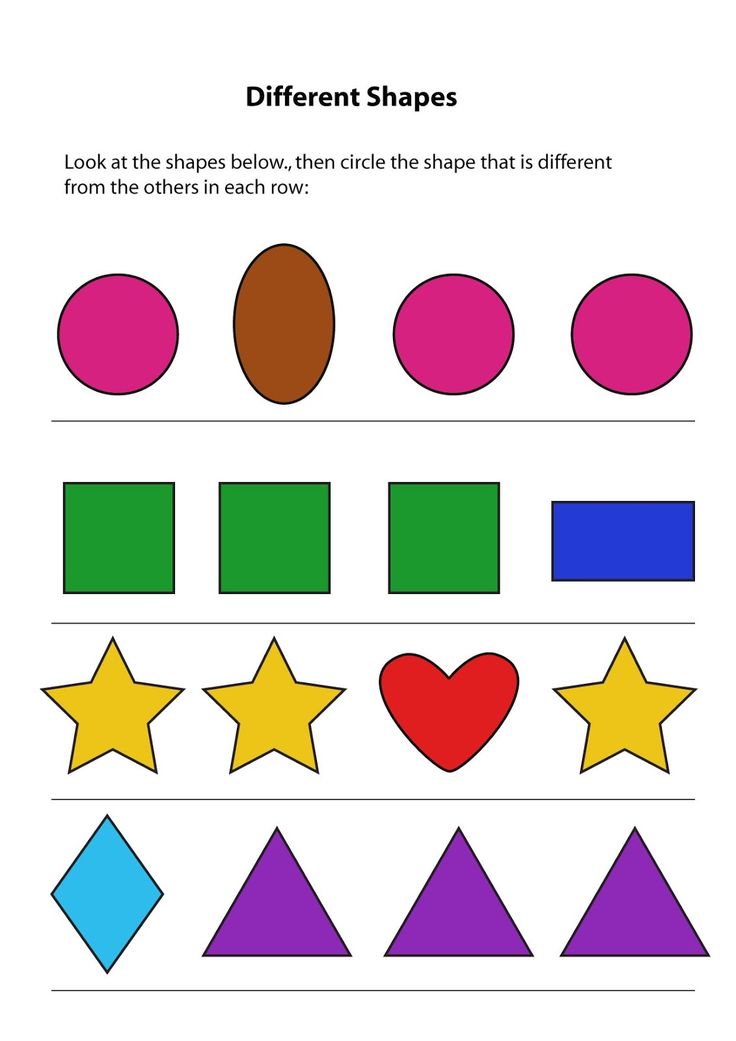 This is especially difficult because these concepts are always relative to the direction the child is facing. For example, if Mario is looking at a table from one direction, he sees a book on his right and a block on his left. When he moves to the opposite side of the table, he sees the reverse. Children are not likely to master left and right until they are older, perhaps in early elementary school. Don’t obsess about their initial failure.
This is especially difficult because these concepts are always relative to the direction the child is facing. For example, if Mario is looking at a table from one direction, he sees a book on his right and a block on his left. When he moves to the opposite side of the table, he sees the reverse. Children are not likely to master left and right until they are older, perhaps in early elementary school. Don’t obsess about their initial failure.
Understanding dual relations, taking different perspectives at the same time, is very difficult for preschoolers. In our previous example, the pickle in the sandwich is at the same time above the bottom piece of bread and below the top piece. How can a pickle endure the ambiguity—the dual identity or split personality—of being simultaneously above and below? Because children tend to be egocentric, that is, to see things and their relations only from one point of view, they find it difficult to deal with dual, or more generally, multiple relations. In this way, children are similar to the pickle.
In this way, children are similar to the pickle.
Another task, locating objects in two dimensions, involves simple dual relations between height and width. Suppose the child sees a grid of unit squares that goes up five spaces and sideways five spaces (and of course has all the appropriate squares in the middle).
Someone has placed an object on the grid, as shown in Figure 9. I ask the child to describe where it is because I can’t see it. She can say it is over there, but that does not help. She can say it’s above that square, but that doesn’t help either. What she has to say is something like this: “Go to the bottom square on the left. Then go four squares up and two squares to the right.”
In everyday life children engage in locating or directional activities. Hopscotch, for example, is about jumping to different squares according to a series of numbers. Another example is board games such as Sorry, in which they may go forward a designated number of spaces and later must go backwards.
At the preschool level, teachers can help children become increasingly skilled at following location directions and understanding them. At snack time, for example, the teacher can say, “When we set the table for snack, we put the cups next to the plates, and we put the juice in the middle of the table where you can all reach it. And remember to put a napkin on this side of each plate.”
Complex relations also include another important mathematical topic, symmetry, which is ubiquitous in nature. The butterfly’s left wing is the mirror image of the other. The human body embodies symmetries: the left hand is a mirror image of the right hand.
Mathematics defines many different kinds of symmetries. Consider the mirror image idea, namely that symmetry bisects a figure in such a way that one side is in the opposite orientation from the other. Imagine that you have some figure on a flat surface. You hold a mirror vertically next to the figure. You then have the figure and the mirror image of the figure. The figure and mirror image are symmetrical. Using a mirror in this way can help children to explore and to understand what line symmetry means. For example, in Figure 10, each shape is symmetrical and each line is a line of symmetry.
The figure and mirror image are symmetrical. Using a mirror in this way can help children to explore and to understand what line symmetry means. For example, in Figure 10, each shape is symmetrical and each line is a line of symmetry.
Children create both two-dimensional and three-dimensional symmetries all the time when they play with blocks. Figure 11 shows an example of how a child can explore symmetries using pattern blocks. Notice that in order to make this figure, the child had to manually rotate some of the blocks, such as the red trapezoids, to produce the mirror image. Young children may need to physically manipulate objects to correctly show the reflection. Further, these experiences can help a child later to develop the ability to see how shapes can be mentally turned or flipped without having to construct them.
Maps. Maps involve a special kind of symbolism showing where things are in relation to one another. You can represent a classroom in many different ways. You can describe it in words. You can take a picture of it. You can do a realistic drawing of it. You can create a smaller three-dimensional model of it. You can create a two-dimensional map of it from a bird’s eye view, above. You can create a topographical map of it. You can create something like a subway map that shows relative position but does not accurately capture distances.
You can describe it in words. You can take a picture of it. You can do a realistic drawing of it. You can create a smaller three-dimensional model of it. You can create a two-dimensional map of it from a bird’s eye view, above. You can create a topographical map of it. You can create something like a subway map that shows relative position but does not accurately capture distances.
Before children can understand how their classroom looks in all these different ways, they must understand point of view or perspective. Infants' and young children's early spatial thinking is often from their own perspective. A child thinks of an object's location in relation to his own location in space. Later, when children realize that they have a distinct point of view, they can begin to imagine how space looks from other perspectives. For example, the child may come to see that the way the classroom looks from her chair is different from the way the classroom looks from a friend's chair, or the way the classroom looks from her spot on the rug differs from the way the classroom looks from the chair the teacher sits on during rug time. It is not easy for young children to de-center and visualize how spaces look from other points of view. Working with maps and models can provide children with experiences that will help them see space from other perspectives.
It is not easy for young children to de-center and visualize how spaces look from other points of view. Working with maps and models can provide children with experiences that will help them see space from other perspectives.
Standard maps (though maybe not topographical) are hard for children to understand because they represent a three-dimensional reality on a two-dimensional space and also because the map is proportionally smaller than the reality. Preschoolers need to learn first how to read simple maps, such as a map of the classroom, and then to create them. Map activities can take place at circle time, where the teacher can use a map to show children where they should sit, or at line-up, when a map can show who goes first, second and so on, so that the child can identify both her own position and her friend’s.
Conclusions for Shape and Space
Shape and space are fundamental mathematical topics that children need to explore. They need to grasp the basic concepts, mathematize and elaborate on their everyday knowledge, and learn to communicate what they have learned. The study of geometry can be deeper than many adults imagine and can provide young children with enjoyable intellectual challenges.
They need to grasp the basic concepts, mathematize and elaborate on their everyday knowledge, and learn to communicate what they have learned. The study of geometry can be deeper than many adults imagine and can provide young children with enjoyable intellectual challenges.
Background Reading
Clements, D. H. Geometric and spatial thinking in young children. In Copley, J. V. (Ed.). (1999). Mathematics in the early years. Reston, VA: National Council of Teachers of Mathematics.
Dehaene, S. (2011). The number sense: How the mind creates mathematics (Rev. and updat ed.). New York: Oxford University Press.
Early Math Collaborative at Erikson Institute. (2014). Big ideas of early mathematics: What teachers of young children need to know (First ed.). Boston: Pearson.
Hawes, Z., Tepylo, D., & Moss, J. (2015). Developing spatial thinking: Implications for early mathematics education In B. Davis and Spatial Reasoning Study Group (Eds. ). Spatial reasoning in the early years: Principles, assertions and speculations (pp. 29-44). New York, NY: Routledge.
). Spatial reasoning in the early years: Principles, assertions and speculations (pp. 29-44). New York, NY: Routledge.
Lehrer, R., Jacobson, C., Kemeny, V., & Strom, D. (1999). Building on children's intuitions to develop mathematical understanding of space. In E. Fennema & T. A. Romberg (Eds.), Mathematics classrooms that promote understanding. Mahwah, NJ: Lawrence Erlbaum Associates, Publishers.
National Research Council. (2009). Mathematics learning in early childhood: Paths toward excellence and equity. Committee on Early Childhood Mathematics, Christopher T. Cross, Taniesha A. Woods, Heidi Schweingruber, Editors. Center for Education Division of Behavioral and Social Sciences and Education, Washington DC: The National Academies Press.
Nora S. Newcombe & Mike Stieff (2012): Six Myths About Spatial Thinking, International Journal of Science Education, 34:6, 955-971
Sarama, J., & Clements, D. H. (2009). Early childhood mathematics education research: Learning trajectories for young children. New York: Routledge.
Why Teach Shapes? Why It Matters and Easy Learning Activities (Plus Printables!)
Why teach shapes? Learn why children need to learn shapes and the best activities to help. Plus printables!
Shapes are one of those things we work on with kids from a young age. We teach what a circle is, a triangle, a square. Colors, letters, numbers, shapes.
These are some of the first things we work on with children.
Colors are easy and fun to point out. Numbers and letters are building blocks for their entire future.
But why do we focus on shapes? Are they just easy?
There are actually some very concrete reasons for teaching toddlers and preschoolers shapes.
Here are 5 big reasons to teach preschoolers and toddlers shapes, plus some fun printables to color or use play-doh for sensory shape fun.
What's In This Post?
- Why Teach Shapes?
- Same or Different
- Categorization
- Problem Solving and Spatial Relations
- Math Skills
- Grab these printables for more shape practice!
- Letter Recognition
- Ways to Learn and Practice Shapes
- Just Talk
- Shape Hunts
- Shape Toys
- Coloring and Tracing
- Play-Doh Shapes
- Shape Sticks
- Shapes Everywhere
- I want the Freebies!
- Thank you!
- Bonus Shape Practice Printables
- Related
Why do we teach shapes to our children?
It isn’t just because it is easy. Shapes are building blocks for several bigger concepts that children will use throughout their schooling and lives.
There are big reasons to start shape learning early. Here are some major concepts they work.
Same or DifferentBasic shapes like circles, triangles, and squares are distinctly different from each other. This makes them great for practicing same and different.
Same and different is the start of really recognizing distinctive traits objects can have. It is also the first level of categorizing.
Are these two objects the same or different? This is the start of basic observation skills.
CategorizationOnce the basic same or different concept is down, children can move into more complicated categorization.
What makes a circle different from a square? This is detailed recognition of traits objects have.
An important skill is being able to find a common characteristic among different items. A great way to practice this is to send your child on a shape hunt.
A great way to practice this is to send your child on a shape hunt.
How many circle objects can they find? How many squares? This shows them that shape is something that many different things can have in common.
Categorization is an important science skill.
Problem Solving and Spatial RelationsShapes are a great way to introduce problem-solving skills.
Take a shape sorter. Your child needs to determine which shape goes in which hole, and then they need to actually fit it in.
This works spatial relation skills as well. How do objects fit together? (As your children grow puzzles work the same sort of skill. How do you recognize what piece fits where?)
Anything that works problem-solving is helpful for children, and this sort of trait recognition is key for future science skills.
Math SkillsI feel math skills are an obvious area that gets worked when you teach your children shapes.
Geometry is in part the study of shapes. Having a basic understanding of them from a young age will make this math class a lot easier for kids.
Having a basic understanding of them from a young age will make this math class a lot easier for kids.
Understanding the characteristics of shapes involves a lot of counting. How many sides does a shape have? This easily folds in number practice when you play with your toddlers and preschoolers.
Pattern recognition comes into play as well. Every time you add a side you get a new shape, this is a pattern. Being able to physically see the difference between a shape with three sides and one with five helps with number sense, a key to all math success.
(Learn more about number sense and why it matters here.)
Grab these printables for more shape practice!
Letter Recognition
This is one of the biggest reasons to work on shapes early. Shapes help with letter recognition.
Think about letters, they are very similar to shapes. The letter V is a triangle missing a side, and the letter O is just a circle.
Understanding what shapes are and that they have meaning starts to build literacy. Practicing distinguishing between a square and a triangle help kids recognize between different letters.
The same is true for reading numerals. Having children draw shapes and working on the lines and angles needed to draw a triangle or rectangle helps them later in life when they are learning to write their letters.
Ways to Learn and Practice ShapesThere are lots of ways to practice shapes. One of my favorite things about this topic is that you can make it more and more challenging as your child learns more and more.
You start with circles, triangles, and squares. Then there are pentagons, hexagons, heptagons, octagons, and more. (This is a great lesson in prefixes too.)
Even with just 4 sides, there are a variety of shapes to work on. Square versus rectangle. Trapezoids, diamonds, parallelograms.
And don’t forget hearts, stars, crescents, and more. There is just so much depth to this simple subject.
There is just so much depth to this simple subject.
Here are some fun and easy ways to work on shapes with your children. Play is key with these.
Just TalkI think we try to make teaching our children too hard sometimes. The simplest way to work on shapes is just to talk about them.
My mother-in-law calls the toddler years the years of two names. Everything you share with your children has two names. For example, you don’t just hand your child a cup you hand them the blue cup. Or we play with the red ball.
Whatever it is, we can naturally add descriptors that are teaching our children. Use shapes the same way. You can read the square book or play with the triangle magnet. You don’t need big explanations, especially with very young toddlers.
Just start using the vocabulary.
Shape HuntsMy kids love going on scavenger hunts. It doesn’t matter what we are looking for, they adore it.
So send your kids on a shape hunt!
I have my children gather up anything that is triangle shaped, or circles. It is easier to focus on one shape at a time with toddlers. Preschoolers can look for more than one shape at a time, adding in sorting practice along with the shape practice.
It is easier to focus on one shape at a time with toddlers. Preschoolers can look for more than one shape at a time, adding in sorting practice along with the shape practice.
You don’t need anything special for this. Regular household items and kid toys come in all shapes. But if you want to make it more specific, try cutting shapes out of construction paper.
Low cost and easy cleanup.
Shape ToysShape sorters are a staple for a reason. You can find them in every price range. (In fact, Ali and Sammy’s favorite shape sorter came from Walgreens for about $5.)
These sorters practice shape recognition, problem-solving skills, and spatial relations, all in one little toy.
You can even make your own. Grab some blocks or other key shapes and trace them onto a shoebox or other box. Then cut the shapes out and let your children sort!
Coloring and TracingColoring books and shape workbooks are a wonderful way to work on shapes. Plus you get work on sitting still and focus skills.
Plus you get work on sitting still and focus skills.
Coloring is a childhood staple that is full of benefits. (Learn all about the benefits of coloring here!)
While your child is coloring, talk about the shapes on the page just as you would talk about the colors they are using. Tracing books provide additional writing practice that will come in handy when they are older.
Play-Doh Shapes
This is one of my favorite ways to work shapes. Sensory activities are stepping-stones for STEM skills in life, plus they help children really engage in what they are learning.
I created some shape printables for my children. You can laminate them and have your child fill the shapes in with Play-Doh. (Or slime, if you have a slime loving child.)
I don’t have a laminator, as much as I want one. So instead of that I purchased a few clear plastic sleeves used for binders and put the printables in those.
Easy to put together and easy to clean up. Head to the bottom of this post to learn how to get these for free to use with your children!
Head to the bottom of this post to learn how to get these for free to use with your children!
A great shape activity you can use as a busy bag activity is shape sticks!
Learn how to make them all here: Shape sticks for Preschool and Toddlers
The short version is to group sticks to make shapes and write the number of sticks needed on each group. So write 3 on three sticks for a triangle, etc. This is a great way to build independent play skills while practicing shapes!
Shapes EverywhereShapes are another one of those seemingly simple topics that have big implications for children. And adults.
Think of all the symbols and signs we can recognize just by the shape, even without words. Octagons are stop signs. An upside down triangle is a warning. Hearts mean love and stars are usually a good thing.
Shapes are such an important first step towards literacy and math skills. Things like shapes seem so simple and basic, yet they are teaching our children more than we can imagine.
You can get these coloring pages and Play-Doh mats for your kids! These are available for download in my free printables section. You can gain access to all the free resources by signing up for the Team Cartwright mailing list. Once you sign up you will get an email with the printables password. This library contains all kinds of learning aids. The color by number pages, train learning activities, and my Lego coding worksheets. It also unlocks my twin tracking worksheets! Signing up also adds you to my email list, which you can, of course, unsubscribe from at any time.
I want the Freebies!
Sign me up to join the team! This gives me access to the free printables and adds me to the Team Cartwright mailing list.
Bonus Shape Practice Printables
Hands-on activities are amazing for learning about shapes. But worksheets can be helpful as well. These three free worksheets are great for shape practice.
But worksheets can be helpful as well. These three free worksheets are great for shape practice.
Your child can practice tracing the shapes and then draw them in with the dots. Finally, practice scissor skills while you cut and paste the pictures with the proper shape. Easy and fun! (And don’t forget to let them color their shapes too.)
Get the worksheets!
Find your next fun activity! Where would you like to start?
How useful was this post?
Click on a star to rate it!
Let us improve this post!
Tell us how we can improve this post?
test at home
Makeup according to the type of face is a sure way to emphasize the dignity of appearance. We will help you determine your type right now
Contents:
- Classification of persons according to form
- How to determine the type of face at home: 2 ways
- How to determine the type of face: test
It's good when beautiful make-up attracts attention. But even better - when he emphasizes the dignity of appearance, natural beauty. This is possible only if the make-up is created taking into account the features of the face. Shades of cosmetics should be in harmony with parameters such as skin tone, eye and hair color, and it is important to use beauty techniques taking into account the type of face. Our material will help you determine the type of face in order to create the most winning images.
But even better - when he emphasizes the dignity of appearance, natural beauty. This is possible only if the make-up is created taking into account the features of the face. Shades of cosmetics should be in harmony with parameters such as skin tone, eye and hair color, and it is important to use beauty techniques taking into account the type of face. Our material will help you determine the type of face in order to create the most winning images.
Classification of faces according to shape
The type of face is usually understood as its shape. When working on a make-up, beauty gurus always take this characteristic into account in order to get the best result.
1
Oval face
© Makeup.ru
This form is considered to be a reference. Of course, such an assessment can be called conditional. But it is considered correct not without reason - the oval is proportional and needs to be corrected in exceptional cases. Even if something needs to be corrected, it is usually not difficult to do this: for example, in the case of a high forehead, the problem can be solved with a bang. As for makeup, there are no special instructions here.
As for makeup, there are no special instructions here.
2
Round face
© Makeup.ru
Many girls with round cheeks think they have a round face. In fact, the form is determined by other signs: this type corresponds to faces with the same distances from the tip of the chin to the forehead and from one temple to the other. There is another important characteristic - the absence of sharp lines, sharp corners; instead of them - soft outlines, smooth contours.
- With a round type, they usually try to visually stretch the face a little and add graphics, thereby achieving harmony. Contouring techniques work best in this sense: highlighting the cheekbones with a bronzer, a slight darkening at the hairline.
3
Square face
© Makeup.ru
This type is characterized by a wide forehead and jaw, angular features, sharp cheekbones.
- Perhaps the most common request with this form is how to make the face oval visually narrower.
 Certain contouring techniques will help. Taking into account the fact that a square type of face, as a rule, corresponds to a “heavy” jaw, make-up with an emphasis on the eyes, and not on the lips, will help to achieve balance.
Certain contouring techniques will help. Taking into account the fact that a square type of face, as a rule, corresponds to a “heavy” jaw, make-up with an emphasis on the eyes, and not on the lips, will help to achieve balance.
4
Triangular face, "heart"
© Makeup.ru
This type is easily recognizable by the narrowing in the lower part of the face and the sharp chin. The forehead, on the contrary, with this form is wide.
- Girls with a triangular face type are not recommended to do styling with volume at the roots. Lush curls just below the shoulders will balance the image, and also a make-up with bright lips, which will divert attention from a large forehead.
5
Diamond type
© Makeup.ru
This type is considered by many to be a kind of oval shape, but here the contour of the face is clearer, more graphic (with a narrow chin, sharp cheekbones).
-
Sometimes, with this face shape, girls tend to optically reduce their high forehead.
 You can do this with bangs or by darkening the hairline with a bronzer.
You can do this with bangs or by darkening the hairline with a bronzer.
How to determine the type of face at home: basic methods
© GettyImages
Visual method
For an experienced make-up artist, one glance is all it takes. Indeed, the visual method is the surest and, moreover, very simple, because it is enough to project the main features of different types onto your face and find matches.
- So, if the face is elongated, with smooth outlines and a slight, barely noticeable downward narrowing, then it is of an oval type.
- With a round shape, the face is wider; there are no narrowings, as well as "graphics" - sharp corners and sharp contours.
-
A pointed chin and broad forehead are signs of a triangular face,
-
Massive jaw without pointing to the chin - a characteristic feature of the square type.
Precise calculations
But there are situations when it is difficult to determine the type “by eye”. Then they resort to simple tests: schematic sketches and simple calculations will clarify the situation at home.
Then they resort to simple tests: schematic sketches and simple calculations will clarify the situation at home.
© GettyImages
1
The first is based on measurements: the distances from the top of the forehead to the bottom of the chin, from ear to ear, and so on. The point is to compare the results and find out which part of your face is the widest. This will be an indication of your type of face.
- For example, if the forehead is the widest, then you most likely have a "heart" face.
- If your chin is wide, then your type is square.
- What was the longest line from the hairline to the tip of the chin? This is a sign of an oval or diamond shape (to clarify the type, pay attention to which outlines prevail - sharp or smooth).
- When the distance from the hairline to the chin coincides with the intertemporal distance, this indicates that you are the owner of a round face type.
So that there is no doubt, compare the results of measurements with the shape of the chin: is it sharp, wide and slightly “rectangular” or rounded? This will serve as clarifying information.
2
One way to simplify this procedure is to repeat the shape of the face on the mirror. This can be done with a marker or felt-tip pen, which is easily washed off with water. Looking at your reflection, try to outline the contour of your face directly on the mirror surface. The resulting figure will leave no doubt about the shape of the face.
How to determine the type of face: test
© GettyImages
1
How to evaluate the shape of the face in terms of proportions?
- symmetrical
- long, long
2
Your face is the widest…
- at the forehead
- on cheekbone line
- at chin and jaw level
3
What is your chin shape?
- round
- pointed
- rectangular
Results
- A symmetrical face with the widest line at the level of the cheekbones and a rounded chin can be classified as a round type.
- The combination of an elongated shape, the greatest width at the cheekbones and a rounded chin indicates that your face type is oval.
- A pointed chin in the same combination is a sign of the rhomboid type.
- A long face with a wide forehead and a pointed chin? It can be argued that your type is "heart".
- And if, according to the results of the test, you get a combination of symmetry, a wide jaw and right angles, your face type is square.
Have you already had to determine the type of face? How did you do it - from a photo or “live”, using calculations or a test? Leave a comment.
Checking and sharing the results of the form
Tip: Learn more about Microsoft Forms, or get started right away and create a survey or quiz. Want to use more advanced branding, question types, and data analysis? Try Dynamics 365 Customer Voice.
Microsoft Forms includes detailed, real-time analytics that provide summary information about responses and individual results for surveys and other types of forms. Results can be exported to Microsoft Excel for more detailed analysis, deleted or printed.
Results can be exported to Microsoft Excel for more detailed analysis, deleted or printed.
View response summary information for form
In Microsoft Forms, open the form for which you want to view results and open the Responses tab.
At the top (or bottom of the mobile site) you'll see summary information about the form, such as the number of responses and the average time respondents took to complete the form. Next to each question, you'll see the number of answers and a chart showing the breakdown of the answers.
Note: The Ideas icon will be visible when information is generated for your form. More.
View details of each question
For more information, select the More Information link under the individual questions on the Answers tab.
Tip: Select column headings to sort the results.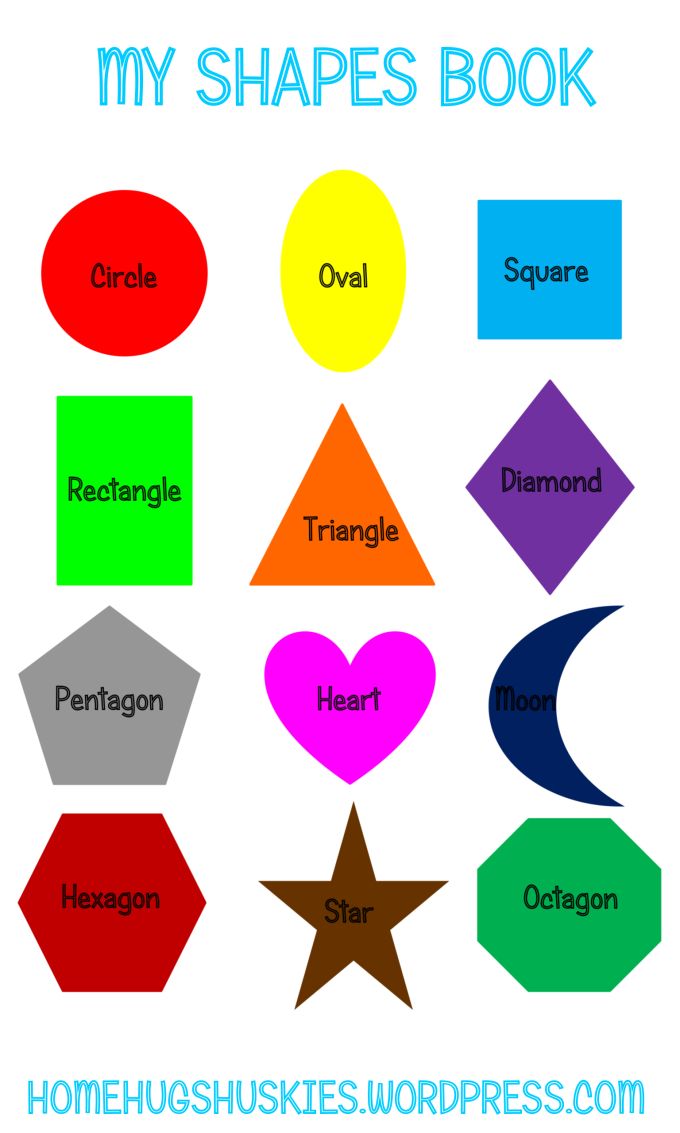
Note: This feature is not yet available on the mobile site.
Checking response data for each question
Select View results, to view individual information for each respondent.
Use the left and right arrows to scroll between the results of other answers.
You can also use this list to navigate to the results of a specific respondent.
Note: If you have more than 50 respondents, scroll down and select Show more respondents to see the remaining answers.
You can also search for a single answer, enter a number (eg 25) in the Respondent field associated with this answer.
Tip: Searching for an individual response by its associated identification number (ID) is especially useful when collecting responses anonymously.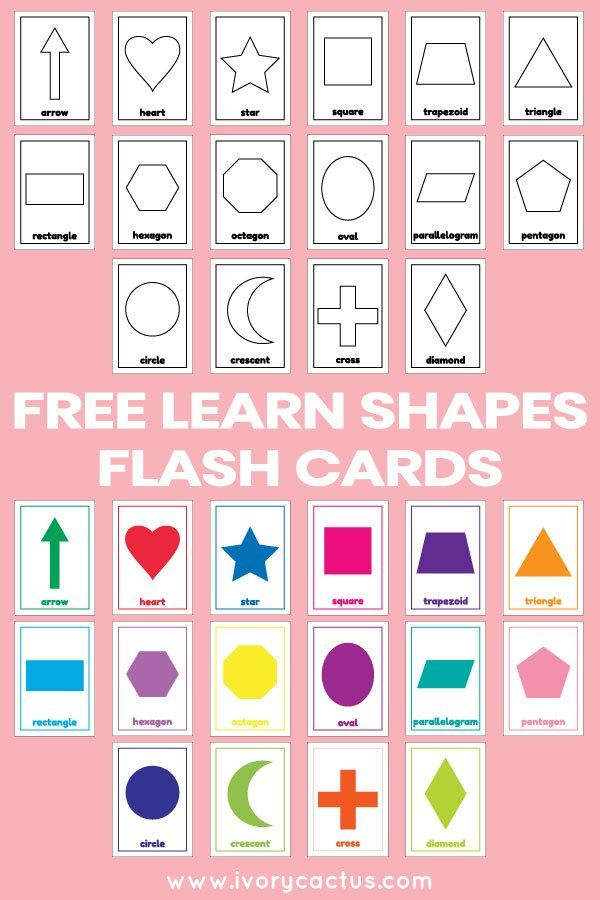 The respondent ID is exclusive to each response that can be found in the first column of the workbook if you export the responses and open them in Excel.
The respondent ID is exclusive to each response that can be found in the first column of the workbook if you export the responses and open them in Excel.
Select More to delete all answers, print a summary of answers, create a summary link to share with others, or sync all answers to a new workbook.
Tip: Learn more about printing forms.
Note: This feature is not yet available on the mobile site.
Viewing the results of a form in an Excel workbook
You can easily view all responses to a form in Microsoft Excel. Select Open in Excel on the Responses tab to get a snapshot of the current response data in the workbook.
Notes:
-
This feature is not yet available on the mobile site.

-
If you created a form in Microsoft Forms and choose to open in Excel , Excel opens that does not have a link or connection to the form.
-
If you created a form in OneDrive and choose to open in Excel, the Excel workbook is automatically stored in OneDrive for work or school and contains a form data connection in OneDrive for work or school mode. Any new response data in the form will be reflected in the workbook.
-
When you create a form in Excel for the web and choose to open in Excel, the Excel workbook is automatically stored in OneDrive for work or school and contains a OneDrive for work or school form data connection.
 Any new response data in the form will be reflected in the workbook.
Any new response data in the form will be reflected in the workbook.
Troubleshooting data export
If you're having download errors, learn how to troubleshoot problems exporting form data to Excel.
Analyzing form results in Excel
In an Excel workbook, information about each question in a form is presented in columns, and each answer is presented in a row. The first five columns display the respondent ID, start and end times for each response, and each respondent's name and email address. Subsequent columns display the respondent's choices.
Notes:
-
This feature is not yet available on the mobile site.
-
The respondent ID is exclusive to each response in the form. To find individual responses and their corresponding respondent IDs, select view results on the form's Responses tab.
-
Any changes made to the workbook do not change the contents of the form.
-
Names and email addresses are not displayed if you allow the form to accept anonymous responses.
Share your results with others
-
Select the Answers tab.

-
Select More options > create summary link .
-
Click Copy .
When you enter this link, anyone with the link can view the response summary for your form.
Notes:
-
If you're on a mobile site, go to the Answers tab at the bottom of the site and select the arrow in the center. You can copy the link or email it.
-
If you're co-creating a form with certain people in your organization, only you, as the owner of the form, can create a summary link for it if it doesn't already exist. Collaborators can still copy and share the summary link, but they can't create it themselves.

Learn more