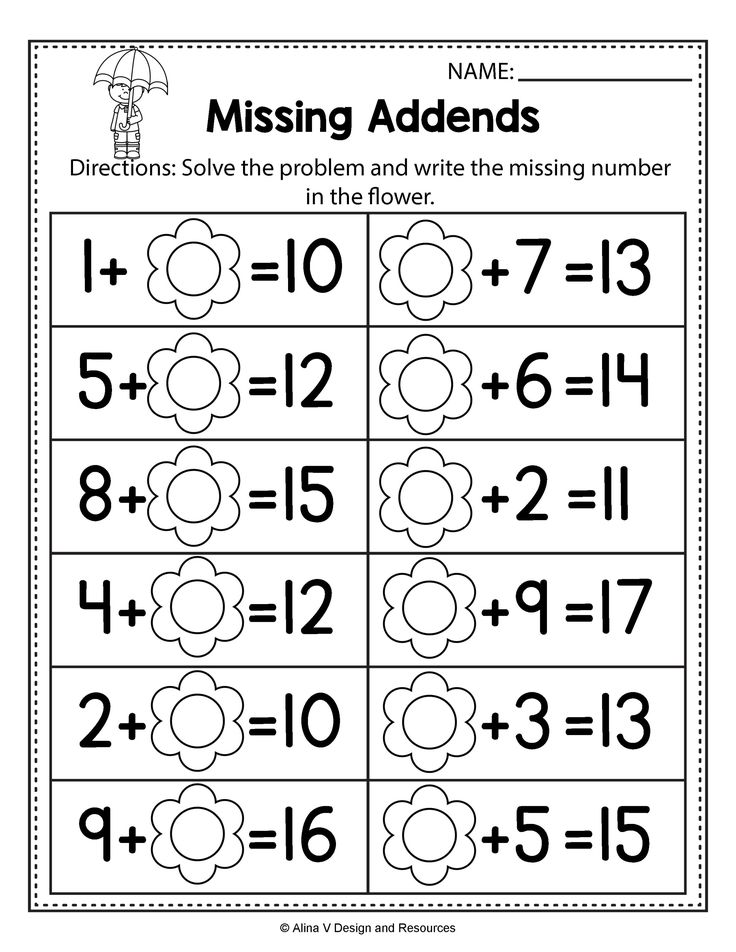
Home » Misc » Mathematics definition for kids

Mathematics definition for kids


Mathematics Facts for Kids

Kids Encyclopedia Facts

A page from al-Khwārizmī's Algebra

Mathematics is the study of numbers, shapes and patterns. The word comes from the Greek word "μάθημα" (máthema), meaning "science, knowledge, or learning", and is sometimes shortened to maths (in England, Australia, Ireland, and New Zealand) or math (in the United States and Canada). The short words are often used for arithmetic, geometry or simple algebra by students and their schools.

Mathematics includes the study of:

  • Numbers: how things can be counted.
  • Structure: how things are organized. This subfield is usually called algebra.
  • Place: where things are and their arrangement. This subfield is usually called geometry.
  • Change: how things become different. This subfield is usually called analysis.

Mathematics is useful for solving problems that occur in the real world, so many people besides mathematicians study and use mathematics. Today, some mathematics is needed in many jobs. People working in business, science, engineering, and construction need some knowledge of mathematics.

Contents

  • Problem-solving in mathematics
  • Areas of study in mathematics
    • Number
    • Structure
    • Shape
    • Change
    • Applied mathematics
  • Famous theorems
  • Foundations and methods
    • History and the world of mathematicians
  • Awards in mathematics
  • Mathematical tools
  • Related pages
  • Images for kids

Problem-solving in mathematics

Mathematics solves problems by using logic. One of the main tools of logic used by mathematicians is deduction. Deduction is a special way of thinking to discover and prove new truths using old truths. To a mathematician, the reason something is true (called a proof) is just as important as the fact that it is true, and this reason is often found using deduction. Using deduction is what makes mathematics thinking different from other kinds of scientific thinking, which might rely on experiments or on interviews.

Logic and reasoning are used by mathematicians to create general rules, which are an important part of mathematics. These rules leave out information that is not important so that a single rule can cover many situations. By finding general rules, mathematics solves many problems at the same time as these rules can be used on other problems. These rules can be called theorems (if they have been proved) or conjectures (if it is not known if they are true yet). Most mathematicians use non-logical and creative reasoning in order to find a logical proof.

Sometimes, mathematics finds and studies rules or ideas that we don't understand yet. Often in mathematics, ideas and rules are chosen because they are considered simple or neat. On the other hand, sometimes these ideas and rules are found in the real world after they are studied in mathematics; this has happened many times in the past. In general, studying the rules and ideas of mathematics can help us understand the world better. Some examples of math problems are addition, subtraction, multiplication, division, calculus, fractions and decimals. Algebra problems are solved by evaluating certain variables. A calculator answers every math problem in the four basic arithmetic operations.

Areas of study in mathematics

Number

Mathematics includes the study of numbers and quantities.It is a branch of science the deals with logic of shape,quantity and arrangement. Most of the areas listed below are studied in many different fields of mathematics, including set theory and mathematical logic. The study of number theory usually focuses more on the structure and behavior of the integers rather than on the actual foundations of numbers themselves, and so is not listed in this given subsection.
Natural numbers Integers Rational numbers Real numbers Complex numbers
Ordinal numbers Cardinal numbers Arithmetic operations Arithmetic relations Functions, see also special functions

Structure

Many areas of mathematics study the structure that an object has. Most of these areas are part of the study of algebra.
Number theory Abstract algebra Linear algebra Order theory Graph theory

Shape

Some areas of mathematics study the shapes of things. Most of these areas are part of the study of geometry.
Topology Geometry Trigonometry Differential geometry Fractal geometry

Change

Some areas of mathematics study the way things change. Most of these areas are part of the study of analysis.
Calculus Vector calculus Analysis
Differential equations Dynamical systems Chaos theory

Applied mathematics

Applied mathematics uses mathematics to solve problems of other areas such as engineering, physics, and computing.
Numerical analysis – Optimization – Probability theory – Statistics – Mathematical finance – Game theory – Mathematical physics – Fluid dynamics - computational algorithms

Famous theorems

These theorems have interested mathematicians and people who are not mathematicians.

Pythagorean theorem – Fermat's last theorem – Goldbach's conjecture – Twin Prime Conjecture – Gödel's incompleteness theorems – Poincaré conjecture – Cantor's diagonal argument – Four color theorem – Zorn's lemma – Euler's Identity – Church-Turing thesis

These are theorems and conjectures that have greatly changed mathematics.

Riemann hypothesis – Continuum hypothesis – P Versus NP – Pythagorean theorem – Central limit theorem – Fundamental theorem of calculus – Fundamental theorem of algebra – Fundamental theorem of arithmetic – Fundamental theorem of projective geometry – classification theorems of surfaces – Gauss-Bonnet theorem – Fermat's last theorem - Kantorovich theorem

Foundations and methods

Progress in understanding the nature of mathematics also influences the way mathematicians study their subject.

Philosophy of Mathematics – Mathematical intuitionism – Mathematical constructivism – Foundations of mathematics – Set theory – Symbolic logic – Model theory – Category theory – Logic – Reverse Mathematics – Table of mathematical symbols

History and the world of mathematicians

Mathematics in history, and the history of mathematics.

History of mathematics – Timeline of mathematics – Mathematicians – Fields medal – Abel Prize – Millennium Prize Problems (Clay Math Prize) – International Mathematical Union – Mathematics competitions – Lateral thinking – Mathematics and gender

Awards in mathematics

There is no Nobel prize in mathematics. Mathematicians can receive the Abel prize and the Fields Medal for important works.

The Clay Mathematics Institute has said it will give one million dollars to anyone who solves one of the Millennium Prize Problems.

Mathematical tools

There are many tools that are used to do mathematics or to find answers to mathematics problems.

Older tools
  • Abacus
  • Napier's bones, slide rule
  • Ruler and Compass
  • Mental calculation
Newer tools
  • Calculators and computers
  • Programming languages
  • Computer algebra systems (listing)
  • Internet shorthand notation
  • statistics software (for example SPSS)
  • SAS programming language
  • R (programming language)

Related pages

  • List of mathematicians
  • Timeline of women in mathematics
  • American Mathematical Society
  • Society for Industrial and Applied Mathematics
    • EASIAM
  • Mathematics Genealogy Project
  • Mathematics Subject Classification

Images for kids

  • 3rd century BC Greek mathematician Euclid holding calipers, as imagined by Raphael in this detail from The School of Athens (1509–1511)

  • This is the Ulam spiral, which illustrates the distribution of prime numbers. The dark diagonal lines in the spiral hint at the hypothesized approximate independence between being prime and being a value of a quadratic polynomial, a conjecture now known as Hardy and Littlewood's Conjecture F.

  • The Babylonian mathematical tablet Plimpton 322, dated to 1800 BC

  • The numerals used in the Bakhshali manuscript, dated between the 2nd century BC and the 2nd century AD

  • Leonardo Fibonacci, the Italian mathematician who introduced the Hindu–Arabic numeral system invented between the 1st and 4th centuries by Indian mathematicians, to the Western World.

  • Leonhard Euler created and popularized much of the mathematical notation used today.

  • Carl Friedrich Gauss, known as the prince of mathematicians

  • Euler's identity, which Richard Feynman once called "the most remarkable formula in mathematics"

  • The front side of the Fields Medal

All content from Kiddle encyclopedia articles (including the article images and facts) can be freely used under Attribution-ShareAlike license, unless stated otherwise. Cite this article:

Mathematics Facts for Kids. Kiddle Encyclopedia.

Mathematics Facts for Kids

Kids Encyclopedia Facts

A page from al-Khwārizmī's Algebra

Mathematics is the study of numbers, shapes and patterns. The word comes from the Greek word "μάθημα" (máthema), meaning "science, knowledge, or learning", and is sometimes shortened to maths (in England, Australia, Ireland, and New Zealand) or math (in the United States and Canada). The short words are often used for arithmetic, geometry or simple algebra by students and their schools.

Mathematics includes the study of:

  • Numbers: how things can be counted.
  • Structure: how things are organized. This subfield is usually called algebra.
  • Place: where things are and their arrangement. This subfield is usually called geometry.
  • Change: how things become different. This subfield is usually called analysis.

Mathematics is useful for solving problems that occur in the real world, so many people besides mathematicians study and use mathematics. Today, some mathematics is needed in many jobs. People working in business, science, engineering, and construction need some knowledge of mathematics.

Contents

  • Problem-solving in mathematics
  • Areas of study in mathematics
    • Number
    • Structure
    • Shape
    • Change
    • Applied mathematics
  • Famous theorems
  • Foundations and methods
    • History and the world of mathematicians
  • Awards in mathematics
  • Mathematical tools
  • Related pages
  • Images for kids

Problem-solving in mathematics

Mathematics solves problems by using logic. One of the main tools of logic used by mathematicians is deduction. Deduction is a special way of thinking to discover and prove new truths using old truths. To a mathematician, the reason something is true (called a proof) is just as important as the fact that it is true, and this reason is often found using deduction. Using deduction is what makes mathematics thinking different from other kinds of scientific thinking, which might rely on experiments or on interviews.

Logic and reasoning are used by mathematicians to create general rules, which are an important part of mathematics. These rules leave out information that is not important so that a single rule can cover many situations. By finding general rules, mathematics solves many problems at the same time as these rules can be used on other problems. These rules can be called theorems (if they have been proved) or conjectures (if it is not known if they are true yet). Most mathematicians use non-logical and creative reasoning in order to find a logical proof.

Sometimes, mathematics finds and studies rules or ideas that we don't understand yet. Often in mathematics, ideas and rules are chosen because they are considered simple or neat. On the other hand, sometimes these ideas and rules are found in the real world after they are studied in mathematics; this has happened many times in the past. In general, studying the rules and ideas of mathematics can help us understand the world better. Some examples of math problems are addition, subtraction, multiplication, division, calculus, fractions and decimals. Algebra problems are solved by evaluating certain variables. A calculator answers every math problem in the four basic arithmetic operations.

Areas of study in mathematics

Number

Mathematics includes the study of numbers and quantities.It is a branch of science the deals with logic of shape,quantity and arrangement. Most of the areas listed below are studied in many different fields of mathematics, including set theory and mathematical logic. The study of number theory usually focuses more on the structure and behavior of the integers rather than on the actual foundations of numbers themselves, and so is not listed in this given subsection.
Natural numbers Integers Rational numbers Real numbers Complex numbers
Ordinal numbers Cardinal numbers Arithmetic operations Arithmetic relations Functions, see also special functions

Structure

Many areas of mathematics study the structure that an object has. Most of these areas are part of the study of algebra.
Number theory Abstract algebra Linear algebra Order theory Graph theory

Shape

Some areas of mathematics study the shapes of things. Most of these areas are part of the study of geometry.
Topology Geometry Trigonometry Differential geometry Fractal geometry

Change

Some areas of mathematics study the way things change. Most of these areas are part of the study of analysis.
Calculus Vector calculus Analysis
Differential equations Dynamical systems Chaos theory

Applied mathematics

Applied mathematics uses mathematics to solve problems of other areas such as engineering, physics, and computing.
Numerical analysis – Optimization – Probability theory – Statistics – Mathematical finance – Game theory – Mathematical physics – Fluid dynamics - computational algorithms

Famous theorems

These theorems have interested mathematicians and people who are not mathematicians.

Pythagorean theorem – Fermat's last theorem – Goldbach's conjecture – Twin Prime Conjecture – Gödel's incompleteness theorems – Poincaré conjecture – Cantor's diagonal argument – Four color theorem – Zorn's lemma – Euler's Identity – Church-Turing thesis

These are theorems and conjectures that have greatly changed mathematics.

Riemann hypothesis – Continuum hypothesis – P Versus NP – Pythagorean theorem – Central limit theorem – Fundamental theorem of calculus – Fundamental theorem of algebra – Fundamental theorem of arithmetic – Fundamental theorem of projective geometry – classification theorems of surfaces – Gauss-Bonnet theorem – Fermat's last theorem - Kantorovich theorem

Foundations and methods

Progress in understanding the nature of mathematics also influences the way mathematicians study their subject.

Philosophy of Mathematics – Mathematical intuitionism – Mathematical constructivism – Foundations of mathematics – Set theory – Symbolic logic – Model theory – Category theory – Logic – Reverse Mathematics – Table of mathematical symbols

History and the world of mathematicians

Mathematics in history, and the history of mathematics.

History of mathematics – Timeline of mathematics – Mathematicians – Fields medal – Abel Prize – Millennium Prize Problems (Clay Math Prize) – International Mathematical Union – Mathematics competitions – Lateral thinking – Mathematics and gender

Awards in mathematics

There is no Nobel prize in mathematics. Mathematicians can receive the Abel prize and the Fields Medal for important works.

The Clay Mathematics Institute has said it will give one million dollars to anyone who solves one of the Millennium Prize Problems.

Mathematical tools

There are many tools that are used to do mathematics or to find answers to mathematics problems.

Older tools
  • Abacus
  • Napier's bones, slide rule
  • Ruler and Compass
  • Mental calculation
Newer tools
  • Calculators and computers
  • Programming languages
  • Computer algebra systems (listing)
  • Internet shorthand notation
  • statistics software (for example SPSS)
  • SAS programming language
  • R (programming language)

Related pages

  • List of mathematicians
  • Timeline of women in mathematics
  • American Mathematical Society
  • Society for Industrial and Applied Mathematics
    • EASIAM
  • Mathematics Genealogy Project
  • Mathematics Subject Classification

Images for kids

  • 3rd century BC Greek mathematician Euclid holding calipers, as imagined by Raphael in this detail from The School of Athens (1509–1511)

  • This is the Ulam spiral, which illustrates the distribution of prime numbers. The dark diagonal lines in the spiral hint at the hypothesized approximate independence between being prime and being a value of a quadratic polynomial, a conjecture now known as Hardy and Littlewood's Conjecture F.

  • The Babylonian mathematical tablet Plimpton 322, dated to 1800 BC

  • The numerals used in the Bakhshali manuscript, dated between the 2nd century BC and the 2nd century AD

  • Leonardo Fibonacci, the Italian mathematician who introduced the Hindu–Arabic numeral system invented between the 1st and 4th centuries by Indian mathematicians, to the Western World.

  • Leonhard Euler created and popularized much of the mathematical notation used today.

  • Carl Friedrich Gauss, known as the prince of mathematicians

  • Euler's identity, which Richard Feynman once called "the most remarkable formula in mathematics"

  • The front side of the Fields Medal

All content from Kiddle encyclopedia articles (including the article images and facts) can be freely used under Attribution-ShareAlike license, unless stated otherwise. Cite this article:

Mathematics Facts for Kids. Kiddle Encyclopedia.

What is mathematics - INFORMAT

Mathematics is the queen of all sciences
Gauss Carl Friedrich

Mathematics is a science historically based on solving problems about the quantitative and spatial relationships of the real world by idealizing the properties of objects necessary for this and formalizing these problems. The science concerned with the study of numbers, structures, spaces, and transformations.

Generally, people think that mathematics is just arithmetic, that is, the study of numbers and operations with them, such as multiplication and division. In fact, mathematics is much more than that. It is a way of describing the world and how one part of it fits into another. Relationships of numbers are expressed in mathematical symbols that describe the universe in which we live. Any normal child can do well in math because “number sense” is an inborn ability. True, for this you need to make some efforts and spend a little time.

The ability to count is not everything. The child needs to be able to express his thoughts well in order to understand tasks and make connections between the facts that are stored in memory. In order to learn the multiplication table, you need memory and speech. This is why some people with brain damage find it difficult to multiply, although other types of counting are not difficult for them.

Knowing geometry well and understanding shape and space requires other kinds of thinking. With the help of mathematics, we solve problems in life, for example, dividing a chocolate bar equally or finding the right shoe size. Thanks to the knowledge of mathematics, the child knows how to save pocket money and understands what can be bought and how much money he will have left. Mathematics is also the ability to count the right amount of seeds and sow them in a pot, measure out the right amount of flour for a cake or fabric on a dress, understand the score of a football game and many other daily activities. Everywhere: in the bank, in the store, at home, at work - we need the ability to understand and handle numbers, shapes and measures. Numbers are only part of a special mathematical language, and the best way to learn any language is to practice it. And it is better to start from an early age.

About mathematics “cleverly”

Usually idealized properties of objects and processes under study are formulated as axioms, then other true properties (theorems) are deduced from them according to strict rules of logical inference. This theory together forms a mathematical model of the object under study. That. Initially, based on spatial and quantitative relationships, mathematics receives more abstract relationships, the study of which is also the subject of modern mathematics.

Traditionally, mathematics is divided into theoretical, which performs an in-depth analysis of intra-mathematical structures, and applied, which provides its models to other sciences and engineering disciplines, and some of them occupy a borderline position to mathematics. In particular, formal logic can be considered both as part of the philosophical sciences and as part of the mathematical sciences; mechanics - both physics and mathematics; computer science, computer technology and algorithmics are both engineering and mathematical sciences, etc. There are many different definitions of mathematics in the literature.

Sections of mathematics
  • Mathematical analysis.
  • Algebra.
  • Analytical geometry.
  • Linear algebra and geometry.
  • Discrete Mathematics.
  • Mathematical logic.
  • Differential equations.
  • Differential geometry.
  • Topology.
  • Functional analysis and integral equations.
  • Theory of functions of a complex variable.
  • Partial differential equations.
  • Probability Theory.
  • Mathematical statistics.
  • Theory of random processes.
  • Calculus of variations and optimization methods.
  • Calculation methods, i.e. numerical methods.
  • Theory of numbers.
Aims and Methods

Mathematics studies imaginary, ideal objects and relationships between them using a formal language. In general, mathematical concepts and theorems do not necessarily correspond to anything in the physical world. The main task of an applied mathematician is to create a mathematical model adequate enough for the real object under study. The task of the theoretical mathematician is to provide a sufficient set of convenient means to achieve this goal.

The content of mathematics can be defined as a system of mathematical models and tools for their creation. The object model does not take into account all its features, but only the most necessary for the purposes of study (idealized). For example, when studying the physical properties of an orange, we can abstract from its color and taste and represent it (albeit not perfectly accurately) as a ball. If we need to understand how many oranges we get if we add two and three together, then we can abstract away from the form, leaving the model with only one characteristic - quantity. Abstraction and the establishment of relationships between objects in the most general form is one of the main directions of mathematical creativity.

Another direction, along with abstraction, is generalization. For example, generalizing the concept of "space" to the space of n-dimensions. The space R n , for n>3 is a mathematical invention. However, a very ingenious invention, which helps to mathematically understand complex phenomena.

The study of intra-mathematical objects, as a rule, takes place using the axiomatic method: first, a list of basic concepts and axioms is formulated for the objects under study, and then meaningful theorems are obtained from the axioms using inference rules, which together form a mathematical model.

Video lecture by Smirnov S.K. and Yashchenko I.V. "What is mathematics":

Related information:

Why do we need mathematics? Why study, the benefits of doing mathematics

Can you clearly explain to the child why he needs to do math? After all, the study of concepts, the laws of mathematics and logic, the solution of mathematical and logical problems requires mental effort. And why is it needed at all?

We reviewed a number of scientific studies and identified real evidence for the benefits of doing math.

Even if you are convinced that your child’s life will not be connected with mathematics, we recommend that you still read our article in order to at least easily answer the questions of the little “why”.

1. Mathematics develops thinking

By studying mathematics and solving problems, the child learns:

  • generalize and highlight the important;
  • analyze and systematize;
  • find patterns and establish cause-and-effect relationships;
  • reason and draw conclusions;
  • think logically, strategically and abstractly.

Just as regular sports training “pumps” the body, makes it healthy, strong and resilient, so regular math exercises “pump” the brain - develop intelligence and cognitive abilities, broaden horizons.

See also: In the article "5 reasons to learn to think like a mathematician" we have analyzed in detail what is the power of mathematical thinking and why to develop it.

2. Mathematics trains memory

Scientists from Stanford University in the USA studied the process of solving mathematical problems by a person and found out that adults use thinking and the skill, brought to automatism, to “get” the answers already there from memory.

Children under 7 often resort to the help of fingers and toes, as well as various substitutes (real objects, counting sticks). In the "transitional period", at the age of 7 to 9, schoolchildren form the "adult" skill of "thinking", comprehending and remembering information.

An interesting study was published in the journal Nature Neuroscience in 2014. First of all, it was devoted to the study of the role of the hippocampus (an area in the brain) in the development of cognitive activity in children. But his indirect conclusions are as follows:

  • if you want your child to have no problems with math at school, train your memory at an early age;
  • solving mathematical problems develops memory.

3. Math builds character

For the correct solution of mathematical and logical problems, attentiveness, perseverance, responsibility, accuracy and accuracy are needed.

The more regularly a child trains these "muscles of character", the stronger they become, the more often they help the child in solving not only educational problems, but also life problems.

LogicLike is the right training platform for 20-60 minutes a day. Solve problems, participate in olympiads in logic and mathematics, develop the will to win and the ability to win!

We create both simple and Olympiad problems that you want to solve:

  • tasks for grade 1;
  • assignments for grade 2;
  • assignments for grade 3.

4. Music for mathematics, mathematics for music

A comprehensive study by Barbara H. Helmrich of the College of Notre Dame in Baltimore found that children who played musical instruments in middle school did significantly better in math in high school.

Scientists have found that the same part of the brain is responsible for solving algebraic problems and processing musical information.

"The largest average difference in algebra scores between any two groups of subjects was found between African-American 'instrumental' groups and groups of 'non-musical' students."

Paradoxically, scientists didn't seem to be interested in feedback.
After all, if the same part of the brain is responsible for the development of mathematical and musical abilities, it is possible that doing mathematics improves musical abilities.

I remember Sherlock Holmes, who was both an excellent detective and a talented violinist. Many will say that the famous English detective is just a fiction, but he had his own real prototype, a mentor and friend of Arthur Conan Doyle. The greatest physicist Albert Einstein was also a passionate violinist.

5. Math Helps You Succeed in the Humanities

It is early mathematical abilities that are a sure prerequisite for the fact that in the future the child will not only understand mathematics well, but also succeed in other school disciplines. Next in importance for contributing to academic success are reading skills and the ability to manage one's attention.

Such conclusions were reached by scientists in the field of education and social policy at Northwestern University in Evanston. During the study, they assessed the relationship of key elements of school readiness (basic skills for school admission - "academic" readiness, attention, social-emotional skills) with further academic success.

Mathematics is an interdisciplinary science, it is closely related to physics, geography, geology, and chemistry. Sociology and economics are inseparable from mathematics, and many of the conclusions of even the usual humanities, such as linguistics, journalism, are based on mathematical models and concepts, mathematical and logical laws.

6. Develops skills for solving everyday problems

Barbara Oakley, PhD, brain stem cell researcher and author of Think Like a Mathematician, emphasizes:

“Mathematics saves us from “magical thinking” - we strive to delve into the essence of things and do not rely on chance and higher powers. ”

The more difficult the math problems become, the more skills are required to solve them. The child learns to reason, build sequences, think through algorithms, juggle several concepts at once, and these skills become a habit.

Thanks to mathematics, we get rid of bad habits:

  • we do not think, but we operate only in exact terms;
  • we do not just memorize information and rules mechanically, but evaluate it, analyze it, reflect in order to understand and learn new material, a new life lesson.

7. Mathematics is the basis of a successful career

If 10-15 years ago the study of foreign languages ​​was considered promising, now you will not surprise anyone with fluency in several languages. Now professional demand largely depends on the understanding of technology, the ability to think, abstract and the ability to solve non-standard problems. It is extremely difficult for those who want to work in the IT field to do without knowledge of mathematics.

Abstract, critical and strategic thinking, analytical skills, the ability to build algorithms are a “must-have” for a good developer.

TOP 5 soft skills. Source: amazonaws.com

Successful mathematics classes give self-confidence, because success in it requires perseverance in striving to solve the most complex, sometimes, at first glance, “unsolvable” tasks and problems.

Test your strength: Math puzzles to help you: 9 selected well-known tasks for ingenuity. How many can you decide?

8. Problem Solving Builds Mental Resilience

Solving mathematical problems helps to improve the emotional background - this activity can relieve anxiety, helps control emotions and prevents stress.

These conclusions were reached by scientists from Duke University in the United States, who were able to prove this in a study published in the journal Clinical Psychology in 2016.

9. Pleasure from "x"

For a serious student of mathematics, mathematical formulas, equations and other logical and mathematical problems embody beauty, harmony and provide the same aesthetic pleasure as music, art and a good joke, says a group of researchers from several universities in the UK.

With the help of functional magnetic resonance imaging, the activity of the brain activity of the subjects was recorded during the demonstration of mathematical equations, formulas and tasks. The results of the study were published in the journal Frontiers in Human Neuroscience in 2014.

How to learn to experience the joy and pleasure of doing mathematics tells the famous American mathematician, a graduate of Harvard University, Stephen Strogatz. Applied Mathematics teacher, award-winning math and teacher in the pages of his book "The Pleasure of X" enthusiastically explains the most significant mathematical ideas in a simple and understandable way.

Try logic and math classes at LogicLike.com!

We are convinced that children, especially those aged 5-9, do not need to be told how important it is to study mathematics. It is much more important to give the child the opportunity to plunge into the world of entertaining interactive mathematics.

Learning on the LogicLike platform, children solve interesting logical problems, earn their first "star" awards for correct answers, play modern logic games - and get not only benefit, but also real pleasure from such mathematics.

Learn more