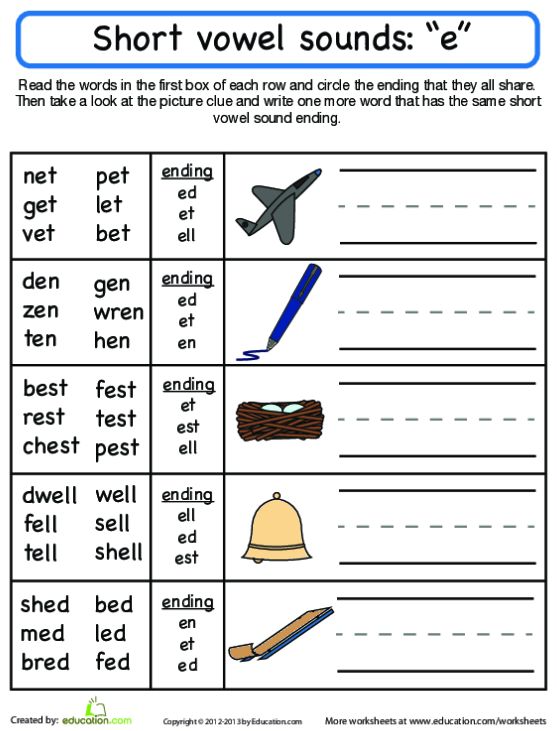
Children learn math
Help Your Child Develop Early Math Skills
Before they start school, most children develop an understanding of addition and subtraction through everyday interactions. Learn what informal activities give children a head start on early math skills when they start school.
Children are using early math skills throughout their daily routines and activities. This is good news as these skills are important for being ready for school. But early math doesn’t mean taking out the calculator during playtime. Even before they start school, most children develop an understanding of addition and subtraction through everyday interactions. For example, Thomas has two cars; Joseph wants one. After Thomas shares one, he sees that he has one car left (Bowman, Donovan, & Burns, 2001, p. 201). Other math skills are introduced through daily routines you share with your child—counting steps as you go up or down, for example. Informal activities like this one give children a jumpstart on the formal math instruction that starts in school.
What math knowledge will your child need later on in elementary school? Early mathematical concepts and skills that first-grade mathematics curriculum builds on include: (Bowman et al., 2001, p. 76).
- Understanding size, shape, and patterns
- Ability to count verbally (first forward, then backward)
- Recognizing numerals
- Identifying more and less of a quantity
- Understanding one-to-one correspondence (i.e., matching sets, or knowing which group has four and which has five)
Key Math Skills for School
More advanced mathematical skills are based on an early math “foundation”—just like a house is built on a strong foundation. In the toddler years, you can help your child begin to develop early math skills by introducing ideas like: (From Diezmann & Yelland, 2000, and Fromboluti & Rinck, 1999.)
Number Sense
This is the ability to count accurately—first forward. Then, later in school, children will learn to count backwards. A more complex skill related to number sense is the ability to see relationships between numbers—like adding and subtracting. Ben (age 2) saw the cupcakes on the plate. He counted with his dad: “One, two, three, four, five, six…”
Representation
Making mathematical ideas “real” by using words, pictures, symbols, and objects (like blocks). Casey (aged 3) was setting out a pretend picnic. He carefully laid out four plastic plates and four plastic cups: “So our whole family can come to the picnic!” There were four members in his family; he was able to apply this information to the number of plates and cups he chose.
Spatial sense
Later in school, children will call this “geometry.” But for toddlers it is introducing the ideas of shape, size, space, position, direction and movement. Aziz (28 months) was giggling at the bottom of the slide. “What’s so funny?” his Auntie wondered. “I comed up,” said Aziz, “Then I comed down!”
Measurement
Technically, this is finding the length, height, and weight of an object using units like inches, feet or pounds. Measurement of time (in minutes, for example) also falls under this skill area. Gabriella (36 months) asked her Abuela again and again: “Make cookies? Me do it!” Her Abuela showed her how to fill the measuring cup with sugar. “We need two cups, Gabi. Fill it up once and put it in the bowl, then fill it up again.”
Estimation
This is the ability to make a good guess about the amount or size of something. This is very difficult for young children to do. You can help them by showing them the meaning of words like more, less, bigger, smaller, more than, less than. Nolan (30 months) looked at the two bagels: one was a regular bagel, one was a mini-bagel. His dad asked: “Which one would you like?” Nolan pointed to the regular bagel. His dad said, “You must be hungry! That bagel is bigger. That bagel is smaller. Okay, I’ll give you the bigger one. Breakfast is coming up!”
Patterns
Patterns are things—numbers, shapes, images—that repeat in a logical way. Patterns help children learn to make predictions, to understand what comes next, to make logical connections, and to use reasoning skills.
Ava (27 months) pointed to the moon: “Moon. Sun go night-night.” Her grandfather picked her up, “Yes, little Ava. In the morning, the sun comes out and the moon goes away. At night, the sun goes to sleep and the moon comes out to play. But it’s time for Ava to go to sleep now, just like the sun.”
Problem-solving
The ability to think through a problem, to recognize there is more than one path to the answer. It means using past knowledge and logical thinking skills to find an answer. Carl (15 months old) looked at the shape-sorter—a plastic drum with 3 holes in the top. The holes were in the shape of a triangle, a circle and a square. Carl looked at the chunky shapes on the floor. He picked up a triangle. He put it in his month, then banged it on the floor. He touched the edges with his fingers. Then he tried to stuff it in each of the holes of the new toy. Surprise! It fell inside the triangle hole! Carl reached for another block, a circular one this time…
Math: One Part of the Whole
Math skills are just one part of a larger web of skills that children are developing in the early years—including language skills, physical skills, and social skills. Each of these skill areas is dependent on and influences the others.
Trina (18 months old) was stacking blocks. She had put two square blocks on top of one another, then a triangle block on top of that. She discovered that no more blocks would balance on top of the triangle-shaped block. She looked up at her dad and showed him the block she couldn’t get to stay on top, essentially telling him with her gesture, “Dad, I need help figuring this out.” Her father showed her that if she took the triangle block off and used a square one instead, she could stack more on top. She then added two more blocks to her tower before proudly showing her creation to her dad: “Dada, Ook! Ook!”
You can see in this ordinary interaction how all areas of Trina’s development are working together. Her physical ability allows her to manipulate the blocks and use her thinking skills to execute her plan to make a tower. She uses her language and social skills as she asks her father for help. Her effective communication allows Dad to respond and provide the helps she needs (further enhancing her social skills as she sees herself as important and a good communicator). This then further builds her thinking skills as she learns how to solve the problem of making the tower taller.
What You Can Do
The tips below highlight ways that you can help your child learn early math skills by building on their natural curiosity and having fun together. (Note: Most of these tips are designed for older children—ages 2–3. Younger children can be exposed to stories and songs using repetition, rhymes and numbers.)
Shape up.
Play with shape-sorters. Talk with your child about each shape—count the sides, describe the colors. Make your own shapes by cutting large shapes out of colored construction paper. Ask your child to “hop on the circle” or “jump on the red shape.”
Count and sort.
Gather together a basket of small toys, shells, pebbles or buttons. Count them with your child. Sort them based on size, color, or what they do (i.e., all the cars in one pile, all the animals in another).
Place the call.
With your 3-year-old, begin teaching her the address and phone number of your home. Talk with your child about how each house has a number, and how their house or apartment is one of a series, each with its own number.
What size is it?
Notice the sizes of objects in the world around you: That pink pocketbook is the biggest. The blue pocketbook is the smallest. Ask your child to think about his own size relative to other objects (“Do you fit under the table? Under the chair?”).
You’re cookin’ now!
Even young children can help fill, stir, and pour. Through these activities, children learn, quite naturally, to count, measure, add, and estimate.
Walk it off.
Taking a walk gives children many opportunities to compare (which stone is bigger?), assess (how many acorns did we find?), note similarities and differences (does the duck have fur like the bunny does?) and categorize (see if you can find some red leaves). You can also talk about size (by taking big and little steps), estimate distance (is the park close to our house or far away?), and practice counting (let’s count how many steps until we get to the corner).
Picture time.
Use an hourglass, stopwatch, or timer to time short (1–3 minute) activities. This helps children develop a sense of time and to understand that some things take longer than others.
Shape up.
Point out the different shapes and colors you see during the day. On a walk, you may see a triangle-shaped sign that’s yellow. Inside a store you may see a rectangle-shaped sign that’s red.
Read and sing your numbers.
Sing songs that rhyme, repeat, or have numbers in them. Songs reinforce patterns (which is a math skill as well). They also are fun ways to practice language and foster social skills like cooperation.
Start today.
Use a calendar to talk about the date, the day of the week, and the weather. Calendars reinforce counting, sequences, and patterns. Build logical thinking skills by talking about cold weather and asking your child: What do we wear when it’s cold? This encourages your child to make the link between cold weather and warm clothing.
Pass it around.
Ask for your child’s help in distributing items like snacks or in laying napkins out on the dinner table. Help him give one cracker to each child. This helps children understand one-to-one correspondence. When you are distributing items, emphasize the number concept: “One for you, one for me, one for Daddy.” Or, “We are putting on our shoes: One, two.”
Big on blocks.
Give your child the chance to play with wooden blocks, plastic interlocking blocks, empty boxes, milk cartons, etc. Stacking and manipulating these toys help children learn about shapes and the relationships between shapes (e.g., two triangles make a square). Nesting boxes and cups for younger children help them understand the relationship between different sized objects.
Tunnel time.
Open a large cardboard box at each end to turn it into a tunnel. This helps children understand where their body is in space and in relation to other objects.
The long and the short of it.
Cut a few (3–5) pieces of ribbon, yarn or paper in different lengths. Talk about ideas like long and short. With your child, put in order of longest to shortest.
Learn through touch.
Cut shapes—circle, square, triangle—out of sturdy cardboard. Let your child touch the shape with her eyes open and then closed.
Pattern play.
Have fun with patterns by letting children arrange dry macaroni, chunky beads, different types of dry cereal, or pieces of paper in different patterns or designs. Supervise your child carefully during this activity to prevent choking, and put away all items when you are done.
Laundry learning.
Make household jobs fun. As you sort the laundry, ask your child to make a pile of shirts and a pile of socks. Ask him which pile is the bigger (estimation). Together, count how many shirts. See if he can make pairs of socks: Can you take two socks out and put them in their own pile? (Don’t worry if they don’t match! This activity is more about counting than matching.)
Playground math.
As your child plays, make comparisons based on height (high/low), position (over/under), or size (big/little).
Dress for math success.
Ask your child to pick out a shirt for the day. Ask: What color is your shirt? Yes, yellow. Can you find something in your room that is also yellow? As your child nears three and beyond, notice patterns in his clothing—like stripes, colors, shapes, or pictures: I see a pattern on your shirt. There are stripes that go red, blue, red, blue. Or, Your shirt is covered with ponies—a big pony next to a little pony, all over your shirt!
Graphing games.
As your child nears three and beyond, make a chart where your child can put a sticker each time it rains or each time it is sunny. At the end of a week, you can estimate together which column has more or less stickers, and count how many to be sure.
References
Bowman, B.T., Donovan, M.S., & Burns, M.S., (Eds.). (2001). Eager to learn: Educating our preschoolers. Washington, DC: National Academy of Sciences.
Diezmann, C., & Yelland, N. J. (2000). Developing mathematical literacy in the early childhood years. In Yelland, N.J. (Ed.), Promoting meaningful learning: Innovations in educating early childhood professionals. (pp.47–58). Washington, DC: National Association for the Education of Young Children.
Fromboluti, C. S., & Rinck, N. (1999 June). Early childhood: Where learning begins. U.S. Department of Education, Office of Educational Research and Improvement, National Institute on Early Childhood Development and Education. Retrieved on May 11, 2018 from https://www2.ed.gov/pubs/EarlyMath/title.html
| Helping Your Child Learn Mathematics With activities for children in preschool through grade 5 This booklet is made up of fun activities that parents can use with children from preschool age through grade 5 to strengthen their math skills and build strong positive attitudes toward math.
Table of Contents
Last Modified: 02/27/2009 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Why do children study mathematics at school? — Math Assistant
Over the years, children, and then as adults, have invariably asked the question: Why should their children study mathematics in school?
I'll try to explain in as much detail as possible.
First, dear parents, let's not forget that we have a mass education, and we teach many different, unique, amazing children at the same time. Perhaps you think that your child will not need math for a future career. Perhaps you think that there is no need for a child to deal with complex subjects in order not to immerse his brain, but to do what he likes. You will be right, in part...
The classroom system, which originated in the 17th century, gave us the opportunity to teach millions of children every year. The system of various lessons allows the student to reveal his abilities and identify inclinations. The exclusion of any subject from the general education program entails devastating consequences both for society and for the individual. If we allow students in the same class to study many subjects, including non-practical ones, then we will give them the opportunity to become who they can be. nine0003
One student can become a mathematician and prove the Riemann Hypothesis (https://ru. wikipedia.org/wiki/Riemann_Hypothesis). One student can become a programmer and develop their own software provision for different areas of activity. One student can become a doctor and solve the problem of chronic diseases, which are rapidly declining population (e.g. cardiovascular disease). One student can be a good psychiatrist and make a discovery in the field of treatment of depression and addictions that "conquer the world" like an avalanche. One student will become a physicist and unravel the mysteries of "dark matter". One student will become teachers and come up with such a learning technology in which everyone will study with pleasure)) Others students can be good pilots, engineers, librarians, builders, testers, philosophers, and so on, the list is endless. But, one must understand that first, all students must go through a stage of collective, mass learning. AND, say: “My child does not need this (mathematics), a little selfish in towards other students in the class. Let's respect that educational system that has developed for our era and try to become successful in it !!! And you can also remember that some 150 years ago in Russia, a literate population was it all? percent of the population.
So, what a good life we live with! nine0003
Secondly, let's continue the first paragraph, I will talk about how why many higher education institutions require results upon admission USE in mathematics. In short, for further professional training. For a long time in our country the paradigm of "fundamental education”, the essence of which boiled down to the fact that the fundamentals of sciences were taught for every specialty. This made it possible not only to form professional ability to perform certain activities, but also to understand where having knowledge, what ways of discovering this knowledge exist and how transform this knowledge for modern realities. Therefore, the mathematical training was in the institutes of culture, and in medical institutes, and in humanitarian institutions. nine0003
Aims of mathematics education in various higher education institutions associated with the concept of "mathematical method", a very complex and not quite a certain concept. The essence of which is that in the study of any object, you have to resort to the apparatus of mathematics to calculate the values considered value, to assess and predict its behavior. Application mathematical apparatus for the study of objects of different nature, whether medical, economic, historical, political or psychological, should be objective, regardless of someone's consciousness, desire or opinion. nine0003
To make it clear: one person is helped by plantain from a headache pain, but this does not mean that from now on doctors will start prescribing everything for those who complain of headache patients plantain. Because it is knowledge and a way not objective. We want to use only those medicines that are scientifically proven and tested, i.e. scientific experiments were carried out.
And what is included in a scientific experiment and its substantiation?!?! Correctly! Mathematical methods for processing the information collected during the experiment. You say: “Well, it’s clear with drugs, but why experiment with people? This is where the fun begins. nine0003
Would you like to be tested by an unknown person during the interview? test, which is a set of some questions or yet a qualitative technique that will reflect all your advantages and disadvantages (of which you certainly don't). The answer is obvious: We all want everyone in our was, scientifically, qualitatively, substantiated! And who should do it? Naturally experts familiar with mathematical methods! Where do they come from experts? Studying in higher education!
As an example, consider the activities of psychologists. For solutions many scientific and practical problems of psychology resort to mathematical methods: use the apparatus of linear algebra to compile sociomatrices, use apparatus of mathematical statistics for checking the effectiveness of activities teachers. Let's take a few examples. Are there significant differences between those who study a foreign language in regular classes, but more hours and those who study fewer hours, but in a language school. How much strong influence of self-control on the creative productivity of the student art school. What factors have the greatest influence on the development emotional intelligence in children? nine0003
Consider another example of very creative professionals - advertising and public relations professionals. In vocational training considers mathematical methods for conducting surveys and correct analysis responses received; mathematical modeling of an advertising company in different conditions. Well, if more practical, then mathematics is always present at advertising budget calculation. Higher education is higher for that, to know how calculation formulas and algorithms are created, what are their advantages and disadvantages. nine0003
Thirdly, mathematics contributes to the development of the student's intellect. You, probably everyone heard the phrase of Lomonosov “Mathematics already needs to be taught that she puts her mind in order. What is meant? Without mathematics, the mind will not be in okay? What is order anyway?
In fact, doing mathematical activities, especially geometry (yes, don’t be surprised, she has the most powerful developmental potential), on the development of certain qualities of intelligence (make a link to an article about intelligence). In a nutshell, when doing mathematics, a student develops skills:
- use different ways of encoding, storing, transformation and reproduction of information;
- exercise control over their own intellectual activity;
- make decisions following a rational path.
More details in the article devoted to the research of M.A. Cold. When a person makes decisions, he can either rely on feelings (I don't know why I do this, I just feel this way) or on the mind (I do this, because it is the most correct way in the circumstances). Familiar feelings, right? Both the first and second paths are, of course, extremes. The mind must be at the service of the soul. For many years, we accustom our thinking work according to certain rules. Such as: what is known, what data significant, which mono to neglect, a familiar situation or I have not dealt with it before encountered that I need to find the answer to the question that I help you find these tools, try it and evaluate the result ...
What does it matter what our "great and mighty brain" does: decides algebraic problem, puts an experiment in physics, or ponders how to enter difficult situation?
Intellectual skills (make reference to the method of formation intellectual skills) are formed over time, therefore regular classes in mathematics, teach the student to perceive the problem as mathematical problem, and accordingly perform sequential actions: “analysis of the problem situation → problem statement → search for the missing information and hypotheses → testing hypotheses and obtaining new knowledge → transfer of a problem into a task (or several tasks) → search for a solution → solution → verification of the solution → proof of the correctness of the solution → conclusion”. nine0003
So, to summarize... Mathematics is studied at school because:
— in conditions of mass education, everyone items;
— mathematics is necessary for the development of mathematical methods in future professional (mainly scientific) activities mastered in higher educational institutions;
- Mathematical activities develop the student, enriching his intellect skills to compare, generalize, classify, abstract, to reason from the particular to the particular and from the particular to the particular, do unique mindset of the student and forms the ability to rationally approach the solution problems. nine0003
After all, to live means to solve problems!!!!
Why is it necessary to study mathematics with a child
Why is it necessary to study mathematics? Every child asks such a question to his parents, not fully understanding how such a complex subject is useful in life. In this article we talk about the role of learning mathematics in the development of a child and explain how to awaken motivation for classes.
⠀
The role of mathematics is really huge in our life. Without this science, the world would be different. If it did not develop, there would be no great discoveries, there would not be those things that we use today. Now more specifically, why you need to love counting from the cradle. nine0003
1. Mathematics trains memory
Memory is one of the most important functions of the psyche, on which other processes are built. Therefore, from early childhood, the child must be taught the ways and techniques of effective memorization of information.
⠀
Early math lessons that are related to counting will help with this. There is an opinion that if children count well, then they have a mathematical mindset. But is it? Not really. Memory and thought processes develop only if the counting is done consciously, and not mechanically. nine0003
⠀
Until the age of 7, children use their fingers or various objects to count. But this skill alone will not develop mathematical thinking. It is the solution of problems that trains the brain and memory, develops the flexibility of the mind, helping to understand the relationship and cover the whole problem in order to find non-standard ways out of various situations.
⠀
Conclusion: solve problems with your child to develop memory.
2. Mathematics teaches you to think
Regular training in the gym strengthens the body, and constant practice of mathematics trains the mind. In the process of computing activity, the child improves:
- ability to reason and analyze;
- think logically;
- highlight the main thing;
- structure and draw conclusions.
See also: “How to develop creative thinking in a child at home? TRIZ Methods for Children from 5 Years Old»
Here is an example of home games that will help a child develop creative thinking.
3. Mathematics teaches you not to give up and not stop there
To solve a problem correctly, children need to be precise, attentive, responsible and persistent.
⠀
The more often your son or daughter pumps these skills, the smarter he will become. In the future, this will help not only with studies, but also in life.
4. Music and mathematics: where is the connection?
Did you know that the same area of the brain is responsible for the development of musical and mathematical abilities? Or that some musical hits gain popularity due to their "mathematical" structure? nine0003
For example, hip-hop wins the hearts of young people with repetitive improvisations and rhythmic beats. Such love is due to the innate need of people for repetitive elements and rhythm.
It follows that parallel studies in these two areas will help your child become the Albert Einstein or Sherlock Holmes of his time in the future.
5. Mathematics Helps You Succeed in Other Sciences
Early math classes lay the foundation on which to build skills in other school subjects. If you look at different disciplines more broadly, you can see that geography, chemistry, physics, biology, drawing are closely related to mathematics. nine0003
⠀
From observations: if a student has problems with algebra and geometry, in 95% of cases he will also have difficulties with physics and chemistry.
⠀
The conclusion is obvious - build a solid base by doing mathematics with your child from early childhood.
6. Everyday tasks seem less complicated and are solved faster
The longer and deeper the child studies mathematics, the better he copes with daily activities in all areas of life. Accurate calculations and calculations teach to reason, think through algorithms of actions and build sequences. Thanks to mathematics, the child learns to check the facts and get to the bottom of the truth, and not to speculate. Learn from life situations and draw conclusions. nine0003
⠀
Read here how to develop logical thinking and prepare a child for learning mathematics with the help of simple games.
7. The study of mathematics and the development of thinking is the key to success in a career
The modern world is an era of technological development, where IT workers are the most in demand.