Counting and numbers
Counting Numbers - Definition, Counting Chart, Examples
Counting numbers are the natural numbers that can be used in counting. They start from 1 and the series continues as 1, 2, 3, 4, and so on. Zero is not included in counting numbers because we cannot count 0. Let us learn more about counting numbers in this article.
| 1. | What are Counting Numbers? |
| 2. | Counting Numbers from 1 to 20 |
| 3. | Counting Numbers from 1 to 100 |
| 4. | Counting Numbers with Hands |
| 5. | FAQs on Counting Numbers |
What are Counting Numbers?
Counting is the process of expressing the number of elements or objects that are given. Counting numbers include natural numbers which can be counted and which are always positive. Counting is essential in day-to-day life because we need to count the number of hours, the days, money, and so on. Numbers can be counted and written in words like one, two, three, four, and so on. They can be counted in order and backward too. Sometimes, we use skip counting, reverse counting, counting by 2s, counting by 5s, and many more.
Counting Numbers from 1 to 20
The counting of numbers always starts from 1 because zero cannot be counted. Let us start counting numbers from 1 to 20 and read their number names so that they can be identified.
Counting Numbers from 1 to 100
Repeated counting of numbers is very essential for children because it helps to know the sound and the order of numbers. Using simple techniques and real-life examples, like counting the number of cookies on a plate can help them memorize numbers easily. Counting numbers from 1 to 100 in words is quite helpful because it helps to grasp the number sense of increasing numbers.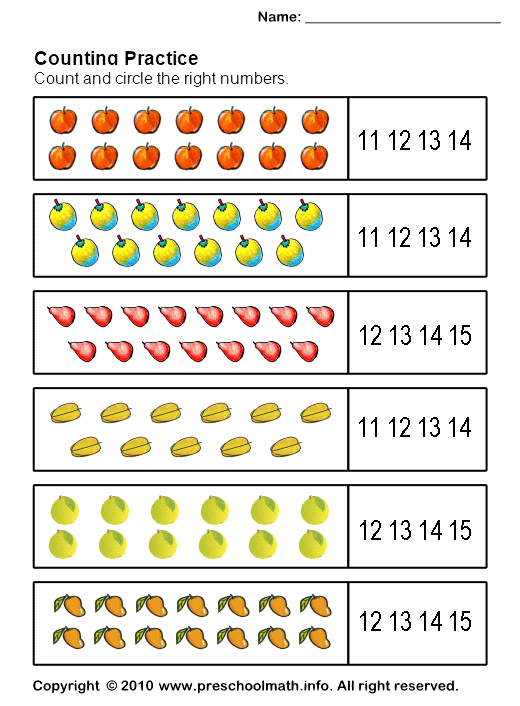 The following chart shows numbers from 1 to 100.
The following chart shows numbers from 1 to 100.
Counting in Numbers with Hands
Counting numbers from 1 to 10 can also be done using our hands and fingers. It is a known fact that visual maths helps children develop math skills faster. Using hands and fingers acts as a link between numbers and their symbolic representation. The following figure shows how the numbers from 1 to 10 can be easily represented with fingers.
Related Articles:
- Numbers
- Numbers in words
- Even Numbers
- Odd Numbers
FAQs on Counting Numbers
What are Counting Numbers in Math?
Counting numbers include natural numbers that can be counted. They start from 1 and the series continues as 1, 2, 3, 4, and so on. Zero is not included in counting numbers because we cannot count 0.
What are Counting Numbers Less than 10?
Counting numbers less than 10 can be listed as, 1, 2, 3, 4, 5, 6, 7, 8, 9.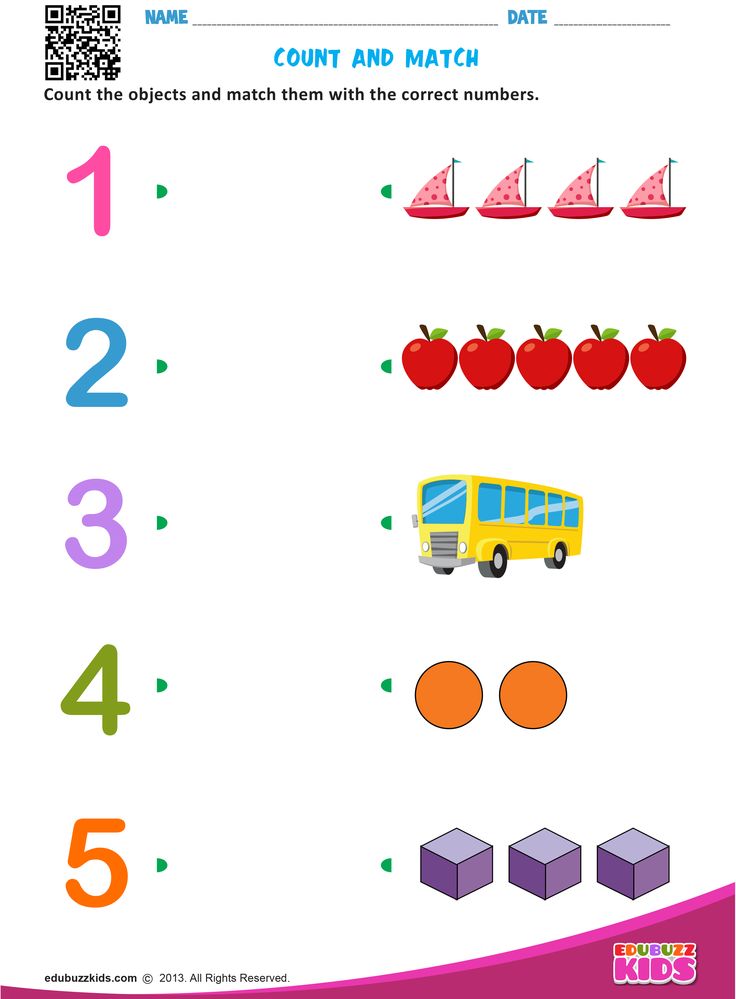
How Many Counting Numbers are there?
There is no limit for counting numbers because they start from 1 and go on till infinity, like 1, 2, 3, 4, 5, and so on. Therefore, it can be said that there are infinite counting numbers.
What Counting Numbers are also Whole Numbers?
All counting numbers are whole numbers because counting numbers start from 1, 2, 3, 4, and so on. However, it should be noted that all whole numbers are not counting numbers because whole numbers include 0, and 0 is not considered to be a counting number since it cannot be counted.
What is the mean of the first 100 Counting Numbers?
The mean of the first 100 counting numbers can be calculated by finding the sum of the first 100 counting numbers, which is, 1 + 2 + 3 +.........100. This sums up to 5050. Then, this sum is divided by the number of counting numbers, that is 100 in this case. So, 5050 ÷ 100 = 50.5. Therefore, the mean is 50.5
Give 5 examples of Counting in Numbers.
There can be many examples of counting numbers:
- Counting numbers from 1 to 5 can be listed as: 1, 2, 3, 4, 5
- Odd Counting numbers from 1 to 10 can be listed as: 1, 3, 5, 7, 9
- Even Counting numbers from 11 to 20 can be listed as: 12, 14, 16, 18, 20
- Counting numbers from 21 to 25 can be listed as: 21, 22, 23, 24, 25
- Counting numbers from 51 to 55 can be listed as: 51, 52, 53, 54, 55
What Is Another Name for Counting Numbers?
Counting numbers can also be called natural numbers because they do not include zero and are positive numbers that start from 1, 2, 3 and the series continues till infinity.
Write the Odd Counting Numbers Between 6 and 16.
Odd numbers are those numbers that are not divisible by 2. So, the odd counting numbers between 6 and 16 are 7, 9, 11, 13, 15.
Write the Even Counting Numbers less than 12.
Even numbers are those numbers that are divisible by 2. So, the even counting numbers less than 12 are 2, 4, 6, 8, and 10.
So, the even counting numbers less than 12 are 2, 4, 6, 8, and 10.
Counting Number – Definition, Count 1 to 100, Counting Chart, Examples
Counting numbers are called natural numbers, and they are named counting numbers because they are used in the counting of the numbers of objects, elements, etc. Counting numbers start from 1 and terminate at infinity. The sequence for counting numbers is 1, 2, 3, 4, and so on. Let’s learn about counting numbers in detail.
What are Counting Numbers?
Counting Numbers are defined as the set of numbers that we use to count things. Counting numbers are called natural numbers. And these numbers are always positive. Counting numbers do not include 0, and this is the reason that whole numbers cannot be called counting numbers. Examples of counting numbers are 1, 2, 3, 4, 5, … etc. Counting numbers are used in everyday life as they are required to count things, objects, money, and so on. Hence, counting numbers can not be decimals, negative numbers, integers, or fractions. Counting numbers are positive numbers, while integers contain both positive and negative numbers. Counting of numbers can be done in many different ways, for instance, reverse counting, counting by 2s (2, 4, 6,…), counting by 3s (3, 6, 9,…), counting by 5s (5, 10, 15,…), and so on. The basic rules of Counting numbers are:
Counting numbers are positive numbers, while integers contain both positive and negative numbers. Counting of numbers can be done in many different ways, for instance, reverse counting, counting by 2s (2, 4, 6,…), counting by 3s (3, 6, 9,…), counting by 5s (5, 10, 15,…), and so on. The basic rules of Counting numbers are:
- Counting numbers do not include 0.
- Counting numbers do not include fractions, e.g., 1/2, 3/4, 5/6, etc.
- Counting numbers do not include negative numbers, e.g: -1, -2, -3 etc.
- Counting number do not include decimals, e.g: 0.85, 0.67, 3.97 etc.
Counting Numbers from 1 to 20
Counting numbers are always generated from 1 because 0 is never included in the counting numbers. Let’s start counting numbers, and to begin, let’s count numbers from 1 to 20, both in digits and in words.
| 1 ⇢ | One | 11 ⇢ | Eleven |
| 2 ⇢ | Two | 12 ⇢ | Twelve |
| 3 ⇢ | Three | 13 ⇢ | Thirteen |
| 4 ⇢ | Four | 14 ⇢ | Fourteen |
| 5 ⇢ | Five | 15 ⇢ | Fifteen |
| 6 ⇢ | Six | 16 ⇢ | Sixteen |
| 7 ⇢ | Seven | 17 ⇢ | Seventeen |
| 8 ⇢ | Eight | 18 ⇢ | Eighteen |
| 9 ⇢ | Nine | 19 ⇢ | Nineteen |
| 10 ⇢ | Ten | 20 ⇢ | Twenty |
Counting Numbers From 1 to 100
We are counting numbers from 1 to 100 because to understand the order of numbers, repeated counting of numbers is important for children. It can be done in everyday life by simply counting anything lying around the house. This includes counting books, money, biscuits, or any countable thing. Counting numbers from 1 to 100 is taught so that the student understands the concept of increasing numbers and patterns more clearly. Below is the counting numbers worksheet from 1 to 100:
It can be done in everyday life by simply counting anything lying around the house. This includes counting books, money, biscuits, or any countable thing. Counting numbers from 1 to 100 is taught so that the student understands the concept of increasing numbers and patterns more clearly. Below is the counting numbers worksheet from 1 to 100:
Counting Numbers with Hands
Counting numbers with hands is a necessary practice for a better understanding of counting numbers for children. Since visual maths helps children grasp the concept easily, counting numbers with hands links the fingers to numbers, and it makes counting easier. Counting from 1 to 10 can be easily done with hands, and once this is learned properly, from 10 to 20 will be a repetition of the same fingers for counting. Below given figure shows the counting of numbers from 1 to 10 with hands.
Examples of Counting Numbers
The example of counting numbers can be found everywhere, even in everyday life.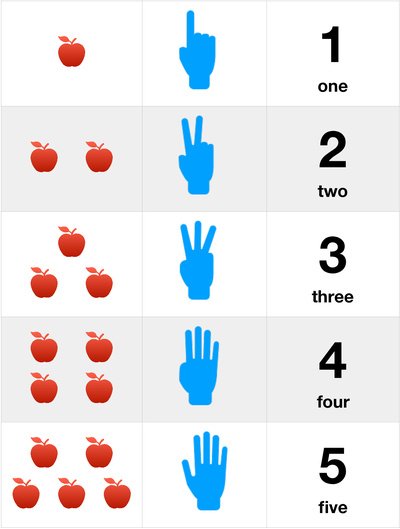 From counting the days in the year to counting the candies distributed in the class, and so on. Let’s look at some of the examples of counting numbers,
From counting the days in the year to counting the candies distributed in the class, and so on. Let’s look at some of the examples of counting numbers,
- Count for the days left for the Durga Puja vacation. (Such as 60 days)
- Count the number of books you have. (Such as – 3 Science, 1 Math, 2 English Books).
- Count for the numbers from 1 to 100. (like 1, 2, 3, 4, 5,… 10 and then for 11 to 20 and so on).
- Count for the number of students in your class. (like 30, 40).
- Count for the number of players in the Indian Cricket team (like 11 payers) and so on.
Counting Numbers Worksheet
Question 1: Complete the following worksheet by counting the numbers by 5.
- 5, ?, 15, 20, ?
- 25, 30, ?, 40
- 1000, ?, 1010, 1015, 1020
Answer:
As the numbers are counted by 5, adding 5 to the previous number will give the next number.
Therefore, the answers are:
- 5, 10, 15, 20, 25
- 25, 30, 35, 40
- 1000, 1005, 1010, 1015, 1020
Question 2: Write the counting numbers from:
- 10 to 20
- 100 to 1000 counting by 100
- 25 to 50
Answer:
- Counting numbers from 10 to 20 ⇢ 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20.
- Counting numbers from 100 to 1000 by 100 ⇢ 100, 200, 300, 400, 500, 600, 700, 800, 900, 1000.
- Counting numbers from 25 to 50 ⇢ 25, 26, 27, 28, 29, 30, 31, 32, 33, 34, 35, 36, 37, 38, 39, 40, 41, 42, 43, 44, 45, 46, 47, 48, 49, 50.
Question 3: Write 11 counting numbers from 9.
Answer:
11 counting numbers from 9 are 9, 10, 11, 12, 13, 14, 15, 16, 17.
Question 4: Write only the even counting numbers from 1 to 20.
Answer:
Even counting numbers will only include even numbers. Even numbers are the number that are completely divisible by 2 leaving 0 as a remainder. So, even counting numbers from 1 to 20 are:
2, 4, 6, 8, 10, 12, 14, 16, 18, 20
Question 5: Write the odd counting numbers from 10 to 50.
Answer:
Odd counting numbers will only include odd numbers. Odd numbers are the number that are not completely divisible by 2. So, odd counting numbers from 10 to 50 are:
11, 13, 15, 17, 19, 21, 23, 25, 27, 29, 31, 33, 35, 37, 39, 41, 43, 45, 47, 49.
FAQs on Counting Numbers
Question 1: What are counting numbers in maths?
Answer:
Counting numbers are the natural numbers and they are used for counting. Counting numbers generate from 1 and go up to infinity. We can count things using counting numbers, however, 0 is not included in counting numbers as we cannot count 0.
Question 2: How many counting numbers are there?
Answer:
There are infinite counting numbers because the definition of counting numbers itself states that they generate from 1 and they terminate at infinity. This shows that there can be infinite counting numbers.
Question 3: What is the mean of the first 100 counting numbers?
Answer:
In order to find the mean of n numbers, first add all the numbers and then divide it by n. Therefore, to find the mean of first 100 counting numbers, the first step is add the first 100 numbers, that is, 1 + 2 + 3 + … 100 = 5050, now the second and the last step is to divide the number by 100, that is, 5050/100 = 50.5. So, the mean of first 100 counting numbers is 50.5.
Question 4: What is 1,000,000,000 in English?
Answer:
1,000,000,000 is a counting number and in English, it is called 1 billion.

Question 5: What is another name for counting numbers?
Answer:
Counting numbers are also called natural numbers as they also generate from 1 and go up to infinity. Natural numbers just like counting numbers also do not include 0.
Related Articles
- How many Counting Numbers are there?
- What is another name for Natural Numbers?
- Is 0 a Natural Number?
COUNT function
excel
Formulas and Functions
Functions
Functions
COUNT function
Excel for Microsoft 365 Excel for Microsoft 365 for Mac Excel for the web Excel 2021 Excel 2021 for Mac Excel 2019 Excel 2019 for Mac Excel 2016 Excel 2016 for Mac Excel 2013 Excel 2010 Excel 2007 Excel for Mac 2011 Excel Starter 2010 More...Less
Function COUNT counts the number of cells containing numbers and the number of numbers in the argument list. Function COUNT is used to determine the number of numeric cells in ranges and arrays of numbers. For example, to calculate the number of numbers in the range A1:A20, you can enter the following formula: =COUNT(A1:A20) . In this example, if five cells from the range contain numbers, then the result will be 5 .
Function COUNT is used to determine the number of numeric cells in ranges and arrays of numbers. For example, to calculate the number of numbers in the range A1:A20, you can enter the following formula: =COUNT(A1:A20) . In this example, if five cells from the range contain numbers, then the result will be 5 .
Syntax
COUNT(value1,[value2],…)
The COUNT function has the following arguments.
-
Value1 is a required argument. The first element, cell reference, or range for which you want to count the number of numbers.
-
Value2; ... is an optional argument. Up to 255 additional items, cell references, or ranges in which you want to count the number of numbers.

Note: Arguments can contain or refer to data of various types, but only numbers are counted.
Remarks
-
Arguments that are numbers, dates, or the textual representation of numbers (for example, a number enclosed in quotation marks such as "1") are taken into account.
-
Boolean values and text representations of numbers entered directly in the argument list are also taken into account.
-
Arguments that are error values or text that cannot be converted to numbers are ignored.
-
If the argument is an array or a reference, then only numbers are taken into account. Empty cells, booleans, text, and error values in an array or reference are ignored.
-
If you want to count boolean values, text elements, or error values, use function COUNT .
-
If you want to count only those numbers that meet certain criteria, use the function COUNTIF or COUNTIF .
Example
Copy the sample data from the following table and paste it into cell A1 of a new Excel sheet. To display formula results, select them and press F2 followed by ENTER. Change the width of the columns, if necessary, to see all the data.
| Data | ||
| 08.12.2008 | ||
| 19 | ||
22.24 | ||
| TRUE | ||
| #DIV/0! | ||
| Formula | Description | Result |
| =COUNT(A2:A7) | Counts the number of cells containing numbers in the range A2:A7. | 3 |
| =COUNT(A5:A7) | Counts the number of cells containing numbers in the range A5:A7. | 2 |
| =COUNT(A2:A7;2) | Counts the number of cells containing numbers in the range A2:A7, given the number 2. | 4 |
Mathematics. Number. Count up to 100
There is no such thing as a "number" in nature. The number is a fiction that allows people to talk to each other more gracefully. If there were no numbers, we would have to say this:
- There is a cow, and a cow, and a cow, and a cow, and a cow grazing in the meadow.
Thanks to numbers, the same idea can be expressed more simply:
— Five cows are grazing in the meadow.
A number taken by itself means nothing. If you just say "three", then you get some kind of nonsense, nonsense, nonsense. Numbers make sense only when they are followed by other words: “three kittens”, “three pigs”, “three children”.
This feature of numbers should be remembered by parents who want to teach their children to count. There is little use in learning a number series with a child like a poem: "One, two, three, four, five ..." - and so on. If the baby knows by heart such a “rhyme”, then this does not mean that he knows how to count. Give him something to count, well, even his own colored pencils, and he will constantly go astray: shifting pencils and reciting a rhyme will not be coordinated.
Numbers for a child from the very beginning should be connected with some objects: “one-pencil, two-pencil, three-pencil”. My own fingers are best suited for this: “one” - and I lightly pull the baby by the little finger, “two” - by the ring finger, and so on - up to ten. Then he, most likely, will want to pull my fingers too - a great opportunity to “fix the material covered”. Counting objects now becomes quite easy: "one" - the little finger touched the first pencil, "two" - the ring finger touched the second pencil. Now it's impossible to lose count.
Then he, most likely, will want to pull my fingers too - a great opportunity to “fix the material covered”. Counting objects now becomes quite easy: "one" - the little finger touched the first pencil, "two" - the ring finger touched the second pencil. Now it's impossible to lose count.
Numbers can be thought of as additional "names" that we give to identical objects collected in one pile. The distribution of such additional "names" is called recalculation. Of particular importance is the "name" received by the most recent item. It gives us an answer to the question: how many items are in the pile?
Having mastered the first ten, you can move on to counting up to twenty. Toes are well suited for this purpose: “One-on-a-foot, two-on-a-foot, three-on-a-foot ...” This does not cause any difficulty. A little later, however, it will be necessary to admit that, in fact, it is not customary to think so. For some reason, instead of the word “foot”, they say “twenty”: “One-twenty, two-twenty, three-twenty . ..”, and for the very last toe they came up with a completely special word: "twenty". At this stage, it makes sense to linger longer and not rush to move on to counting up to a hundred. Otherwise, it may turn out that the child will subsequently confuse "thirteen" and "thirty", "sixteen" and "sixty".
..”, and for the very last toe they came up with a completely special word: "twenty". At this stage, it makes sense to linger longer and not rush to move on to counting up to a hundred. Otherwise, it may turn out that the child will subsequently confuse "thirteen" and "thirty", "sixteen" and "sixty".
Counting from eleven to twenty is the most difficult, and it should be easier to go further - it’s only a pity that the fingers are over. It's time to get children's accounts: ten rows of ten beads. Our goal is not at all for the child to name all the numbers in a row from one to one hundred. Counting to a hundred is just as difficult and pointless for a toddler as it is for an adult to count to a thousand. It is important that the child be able to: (1) name the number of beads set aside, (2) independently set aside a certain number of beads, and (3) going through the abacus, count within a not too large gap, for example, from \ (75 \) to \ (85 \ ).
Beads are deposited as follows. When all the beads have gathered on the right side, it is “not at all”, “zero” (or “zero” - as you like). "One" or "one" means that one bead of the top row has moved to the left side. "Ten" - the entire top row is on the left side. "Eleven" - one bead from the second joins the already set aside ten beads from the top row. "Twenty" - the top two rows have completely moved to the left side. "Thirty" - the top three rows are on the left side, and so on. (When it's time to count on the accounts "in an adult way", the beads will be deposited in a different way - starting from the bottom row.)
When all the beads have gathered on the right side, it is “not at all”, “zero” (or “zero” - as you like). "One" or "one" means that one bead of the top row has moved to the left side. "Ten" - the entire top row is on the left side. "Eleven" - one bead from the second joins the already set aside ten beads from the top row. "Twenty" - the top two rows have completely moved to the left side. "Thirty" - the top three rows are on the left side, and so on. (When it's time to count on the accounts "in an adult way", the beads will be deposited in a different way - starting from the bottom row.)
It would be good to lead the child to the idea that when it comes to numbers more than ten, it is not at all necessary to count them or set them aside from beginning to end one bead at a time. For example, if you want to postpone “\ (23 \)”, then you can immediately postpone the entire first row and say “ten”, then the entire second row and say “twenty”, and only then comes “twenty-one”, “twenty-two ", "twenty three".
Now you can introduce your child to writing numbers. Single digits are not a problem - they are well absorbed as the child learns to write numbers. And it takes some effort to interpret two-digit numbers. How, for example, to decrypt the entry "\(38\)"? The first digit indicates that \ (3 \) of the upper rows (\ (3 \) full ten) must be completely postponed on the accounts. The second number means that \(8\) more individual beads should be put aside on the next row. When the child stops confusing numbers like "\(16\)" and "\(61\)", we can assume that the initial stage of learning has been successfully completed.
Synopsis
1. The numbers are additional "names" that we give when recounting the same items collected in one pile. The "name" of the last item gives us the answer to the question: How many items are in the pile?
2. It is convenient to learn counting up to \(10\) on your own fingers. When switching to counting up to \(20\), you can connect your toes. It is convenient to train in the account up to \ (100 \) on children's accounts.
It is convenient to train in the account up to \ (100 \) on children's accounts.
Tasks
1.1.1. Count everything possible that catches your eye: coins, pebbles, steps. Especially the child likes to count sweets.
1.1.2. Countdown of a given amount: “You can take ten pieces of chocolate for yourself. Please take fifteen steps!”
1.1.3. What number comes after five, nine, nineteen, etc.? What number comes before six, ten, twenty?
1.1.4. Some popular board games are great for introducing children to the world of numbers. Especially dominoes. “I took seven bones. Take the same, please! Now I have seven and you have seven, but how many are left in the market? How many points are there? That's right, six. And here are six. And how much will it be? But, look, there are no glasses at all. It's zero, not at all. Let's count all the bones and make sure everything is in place. There must be twenty-eight of them in all. Etc. etc." Another great game is cards, a throw-away fool.