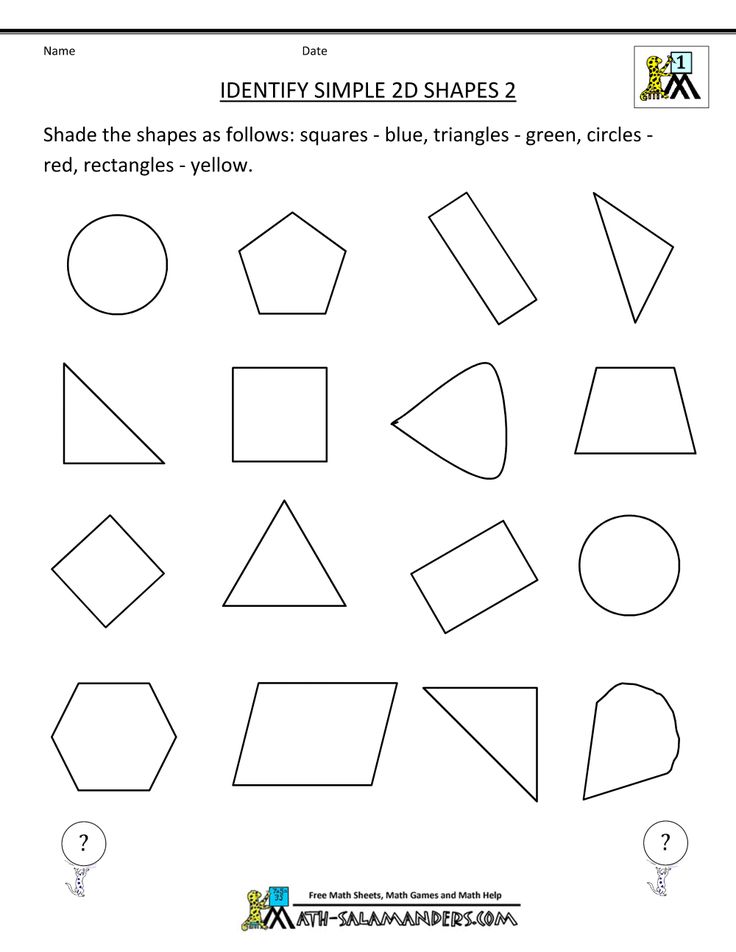
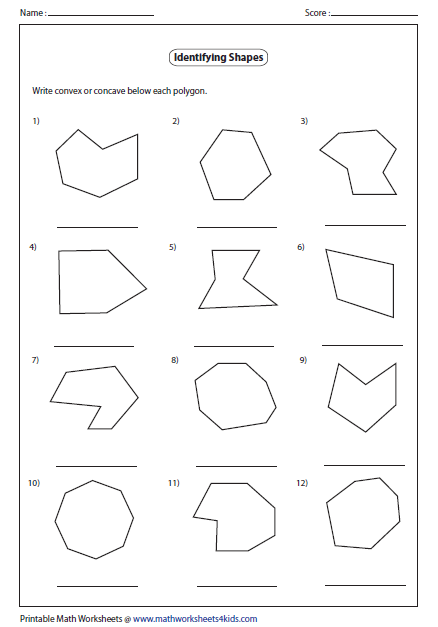
Identification of shapes
Shapes – Definition with Examples
What are Shapes?
In geometry, a shape can be defined as the form of an object or its outline, outer boundary or outer surface.
Everything we see in the world around us has a shape. We can find different basic shapes such as the two-dimensional square, rectangle, and oval or the three-dimensional rectangular prism, cylinder, and sphere in the objects we see around us. These geometric shapes appear in objects we see as credit cards, bills and coins, finger rings, photo frames, dart boards, huts, windows, magician’s wands, tall buildings, flower pots, toy trains, and balloons.
Recommended Games:
Different Types of Shapes
Shapes can be classified into open and closed shapes.
| In geometry, an open shape can be defined as a shape or figure whose line segments and/or curves do not meet. They do not start and end at the same point. | In geometry, a closed shape can be defined as an enclosed shape or figure whose line segments and/or curves are connected or meet. |
Closed geometric shapes can further be put into two broad categories, namely two-dimensional and three-dimensional shapes.
| The 2-Dimensional shape is flat. | A 3-Dimensional Shape is a solid shape. |
| It has two dimensions, that is, length and width. | It has two dimensions, that is, length, width, and depth. |
Here’s a list of 2-D or two-dimensional shapes with their names and pictures:
| Two-Dimensional Geometric Shapes |
Here’s a list of 3-D or three-dimensional shapes with their names and pictures:
| Three-Dimensional Geometric Shapes |
The color, overall size, and orientation called the non-defining attributes of a two-dimensional or three-dimensional shape do not define or affect the shape in any way. These attributes can change without any effect on the shape.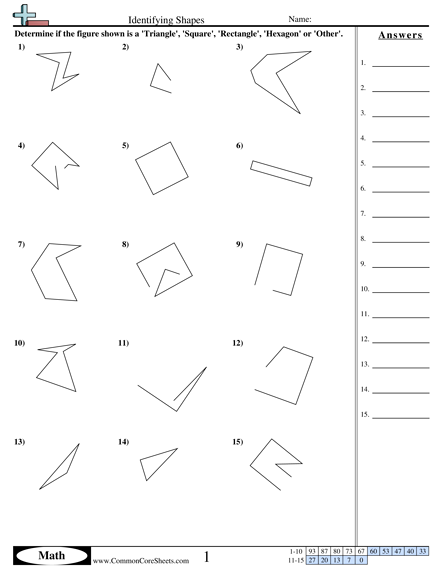
On the other hand, defining attributes such as the number of sides (parallel or non-parallel, straight or curved), vertices, edges, and faces of a shape, whether the shape is open or closed, and the angle measures determine the shape of a two-dimensional or three-dimensional object. Any change in these defining attributes will change the shape.
Recommended Worksheets:
Solved Examples on Shapes
Example 1: Name the shapes.
- A polygon with 6 sides.
- Outline of a door.
- When you fold square corner to corner.
- A square and a triangle on top of it.
Solution:
- Hexagon
- Rectangle or quadrilateral
- Triangle
- Pentagon
Example 2: Classify the given letters as open shape or closed shape.
C, D, L, M, O, S, U, V, Z
Solution:
Open shape: C, L, M, S, U, V, Z
Closed shape: D, O
Example 3: Identify the solid shape of given objects.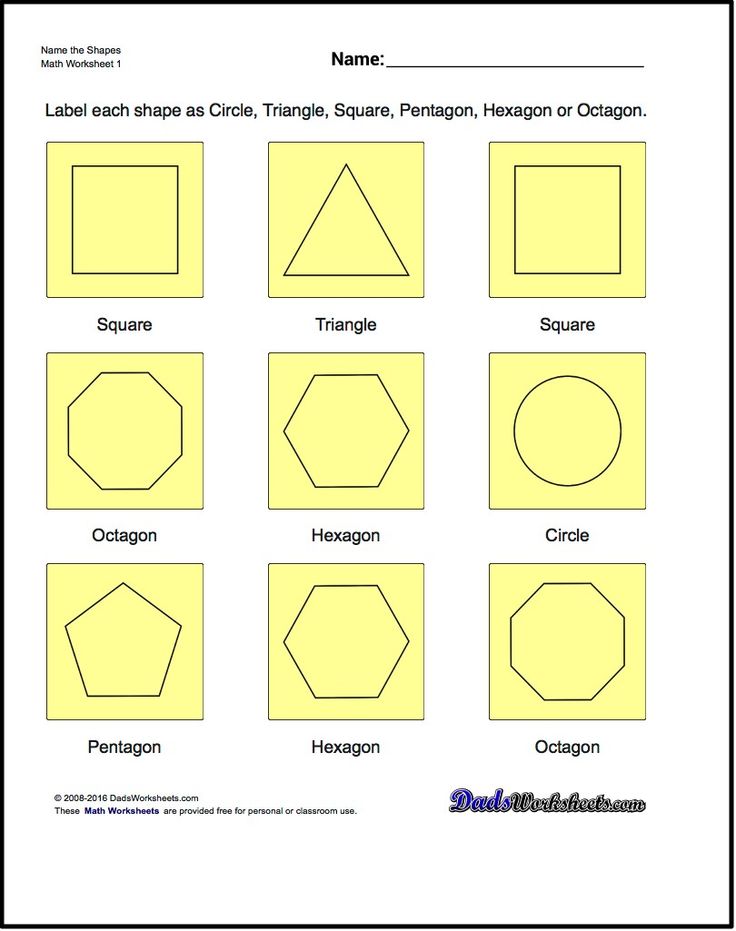
- Globe
- Book
- Cold drink can
- Dice
Solution:
- Sphere
- Cuboid
- Cylinder
- Cube
Example 4: Why is the crescent-shaped moon not a polygon?
Solution:
Crescent shape moon is not a polygon as it has curved lines.
Practice Problems
1
What is 8-sided polygon known as?
hexagon
heptagon
octagon
quadrilateral
Correct answer is: octagon
A polygon with 8 sides is known as octagon.
2
How many dimensions does a solid shape have?
1
2
3
depends on the shape
Correct answer is: 3
All solid shapes are 3-dimensional shapes.
3
Which of the following statements is incorrect?
closed shapes can have only straight sides.
closed shapes have definite area.
start and end point of a closed shape are the same.
start and end point of a open shape are the different.
Correct answer is: closed shapes can have only straight sides.
Closed shapes are shapes whose start and end points are the same. It is not necessary that it is formed by only straight sides.
The 9 Most Common Shapes and How to Identify Them
You've probably learned a lot about shapes without ever really thinking about what they are. But understanding what a shape is is incredibly handy when comparing it to other geometric figures, such as planes, points, and lines.
In this article, we'll cover what exactly a shape is, as well as a bunch of common shapes, what they look like, and the major formulas associated with them.
What Is a Shape?
If someone asks you what a shape is, you'll likely be able to name quite a few of them. But "shape" has a specific meaning, too—it's not just a name for circles, squares, and triangles.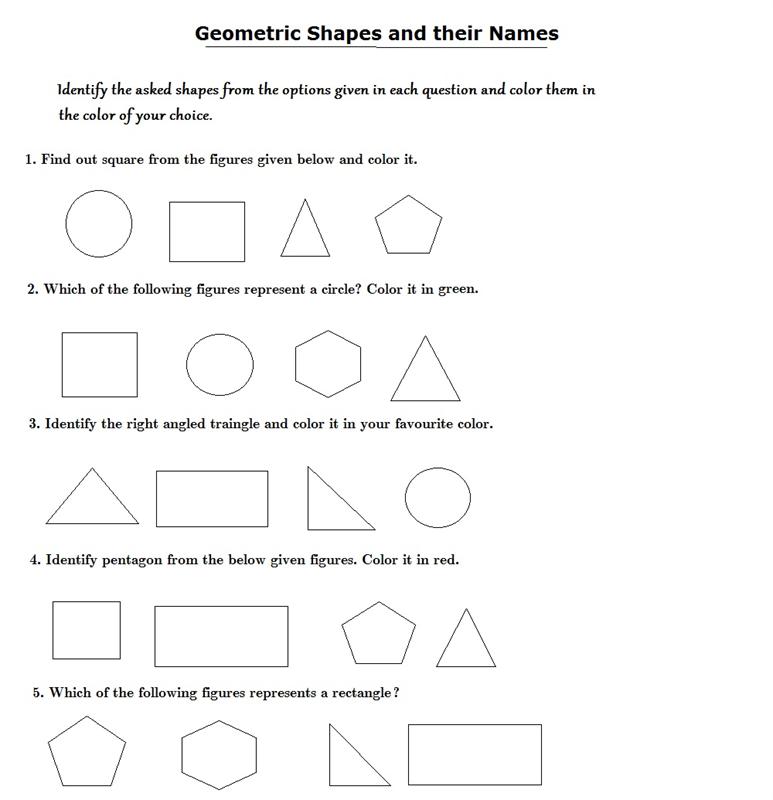
A shape is the form of an object—not how much room it takes up or where it is physically, but the actual form it takes. A circle isn't defined by how much room it takes up or where you see it, but rather the actual round form that it takes.
A shape can be any size and appear anywhere; they're not constrained by anything because they don't actually take up any room. It's kind of hard to wrap your mind around, but don't think of them as being physical objects—a shape can be three-dimensional and take up physical room, such as a pyramid-shaped bookend or a cylinder can of oatmeal, or it can be two-dimensional and take up no physical room, such as a triangle drawn on a piece of paper.
The fact that it has a form is what differentiates a shape from a point or a line.
A point is just a position; it has no size, no width, no length, no dimension whatsoever.
A line, on the other hand, is one-dimensional. It extends infinitely in either direction and has no thickness. It's not a shape because it has no form.
It's not a shape because it has no form.
Though we may represent points or lines as shapes because we need to actually see them, they don't actually have any form. That's what differentiates a shape from the other geometric figures—it's two- or three-dimensional, because it has a form.
Cubes, like those seen here, are three-dimensional forms of squares—both are shapes!
The 6 Main Types of Two-Dimensional Geometric Shapes
Picturing a shape just based on definition is difficult—what does it mean to have form but not take up space? Let's take a look at some different shapes to better understand what exactly it means to be a shape!
We often classify shapes by how many sides they have. A "side" is a line segment (part of a line) that makes up part of a shape. But a shape can have an ambiguous number of sides, too.
Type 1: Ellipses
Ellipses are round, oval shapes in which a given point (p) has the same sum of distance from two different foci.
Oval
An oval looks a bit like a smooshed circle—rather than being perfectly round, it's elongated in some way. However, the classification is imprecise. There are many, many kinds of ovals, but the general meaning is that they are a round shape that is elongated rather than perfectly round, as a circle is. An oval is any ellipses where the the foci are in two different positions.
Because an oval is not perfectly round, the formulas we use to understand them have to be adjusted.
It's also important to note that calculating the circumference of an oval is quite difficult, so there's no circumference equation below. Instead, use an online calculator or a calculator with a built-in circumference function, because even the best circumference equations you can do by hand are approximations.
Definitions
- Major Radius: the distance from the oval's origin to the furthest edge
- Minor Radius: the distance from the oval's origin to the nearest edge
Formulas
- Area = $\Major \Radius*\Minor \Radius*π$
Circle
How many sides does a circle have? Good question! There's no good answer, unfortunately, because "sides" have more to do with polygons—a two-dimensional shape with at least three straight sides and typically at least five angles. Most familiar shapes are polygons, but circles have no straight sides and definitely lack five angles, so they are not polygons.
Most familiar shapes are polygons, but circles have no straight sides and definitely lack five angles, so they are not polygons.
So how many sides does a circle have? Zero? One? It's irrelevant, actually—the question simply doesn't apply to circles.
A circle isn't a polygon, but what is it? A circle is a two-dimensional shape (it has no thickness and no depth) made up of a curve that is always the same distance from a point in the center. An oval has two foci at different positions, whereas a circle's foci are always in the same position.
Definitions
- Origin: the center point of the circle
- Radius: the distance from the origin to any point on the circle
- Circumference: the distance around the circle
- Diameter: the length from one edge of the circle to the other
- $\bo{π}$: (pronounced like pie) 3.
 2$
2$
Type 2: Triangles
Triangles are the simplest polygons. They have three sides and three angles, but they can look different from one another. You might have heard of right triangles or isosceles triangles—those are different types of triangles, but all will have three sides and three angles.
Because there are many kinds of triangles, there are lots of important triangle formulas, many of them more complex than others. The basics are included below, but even the basics rely on knowing the length of the triangle's sides. If you don't know the triangle's sides, you can still calculate different aspects of it using angles or only some of the sides.
Definitions
- Vertex: the point where two sides of a triangle meet
- Base: any of the triangle's sides, typically the one drawn at the bottom
- Height: the vertical distance from a base to a vertex it is not connected to
Formulas
- Area = ${\base*\height}/2$
- Perimeter = $\side a + \side b + \side c$
Type 3: Parallelograms
A parallelogram is a shape with equal opposite angles, parallel opposite sides, and parallel sides of equal length. You might notice that this definition applies to squares and rectangles—that's because squares and rectangles are also parallelograms! If you can calculate the area of a square, you can do it with any parallelogram.
You might notice that this definition applies to squares and rectangles—that's because squares and rectangles are also parallelograms! If you can calculate the area of a square, you can do it with any parallelogram.
Definitions
- Length: the measure of the bottom or top side of a parallelogram
- Width: the measure of the left or right side of a parallelogram
Formulas
- Area: $\length*\height$
- Perimeter: $\Side 1 + \Side 2 + \Side 3 + \Side 4$
- Alternatively, Perimeter: $\Side*4$
Rectangle
A rectangle is a shape with parallel opposite sides, combined with all 90 degree angles. As a type of parallelogram, it has opposite parallel sides. In a rectangle, one set of parallel sides is longer than the other, making it look like an elongated square.
Because a rectangle is a parallelogram, you can use the exact same formulas to calculate their area and perimeters.
Square
A square is a lot like a rectangle, with one notable exception: all its sides are equal length. Like rectangles, squares have all 90 degree angles and parallel opposite sides. That's because a square is actually a type of rectangle, which is a type of parallelogram!
For that reason, you can use the same formulas to calculate the area or perimeter of a square as you would for any other parallelogram.
Rhombus
A rhombus is—you guessed it—a type of parallelogram. The difference between a rhombus and a rectangle or square is that its interior angles are only the same as their diagonal opposites.
Because of this, a rhombus looks a bit like a square or rectangle skewed a bit to the side. Though perimeter is calculated the same way, this affects the way that you calculate the area, because the height is no longer the same as it would be in a square or rectangle.
Definition
- Diagonal: the length between two opposite vertices
Formulas
- Area = ${\Diagonal 1*\Diagonal 2}/2$
Type 4: Trapezoids
Trapezoids are four-sided figures with two opposite parallel sides. Unlike a parallelogram, a trapezoid has just two opposite parallel sides rather than four, which impacts the way you calculate the area and perimeter.
Definitions
- Base: either of a trapezoid's parallel sides
- Legs: either of the trapezoids non-parallel sides
- Altitude: the distance from one base to the other
Formulas
- Area: $({\Base_1\length + \Base_2\length}/2)\altitude$
- Perimeter: $\Base + \Base + \Leg + \Leg$
Type 5: Pentagons
A pentagon is a five-sided shape. We typically see regular pentagons, where all sides and angles are equal, but irregular pentagons also exist. An irregular pentagon has unequal side and unequal angles, and can be convex—with no angles pointing inward—or concave—with an internal angle greater than 180 degrees.
We typically see regular pentagons, where all sides and angles are equal, but irregular pentagons also exist. An irregular pentagon has unequal side and unequal angles, and can be convex—with no angles pointing inward—or concave—with an internal angle greater than 180 degrees.
Because the shape is more complex, it needs to be divided into smaller shapes to calculate its area.
Definitions
- Apothem: a line drawn from the pentagon's center to one of the sides, hitting the side at a right angle.
Formulas
- Perimeter: $\Side 1 + \Side 2 + \Side 3 + \Side 4 + \Side 5$
- Area: ${\Perimeter*\Apothem}/2$
Type 6: Hexagons
A hexagon is a six-sided shape that is very similar to pentagon. We most often see regular hexagons, but they, like pentagons, can also be irregular and convex or concave.
Also like pentagons, a hexagon's area formula is significantly more complex than that of a parallelogram.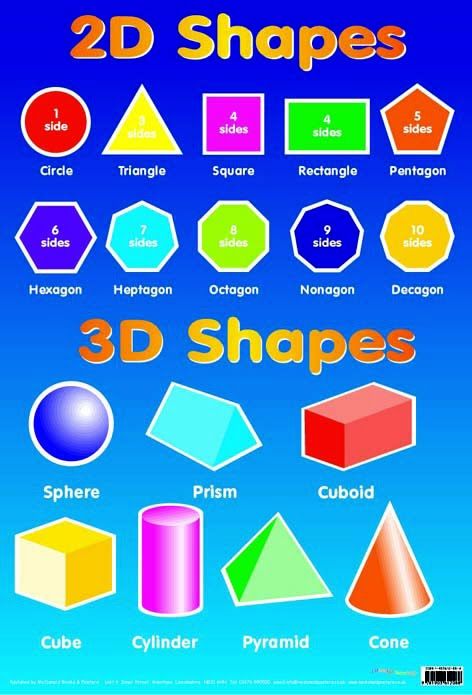
Formulas
- Perimeter: $\Side 1 + \Side 2 + \Side 3 + \Side 4 + \Side 5 + \Side 6$
- Area: ${3√3*\Side*2}/2$
- Alternatively, Area: ${\Perimeter*\Apothem}/2$
What About Three-Dimensional Geometric Shapes?
There are also three-dimensional shapes, which don't just have a length and a width, but also depth or volume. These are shapes you see in the real world, like a spherical basketball, a cylindrical container of oatmeal, or a rectangular book.
Three-dimensional shapes are naturally more complex than two-dimensional shapes, with an additional dimension—the amount of space they take up, not just the form—to include when calculating area and perimeter.
Math involving 2D shapes, such as those above, is called plane geometry because it deals specifically with planes, or flat shapes. Math involving 3D shapes like spheres and cubes is called solid geometry, because it deals with solids, another word for 3D shapes.
2D shapes make up the 3D shapes we see every day!
3 Key Tips for Working With Shapes
There are so many types of shapes that it can be tricky to remember which is which and how to calculate their areas and perimeters. Here's a few tips and tricks to help you remember them!
#1: Identify Polygons
Some shapes are polygons and some are not. One of the easiest ways to narrow down what type of shape something is is figuring out if it's a polygon.
A polygon is comprised of straight lines that do not cross. Which of the shapes below are polygons and which are not?
The circle and oval are not polygons, which means their area and perimeter are calculated differently. Learn more about how to calculate them using $π$ above!
#2: Check for Parallel Sides
If the shape you're looking at is a parallelogram, it's generally easier to calculate its area and perimeter than if it isn't a parallelogram.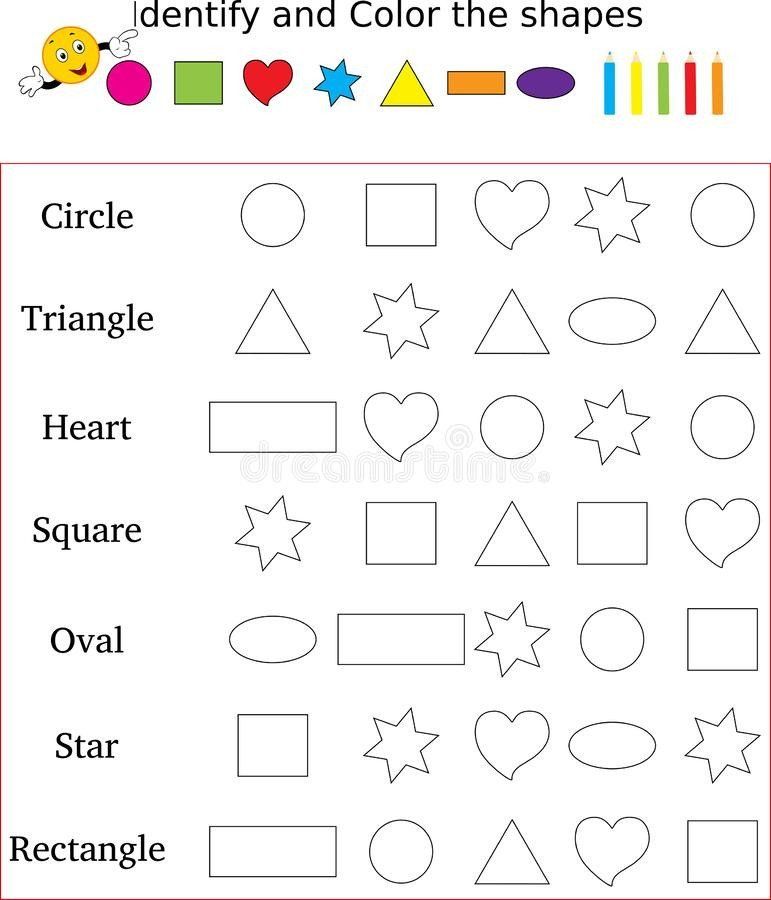 But how do you identify a parallelogram?
But how do you identify a parallelogram?
It's right there in the name—parallel. A parallelogram is a four-sided polygon with two sets of parallel sides. Squares, rectangles, and rhombuses are all parallelograms.
Squares and rectangles use the same basic formulas for area—length times height. They're also very easy to find perimeter for, as you just add all the sides together.
Rhombuses are where things get tricky, because you multiply the diagonals together and divide by two.
To determine what kind of parallelogram you're looking at, ask yourself if it has all 90-degree angles.
If yes, it's either a square or a rectangle. A rectangle has two sides that are slightly longer than the others, whereas a square has sides of all equal length. Either way, you calculate the area by multiplying the length times the height and perimeter by adding all four sides together.
If no, it's probably a rhombus, which looks like if you took a square or rectangle and skewed it in either direction.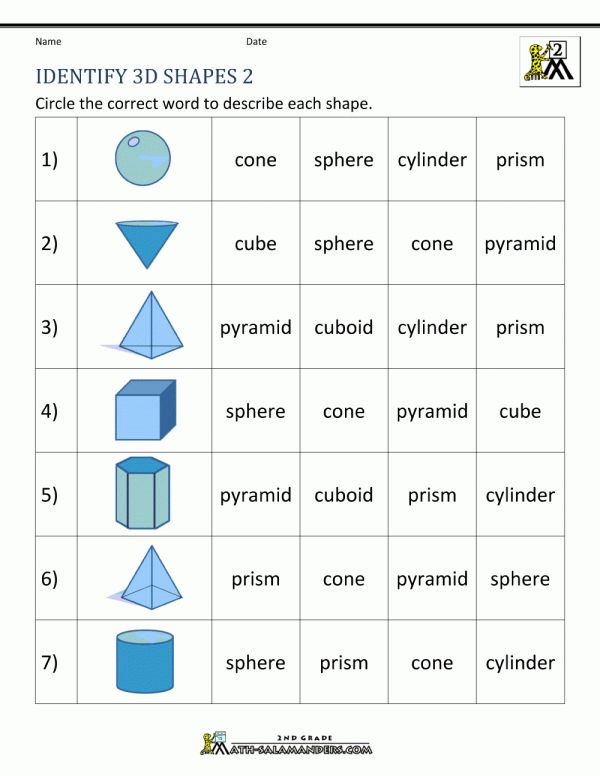 In this case, you'll find the area by multiplying the two diagonals together and dividing by two. Perimeter is found the same way that you would find the perimeter of a square or rectangle.
In this case, you'll find the area by multiplying the two diagonals together and dividing by two. Perimeter is found the same way that you would find the perimeter of a square or rectangle.
#3: Count the Number of Sides
Formulas for shapes that don't have four sides can get quite tricky, so your best bet is to memorize them. If you have trouble keeping them straight, try memorizing the Greek words for numbers, such as:
Tri: three, as in triple, meaning three of something
Tetra: four, as in the number of squares in a Tetris block
Penta: five, as in the Pentagon in Washington D.C., which is a large building in the shape of a Pentagon
Hexa: six, as in hexadecimal, the six-digit codes often used for color in web and graphic design
Septa: seven, as in Septa, the female clergy of Game of Thrones' religion, which has seven gods
Octo: eight, as in the eight legs of an octopus
Ennea: nine, as in an enneagram, a common model for human personalities
Deca: ten, as in a decathlon, in which athletes complete ten events
What's Next?
If you're prepping for the ACT and want a little additional help on your geometry, check out this guide to coordinate geometry!
If you're more the SAT type, this guide to triangles on the SAT geometry section will help you prepare for the test!
Can't get enough of ACT math? This guide to polygons on the ACT will help you prepare with useful strategies and practice problems!
Have friends who also need help with test prep? Share this article!
Melissa Brinks
About the Author
Melissa Brinks graduated from the University of Washington in 2014 with a Bachelor's in English with a creative writing emphasis.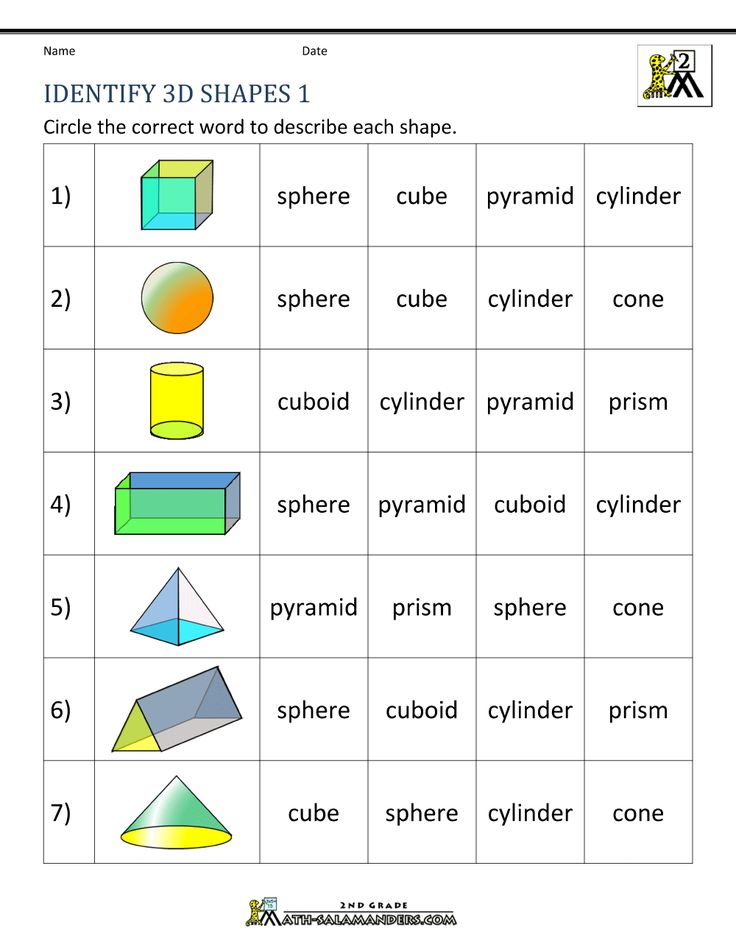 She has spent several years tutoring K-12 students in many subjects, including in SAT prep, to help them prepare for their college education.
She has spent several years tutoring K-12 students in many subjects, including in SAT prep, to help them prepare for their college education.
Identification: forms, varieties
The concept and forms of forensic identification
It is necessary to consider the types and forms of forensic identification based on the fact that they belong to the category of qualification and should be considered differently.
Forensic identification exists in two main forms: forensic and non-forensic.
After the fact of a crime is established, operational-search workers begin a targeted search for the offender, the victim, witnesses and eyewitnesses, as well as facts and data that will be important in solving the crime. In the process of operational-search activities, investigators identify wanted persons on the basis of photographs, subjective portraits and descriptions that are essentially identification. nine0005 Note 1
Checks of forensic records in the process of search activities in some cases can also be considered as an identification study.
The result of such procedural actions is the preparation of official documents, such as a certificate, a report, etc. Such documents are not considered in the criminal process and are not considered as a source of evidence. Such identification does not apply to procedural.
Identification studies in the procedural form are used much more widely than non-procedural ones. There are two main varieties of the procedural form of research:
- presentation by the investigator for identification by a witness, victim, suspect or accused of a person or object, which in essence is the identification of an object that is imprinted in the memory of a person participating in the identification with the object presented for identification;
- carrying out an identification examination.
Types of forensic identification
Currently, the classification of forensic research is based on the properties and nature of the objects of identification. nine0005
nine0005
Based on the properties of an identifiable object, forensic identification can be divided into: and the nature of the surface; Example 1
An example of this type is the identification of people by the reliefs of the skin of the hands or the identification of chisels by the structural features of the cutting edges. nine0005
- identification through functional-motor complexes based on the uniqueness of the interaction of the object's parts;
An example of such an interaction is the gait of people and their handwriting, or the features of the operation of printers, sewing machines, etc., which make up a functional complex.
- identification by the composition and structure of the object, which is a relatively new type of forensic identification. At the same time, different groups of objects are distinguished: total, such as sheets in one notebook or cigarettes in one pack, and amorphous - liquid or bulk substances.
 In the process of creation, manufacture, cohabitation and simultaneous impact on such objects by external factors, they lead to the creation of a set of properties that can be identified. nine0018
In the process of creation, manufacture, cohabitation and simultaneous impact on such objects by external factors, they lead to the creation of a set of properties that can be identified. nine0018
Based on the nature of the object that can be identified, forensic identification is divided into:
- identification through material-fixed representations, used in cases where the identifying object displays aspects of the external structure of the object of identification;
Thus, people are identified by traces of hands and feet, traces of teeth and clothes, as well as burglary tools by the nature of the damage.
- identification of the whole in parts based on the features that make up the identification complex contained in the edges and lines of separation of the parts, surfaces and separation planes and in the internal structure of the parts;
- identification by mental image, based on the possibility of imprinting the object of identification not only in the form of a material trace, but also in human perception and memory;
An example of this type of identification is the presentation of objects for identification. nine0005
nine0005
- identification by description and features, based on the fixation and storage of numerous information in the form of a verbal description. This form of fixation is one of the oldest and most traditional. Such descriptions can also display a whole set of identification features that characterize the object. In some cases, such complex descriptions serve as the basis for the identification process.
It is necessary to take into account the subjectivity in the presentation of a set of features that characterize the object on the part of the witness and the subjectivity of the perception of such a presentation on the part of a law enforcement officer. That is why this kind of identification is most often carried out in the form of non-procedural. nine0005
- identification by scent trail, in which detection is carried out with the help of search dogs by the scent trail of an object or person from the scene. Previously, this type of identification was classified as non-procedural, but now the applied olfactory examination and its results in the investigation process have the value of evidence.
Forensic diagnostics
Despite the importance of forensic identification, many evidentiary facts in criminal cases are established without the use of the latter. That is, part of the research in criminal cases is not identification. Research previously classified as non-identification, since 1972 in the literature on forensic science received the definition of "diagnostic". Today, forensic diagnostics consists of studies that determine the state of the object, the changes that have occurred in it, their causes and mechanisms that are associated with the crime.
Diagnostic studies are aimed at solving such issues as: the presence of certain traces on objects, determining the nature of such traces and the object that left them; elucidation of the mechanism of formation of such traces; determination of the suitability of the traces left to identify the object that left them; calculation of the direction and speed of movement of objects; finding out the possibility of firing a shot from a particular type of weapon without interaction with the trigger; assignment of an object to a certain type of weapon, etc.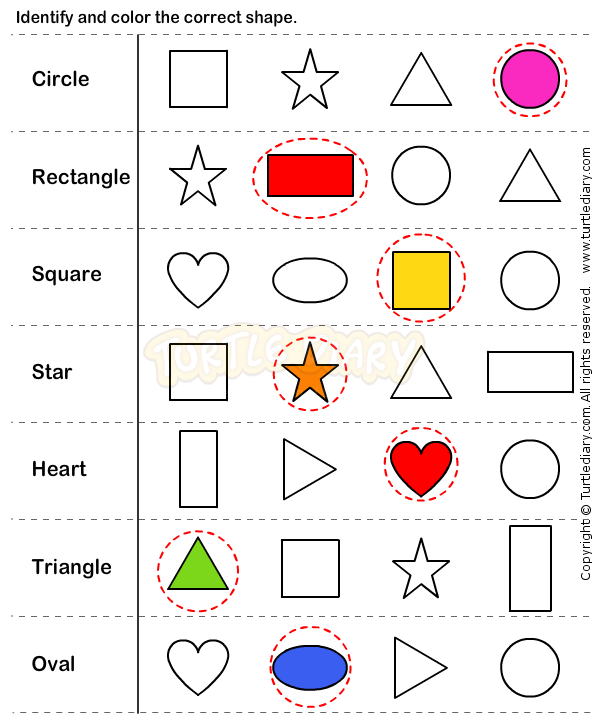
It should also be noted that the non-procedural solution of diagnostic issues is present in many other cases.
Example 5In cases where a search, inspection, investigative experiment or other investigative action is carried out, investigators and specialists, when identifying traces, first determine the nature of such traces, the possible mechanism, and the object that formed them, etc. Further, all objects are subject to examination.
nine0004 Author: Ekaterina Sveklova-BogdanovaLecturer in civil law
Navigation through articles
Previous article
European Court of Human Rights
Next article
Document Examination Rules
- Conducting interrogation of witnesses and victims in a conflict situation nine0018
- Explosive expertise
- Bribery: forensic characteristics
- The main provisions of the forensic methodology nine0018
- Documentation of the progress and results of the inspection of the scene
- All topics by law
Learn more
Land labor and agricultural legislation nine0005
-
Type of work:
Practical work
-
Completed:
November 2, 2022
nine0018 -
Cost:
RUB 2,300
Order the same work
Conclusion, amendment and termination of a civil law contract
-
Type of work:
Report
nine0017 -
Cost:
RUB 1,200
nine0021 -
Make tasks according to the methods
Order the same work nine0005
-
creating documents
-
Type of work:
Test
-
Completed:
September 25, 2021
-
Cost:
nine0004 800 rub
Order the same work
-
-
distance exam nine0005
Order the same work
-
Violations of criminal procedure law under the laws of the United States and EU countries nine0005
-
Type of work:
Coursework
-
Completed:
June 9, 2021
nine0017
Cost:
RUB 3,700
-
Completed:
September 26, 2022
Order the same work
Order the same work
nine0004 View all works on land lawClient identification
Dear Customer!
We remind you that in order to comply with the Federal Law of August 7, 2001 No.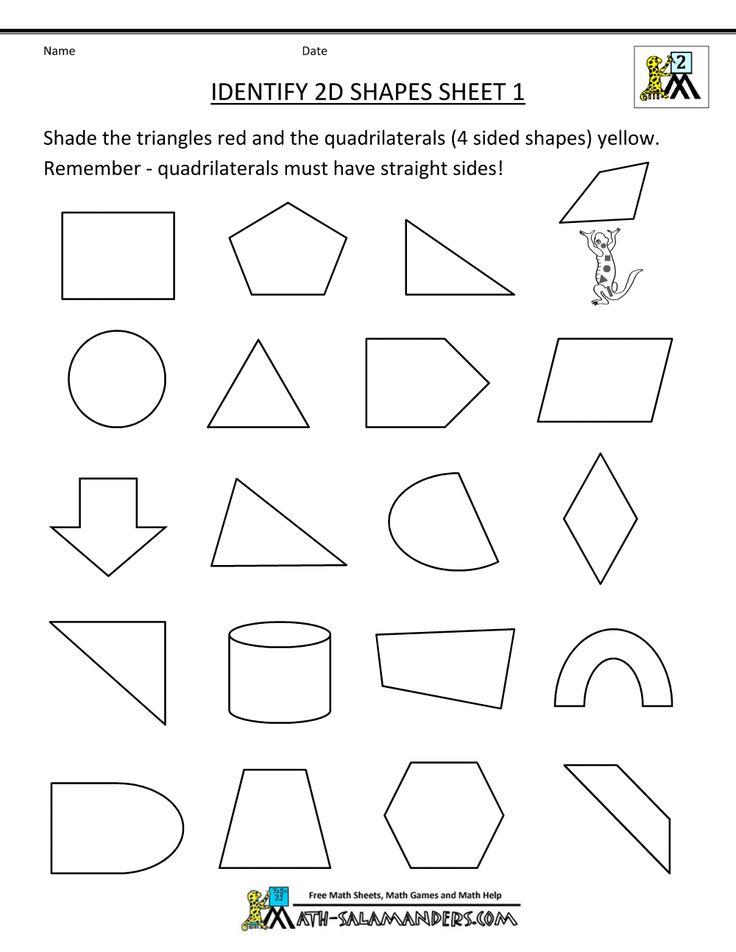 115-FZ “On combating the legalization (laundering) of proceeds from crime, and Financing of Terrorism” VEB.RF annually updates information about its clients, their representatives, beneficiaries and beneficial owners. In turn, clients are obliged to provide information necessary for the implementation of the above Federal law. nine0005
115-FZ “On combating the legalization (laundering) of proceeds from crime, and Financing of Terrorism” VEB.RF annually updates information about its clients, their representatives, beneficiaries and beneficial owners. In turn, clients are obliged to provide information necessary for the implementation of the above Federal law. nine0005
For your convenience, we offer three ways to update your identification information:
When preparing updated identification information, we suggest that you familiarize yourself with the content of the fields of the actual forms of questionnaires placed below.
If it is impossible to provide documents and / or information, please send a letter explaining the reason.