Math work for first graders
1st Grade Math Worksheets
Rich with scads of practice, the CCSS aligned printable 1st grade math worksheets with answer keys help kids solve addition and subtraction problems within 20, extend their counting sequence, understand place value and number systems, measure length and compare sizes, tell time, count money, represent and interpret data, and know the attributes of 2D and 3D shapes in geometry. Our free math worksheets for grade 1 kids give you a peek into what's in store!
All
Addition
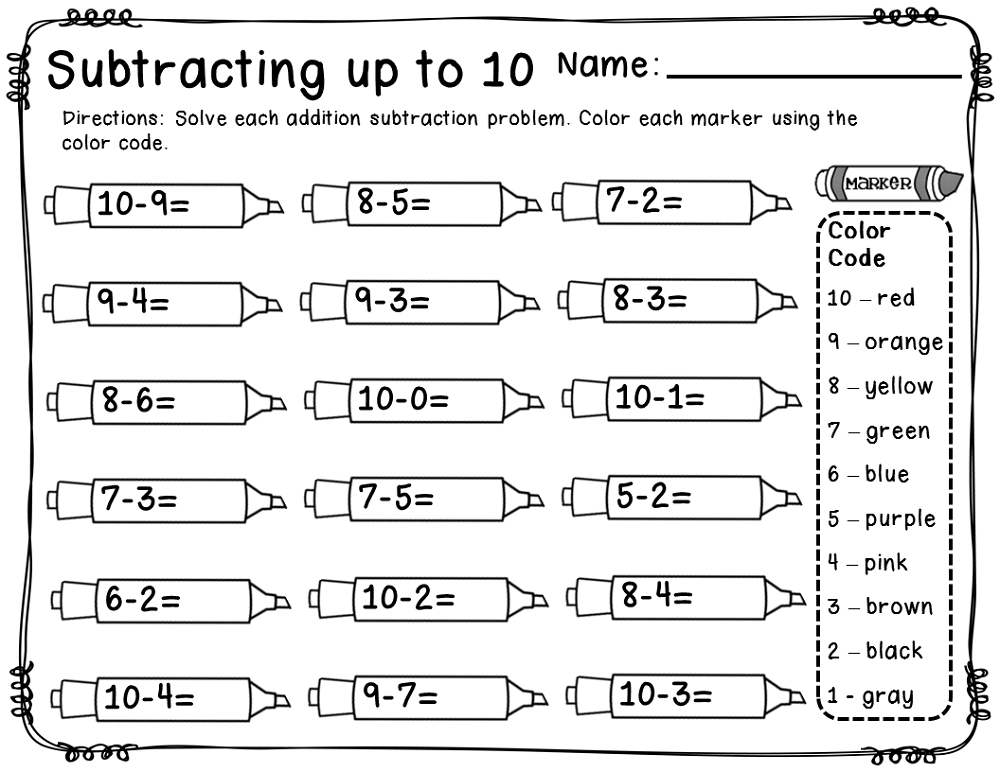
Subtraction
Patterns
Counting
Place Value
Number System
Measurement
Time
Money
Data & Graphs
Geometry
Explore 2,200+ First Grade Math Worksheets
Counting and Adding Pictures
The pictures in two groups present a fascinating array of addition equations for 1st grade kids. Count the pictures in the two groups separately, and then combine the two to find the total number.
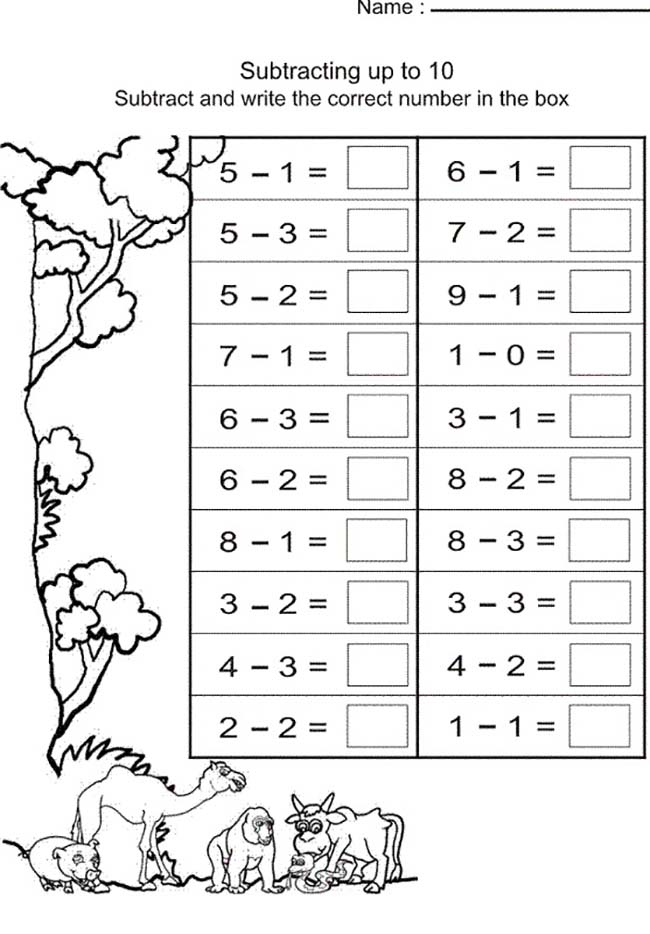
Subtracting on Number Lines | 0 to 10
Get the little hoppers to draw hops on the number lines in these printable grade 1 math worksheets and complete the subtraction equations involving numbers up to 10.
Identifying the Next Picture in a Repeating Pattern
Develop pattern awareness in kids with this set of pdf worksheets. Study the pattern, identify the pattern's core or terms that repeat in the same order and make a logical prediction of what comes next.
Reading and Writing Numbers from 1 to 25
Fluency with numbers is vital in first grade math. Task kids to look at the top of this printable chart, identify and read the numbers from 1 to 25 repeatedly, and copy them to complete the table.
Base Ten Blocks | Tens and Ones
Visualizing numbers is easy with place value blocks or base-10 blocks. Get kids to count the units and rods in the base-10 blocks and write the base-10 numerals.
Identifying Greater and Smaller 2-Digit Numbers
Cracking these 1st grade math worksheet pdfs is a true measure of your place value skills.
Compare 2-digit numbers using symbols in Part A. Circle the greater number in Part B, and the smaller number in Part C.
Ordering Objects from the Shortest to the Longest
Arranging objects of three different sizes from the shortest to the longest, numbering them 1, 2, and 3 respectively, is all that is expected of grade 1 kids.
Telling Time | Hourly Increment
With these pdf math worksheets for grade 1 kids at your disposal, the time is ripe to practice reading clocks to tell the time in whole hours, and choosing the clock face that depicts the specified time.
Counting Dimes
Develop skills in counting the dimes and expressing the amount in dollars, trading dimes for dollars, and converting between them in word problems with this compilation of first grade math worksheet pdfs.
Counting Tally Marks
Let's travel back in time and practice counting using tally marks.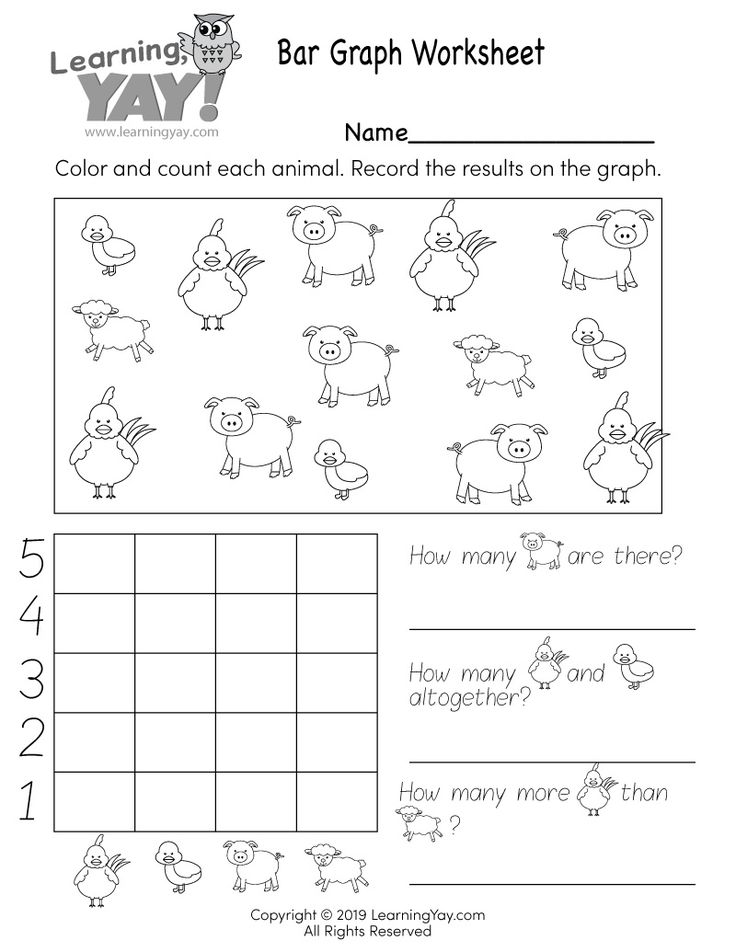
First grade kids have a blast reading and counting each set of tally marks and writing the value it represents.
Identifying 2D Shapes | MCQ
Can the little architects of grade 1 distinguish between a rectangle and a square? Watch them recognize the two-dimensional figures and check the appropriate option that best describes each.
Number Line Addition | 0 to 10
This stack of 1st grade math worksheets has pre-drawn hops on the number lines. The starting-point of the hops, and the number of hops are the two addends and the endpoint is the sum.
Writing Subtraction Equations from Number Lines | 0 to 10
Examine the hops on the number line; identify the minuend, subtrahend, and the difference. Once this is done, completing the subtraction sentence is not a hard nut to crack!
Repeating Patterns | Cut and Glue Activity
Add a spark of fun with repeating picture patterns in this bundle of printable math worksheets for 1st grade kids.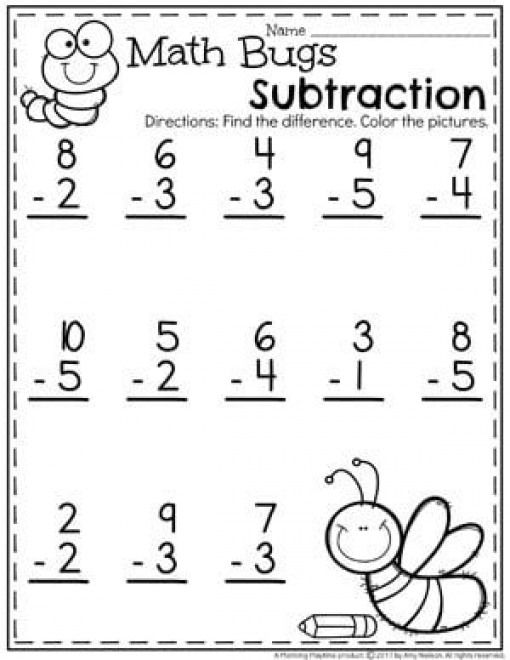
Comprehend the pattern, cut out the graphics and glue the one that comes next in order.
Display Chart - Numbers 1 to 25 | Theme based
The crawling number snails get kids to instantly memorize numerals from 1 to 25. This show-and-tell chart comprising snails-shells inscribed with numbers is a compulsive-print.
Next »
1st Grade Math Worksheets
Share to PinterestPinterestShare to FacebookFacebookShare to PocketPocketShare to TwitterTwitterShare to EmailEmailShare to FlipboardFlipboardWorksheets
Printables
PuzzlesT-Shirts
Math Worksheets
Go Ad Free!
Core Math Worksheets
Fraction Worksheets
Word Problems
Algebra
Other Worksheets
Measurement & Conversions
Patterns and Puzzles
Color by Number
Holiday & Seasonal
Early Learning
Printables
Calculators
Math Worksheets by Grade
Worksheet News
Base 10 Blocks
Base ten blocks worksheets that teach basic addition, subtraction, number sense and place value using visual representations of quantity.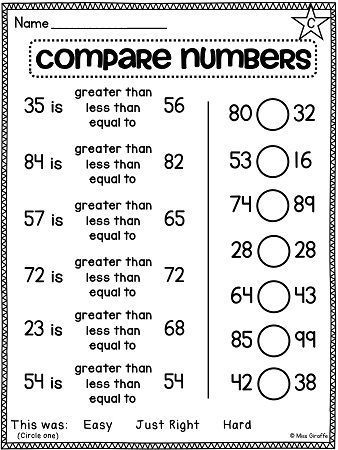
Get your first grade student started with these basic math skill worksheets!
Base 10 Blocks
Addition Worksheets
This is the main page for the addition worksheets. Follow the links for Spaceship Math Addition worksheets, multiple digit addition worksheets, no-carrying addition worksheets and other addition topics. These addition worksheets are free for personal or classroom use.
Addition Worksheets
Subtraction Worksheets
This is the main page for the subtraction worksheets. Follow the links for Spaceship Math Subtraction worksheets, timed subtraction tests, multiple digit subtraction worksheets, simple borrowing and regrouping worksheets, and math worksheets with mixed addition and subtraction problems
Subtraction Worksheets
Fact Family Worksheets
Fact family worksheets focus on sets of related math facts, not specific operations.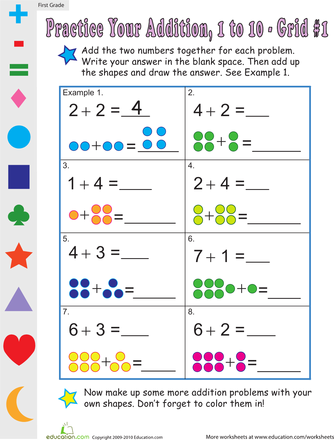
Teach your kids addition and subtraction at the same time, and reinforce the relationships in a fact family! Two fact families are introduced at each level and allow for progressive practice, or just use the worksheets at the end for comprehensive fact family review.
Fact Family Worksheets
Ordering Numbers
Practice ordering numbers worksheets for with multiple numbers in ascending (greatest to least) and descending (least to greatest) orders. Includes whole numbers, decimal numbers and negative numbers. Similar sets of ordering numbers worksheets are presented in both horizontal and vertical formats.
Ordering Numbers
Printable Flash Cards
This page contains free printable flash cards for each math operation. Print the 'worksheet' on the front, then turn the page over and print the 'answer key' version on the back. Some sets have duplicate facts for the more difficult problems near the end so that the sets end up on a multiple of pages.
Those cards are clearly marked as duplicates... use them for extra practice on the harder problems, or put them aside if you want a set with only one flash card for each math fact.
Printable Flash Cards
Hundreds Chart
Every hundreds chart you could imagine! If you're teaching basic counting, number sense, rounding or the basics of arithmetic, you can use a number chart like one of these to speed up building math skills.
Hundreds Chart
Place Value Chart
This page has printable place value charts. In the decimal numbering system, the position (or 'place') of an individual digit in a number determines its value relative to other digits. When a number is written in standard form with groups of three place values separated by commas, each of those groups is called a period. Building number sense by understanding place values is an important early math skill, and these place value charts provide a way to break numbers down to better understand the significane of each digit.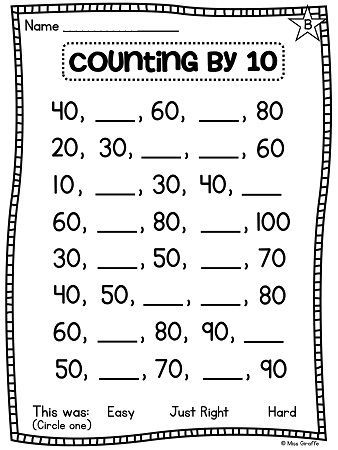
There are place value chart variations for whole numbers only, decimal numbers, and very large numbers. There are different place value chart layouts that reinforce just the place value as well as the period value.
Place Value Chart
Telling Analog Time
Practice worksheets for telling analog clock time, including both reading time and drawing clock faces.
Telling Analog Time
Handwriting Paper
Printable hand writing paper templates in a variety of line heights, including 3-line practice paper in both normal and wide layouts, blank story paper, and regular lined paper for older grade students. Check out the numbered blank spelling test templates!
Handwriting Paper
Graph Paper
Free printable graph paper, grid paper and dot paper for math problems, crafts, zentangling, landscape design, architecture or just simple doodling. All graph paper styles include inch and centimeter variations.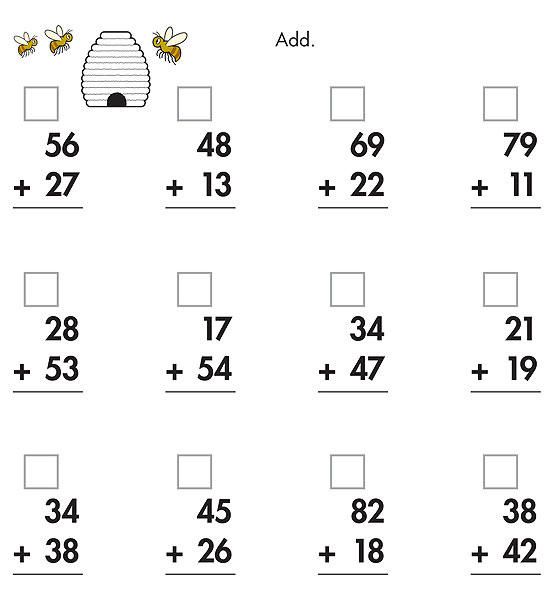
All of these PDF files are designed to print on 8.5 x 11 inch paper.
Graph Paper
Picture Math Addition
These printable worksheets use pictures and grouping to build a conceptual understanding of addition. These worksheets start out with simple addition picture problems where only basic counting skills are required to come up with addition number sentences, but later worksheets require students to produce a similar grid illustration to demonstrate their understanding of addition concepts. These are a perfect first instruction to addition for students in preschool, kindergarten or first grade.
Picture Math Addition
Picture Math Subtraction
These printable worksheets use pictures and grouping to build a conceptual understanding of subtraction. These worksheets start out with simple subtraction picture problems where only basic counting skills are required to come up with subtraction number sentences, but later worksheets require students to produce a similar grid illustration to demonstrate their understanding of subtraction concepts.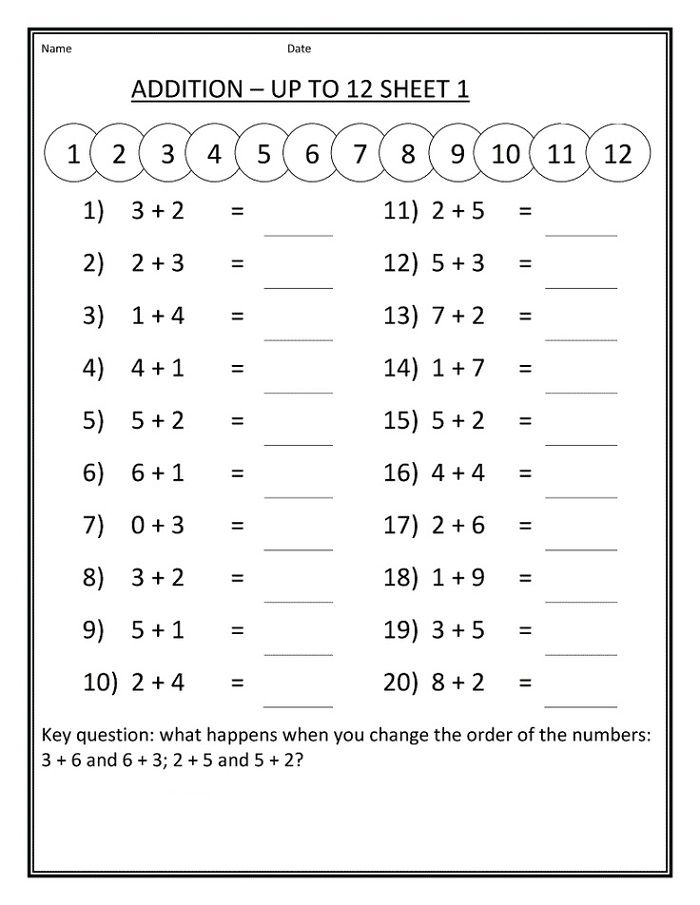
These are a perfect first introduction to subtraction for students in kindergarten or first grade.
Picture Math Subtraction
Picture Math Multiplication
These printable worksheets use pictures and grouping to build a conceptual understanding of multiplication. These worksheets start out with simple multiplication picture problems where only basic counting skills are required to come up with subtraction number sentences, but later worksheets require students to produce a similar grid illustration to demonstrate their understanding of multiplication concepts. These are a perfect first introduction to multiplication for students in second grade, third grade or fourth grade.
Picture Math Multiplication
Money
These printable money worksheets feature realistic coins and bills in problems for identifying coins, making change, counting coins, comparing amounts of money. They build foundational recognition and counting skills in Kindergarten and first grade to prepare for full money practice necessary to pass second grade.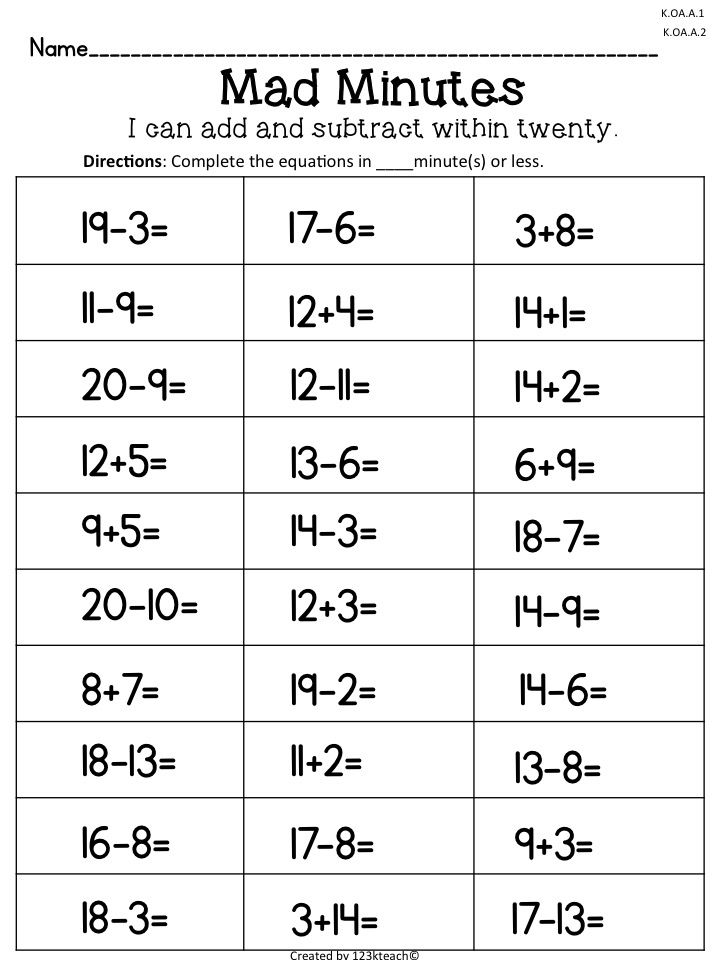
Money
Spaceship Math Check-Off
Spaceship check-off pages (complete with the Spaceship ship!) for tracking progress on the Spaceship Math or Rocket Math worksheets for each of the four basic operations.
Spaceship Math Check-Off
Color By Number
These coloring worksheets feature simple color by number instructions for young students who are either just learning their numbers or as a reward activity for older kids. You'll find a growing set of holiday and seasonal themes that I'll be adding to over time... Please check back often for updates, or if you have a suggestion send me a note at the contact link below!
Color By Number
Valentine's Day
Looking for worksheets to make learning math on Valentine's Day a bit more fun? This page has a collection of color by number worksheets appropriate for kindergarten through fourth grade, covering addition, subtraction, multiplication and division operations.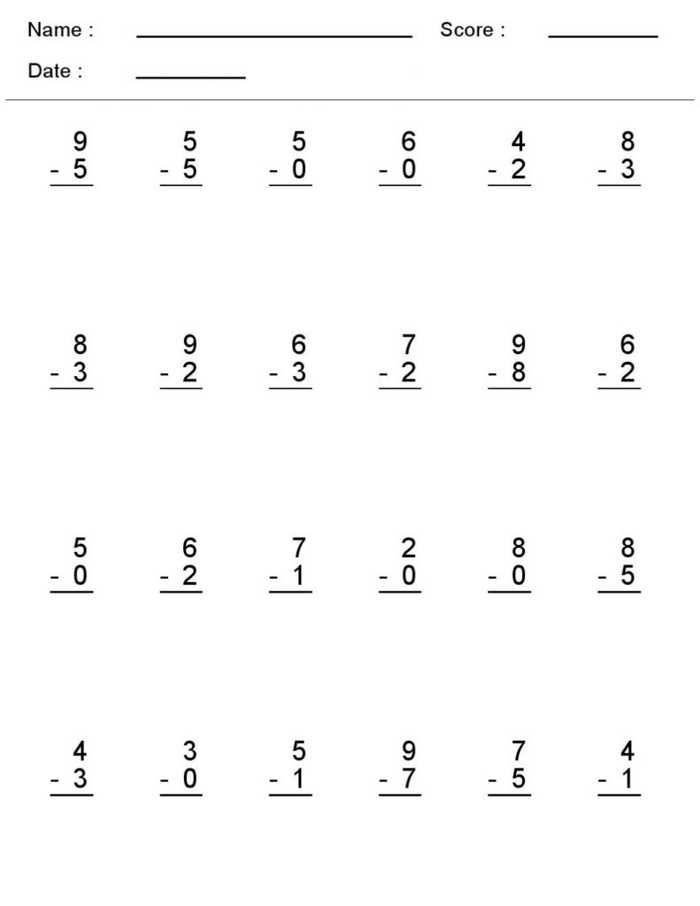
There are also a collection of simple math exercises with fun Valentine's Day themes.
Valentine's Day
Earth Day
Looking for worksheets to make learning math on Earth Day a bit more fun? This page has a collection of color by number worksheets appropriate for kindergarten through fourth grade, covering addition, subtraction, multiplication and division operations. There are also a collection of simple math exercises with fun Earth Day themes.
Earth Day
St. Patrick's Day
You can't rely solely on the luck of the Irish when it comes to math, but these St. Patrick's Day do make it a bit more fun! This page has a collection of color by number worksheets appropriate for kindergarten through fourth grade, covering addition, subtraction, multiplication and division operations. There are also a collection of simple math exercises with fun St. Patrick's Day shamrock themes.
St.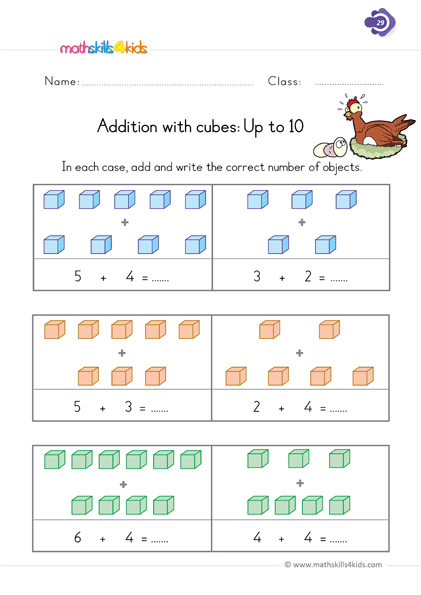
Patrick's Day
Spring
What better time of year to start growing some new math skills than Spring! This page has a collection of color by number worksheets appropriate for kindergarten through fourth grade, covering addition, subtraction, multiplication and division operations. There is also a collection of simple spring math worksheets with fun spring flower themes, along with a multiplication chart, hundreds chart, graph paper and coordinate plane!
Spring
Word Search Puzzles
Use these math word search puzzles to introduce vocabulary and terms to grade school students as they are introduced to new math concepts! These word search puzzles include sets for various Common Core aligned grade levels, along with specific topics for geometry, algebra and more!
Word Search Puzzles
First Grade Math Worksheets
1st grade math is the start of learning math operations, and 1st grade addition worksheets are a great place to start the habit of regular math practice.
The math worksheets in this section are selected especially for first graders and working with them will build a solid foundation for math topics as students move on to higher grades. Students who master addition in first grade might get an early jump on subtraction math fact practice, and may even learn a few early multiplication facts. This is an exciting time for students learning math, and keeping the challenge and enthusiasm for math at a high level is a worthwhile endeavor for parents and teachers. The selection of 1st grade math worksheets here should be an excellent map for that journey and should provide a great headstart to 2nd grade math as well!
Topics of research papers in mathematics
Attention! To repeat and consolidate the multiplication table and division table, we offer our game programs Multiplication table in cartoons and Division table in cartoons.
This page provides a general list of topics of research papers in mathematics for grades 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 and 11, which can be accessed by clicking on the links and then on the pages you can select the topic of the project most appropriate to the level of knowledge and skills of the child.
The project activity of the student begins only after the topic of the research work is approved by the supervisor (teacher).
The various topics of mathematics projects on this page can be taken as a basis, supplemented and changed in accordance with the goals of the study and the idea of the project.
- Topics for projects in mathematics for grades 1, 2, 3, 4
- Topics for projects in mathematics for grade 5
- Topics for projects in mathematics for grade 6
- Topics for projects in mathematics for grade 7
- Topics for projects in mathematics for grade 8
- Topics for projects in mathematics for grade 9
- Topics for projects in mathematics for grade 10
- Topics for projects in mathematics for grade 11
Properly chosen topics for projects in mathematics for students in grades 5, 6, 7, 8, 9, 10 or 11 contribute to the fact that work on them will be really exciting, informative and interesting.
Especially if this research project in mathematics is carried out by a group of children.
- Research topics in the history of mathematics
- Algebra Research Topics
- Logic research topics
- Geometry research topics
- Research papers on stereometry
- Research topics in combinatorics
- Research topics for mathematical games
- Topics of research papers in mathematics in subjects
The following topics for research papers and projects in mathematics are approximate, some of them can be combined into one topic if there are common tasks and goals of the study.
Topics for research projects in mathematics
Topics for design work in mathematics:
Author's tasks.
Aliquot fractions
Arithmetic of remainders. Modulo comparisons.
Without measuring stick
Without measuring stick, or measure with bare hands.
Endless world of numbers.
Divine number
Letter in a cube.
Quick account - easy and simple!
Quick calculation without a calculator.
In the depths of centuries, or As the ancients believed.
In the world of time (collection of creative tasks).
In the world of puzzles and labyrinths.
In the world of amazing numbers.
In search of optimal solutions.
In the realm of giant numbers.
Omnipresent mathematics.
Great tasks
Magnificent seven
Magnificent numbers.
Types of tasks for logical thinking.
Types and properties of movements.
Types of word problems and their solution.
Influence of the speed of falling raindrops on the speed of human movement during rain.
Harmony of law reigns in everything...
Time and its measurement
Time cannot be stopped, but can it be measured?
Time to work and time to rest.
Everything is a number
Everything about the "three" and a little more.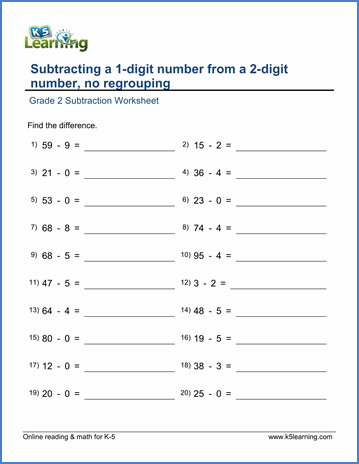
..
Everything about the number 13
Everything about the number 7
Is it always 2 x 2 = 4?
Calculation of the speed of the river.
Gallery of wonderful numbers.
Gallery of numerical curiosities.
Harmony and mathematics
Genetic code and Pythagorean square.
Geography of numbers
Hypothesis about the origins of the golden section.
Match Puzzle
Graphic methods and geometric considerations in solving problems in mathematics Graphic techniques in solving problems in mathematics.
Graphical method for solving plot problems.
Graphical way to multiply numbers.
Two ways to solve logical problems
Actions on numbers in different number systems.
Zero's birthday
Pi's birthday
Children's tasks for adult children.
Old Russian problems
Ancient number systems.
Ancient but eternally young prime numbers
Friendly triplets of numbers.
Friendly numbers
Heat of cold numbers
Living mathematics
Living nature and symmetry.
Paper strip mystery.
Riddle of Ramanujan
Riddles of the number series
Mysterious world of numbers.
Tasks from an old textbook.
Problems from Ethiopia
Problems for all occasions
Problems for the movement of two objects.
Problems on the movement along the river
Problems on checkered paper. Peak formula.
Problems based on local material
Tasks for the largest and smallest values of quantities and methods for their solution.
Problems for optimization
Problems for liquid transfusion.
Cutting tasks
Outdoor tasks.
Problems for parity
Problems about labyrinths
Problems about four colors.
Topics for research projects in mathematics
Topics for research projects in mathematics:
Tasks of increased difficulty "on the move".
Tasks with restrictions.
Problems with the same numbers.
Problems with parameters
Problems with matches
Old and old problems.
Problems that could become theorems.
Wonderful numbers. Friendly numbers and twin primes.
Entertaining logic in mathematics
Entertaining problems
Entertaining problems of the distant past.
Entertaining problems in mathematics.
Entertaining numbers
Zanimatika
Entertaining flocks of prime numbers.
The origin and evolution of a mathematical problem.
Why does a person need measurements at different times?
Familiar and unfamiliar magic number Pi.
Acquaintance with symmetry
Measuring time.
Isoperimetric problem, or Dido's problem.
Exploring the possibility of using drawing in mathematics lessons.
Interesting and fast ways and methods of calculations.
Interesting and intellectual puzzles.
The art of guessing numbers
Use of mathematical cutting games.
The use of some provisions of number theory for solving problems of increased difficulty.
The use of ancient measures of length and weight for solving and compiling problems.
Study of mathematical abilities.
Study of the method of solving problems in various ways.
Study of a series of natural numbers.
Calculating time
How big is a million?
How to measure time?
How to measure the distance between relatives.
How to find a solution to the problem
How to cut a pie?
How to count without a computer and a calculator.
Calendars of time
Calendar distance of centuries
Calculators.
Square wheel - truth or myth?
Contact numbers and the thirteen ball problem.
Piggy bank of non-standard tasks in mathematics.
The Queen of Mathematics
Beautiful and fast ways of computing
Beauty in symmetry
Beauty and mathematics
Beauty through the prism of science
Cryptograms - cryptography of the past, present and future
Cryptography
Cryptography and cryptanalysis.
Cryptography and mathematics
Cryptography and steganography.
Cryptography as a method of encoding and decoding information.
Cryptography, mathematical algorithms for encryption.
Cryptography. The basics of encryption and the history of development.
Cryptography. Methods of its practical application.
Cryptography. Science of ciphers
Crystallography and mathematics
Winged mathematical expressions.
Curiosities, sophisms, paradoxes in mathematics.
Deft compasses
Magic secrets of the number 7
Magic numbers
Magic numbers in nature
Magic numbers and figures
Magic number "Pi"
Magic number of Scheherazade.
The magic of numbers
The magic of numbers 3, 11, 13
Mathematics in life: calculation of the repair work of the premises.
Mathematics in my future profession.
Mathematics around us
Mathematics on the chessboard.
Prodigy mathematicians
Mathematical processing of experimental data.
Mathematical formula of beauty.
Math Gems
Math Presentations
Mathematical sophisms.
Mathematical terms.
Mathematical billiards.
Mathematical calendar for schoolchildren.
Mathematical pendulum
Mathematical assistant
Mathematical modeling of the global development of mankind.
Mathematical modeling and its practical application.
Mathematical modeling as a way to solve problems (problems).
Mathematical modeling of the environment.
Mathematical modeling.
Mathematical description of random phenomena.
Mathematical journey into the world of harmony.
Materials for mathematical leisure.
The world of numbers, sounds and colors
Modeling of composite tasks.
The world of big numbers.
Modeling of text tasks.
Visual topology
Unknown about the known, or How to make a discovery. Is pi equal to 4?
Some interesting dependencies.
Unusual in ordinary numbers
Non-standard problems
Non-standard problems at Olympiads in Mathematics.
Zero has a special place in mathematics.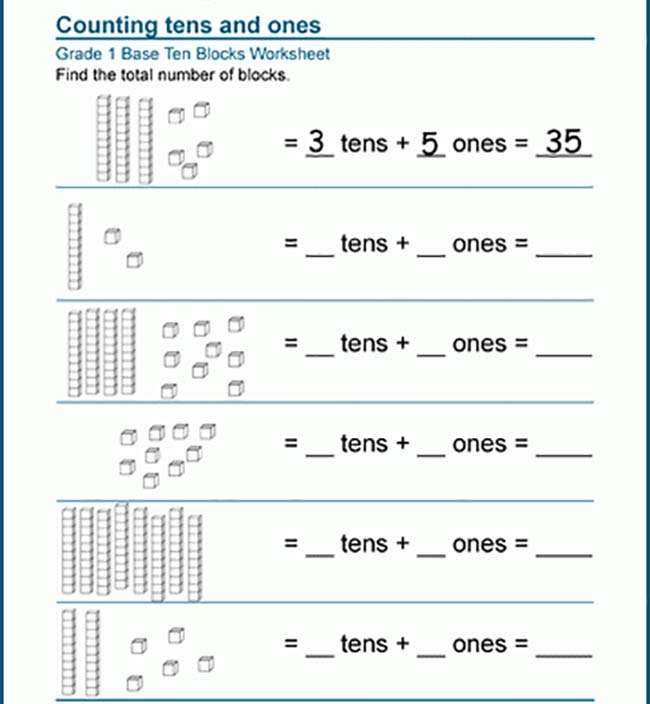
Numbering and number systems.
Numerology
Numerology - the magic of numbers
Numerology - myth or reality?
Numerology is the science of numbers in our life.
Numerology - modern science
Numerology in human life
Numerology: science or delusion?
In one stroke
Description of beauty and harmony of nature by mathematical relation.
Definition in the course of mathematics
Optical illusions and their application
Ornament as an imprint of the soul of the people.
Ornamental and geometric art by M. Escher.
Ornaments
From fingers to calculator
Discovery: coincidence or regularity?
The charm of prime numbers.
Palindromes in mathematics
Parameter. Dynamic illustrations for problem solving.
Letter with a secret
Planet of numbers
Through the pages of ageless Russian textbooks on mathematics.
Practical advice of mathematicians.
Traditions of antiquity far away (solution of ancient problems)
Devices, tools and devices for calculations.
Applied problems
Application of graphical methods in solving text problems.
The use of satellite images in the lesson of mathematics.
Pi calculation check.
Even parity check
Prime numbers
Contradiction of a consistent statement.
Journey to the origins of geometry.
Development of the concept of "infinity" in mathematics.
Talk about zero
Different ways of solving word problems.
The real world of imaginary numbers.
Recurrent relations and their application.
Solving diophantine equations
Solving problems by estimation method
Solving problems on mixtures and alloys.
Solving problems for compliance and exclusion of incorrect answers.
Solving problems according to ready-made drawings.
Solving problems on the topic "Movement along the river".
Solving optimization problems in mathematics.
Solving old problems
Solving text problems
Solving equations in integers.
The most interesting number
The secret of successful problem solving.
The seven greatest mysteries of mathematics.
Serious and funny in numbers
Symmetric prime numbers.
Number systems
Hidden modules
Perfect numbers
Perfect numbers. friendly numbers.
Perfect numbers. Mersenne prime numbers.
Reduced division using Horner's circuit.
Tasks will help you to save your health
Ways and techniques of quick calculations.
Ways of representing numbers in different number systems.
Methods for solving problems on the movement of bodies
Methods for the oral squaring of numbers.
Comparative analysis of the stability of some well-known ciphers.
Ancient problems
Ancient problems of ancient peoples.
Ancient entertaining problems
Counting without a calculator
The mystery of even numbers
The mystery of Pi
Text problems in the school mathematics course.
Text problems and modeling.
Text problems for movement
Text problems for mixtures, alloys and solutions.
Text tasks for collaboration.
Mathematics textbook: yesterday, today, tomorrow
Figured numbers
Philosophical mystery of numbers
Philosophical aspects of mathematics
Finno-Ugric number system among other systems.
Folklore problems
Whole numbers and temperature measurement.
The price of one minute
Continued fractions
Number "9" in Tuvan numerology.
Digital roots
Numbers in our life.
Numbers around us
Pythagorean numbers and the beauty of the world.
Numbers rule the world
Numbers rule the world. Is it possible to imagine a world without numbers?
Numbers with proper names.
Number P.
A number that is larger than the Universe.
Numerical inequalities
The sixth mathematical action.
Six mathematical operations.
Ciphers and cryptograms.
Ciphers and mathematics
These amazing quaternions.
Jump to sections:
Research papers in mathematics
Research methods
If you want to place a link to this page, set one of the following codes on your site or forum:
Link code to the page with topics of mathematics projects:
Mathematics research topics links to the forum with topics of research papers in mathematics:
[URL=http://obuchonok.ru/node/431]Themes of research papers in mathematics[/URL]
If you like the page, share it on social networks:
About us - online courses and lessons in mathematics
About us - online courses and lessons in mathematics8 (499) 348-18-12
8 (800) 775 26 71
Calls within Russia are free.
Daily from 9:00 to 21:00
What does it mean to learn mathematics?
To learn mathematics is to learn how to generate an idea for solving a problem.
Everything else that is done in mathematics lessons is serviceable in relation to this educational goal, which is facing both the student and the teacher. For example, if a child is asked to solve an unfamiliar problem, then we will hear in response “I don’t know” or “we didn’t pass”. But if I know how to solve the problem, then all the mathematical work has essentially been done, only the technical work remains. Creative work just consists in not knowing how to solve a problem, to find a way to solve it. This is the essence of scientific research and the main goal of teaching mathematics.
The main pedagogical task
For the vast majority of people, unfortunately, there is a belief that there are two kinds of brains in the world. One is capable of mathematics and the other is not capable of mathematics. Therefore, the main pedagogical task is to convince a child or an adult that his abilities are not limited, that there is only one kind of brain - the highest, and to help everyone become reasonably confident in their abilities.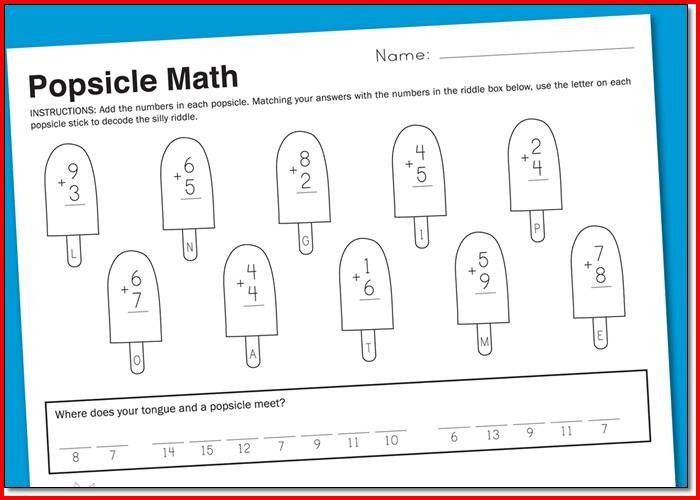
Intrinsic motivation
Why is interest more important than knowledge and grades? When a child is interested, he develops intrinsic motivation. This is a long-term solution to the learning problem. If we catch and develop interest, then the child begins to learn independently and overcomes any difficulties with enthusiasm. The triggering mechanism of interest is based on subtle tools. External incentives in the form of praise and evaluation do not work well. Emotional contact between an adult and a child, non-verbal approval and support is needed. In addition, the child himself must make sure that he succeeds.
Catch the child's interest
We present the child with the widest possible choice of types of visual tasks. It is visual tasks that form the basis of the first stage of acquaintance with mathematics, regardless of the age of the child. There are an infinite number of visual types of problems. We present the child with a choice of at least 20 types of tasks outside the school curriculum.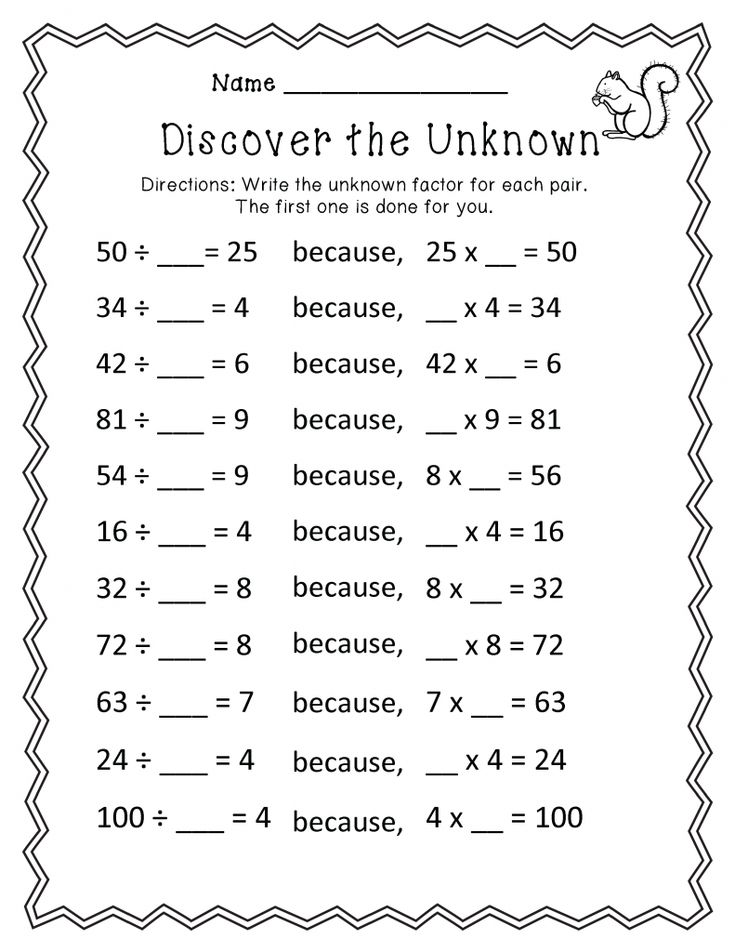
Among the variety of tasks, the child will surely find something that will interest him personally, and his positive attitude towards mathematics is further built on this.
Visual tasks
The same tasks in the mathematical sense of the word can be perceived by students in completely different ways due to different design. There is a type of problem that decisively surpasses all others in its value at the stage of the student's acquaintance with mathematics. Precisely because they are very emotional, and solving them is a very emotional matter.
They also have other advantages:
- the level of difficulty of visual tasks varies from very simple to very difficult within the same topic, which allows you to work with children of different ages and levels at the same time
- the solution of a visual problem is at the same time its design
- they evoke an emotional response
- visual tasks are understandable for those who cannot read or understand the text poorly; they allow not to be distracted at the first stage by the meaning, but to immerse themselves in the process of finding solutions
- educate the culture of figurative thinking
- help form the habit of further translating text tasks into visual images
- visual tasks allow you to work in the zone of proximal development, which is determined by the child himself.
Visual problems have many solutions, which we will talk about later.
The images themselves remind of themselves, that is, the child will continue to solve these problems even when he leaves the lesson, and thus the process of insight and search for a solution will continue and, like a real mathematician, will teach the child to think about solutions in the future and return to the problem in the imagination.
Let's start without explanations
Mechanical memorization and the desire to work out algorithms leads to the fact that when solving problems, children do not try to develop their thoughts, but feverishly begin to substitute algorithms they know without delving into the essence of the problem. That is why we do not explain right away, but let the children face the situation of finding a solution. We form the habit of putting forward our ideas, we give the opportunity to see many options for other children, and only then we discuss, analyze these solutions.
Very often, the habit of using ready-made algorithms leads to the fact that children do not find the right one and fall into a stupor if they encounter even a slight change in the conditions of the problem.
In life, the process of solving a problem is
completely different:
- we almost always have many solutions
- no ready-made algorithms
- There are no sufficient conditions that need only be substituted to get an answer. In practice, we are faced with the fact that conditions are either redundant or
insufficient
- we are not instructed in advance how to solve, we are not prepared for what task in life will appear tomorrow and we are not allowed to work out the same situation several times
- life sets us unpredictable tasks every day
- moreover, not all tasks in life must be solved immediately, there is time for reflection
- task conditions may change frequently
- we ourselves pose questions to the tasks in life
We do not “work through” the topics, but return
to visit them as “to grandma for pies”
If the material is learned well, the child will remember it in two days, and in two months, and in two years.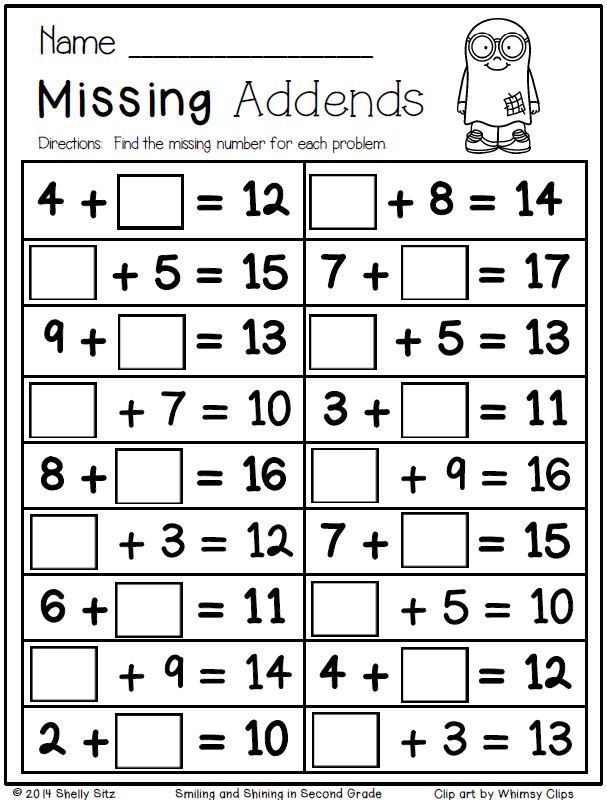
But how can this be achieved? Someone believes that for this you need to carefully go through the material during the time that is allotted for this during the curriculum, that is, “work out” the topic. Matclass offers a different approach. A new topic can be studied, for example, for 5 minutes. And then within 5 minutes - tomorrow. And the day after tomorrow. Since mastering mathematics is a long-term process, you can return to this topic in six months, and in a year, and in two - gradually complicating it. Therefore, in a very lightweight form, in a playful way, kids can be given material from both high school and high school. Gradually, the tasks need to be complicated. Therefore, in a few years, the material for the child will become familiar, but at the same time it will remain interesting, just like pies from a grandmother. Creative thinking is formed through a wide variety of associations. The more diverse associations, the stronger and more diverse the neural connections, that is, the speed of thinking, intuition.
Children need to be surprised, to show them familiar topics from completely unexpected and different sides. It is important that desire and interest always prevail over routine. And then “even work will turn first to Vanyusha with its elegant side” (N. Nekrasov “Peasant Children”)
Children compose problems
There are a lot of students (about 20–30%) who are very fond of composing problems. They come to a real interest in mathematics through problem solving. It is especially good when the teacher, parent and student compose tasks for each other. Writing problems has become a natural part of the work in Matclass and provides many advantages. Do I need to learn how to write problems? Maybe it's everyone!
A math teacher is a teacher
of everything in the world
It's really good when your teacher is the teacher of everything in the world. And a mathematics teacher is able to build bridges to all sciences due to the universality of the techniques that he teaches.
Matklass has experience in teaching history with the help of mathematics, and on field programs physics, geography, art, and sports were intertwined with mathematics. The interweaving of sciences is becoming an exciting route for education and self-education.
Time to play
Sometimes children like to sit under the table, covering it with a blanket from all sides, some kind of house appears. And it may turn out that a student who does not want to do mathematics, sitting at the table, in a hut, will do it with interest. The game provides an opportunity not only to emotionally engage, but also to move and switch. During movement, the brain works more actively, and at the moments of a change in activity, a subconscious linking of scattered information may occur and the idea of solving a complex problem is born.
Mathematics lesson - a place for
experiments and questions
Experiments are the best way to develop thinking, and the ability to ask questions teaches children to think deeply.
Boring classes
Mathematics lessons should not always be interesting. Sometimes classes have to be boring and even boring. Because math is work. We gradually accustom the student to the fact that it is useful to make efforts and do not only very interesting, but also very boring things.
Starting with the simplest
Sometimes it is important to give a child an elementary task. Such that he had the thought “yes, it’s very simple.” Sometimes you need to return to the simplest tasks after the difficult ones. This builds self-confidence.
It's not too late after three
Thinking can be developed at any age. Very often we hear from adults “I myself would like to teach mathematics like that”. Lessons are open to parents. We work not only with children, but also with adults.
Help your child change their perception
of their abilities
Many adults have insecurities about the child's learning.
That is why parents' assessment of a child's abilities very often depends on the teacher's assessment. In this sense, it is important for the teacher to constantly help both parents and children believe in their unlimited possibilities and help the child to positively perceive himself.
Performer or strategist?
It can be interesting for elementary grades when you explain to them some material for the senior grades - for the 9th, 10th, 11th or 1-5th year of the university. Of course, far from everything can be explained there, but there is such material that is available even to a first grader. In fact, we trust the child to choose the learning strategy!
Age groups
No matter how hard the supporters of testing and selection try to create “equal” classes, leaders and laggards appear in each such “equal” class. When the main task is to introduce children to a variety of tasks, and not to present in finished form the tools, algorithms and knowledge that are needed to solve these problems, splitting by level of knowledge and age does not make sense.
The extraordinary thinking of the younger ones helps the older ones, all children better show and develop their personal qualities. They are more curious, not afraid of difficulties, persistent and willing to take risks. A benevolent psychological atmosphere appears, the habit of learning from each other, the little ones feel more confident solving problems together with the elders, and the older ones have the opportunity to repeat the topic with the younger ones. Everyone becomes the first in different tasks and areas of mathematics, children get used to seeing different solutions, which in the end develops their thinking. In the age group, children constantly change roles in the classroom, try different levels of complexity. An intellectual environment for development is born in a group of different ages.
More effective in a group
Communication and teamwork are two key skills that are in demand along with professional skills. Sociable children are more capable of self-learning.
If we discard individual extreme cases when individual lessons with a tutor are necessary for special reasons, group lessons, built taking into account individual interest, bring more benefits to the child.
- feel like part of an intellectual community
- form the habit of looking for many solutions
- develop the ability to interact in a team
- learn from each other
- develop your range of interests through group work
- form internal self-assessment
- move from education to self-education
And how to evaluate the results?
In the traditional approach, the object of the current assessment is knowledge, the ability to solve certain types of problems, speed of work, accuracy of design, perseverance, homework. How can you assess the level of development of thinking and interest in the subject? According to parents, children begin to do homework on their own with pleasure, new words, special terms appear in the lexicon, quick wits, a desire to discuss tasks are clearly manifested, children begin to calmly respond to mistakes and do not stop if something does not work out.
In other words, such changes cannot be overlooked!
Are grades improving at school?
Yes, but some time must pass. For each child, the period is individual. Self-confidence comes naturally and gradually. Starting with visual problems, step by step, everyone can learn the art of understanding textual and logical problems. Thanks to the habit of working at their own pace, children learn to work without mistakes and double-check their actions on control and test work. We do not promise miracles. We are waiting for joint work - the child, parents and teachers. He will be joyful and creative. Without stress, tears and coercion. Sometimes, it is important for a child to give an elementary task. Such that he had the thought “yes, it’s very simple.” Sometimes you need to return to the simplest tasks after the difficult ones. This builds self-confidence.
Lesson program
- 1. Visual problems with many solutions
- 2. The art of reading the conditions of problems and choosing an approach to their solution
- 3.
Oral operations with numbers. Multiplication of three-digit numbers, raising to a power, decomposition of a number into prime factors - 4. Visual geometry and stereometry. Tasks for transformation, construction of the simplest sections of polyhedra - a cube, a pyramid, a prism - and more
- 5. Number systems
- 6. Codes
- 7. Combinatorics
- 8. The concept of equations and inequalities
- 9. Matrices
- 10. Elements of vector algebra
- 11. Algorithms
- 12. Elements of set theory
- 13. Boxes
- 14. Euclidean and non-Euclidean geometries
- 15. History of mathematics
- 16. Types of evidence. Evidence to the contrary. Method of mathematical induction. The evidence is direct and indirect. Comparison of mathematical proof with proofs of other sciences.
- 17. Mathematical games and games with elements of mathematics
- 18. Mathematical experiments
- 19.
Writing tasks - 20. Auxiliary disciplines in relation to mathematics
- And this is just a small tip of the mathematical iceberg that children can see in the classroom in Matklass
Participation in the Olympiad
Participation in the Olympiad becomes a natural consequence of creative activities and depends on the personal desire of the child.
About Matklass
Matklass was conceived and created as a community of active parents and creative mathematics teachers with the aim of giving everyone the opportunity to receive a versatile mathematical education. We want to help everyone believe that mathematics is part of the culture, it manifests itself in all areas of life. Matclass brought together parents and teachers of mathematics in order to captivate children together, vaccinate them against boring school mathematics and help them move from education to self-education.
Mathematics can teach you a lot. You can just learn the formulas, or you can learn to set yourself new tasks and look for their solutions.
You can learn to act according to the rules, or you can learn to make rules and algorithms yourself. You can follow someone's instructions, or you can create a program yourself and execute it. It all depends on your goal. Mathematician is a creative profession. When we decided to open the first circle of fascinating mathematics Matclass, we thought that we just needed to find a good teacher. But gradually we realized that there are very few good teachers, and the path from children's interest to the internal motivation and talent of the child lies through creativity. And that is why children need creative teachers. So the system of retraining of teachers was born.
We set a goal - to make sure that there are a critical number of creative mathematics teachers, and now we are teaching
teachers and opening circles all over Russia.
Join
Ordinary math teachers have such a curse - "humanitarian mindset". Surely you heard him? They consider this a polite address, but the person who hears it immediately understands that he is being called a fool.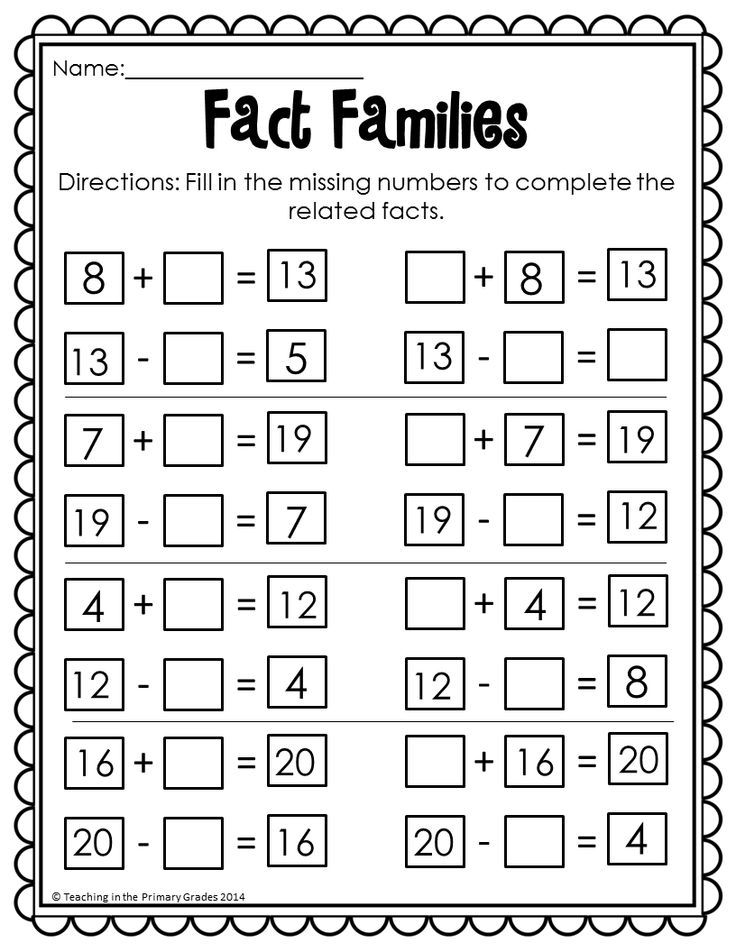
I once met a successful businessman. He gave the impression of an absolutely self-confident person, he achieved a lot in life. When, in a friendly atmosphere, we talked with him, he said that at school he always had deuces in mathematics. And suddenly he asked: "Tell me, am I mentally complete or not?" ... and began to cry ... I carried this shock through my whole life. Mathematics has disfigured this man morally. All further successes did not convince him of his own usefulness. And he carried his uncertainty somewhere in the subconscious throughout his life. Unfortunately, most people who come into contact with mathematics get the impression that there are two kinds of brains. One variety is “math brains”, the other variety is all the rest. This is the deepest delusion! But, unfortunately, most people take this self-doubt out of math lessons and stay with it for life.
Khmelinsky Petr Vadimovich
Methodological leader of the Matclass and the Mathematical Center.
Therefore, the first duty of the teacher of mathematics is to instill in the student a justified idea that there is only one kind of brain - the highest.
