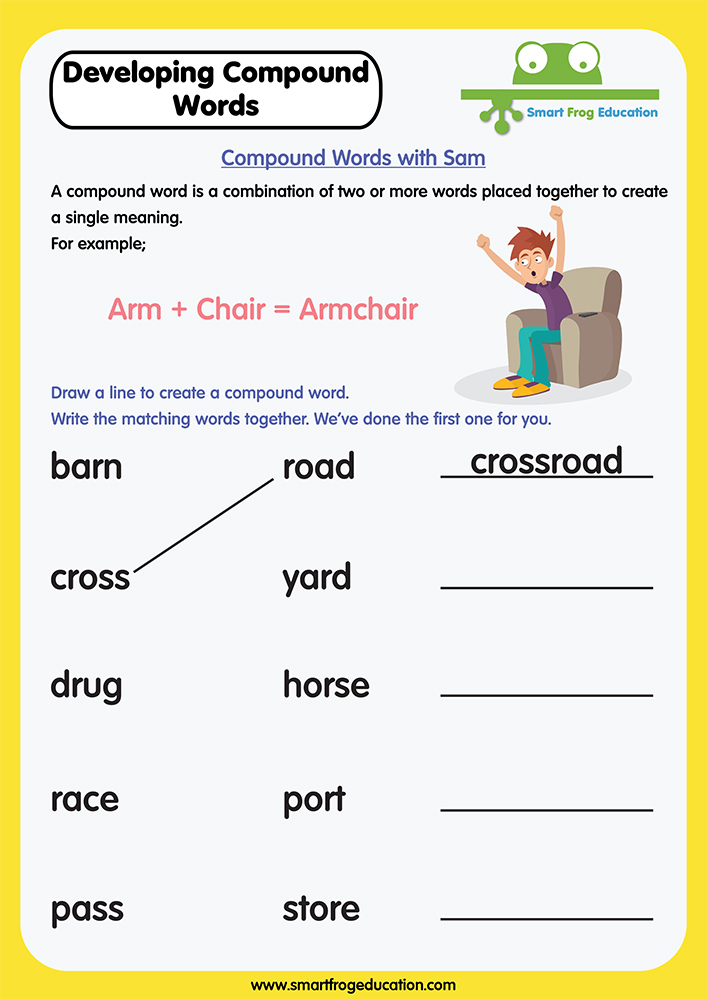
Math your way
Mathematics Their Way - The Curriculum Choice
Mathematics Their Way is a textbook written by Mary Baratta-Lorton and published by the Center for Innovation in Education, and later by Addison-Wesley (1995). The text grew out of her desire to mathematically reach all of the children in her inner city classroom, especially those who had not had much experience with math or who did not possess a natural penchant for math. It is intended for use by kindergarten through second grade, and utilizes a hands-on, activity-centered approach to teaching basic math skills for these levels.
Since it relies on hands-on activities, there is only a teacher’s book, though a collection of newsletters written by Mary Baratta-Lorton have also been collected and published as Summary Newsletter by Addison-Wesley. There are several other available resources, including a collection of full-size black-line masters.
The textbook is organized topically. It opens with a chapter discussing methods for free exploration of the math manipulatives and materials, then continues, covering pattern (three chapters of increasing difficulty and depth), sorting and classifying, counting, comparing, graphing, number at the concept level, number at the connecting level, number at the symbolic level, and place value. While the topics at the beginning of the book are more basic than those in the second half, it is not a book to be approached strictly chronologically. Rather, it is intended as a buffet of math activities for the young learner.
Structured for use in a classroom of students participating in learning stations and full-class math experiences, multiple chapters may be utilized at the same time. The author included a loose schedule of chapters by grade, along with a general checklist of activities for the teacher, but the choice of activities is up to the family and situation.
Many activities draw from a variety of collected objects which serve as manipulatives. The items used are listed in a glossary at the back of the book. At first glance, the glossary of materials appears to be extensive, but the author’s intent was for the items to be every-day, easy to find objects. In fact, she asked her students to bring in many of them. So, while the items are not necessarily hard to locate or duplicate, and certainly do not have to be expensive, there is quite a bit of gathering to be done. I suggest that you gather most of them before beginning.
I suggest that you gather most of them before beginning.
There are a few things to know about the book. It was produced for classroom use, and at first glance, many of the activities seem contingent upon a room full of students. I have not found this to be a major problem. In our home school, we have adapted the activities to accommodate one or two learners, instead of the classroom for which it was initially written. There are a couple of activities that I have by-passed due to the number of students necessary to complete them, but by and large, the hands-on nature of the text has been a huge plus for my children. It has enabled them to truly learn math concepts, rather than rote math facts without any real basis.
Additionally, the book has tempered my natural impulse, which would have been to lean heavily on a workbook or more traditional textbook since I am not entirely comfortable teaching math. The program has encouraged me to let my children develop a more complete understanding of numbers and processes independent of paper math. My daughter has been allowed to develop an understanding of numbers and numerical processes without the burden of writing. Now that we are transitioning into paper math, the concepts are cemented enough that my daughter recognizes the processes that she is describing in writing and is comfortable working with them. This textbook leads to understanding, and only then to recording math.
My daughter has been allowed to develop an understanding of numbers and numerical processes without the burden of writing. Now that we are transitioning into paper math, the concepts are cemented enough that my daughter recognizes the processes that she is describing in writing and is comfortable working with them. This textbook leads to understanding, and only then to recording math.
In our case, I spent the summer before my daughter started Kindergarten gathering materials. I made it a family affair, so aunts and grandmothers pitched in as well, sending items as they found them. I decided to collect lids, small cars, small animal figures, erasers, beads, buttons, twist ties, plastic bread ties, and small acorns. I also worked on putting together the other necessary supplies. The things that I couldn’t find, I either substituted with something else or checked to see if they were really necessary. Then we got started with the hands-on math experience. We counted, sorted, classified, compared, and graphed using a variety of activities and methods. The kids—because they all wanted to play with the new “toys”—didn’t even realize that they were doing math. It seemed more like playing with Mama. And it was fun. And it was not high pressure. And my kids always got to “play” until they understood the math idea. And this Mama who wasn’t extremely comfortable teaching math, did just fine!
The kids—because they all wanted to play with the new “toys”—didn’t even realize that they were doing math. It seemed more like playing with Mama. And it was fun. And it was not high pressure. And my kids always got to “play” until they understood the math idea. And this Mama who wasn’t extremely comfortable teaching math, did just fine!
For Kindergarten, we used the activities in Mathematics Their Way almost exclusively. (I will mention that though I opted to use this book as the primary text for Kindergarten and first grade, it would be a lovely supplement to any math program. Its buffet-style, activity-laden nature could easily be gleaned for activities to complement math topics as they were studied.) For first grade, we have continued using Mathematics Their Way, but I have added several living math books every week or so, choosing subjects that build and develop mathematically. We have even written a few things down, as we slowly transition into paper math. Even though I plan to use a textbook for guidance next year, and to include a lot more recording of math concepts, I will continue to use the hands-on approach that I have learned with Mathematics Their Way, because I think that it makes math “real” to little learners.
Unless you have a friend to loan you a copy, the text can be difficult to find in print for a reasonable price. At the time of this writing there were ten or twelve on ebay, varying in price from $35 to $110. However, the Center for Innovation in Education maintains a website, which includes a PDF version of the text that is intended for previewing, as well as an on-line version of the summary newsletter. They also offer the print version of the book for $57.95, a materials kit, intended for classroom use, for $139, and a variety of other support items.
For young mathematical minds, I highly recommend Mathematics Their Way!
Susan is a homeschooling mom of three—a preschooler, an almost kindergartner, and a first grader. They spend their days reading on the couch, playing with numbers, and making big, fun messes in a Spirit-led, Well-Trained Mind-inspired classical-Charlotte Mason-traditional model of home education.
Susan S. (26 Posts)
Susan currently home educates a third grader, a first grader, and a nearly Kindergartner. Her background is public school-traditional, but she and her husband are drawn to the classical emphasis on order and the logical accumulation of knowledge. They have added in Charlotte Mason’s emphasis on living books, nature study, and artist and composer study, since it offers such a beautiful complement to the sometimes heavy feel of classical. They even do a little lapbooking and notebooking at their house. Finally, they have covered all of this knowledge-acquiring with prayer, asking the Great Teacher to lead and instruct their family in His Way.
Her background is public school-traditional, but she and her husband are drawn to the classical emphasis on order and the logical accumulation of knowledge. They have added in Charlotte Mason’s emphasis on living books, nature study, and artist and composer study, since it offers such a beautiful complement to the sometimes heavy feel of classical. They even do a little lapbooking and notebooking at their house. Finally, they have covered all of this knowledge-acquiring with prayer, asking the Great Teacher to lead and instruct their family in His Way.
Math Your Way To Victory. The Legendary game team has released… | by Alex Berg
The Legendary game team has released the formulas that dictate how much damage is done by individual heroes in a battle. We’re going to go through it term by term and calculate a hypothetical attack so that we can see how everything works.
By better understanding the interplay between attack, damage, power gems, match combos, you’ll be able to build stronger, faster decks.
Here is the final equation for how much damage each hero does on an attack:
Total Damage = ATK Value * Total Match Value * Combo Multiplier * Affinity Modifier * Leader Bonus * Damage Bonuses * Slayer Bonus — Enemy Armor
Some of those terms have their own equations associated with them with their own terms, and we’ll tackle those as we come to them.
That first term, ATK Value, is one of those, so we’ll tackle it now:
ATK Value = Base ATK Stat * (1 + sum of all % ATK Increases + 0.25 * total number of Commander Gem bonuses in all matches) + Flat ATK bonuses
ATK Value: This is what happens to a hero’s attack stat after it’s been modified by all the various things in a battle that can tweak attack. Let’s go through each of these terms and plug in reasonable values so that we can see how an ATK Value comes together.
Base ATK Stat: This is the attack stat in the lower left hand corner on the front of a hero’s card.
Though these values can vary widely, we’re going to presume a Base ATK value of 2500 for our hypothetical hero.
Sum Of All % ATK Increases: Percentage ATK increases can come from Battle Skills, Passive Skills, or Relic Effects. No matter what their source, all ATK increases are added — NOT multiplied — to determine how much they affect the outcome of the ATK.
“Skills and relics are pretty specific if they are giving an ATK modifier (most relics) or a DMG modifier (most leader skills),” says James Marr, Executive Producer of Legendary.
“One important note is that ATK Scaling and DMG Buff stack with each other differently. ATK multipliers are added together (so two scaling relics with +45% add together for a +90%) but DMG Buffs multiply together (so two leader skills with 300% multiply together to 900%).”
Just remember that ATK and DMG are treated differently by this equation. For sake of our current example, we’ll use the example of two 45% attack buffs combining for a 90% buff, which we’ll represent in our equation with a 0. 9. We’ll return to damage buffs later on.
9. We’ll return to damage buffs later on.
Total Number Of Commander Gem Bonuses In All Matches: This term comes into play only during Commander events. During Slayer events, GVG, Forge Boss fights or any other battle, this term reduces to zero.
Commander Gem Bonuses are affinity-specific. In a situation where you have an Earth Affinity 4x gem in one match and a Water Affinity 4x gem in another match, this term would not be eight — it would be four for your Earth heroes and four for your Water heroes.
For our example, let’s assume that we are in a Commander event, and have matched two 4x Commander gems and one 2x Commander gem for a total of 10 Commander Gem Bonuses.
Flat ATK Bonuses: The main kind of Flat ATK bonus you are likely to encounter is the bonus from a relic.
These bonuses can be enhanced by powering up a card outside of battle, but once a battle begins they are locked in place. Whether or not a bonus applies to a particular hero will depend on whether or not that hero matches the necessary affinity and species type.
We’ll assume for our example that our hero is receiving a 1,000 Flat ATK bonus. And yeah, we know that most six star relics start off at 937, but 1,000 makes the math ever so slightly easier to follow, so that’s what we’re going with here.
Now that we’ve got values for all of our terms, we can calculate ATK Value. So you don’t need to scroll up, here’s the equation again:
ATK Value = Base ATK Stat * (1 + sum of all % ATK Increases + 0.25 * total number of Commander Gem bonuses in all matches) + Flat ATK bonuses
…and now here’s the equation with all of the values we just defined filled in:
ATK Value = 2500 * (1 + 0.9 + 0.25 * 10) + 1000
ATK Value = 2500 * (4.4) + 1000
ATK Value = 11000 + 1000
ATK Value = 12000
We’ll come back to that number after we fill in the rest of the terms of our damage equation. As a refresher, here it is again:
Total Damage = ATK Value * Total Match Value * Combo Multiplier * Affinity Modifier * Leader Bonus * Damage Bonuses * Slayer Bonus — Enemy Armor
The next term, Total Match Value, represents all the gems that have been matched during an attack, which is represented mathematically this way:
Total Match Value = Match Value 1 + Match Value 2 + … + Match Value X
Each match of three or more gems has its value calculated separately, and then all those values are summed to give the Total Match Value. The value of each match can be calculated using this formula:
The value of each match can be calculated using this formula:
Match Value = Number Of Power Gems In The Match * 0.25 * (number of gems in the match + 1)
There are really only two terms to define in this equation, both of which are fairly self explanatory.
Number Of Power Gems In The Match: This value is NOT the combined face value of any matched power gems — it is just how many power gems were in the match. The face value of any matched power gems will be calculated in a later step. If there are no power gems in a match, this term becomes one, in order to avoid zeroing out the equation.
For our calculation, we’ll assume that we matched three power gem IVs and one power gem III, giving us a 4 for this step of the calculation.
Number Of Gems In The Match: This is the number of gems contained in any given match. Whether or not these are ordinary gems, power gems, or commander gems does not matter for this step of the calculation — if it was a part of the match, then it should be included here.
We will assume that we’ve matched 5 gems for the current attack.
We’ll also assume that the current attack only has one match, for sake of simplicity. That makes our Total Match Value equal to Match Value 1, which is:
Match Value 1 = Number Of Power Gems In The Match * 0.25 * (number of gems in the match + 1)
Match Value 1 = 4 * 0.25 * (5 + 1)
Match Value 1 = 1 * (6)
Match Value 1 = 6
A noted exception to the Match Value formula is the Match Value for a Team Up Attack, when two power gems are swapped, sucking up unmatched gems matching their affinities.
This requires a separate calculation- for our current example, we will not presume that a Team Up Attack has been made, but are including the relevant math here for sake of thoroughness.
The two power gems swapped in a Team Up Attack count as a three gem match by themselves for the purpose of calculating the Match Value, and the other gems on the board that get sucked up add to the number of gems in the match.
For Team Up Attacks, you do not multiply the number of power gems when calculating Match Value. Because of this, the Match Value of a Team Up Attack is always:
Match Value (Team Up Attack) = 0.25 * (number of gems sucked up + 4)
And since a Team Up Attack counts as its own match, Team Up Attacks add to the Combo Multiplier, the next term in our equation.
The Combo Multiplier takes into account the total number of matches made as well as the face value of all power gems matched:
Combo Multiplier = 0.75 + 0.25 * (Sum Of Power Gem Values + Number Of Matches)
Combo Multipliers are displayed in game just before an attack is madeSince we’ve already determined that we’ve made one five gem match, we know that the Number Of Matches will be equal to 1. That leaves us with only one term to define:
Sum Of Power Gem Values: This is the combined total of all the face values of the power gems contained in a given match. If a match included three power gem IVs and one power gem III, for instance, this value would be fifteen, not four.
If a match included three power gem IVs and one power gem III, for instance, this value would be fifteen, not four.
Since that’s the exact scenario we’ve already committed to, we’ll substitute in 15 for this term in our calculation of the Combo Multiplier. If there are no power gems, this term becomes zero — since there is always at least one match made, a zero in place of this term has no danger of nullifying the rest of the equation.
Knowing the values of these terms allows us to calculate our Combo Multiplier:
Combo Multiplier = 0.75 + 0.25 * (Sum Of Power Gem Values + Number Of Matches)
Combo Multiplier = 0.75 + 0.25 * (15 + 1)
Combo Multiplier = 0.75 + 0.25 * (16)
Combo Multiplier = 0.75 + 4
Combo Multiplier = 4.75
Let’s return to our Total Damage formula, both as a refresher and to spare ourselves the tedium of scrolling up:
Total Damage = ATK Value * Total Match Value * Combo Multiplier * Affinity Modifier * Leader Bonus * Damage Bonuses * Slayer Bonus — Enemy Armor
We have five terms left, none of which have separate equations attached to them, which allows us to define them a little more easily.
Affinity Modifier: This term represents the relative strength or weakness of an attacker/defender match up, and will be a .5, 1, or 2. In the case of weak matchups, such as Earth attacking Fire, the Affinity Modifier will be a .5. For strong matchups, such as Earth attacking water, the Affinity Modifier is 2, and the Affinity Modifier is 1 for even matchups, such as Earth attacking Earth.
Attacking in the direction of the arrows grants an Affinity Modifier of 2There are hero cards which can affect this value, but they are not particularly common. In instances where a battle skill strengthens one affinity’s attack against another, this term is replaced by the following equation:
Affinity Modifier = Base Affinity Value (0.5, 1, or 2) * Hero Ability Amount
For our calculation, we’ll assume a strong matchup with no modifying abilities, giving us an Affinity Modifier of 2.
Leader Bonus: If your hero is affected by a Leader Skill, then this is where it gets factored in. In situations where your hero is unaffected by any Leader Skill, the value of this term defaults to one. For our purposes, we’ll assume our hero is being buffed by a Leader Skill that grants a 300% damage boost, and use 3 for this term in our calculations.
In situations where your hero is unaffected by any Leader Skill, the value of this term defaults to one. For our purposes, we’ll assume our hero is being buffed by a Leader Skill that grants a 300% damage boost, and use 3 for this term in our calculations.
Damage Bonuses: If there are any active skills or relic effects that boost your hero’s Damage (which, again, is different from attack), then they go here. In situations where there are multiple Damage Bonuses, they stack multiplicatively, not additively like attack bonuses do.
Two 300% Attack Bonuses would add together to become a 600% bonus. But — if you had two separate 300% Damage Bonuses, those would become a 900% bonus. For our purposes here, we’ll presume that’s the case, and use a 9 for this value.
Slayer Bonus: During a Slayer event, Slayer heroes would have their Slayer multipliers factored in here. Since we’ve already determined that our attack is happening during a Commander event, we won’t deal with this factor here — we’ll use a 1.
Enemy Armor: How much defense the enemy has. Though this value doesn’t affect your damage output, it does affect how much damage you’ll actually do. Enemy Armor values vary wildly, depending on the level and type of enemy. For mid to high level bosses, 10,000 is a reasonable value — though that’s very high for campaign bosses.
Now that we’ve defined every term in the Total Damage equation, we can make a Total Damage calculation for a hypothetical one match attack with several power gems against a weak affinity during a Commander event. Here goes:
Total Damage = ATK Value * Total Match Value * Combo Multiplier * Affinity Modifier * Leader Bonus * Damage Bonuses * Slayer Bonus — Enemy Armor
Total Damage = 12000 * 6 * 4.75 * 2 * 3 * 9 * 1–10000
Total Damage = 18468000–10000
Total Damage = 18,458,000
By substituting different values in for each of the terms, you can gain a greater understanding of the potential of each of your cards, and what kinds of attacks they’re best suited for. Happy mathing!
Happy mathing!
| Anastasia Borisovna Kalinina, Evgenia Markovna Katz, Anton Mikhailovich Tilipman Moscow: VAKO, 2013. 384 p.
How can I introduce my child to mathematics? How to show that mathematics is beautiful? How not to scare, but to captivate? These and many other questions set by the authors of the book. The book contains many problems of various difficulties. The book is addressed to all those parents and teachers who are engaged in preparing children for school, primary school teachers, leaders mathematical circles, organizers of Olympiads. We are pleased to publish this book with the kind written consent of all three authors of the book. (with the rights of the publisher terminated). W.D. Arnold 0029 | Tasks | Answers and1. | Fourth excess | 6 | 190 | 2. | 70029 7 | 19000 9000 9000 | 3. 193 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 4. | Brothers and sisters | 193 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
5. | I am | 11 | 195 | 6. | from the letter to the letter | 13,0008 | 197 000 9000 9000 | 7. You | 18 | 197 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 8. | increase and decrease | 24 | 200 | 9. | sequences | 25 | 200 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 10. | Counting | 27 | 203 | 9000 9000 9000 | 9000 9000 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 12. | Playful Lyova | 31 | 208 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 13. | Evenness | 30029 210 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 14. | Narisui scheme | 36 | 213 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 15. | SPILS | 40 | 224 | 16 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 17. | Count all rectangles | 45 | 235 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 18. | Count all triangles | 9002|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 22. | 79 | 260 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
29. | Items and their properties83 | 261 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 28. | of the set and their intersections | 87 | 262 | 29. | Cleep | 9000 | 265 9000 9000 | 9000. | 265 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 31. | Tasks with matches | 102 9124 | 290 | 37. | Objects | 126 | 296 | 38. | The amount and difference are | 127 9000 297 9000 9000 9000. | 129 | 300 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 40. | Weighing | 133 | 303 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 41. | comparisons | 137 | 311 | 42. | 138 9000 | 313 9000 9000 9000 | 43. | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 44. | Calendar | 143 | 319 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
45. | Clock time | 147
FOREWORDDear teachers! Moms and dads! Grandmothers and grandfathers! Before you is not an ordinary book. We have collected in it many rather difficult problems, but we want to show that mathematics is not difficult. We have drawn more than one and a half thousand unpretentious schematic pictures, but we want to show that mathematics is beautiful. This book contains many words and thoughts, but our main thought is written in just four words: mathematics is in your hands! Our problem book is designed for ages 6 to 10, but the book may be useful for some five-year-olds, and older students. This book is available to literally everyone. It is not for geeks, although it contains a number of tasks that many adults will rack their brains over. The book consists of two parts: the first contains problems, the second contains answers and detailed solutions. Tasks are grouped in 64 sections. Each next section is usually a little more difficult than the previous one. Tasks within sections also arranged in ascending order of difficulty. Most sections are preceded by two separate introductions for adults and children. To distinguish them, the following conventions are used:
Introductions for adults are methodological recommendations, appeals to children contain explanations for the task. Many students think that mathematics is a table multiplication and hundreds of examples and tasks of the same type. Mechanical manipulations with numbers and cells in a notebook produce children have a persistent and undeserved dislike for mathematics. Our the goal is to overcome this hostility, not to let it form. With the help of this book, we are not trying to develop automatism in children, we do not set ourselves the goal of coaching them on the tasks of or some other kind. Our tasks are not templates, they do not need to be solved for speed or for quantity - they teach to reason. You can and should learn this, regardless of whether whether the child has gone to school or not yet, whether the child can read and even count. Mathematics is not only the ability to count objects and comparing numbers is, first of all, the ability to think logically. The complexity of the tasks in this collection varies widely. This gives you the opportunity to work for a child with any level of mathematical training. But one should beware of the temptation to give the child tasks as much as possible. complex, to the limit of its capabilities. Difficult tasks that stand alone cause confusion in many children, self-doubt. You can jump further from a run than from a stop, and therefore do not forget that simple tasks should not be neglected. Self-confidence helps to consolidate interest, insecurity destroys it. In many textbooks there are problems of increased complexity, the so-called "problems with an asterisk". The complexity of these problems, as a rule, are not computational - they cannot be solved using standard, pre-known patterns. One way to guess is to draw an auxiliary schematic picture. It is important to teach children to see what From a mathematical point of view, the relation "Denis is older than Grisha" means exactly the same as "Galya's braid is thicker than Zhenya's." A correctly drawn diagram reveals the mathematical meaning of the problem and significantly simplifies its solution. The scheme is able to make even a very complex task simple, and an incomprehensible and long condition short and accessible. It may even happen that the circuit itself turns out to be the answer to the problem. That's why we rate the tasks in our book on two dimensions: "difficulty" and "visibility." We traditionally designate the level of difficulty with asterisks (*), from one to five. The visibility level is denoted by the “sun” (☼) — the task can be assigned from zero to three such symbols. For many children, for solving a number of problems, additional visual material that can be touched by hands is useful: counting sticks, pre-cut geometric shapes. Sometimes other props may be required: strips of paper, paper chains, scissors, glue, stapler, matches, peas, plasticine balls. All this is useful to prepare in advance and use as needed. Problems of some types (sections 2, 4, 5, 6, 7, 8, 11, 20, 21, 29, 33, 34, 38) children can invent and themselves - for each other, for an exchange. This form of work increases interest in the subject and motivation. The main thing that adults need to remember: the child should be interested! We may not notice it, but mathematics the whole surrounding world is permeated: snowflakes and pineapples, rainbows and music - the beauty of our world is largely described by mathematics. This world should not cause despondency and hostility in children. In conclusion, we want to say that we will be grateful to everyone who will tell us how our book was used: what was the age children, the format of the classes, which sections you used in your work, which aroused the greatest interest in the children. We also We will accept with gratitude your comments, wishes and suggestions aimed at improving the book in subsequent editions.
Permanent address of this page: http://math. | Subject catalog Magazine Archives | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Olympiad “Mission Possible. Your calling is a financier!
Registration will last until November 30 →
Rating6.5
- For
- all
- 8
- 9
- 10
- 11 classes
English 8–11 grades
7.5
| Sep | Oct | Nov | Dec | Jan 2023 | Feb | Mar | Apr | May | Jun | Jul | Aug |
Oct 1...Nov 30 Oct 1...Nov 30 Registration
Dec 9...10 Dec 9...10 Qualifying round
Feb 1 Feb 1 Final stage
Informatics grades 8–11
7.-thumb.jpg) 6
6
| Sep | Oct | Nov | Dec | Jan 2023 | Feb | Mar | Apr | May | Jun | Jul | Aug |
Oct 1...Nov 30 Oct 1...Nov 30 Registration
Dec 5...6 Dec 5...6 Qualifying
Feb 3 Feb 3 Final stage
History8–11 grades
7.5
| Sep | Oct | Nov | Dec | Jan 2023 | Feb | Mar | Apr | May | Jun | Jul | Aug |
Oct 1. ..Nov 30 Oct 1...Nov 30 Registration
..Nov 30 Oct 1...Nov 30 Registration
Dec 5...6 Dec 5...6 Qualifying
Jan 23 Jan 23 Final stage
Mathematics grades 8–11
8.3
| Sep | Oct | Nov | Dec | Jan 2023 | Feb | Mar | Apr | May | Jun | Jul | Aug |
Oct 1...Nov 30 Oct 1...Nov 30 Registration
Dec 9...10 Dec 9-10 Qualifier
Jan 30 Jan 30 Final round
Social studies grades 8–11
7.0
| Sep | Oct | Nov | Dec | Jan 2023 | Feb | Mar | Apr | May | Jun | Jul | Aug |
Oct 1.

 For each problem, an answer is given, for the majority - a detailed solution.
For each problem, an answer is given, for the majority - a detailed solution. 
 Our book is not only and not so much for those who are already interested in mathematics. On the contrary, our main goal is to interest even such a child, who thinks that mathematics is difficult, boring and not for him. This book does not replace existing school textbooks, but serves as a significant addition to any of them.
Our book is not only and not so much for those who are already interested in mathematics. On the contrary, our main goal is to interest even such a child, who thinks that mathematics is difficult, boring and not for him. This book does not replace existing school textbooks, but serves as a significant addition to any of them.  This skill is needed everywhere: in biology and in linguistics, in the store and in the mountains, in the classroom and on a desert island - and that is why mathematics is fundamental.
This skill is needed everywhere: in biology and in linguistics, in the store and in the mountains, in the classroom and on a desert island - and that is why mathematics is fundamental.  "Asterisk" means the need for insight, the need to guess something new. But how do you learn to guess?
"Asterisk" means the need for insight, the need to guess something new. But how do you learn to guess?  A complex and elusive task is more difficult than a complex and visual one.
A complex and elusive task is more difficult than a complex and visual one.  Math is beautiful! Let's help children see this beauty!
Math is beautiful! Let's help children see this beauty!  ru/lib/576
ru/lib/576