Develop number sense

What do people with good number sense do?

• compose and decompose numbers to solve calculations
• compute mentally using a variety of strategies
• make reasonable estimates
• understand quantity and magnitude
• reason with numbers

What does it mean to decompose and compose numbers to calculate? Let’s look at an example. In a fifth grade class students were solving a word problem that required the product of 25 x 26. Instead of assuming everyone would find the product in the same way, students are encouraged to find a variety of methods.

Before you read on, think of some ways you might multiply 25 x 26. Students had a variety of responses:

25 x 26 is the same as 26 x 25

Think of 26 as 20 + 6 20 x 25 = 500 6 x 25 = 150 26 x 25 = 650

Another student said he did it similarly but instead of 20, multiplied by 10 twice.

Still another suggested that beginning with 30 x 25 was easier than 26 x 25:

30 x 25 = 750, but that is four 25’s too many 4 x 25 = 100 750 -100 = 650

Some other solutions included the following:

Think quarters: 4 x 25 = 100 26 ÷ 4 = 6.5 6.5 x 100 = 650

or 100 x 26 = 2600 2600 ÷ 4 (since there are 4 25’s in 100) = 650

One student suggested that 25 x 26 was too difficult, but it was the same as 13 x 50 because she doubled the 25 and halved the 26.

13 x 50 = (10 x 50) + (3 x 50 ) = 500 + 150 = 650

Finally one student said she studied a table of square numbers in the room and saw that 25 x 25 = 625 and 1 more 25 is 650.

Classrooms that nurture number sense Of course this variety and number of strategies doesn't occur naturally, but only in classrooms where students are encouraged to take risks, to look for structures like doubling and halving factors, to use known facts to figure out unknown ones, and most of all to share and examine each others’ thinking. Students work in pairs to come up with solutions, first with easier problems (think 11 x 15) then with those of increasing complexity.

Student solutions are posted, discussed, and whenever possible, generalized. (26 = 20 + 6 or knowing that 4 x 25 equals 100 enables you to multiply by 100 and divide by 4 when multiplying by 25.)

Finally, providing visual models for procedures deepens understanding. For instance an area model helps students understand the distributive property.

20 x 25 = 500 6 x 25 = 150 26 x 25 = 500 + 150 = 650

Is the answer the same as one derived with the paper pencil algorithm? Of course, but which develops number sense? Which encourages students to use properties like the commutative and distributive property? Which enables students to solve more complicated problems mentally?

Think how different it would be if students merely wrote 25 x 26 vertically and then did something like this:

26 x 25

5 x 6 = 30, carry the 3, put down 0, 5 x 2 = 10, add 3 = 13. And so on. No number sense. No place value. No idea if the answer is reasonable.

Taking the time to thoroughly examine one or two calculations in depth, similar to the above, encourages students to focus on thinking—not merely on getting the correct answer. It leads both to number sense and to underlying math concepts.

Andy Clark will be presenting at the 2017 Math in Focus Institute, July 26–28, in Lafayette Hill, PA.

5 Strategies for Developing Number Sense

Many upper elementary teachers notice a severe lack of number sense in their students, and that makes high levels of math extremely difficult for students. Number sense refers to “a well organized conceptual framework of number information that enables a person to understand numbers and number relationships and to solve mathematical problems that are not bound by traditional algorithms”. There are five components that characterize number sense: number meaning, number relationships, number magnitude, operations involving numbers, and referents for numbers and quantities. This post shares some strategies I use to develop number sense in upper elementary students. It’s certainly not too late to develop number sense with third, fourth, and fifth graders. Before we get started, I’d love to share a brief introduction to number sense video by Jo Boaler.

Hopefully, you’ll be able to use some of the ideas and strategies below to develop a number sense with your upper elementary students. More than likely, you’re already doing several of these things!

Browse the Number Sense Strategies:

1. Number Talks
2. Math Facts
3. Conceptual Instruction
4. Communication
5. Time

Number Sense Strategy 1: Number Talks

I heard about number talks several years before I ever tried implementing them. I convinced myself that they were a complete waste of my time and just another buzz word in education. However, I saw other math teachers that I greatly respected begin implementing number talks and sharing the success they were seeing. I was in fear of missing out, so I reluctantly gave number talks a try. To be honest, I did not have any positive expectations.

I am now happy to share that I was completely wrong! Number talks now have a valuable place in my classroom. My favorite number talks resource is Number Talks by Sherry Parrish. It is a long, content heavy book, but it’s not necessary to read cover to cover. I started by selecting the essential portions and teaching strategies as I learned them. One of my favorite things about number talks is that I don’t need any special resources or materials. I use the book for my lessons and my dry erase board. That’s it!

I quickly realized that number talks are an amazing routine that will help improve students’ number sense, and this is as important for upper elementary students as it is primary students. I’m sure we’ve all had students who have relied on rote algorithms to complete math problems, but these students did not understand what they are doing. We’ve all seen the same error patterns over and over again (forgetting to carry the one, subtracting from bottom to top, etc.) and these errors show how the lack of number sense impacts computation. When we develop number sense, students understand the underlying concepts of math operations.

When it’s time for our number talk, I have students sit on the carpet in front of my whiteboard. My rug isn’t quite large enough for my whole class, but we improvise the best we can. When students come to the rug, they don’t need to bring anything with them, since everything is done mentally. I use my Number Talks book to select 2-4 math problems for my students to solve for the day. I follow the order of suggested lessons in the book, but I’m sure you can skip around too! Next, I write one of the math problems on the board. I typically write the problem horizontally to discourage students from using the algorithm.

As students think about the problem, they hold their fist to their chest, and when they determine the solution, they replace their fist with a thumbs up. While students are waiting for the rest of the class to solve the problem, they should think of other strategies they could use and indicate the number of strategies they’ve though of by holding up two, three, or even four fingers to their chest. I like this procedure, because I can see who has solved the problem and who is thinking. It also prevents students from becoming intimidated by students who solve the problems quickly.

I ask students to share their answers, and I write all of the answers on the dry erase board. I then have students share how they solved the problem. As students explain their thinking, I write what they are telling me they did. After we go through the problem, students share if the strategy worked or not.

I explicitly teach the strategies from the Number Talk book before I expect students to apply that particular strategy, which is completely different from how I typically teach math. In a normal math lesson, I want students to construct their own rules and strategies, but this is a bit different. As I teach each strategy, I add them to our number talk anchor chart. The only bit of an issue that we ran into was that my students were coming up with too many different strategies. Our number talks could have gone on forever if I let them. However, I used this to guide discussions on the importance of selecting the most efficient strategy and how to determine which strategies were efficient.

To keep track of what problems I had used and who shared each day, I created forms for each strategy used for each operation. This is a great addition to a data notebook or teacher binder! You can download a free copy at the link below.

Free Number Talk Resource

Get free number resouces by signing up below!

I can say with complete confidence that number talks are playing a significant role in improving my students’ number sense. They’ve also opened my eyes to why some students continue to make the same errors again and again and why some types of problems cause students so much difficulty. Even if you only have time for them two or three days a week, I strongly encourage everyone to at least give it a try!

Number Sense Strategy 2: Math Facts

As an upper elementary teacher I do focus on the memorization of math facts. I’ve found that higher levels of math are extremely difficult for students who do not know their basic math facts. However, I’m not a proponent of isolated memorization or high pressure timed tests either. Many of us have standards that include ‘fluency’ as a goal, and this fluency comes about when students develop number sense. But isolated practice and repetition will not develop number sense in our students.

Research tells us that the best mathematics classrooms are those in which students learn math facts through engaging activities that focus on mathematical understanding rather than rote memorization. When students focus on memorization, they often memorize facts without number sense. This means they are prone to making errors and are not flexible in their thinking. The best way to develop fluency with numbers is to develop number sense and to work with numbers in different ways, not to blindly memorize without number sense. There are three strategies I use to help students learn their math facts and develop number sense.

One strategy is through my Multiplication Fact Booklets. As students work through their booklets, they gain a conceptual understanding of each multiplication fact. They are able to solve the fact with multiple strategies, begin to observe patterns, and develop a mathematical vocabulary.

Another strategy I enjoy is incorporating meaningful multiplication games into my instruction. Games are effective and eliminate the high stress that timed tests bring to many students. Math games can be played for homework, morning work, math centers, or early finishers. I’ve created a set of Weekly Multiplication Games, and we focus on one game a week.

Xtra Math

When I first started teaching, I had a firm rule that for me to consider a student fluent with a math fact, s/he must be able to solve the math fact in 3 seconds or less. Over the years I’ve learned that some students will be slower when memorizing a fact or recalling a fact, but they still have exceptional math potential. I’ve shifted my mindset to allow for flexibility and reasoning in students’ thinking. I use Xtra Math as a multiplication fact practice tool, and I customize the program so my students have six seconds to solve a problem, rather than the defaulted three seconds. This gives my students the opportunity to apply multiplication strategies they’ve learned and builds math confidence with my students. Of course, if a student is sailing through without effort, I can adjust the time to 3 or even 1.5 seconds.

Flash Cards

I also like to put a spin on multiplication flash cards. With this twist, students match the cards with the same numerical answer. This activity encourages an understanding of multiplication as well as rehearsal of math facts. This is a great way to practice math facts while developing number sense. You can download a free set here.

Number Sense Strategy 3: Conceptual Instruction

To help our students continue to develop number sense and make sense of math, our math instruction must be conceptual, rather than a series of procedures. By far, the book with the biggest impact on my math lessons is Teaching Student-Centered Mathematics by John Van de Walle. The first time I read it, Van de Walle stepped all over my toes. I have many notes in the margins from the first time I read the book where I questioned everything I read. I’ve since reread the book several times, and acknowledge that I had a lot to learn when I first started teaching. I still do! This book challenges me to continually look at my math lessons and to find ways to improve those lessons.

When I started teaching, I often focued on algorithms, tricks, and shortcuts without first ensuring conceptual understanding. Algorithms and shortcuts are not necessarily bad. However, the key to using them correctly, lies in the sequence of events that occur in learning math concepts. Our math instruction must first ensure that students’ conceptual understanding is deeply embedded. When students have truly mastered a concept, and they should be able to show all the detailed steps in a process, explain why those steps occur. Once students reach this point, we can expose them to more efficient ways to express or perform those same processes. When we teach algorithms and shortcuts first, they become the means to get to the answer. When we bypass conceptual understanding, it is harder for students to understand more complex topics as they advance in school.

Over the years, I’ve modified my math instruction to allow students to construct their own understanding. For example, when using maipulatives, I no longer structure my lessons so that students are directed in exactly how to use the manipulatives.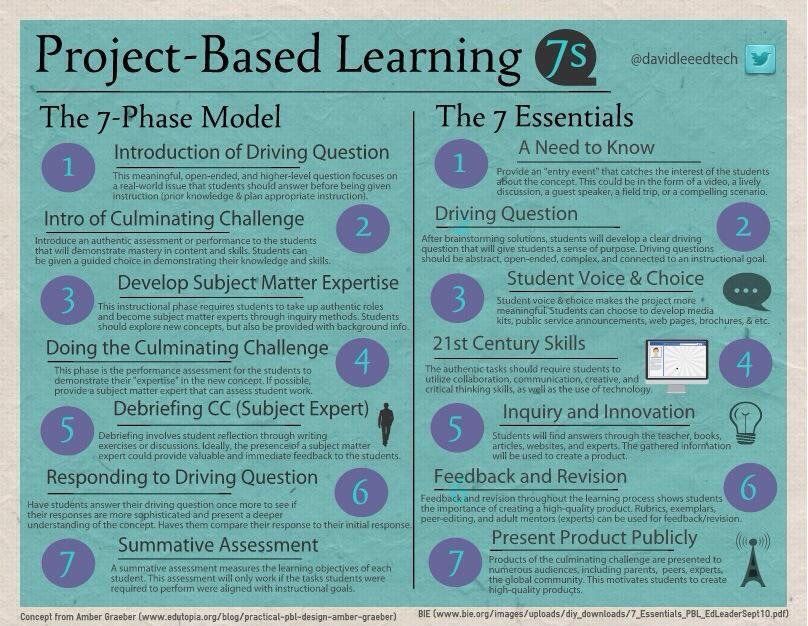 When I do this, my students are blindly following directions and look like they understand. But a rote procedure with a model is still a rote procedure. When getting answers rather than solving problems becomes the focus, students will gravitate to the easiest method available to get answers.

This does not help a student’s number sense, because the student is not reasoning with numbers. We must allow math to be problematic for students. A problem is a task where students have no prescribed or memorized rules or methods, nor is there a perception by students that there is a specific solution method. These problems focus students’ attention on ideas and sense making.

An example of a task that would be problematic for students is this divisibility task. In this assignment, students must add a digit to the ones place to create a number that is divisible by three, and students explain how they chose that number. Yes, I could simply have students memorize divisibility rules, but there is no application or problem in that lesson.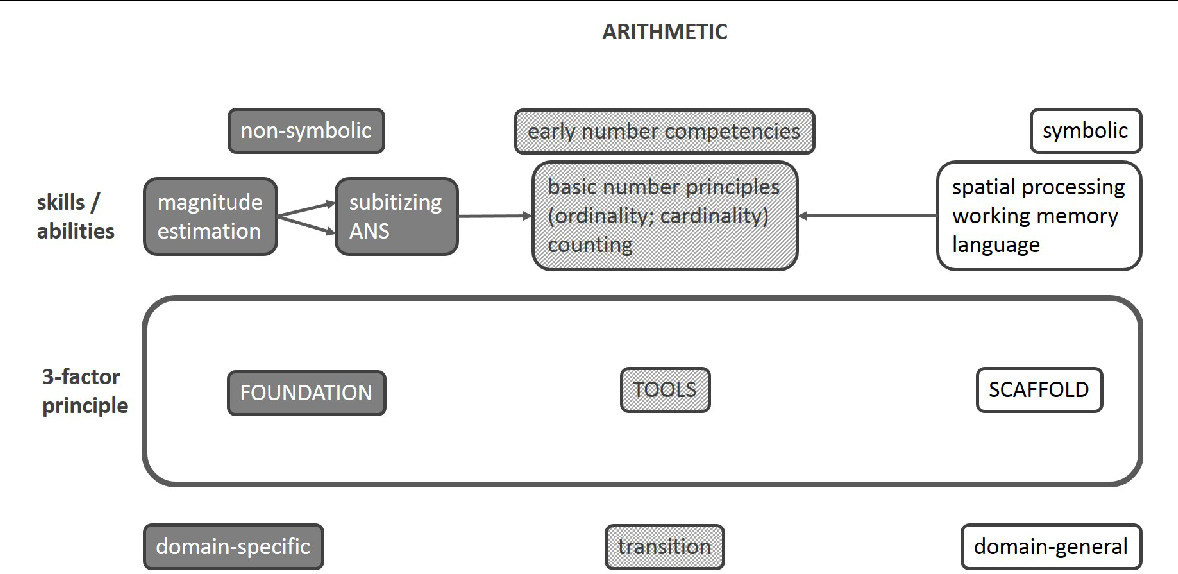 We could make a cute craft or sing songs, but without authentically understanding the rule, students will not be able to apply what they have learned. Of course, I’m not criticizing crafts and songs! They’re a tool, but they are not enough for conceptual learning. You can download the divisibility task here.

Number Sense Strategy 4: Communication

My students ask me all the time why they have to write so much in math! Students should be able to think through what methods make sense and discuss the use of different methods. Communication of math ideas help students solidify their understanding of mathematics. Math can be thought of as a language, and communication plays an important role in making mathematics meaningful.

I occasionally have students write about math through Math Journal Prompts. I had my prompts for the year bound into books for my students, and they regularly write in their journals. The prompts are not word problems or multi part problems. They’re unique in the sense that they’re opened ended and encourage metacognition and evaluation.

I also try to regularly incorporate writing in my math tasks. I have students explain how they solved the problem using multiple representations. Getting a good response is typically like pulling teeth at the beginning of the school year. But with extensive modeling and practice, students always come around.

Number Sense Strategy 5: Time

I was hesitant to add time to this post, because it’s something we don’t have a lot of control over as teachers. As I write this, I recognize that I’m preaching to the choir. I get it. I’m teaching full time, so I’m right there with you. However, it’s hard to mention the topics above without at least mentioning time. We all know that there isn’t enough time in the day to do all that we need to do, and there isn’t enough time in the school year to effectively teach all of our standards.

One thing we have to look at is our daily schedule. I’ve heard of classrooms with math blocks of 25 and 30 minutes. With that short of time frame, there is no way to adequately teach math standards and build number sense. Many of us have no control over the time allotted for each subject, but if possible, I recommend at least an hour a day for math. With our daily time crunch, it’s important to eliminate as much down time and transition time as possible.

Every year I feel I have to move so fast through math standards that my students don’t have time to truly understand and conceptualize the standards. Conceptual instruction takes longer than procedural instruction, so I’m not able to rapidly move through the standards. This leaves me in a panic after Christmas, because I have little time left to teach so many things. I’m learning to spend the bulk of my time on my essential standards or power standards. Those are the standards that it’s important for students to master for success in math in later years. For me, those are my place value, addition, subtraction, multiplication, division, fraction, and decimal standards. I absolutely teach my other standards, but I don’t go as deep as I would like because of time constraints.

In my experience, math is a subject that is often short changed. Whether it’s professional development, early intervention programs, RTI, or after school tutoring, there always seems to be less of an emphasis on math. I teach reading too, so I’m completely on board with supporting students as much as possible with reading, but I don’t think math should be neglected either.

I’m learning, not there yet, to not waste my energy complaining or worrying about what I can’t control. I’ll continue to advocate for high quality math instruction, but if I only focus on what’s wrong, I won’t make positive gains. I tutor one afternoon a week after school, because I know it’s a need for my students. A coworker and I have set aside time for biweekly professional development, because we’re not satisfied with where we are in our instruction. We want to continually improve. These are things I can do something about, and the rest…. well, maybe one day.

I’ve found that the best way to instill number sense is through my math warm-ups. This incorporates each of these strategies, using only about 10 minutes a day-at most! If you’d like to learn more about my warm-ups, be sure to check this out. I also have a post with more information on math warm-ups.

How number sense affects math learning

You may have heard the term number sense in the context of mathematics, but what does it really mean and how does it apply to children who are bad at math? Find out which skills affect number sense and how to help your child develop them.

What number sense really means

What people sometimes call number sense is actually a set of skills that allow you to work with numbers. This includes abilities:

• Understand quantity.

• Understand concepts such as "more" and "less" in the contexts of quantity and size.

• Understand the relationship between one object and a group of objects (for example, "seven" means one group of seven objects).

• Understand the symbols that represent quantities (for example, 7 means "seven").

• Compare numbers (for example, 12 is greater than 10 and 4 is half of 8). nine0003

• Understand the sequence of numbers in a list: 1st, 2nd, 3rd, etc.

Some people have a better sense of numbers than others. Children diagnosed with dyscalculia often lack these basic skills. This can interfere both in school and in everyday situations.

​

How Number Sense is Used to Learn Math

Math requires children to manipulate numbers in addition, subtraction, multiplication, and division, all of which require strong number sense skills. Children who are well developed in these skills can quickly compare groups of objects and determine which group is larger and which is smaller. They understand what it means when the number of objects in a group increases or decreases, and they understand how to assemble groups and break them down into smaller pieces. They also understand that characters (numbers) can be represented by objects. For example, when they see a bunch of seven beads, they understand that this number is 7.

​

Difficulties with calculations

If your child has poor number sense skills, they may have difficulty even with simple math equations. For example, it may not understand the meaning of adding or subtracting objects from a group.

​

Let's remember our bunch of seven beads. If you remove two beads, the child may not realize that the number of beads has decreased. He may not realize that by subtracting two beads, a group of seven became a group of five. nine0003

​

In the same way, if you add three beads to the pile, he may not realize that the number of beads has increased. And he may not realize that by adding three beads, a group of seven became a group of ten.

​

Due to poor number sense skills, the child may also not be given multiplication. He may not realize that it is easier to combine objects from several groups by multiplying them rather than adding them. Also, this weakness can affect its ability to divide. The child may not realize that division is the easiest way to break groups down into their component parts. nine0003

• Some people use the term "number sense" to describe a group of key skills in mathematics.

• Children who do not do well in math (especially children diagnosed with dyscalculia) often have poor development of these skills.

• Over time, number sense skills can be developed and strengthened.

Number sense is determined by environment, not genes – News – IQ: Research and Education Portal – Higher School of Economics National Research University

Number sense is an evolutionary ability inherent in humans and animals. It is equally developed in both urbanized societies and representatives of nationalities who do not know double-digit numbers. Recent studies confirm that individual differences in the accuracy of the number sense depend little on genetic factors. The report on this was presented by the Director of the International Laboratory of Goldsmiths College of the University of London and the Head of the Laboratory of Cognitive Research and Psychogenetics of Tomsk State University Yuliya Kovas

Number sense is a person's innate survival-related ability to determine an approximate measure or quantity by eye, ear and weight. This ability is not yet sufficiently studied by science. In particular, scientists are concerned about the extent to which the sense of number is related to heredity, and how it correlates with mathematical abilities. That is, for example, if a person is a mathematical genius, does this mean that he is superior to other people in the sense of number? nine0003

A special study in the UK was devoted to these topics. Project Manager Julia Kovas presented the results in the report "The Nature of Individual Differences in Number Sense and Mathematical Ability" as part of the HSE Cognitive Research Seminar.

The project analyzed tests performed by 16-year-old twins participating in the UK Twins Early Development Study (TwinsEarlyDevelopmentStudy, TEDS) . The study included data from 837 pairs of monozygotic and 1422 pairs of dizygotic twins. The results showed that both monozygotic and dizygotic couples are only moderately similar in number sense. In addition, the researchers concluded that there is no difference in the development of the level of number sense in men and women.

The mysterious ability to guess the number

In everyday life, a person does not think about the fact that he has a special ability to sense the number, because it is taken for granted. “We enter the airport building and can determine by eye how many people there are in the zone of visibility available to us. Everyone can guess how many people are in the audience right now,” Kovas noted during the presentation of her report. Picking up an object, a person is able to determine its approximate weight or catch by ear how many blows sounded in a certain period of time. nine0081 Animals also have the ability to sense a number. And this is understandable from an evolutionary point of view. Animals, like people in the early stages of their development, in order to survive, had to understand where there are more resources for food, and where the greatest danger comes from.

Studies show that babies have a sense of number. Scientists have been experimenting to see if babies can tell the difference between fewer and more dots on a screen. The results confirmed that babies as young as a few months old are able to distinguish between more and fewer dots when the difference is quite large (eg 1:2). The sense of number develops in a person with age and reaches a peak by the age of 30, remaining at this level almost all his life. nine0003

The level of education and literacy does not affect the development of a sense of number. According to Kovas, scientists have studied one of the tribes of the Amazon (Munduruku), whose representatives were isolated from civilization quite recently. Children from this tribe did not go to school. And the local language has words for just five numbers. To indicate a larger number, the representatives of the tribe use the word "many". But studies have shown that their sense of number is developed to the same extent as the average representative of Western countries. nine0003

A gift from nature, not from parents

Nevertheless, since the sense of number is associated with numbers and, accordingly, with mathematics, it is logical to assume that there may be a relationship between the level of development of the sense of number and mathematical abilities.

In the course of the study, the authors set the task of answering two questions. The first is why some people can quantify more accurately than others - what influences the development of a sense of number more - genetics or environment. The second relates directly to mathematics - how much the sense of number is connected with mathematical abilities. nine0003

Exploring this area, according to Kovas, was not easy enough. Firstly, genetics still largely remains an unexplored area, and secondly, in order to obtain convincing conclusions, it is important to have the results of studies of children of different ages.

However, during the work on the project it was possible to confirm that the influence of genes on the level of development of the sense of number is secondary. Monozygotic twins have 100% genetic similarity, dizygotic twins share 50% of variable genetic factors. However, both of them showed similar differences in the sense of number, which cannot be said about the ability for mathematics. nine0082 Mathematical ability of people is 60% determined by genetic factors, at least in many studied populations.