Triangle shaped objects in the classroom
Classroom Triangles - Activity - TeachEngineering
(1 Rating)Click here to rate
Quick Look
Grade Level: 8 (7-9)
Time Required: 45 minutes
Expendable Cost/Group: US $0.00
(minimal or no cost if using school-provided or borrowed compasses)
Group Size: 1
Activity Dependency: None
Subject Areas: Earth and Space, Geometry, Measurement
Share:
TE Newsletter
Summary
Students use bearing measurements to triangulate and determine objects' locations. Working in teams of two or three, they must put on their investigative hats as they take bearing measurements to specified landmarks in their classroom (or other rooms in the school) from a "mystery location.Engineering Connection
Engineers use triangulation technology for many applications. For example, triangulation calculations are used for shape reconstruction of 3D geographic objects such as aquifers, ocean currents and weather fronts. Triangulation calculations are used in medical devices to detect heart rhythm disorders. Triangulation is also used in manufacturing or prototyping processes that create solid objects of polymer materials from the information provided on computer drawings.
Learning Objectives
After this activity, students should be able to:
- Describe how to use a compass to do triangulation and determine their location,
- Identify the intersection of two points,
- Determine a landmark on a map
- Explain the relationship between triangulation technology and other fields of study (such as mathematics)
Educational Standards
Each TeachEngineering lesson or activity is correlated to one or more K-12 science,
technology, engineering or math (STEM) educational standards.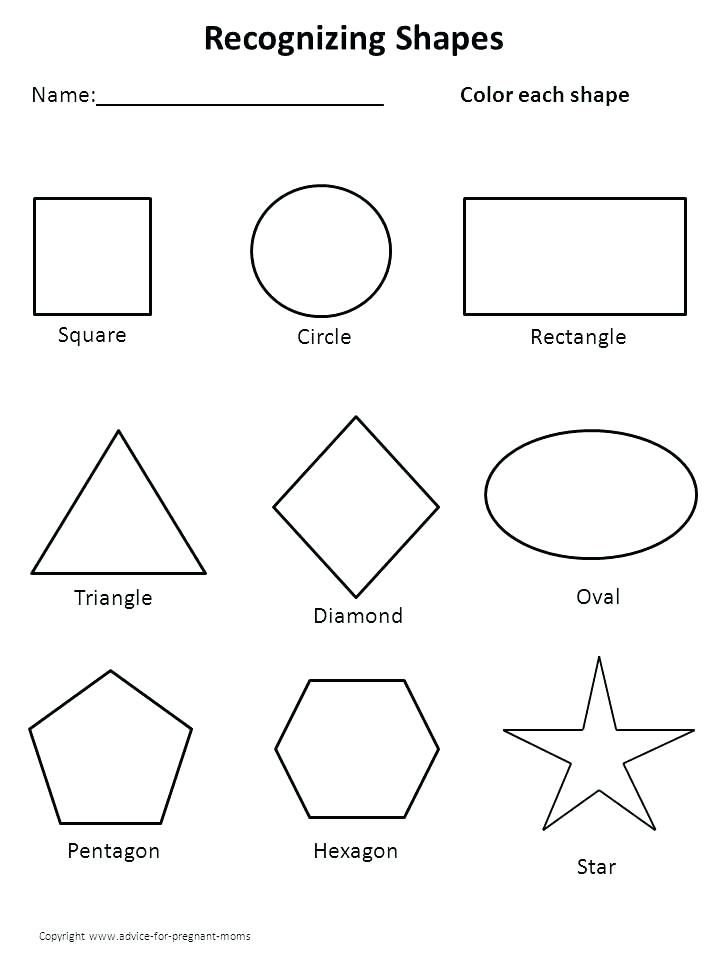
All 100,000+ K-12 STEM standards covered in TeachEngineering are collected, maintained and packaged by the Achievement Standards Network (ASN), a project of D2L (www.achievementstandards.org).
In the ASN, standards are hierarchically structured: first by source; e.g., by state; within source by type; e.g., science or mathematics; within type by subtype, then by grade, etc.
Common Core State Standards - Math
-
Solve problems involving scale drawings of geometric figures, including computing actual lengths and areas from a scale drawing and reproducing a scale drawing at a different scale.
(Grade
7)
More Details
View aligned curriculum
Do you agree with this alignment? Thanks for your feedback!
-
Draw (freehand, with ruler and protractor, and with technology) geometric shapes with given conditions.
Focus on constructing triangles from three measures of angles or sides, noticing when the conditions determine a unique triangle, more than one triangle, or no triangle.
(Grade
7)
More Details
View aligned curriculum
Do you agree with this alignment? Thanks for your feedback!
International Technology and Engineering Educators Association - Technology
State Standards
Suggest an alignment not listed aboveWhat alternate alignment do you suggest for this content?
Subscribe
Get the inside scoop on all things TeachEngineering such as new site features, curriculum updates, video releases, and more by signing up for our newsletter!
PS: We do not share personal information or emails with anyone.
Materials List
Each student needs:
- 1 copy of the Classroom Triangles Worksheet
- pencil
- graph paper
- ruler
- compass (students may share if necessary)
- tape measure (students may share if necessary)
Worksheets and Attachments
Classroom Triangles Worksheet (pdf)
Visit [www.teachengineering.org/activities/view/cub_navigation_lesson06_activity1] to print or download.More Curriculum Like This
Middle School Lesson
Getting to the Point
Students learn how to determine location by triangulation. After the process of triangulation is described, students practice finding their locations on a worksheet, in the classroom and outdoors.
Getting to the Point
Middle School Lesson
Topo Map Mania!
Students learn to identify the common features of a map. Through the associated activities, students learn how to use a compass to find bearing to an object on a map and in the classroom.
Topo Map Mania!
Middle School Activity
Topo Triangulation
In this activity, students learn how to read a topographical map and how to triangulate with just a map. Students practice converting a compass measurement to a protractor measurement, as well as reverse a bearing direction (i.
e., if they know a tree's bearing is 100 degrees from you, they can deter...
Topo Triangulation
Middle School Lesson
GIS, Mathematics and Engineering Integration
Students explore using a GPS device and basic GIS skills. They gain an understanding of the concepts of latitude and longitude, the geocaching phenomenon, and how location and direction features work while sending and receiving data to a GIS such as Google Earth.
GIS, Mathematics and Engineering Integration
Introduction/Motivation
You have a problem — you think. You know where "something" is, but you do not know where YOU are.
So, how can you even begin to determine your location if you do not have a clue as to your whereabouts? You can use triangulation to determine your location. Triangulation is based on finding an unknown location using angle measurements to two known locations. Mathematically, the two known positions define the two vertices (and length of the one side) of a triangle, and the two bearing measurements define two of the angles of the triangle. To get a clearer picture of this process, assume you know where your home is, you know where the movie theatre is, but you do not know how to describe where your school is located. Luckily, you have a compass and a map. You can take a bearing measurement from the school to the cross streets nearest your home and the movie theatre, triangulate the two and determine the location of your school. If you can draw a straight line, then finding your school's location is as simple as 1,2,3!
If you are not lost, for what other reasons would you need to know about and how to take bearing measurements for the purpose of triangulation? Mountaineers, for example, need to know this information so that they may safely hike in the mountains and not get lost or put themselves in danger.
Engineers use bearings to determine where to build roads, lay underground wires and pipes and construct bridges and dams. This activity teaches you how to take a bearing measure and apply triangulation to determine locations in the classroom. Afterwards, you should be able to use a map and a compass to determine many unknown locations.
Procedure
Before the Activity
- Take 3-4 bearing measurements to objects in the classroom from one location (important that you do this before your students begin this activity). Take three bearing measurements of each object from one "mystery" location that the students will try to figure out.
- Create a classroom map. On a piece of graph paper create a scale that maps one square of the paper to a reasonable length in the classroom. You can use a tape measure, or if there are square tiles on the floor, you can count tiles and fractions of a tile.
- Determine the direction to magnetic north in your classroom. Pick the wall that is most directly to the north as "classroom north." You should orient your classroom map so that the walls closest to north-south are on the left and right sides, and the closest to east-west walls are on the top and bottom. Draw a pseudo magnetic declination indication on your class map that shows which way magnetic north points with respect to your classroom north.
- Draw the outline of the room on the paper, and mark several fixed landmarks that are easy to sight. You can use things like the edge of a desk, the doorknob, a specific shelf of a bookshelf or the edge of the chalkboard. Alternatively you could hang up "markers" on the walls as your landmarks. Make sure that the landmarks are correctly placed on the classroom map, and make two or more copies for each team.
- Practice taking a bearing measurement from one location in the classroom to each of the landmarks, making the "declination" adjustment and figuring out where you are.
Be sure to keep at least one "clean" copy of the classroom map, as you will be giving each student a copy. It is advised to make a teacher's copy which shows the bearings.
With the Students
- Hand out a copy of the classroom map that you created.
- Illustrate on the chalk/white board how to draw a bearing line on the map through the landmark and how to find the intersection of two bearing lines. Identify the x, y (horizontal and vertical) coordinates of the intersection, and show them the location in the classroom from where the measurements were taken. Have the students explain in their own words how to take a bearing.
- Give the class the bearing measurements that you made from an interesting point in the classroom to three landmarks. Have the students work in teams of two or three to figure out the location using triangulation.
- Hand out the Classroom Triangles Worksheet to each student.
- Now have them use a compass to take their own bearing measurements to classroom landmarks, and mark their location on the worksheet.
They can check their answers using a tape measure or by counting floor tiles. - Discuss the results of the activity. Ask students what they learned. Did they have trouble finding two or three bearings? Ask the students how they decided which landmarks to use. How did this affect their results? (Answer: If the landmarks are almost along the same line, you do not get very reliable results. If the landmarks are at close to 90 degrees apart, you get the best results.) If they sited to three landmarks, how big was their triangle that bounded their position?
Assessment
Pre-Activity Assessment
Activity Review: With students, review activity topic and discuss findings as a class.
- Review how to take a bearing. Pass out compasses to each student (or pair of students) and go over the steps of how to take a bearing, using the front of the classroom or the teacher's desk.
- Have students volunteer to explain how to take a bearing in their own words.
Activity Embedded Assessment
Worksheet: Have the students complete the activity worksheet; review their answers to gauge their mastery of the subject.
Post-Activity Assessment
Pairs Check: After students finish working individually on worksheets, have them compare answers with a peer, giving all students time to finish the worksheet.
- Have them check for location accuracy using their compass and the known bearings.
Discussion/Questions: Ask the students and discuss as a class:
- What did you learn?
- Did you have trouble finding a location using two or three bearings?
- How did you decide which landmarks to use? How did this affect your results? (Answer: If the landmarks are almost along the same line, you do not get very reliable results. If the landmarks are at close to 90 degrees apart, you get the best results.)
- If you sited to three landmarks, how big was your triangle that bounded the position?
Hunt for Red October: Tell students about the movie "The Hunt for Red October.
" In this movie the submarine loses power to its navigation equipment. Using a map, one crew member gives the captain some bearings and distances to get them through a dangerous ravine. The crew member has to get his bearings just right or the submarine will crash.
- For this activity, have the students create their own dangerous ravine trail map and write instructions for how someone could navigate through it. For example, if using graph paper, 1 block could equal 1 mile. The student's directions might read "First, go N40 for 6 blocks" and so on. Have the students exchange maps and see if they can follow each other's directions and make it through the ravine.
Troubleshooting Tips
When you draw the classroom map, make sure to leave space between the walls and the edge of the paper. If the classroom is very skewed relative to north, it might be very confusing to take accurate bearing measurements.
Also when drawing the classroom map, select objects that are roughly at waist to chest level so that the students can easily take accurate bearing measurements.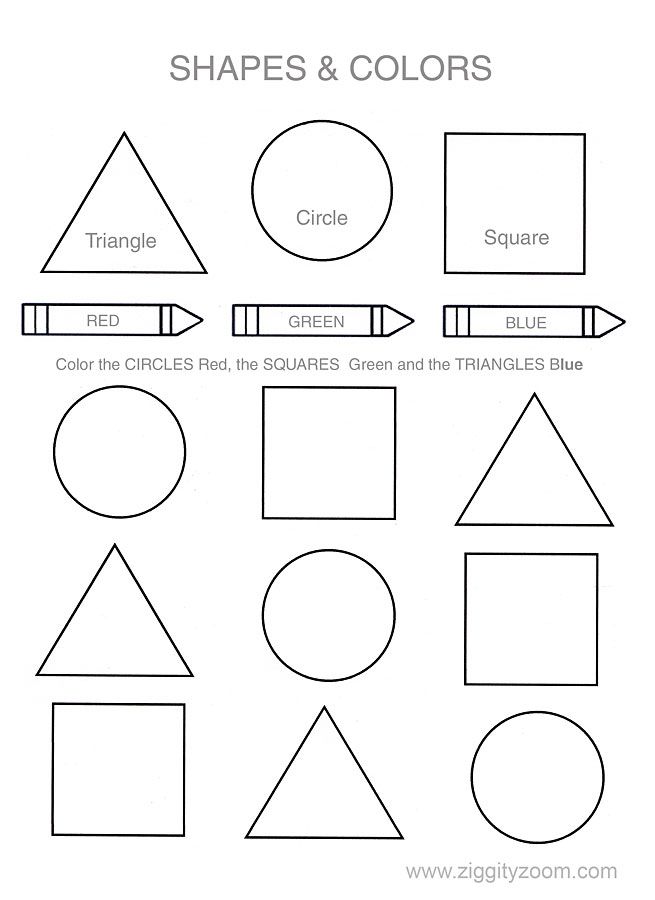
Use things like the edge of a desk, a specific shelf of bookshelf, the doorknob, or the edge of the chalkboard.
Activity Extensions
Working in teams of two, have students create a classroom map. On a piece of graph paper, have them create a scale that maps one square of the paper to a reasonable length in the classroom. They can use a tape measure, or if there are square tiles on the floor, have them count tiles and fractions of a tile. Have students indicate north on their maps and the location of different classroom objects and take the bearing measurement from specific locations. Students should compare maps with other teams and discuss their observations/measurements as a class.
Activity Scaling
- For lower grades, have students work in groups to complete the worksheet.
- For upper grades, have students work alone to complete the worksheet.
Copyright
© 2004 by Regents of the University of Colorado.Contributors
Matt Lippis; Penny Axelrad; Janet Yowell; Malinda Schaefer ZarskeSupporting Program
Institute of Navigation and Integrated Teaching and Learning Program, College of Engineering, University of Colorado BoulderAcknowledgements
The contents of this digital library curriculum were developed under a grant from the Satellite Division of the Institute of Navigation (www.ion.org) and National Science Foundation GK-12 grant no. 0338326.
Last modified: August 10, 2017
List the names of the things of triangle shaped objects.
Answer
Verified
123.
6k+ views
Hint: In order to list the things of triangle shaped objects, first we will understand what a triangle means and what its properties are. Also, we will see what it looks like. Then to solve this problem, we will basically look around ourselves and sort the objects that satisfy the conditions to be a triangle. And hence we will get the required result.
Complete answer:
Now first let’s understand what a triangle is and how it looks.
A triangle is a closed shaped object which is made up of three sides and also consists of three edges and three vertices.
Basically, there are three types of triangles:
1) Scalene triangle: A triangle in which all sides are of different length.
2) Isosceles triangle: A triangle in which two sides are of equal length.
3) Equilateral triangle: A triangle in which all sides are of equal length.
Now, just take a look at the below figures:
These all represent triangles with different sizes.
Now let’s look around and list all the things which are triangular shaped.
There are certain things which are triangular shaped objects like some of them are:
1) sandwich
2) a slice of Pizza
3) Triangular Ruler
4) Birthday banners
5) Bermuda Triangle
6) Traffic signs
7) Nachos chips
Note:
To solve this type of question, the key is to remember the definition of triangle. Students should also know what a triangle looks like. Also note that there are other things also which are in the triangular shape, so it is not compulsory to write the same things which are mentioned above. You can also write on your own.
Recently Updated Pages
Calculate the entropy change involved in the conversion class 11 chemistry JEE_Main
The law formulated by Dr Nernst is A First law of thermodynamics class 11 chemistry JEE_Main
For the reaction at rm0rm0rmC and normal pressure A class 11 chemistry JEE_Main
An engine operating between rm15rm0rm0rmCand rm2rm5rm0rmC class 11 chemistry JEE_Main
For the reaction rm2Clg to rmCrmlrm2rmg the signs of class 11 chemistry JEE_Main
The enthalpy change for the transition of liquid water class 11 chemistry JEE_Main
Calculate the entropy change involved in the conversion class 11 chemistry JEE_Main
The law formulated by Dr Nernst is A First law of thermodynamics class 11 chemistry JEE_Main
For the reaction at rm0rm0rmC and normal pressure A class 11 chemistry JEE_Main
An engine operating between rm15rm0rm0rmCand rm2rm5rm0rmC class 11 chemistry JEE_Main
For the reaction rm2Clg to rmCrmlrm2rmg the signs of class 11 chemistry JEE_Main
The enthalpy change for the transition of liquid water class 11 chemistry JEE_Main
Trending doubts
| Preparation for active educational and cognitive activity . 5 minutes. | |
| Task: actualization of knowledge and skills, providing motivation, acceptance of the goal, readiness for active educational and cognitive activity. | |
| Teacher activities | Student activities |
| Greet students, check classroom and equipment ready; emotionally sets up for learning activities Math, friends Presentation | Teachers are listening. Demonstrate readiness for the lesson, organize the workplace. |
| - And today we will devote our lesson to one of the sections of mathematics - Geometry. | Listen and tune in to work. Answer questions. |
| Acquisition of new knowledge and ways of doing things | |
| Task: provision of perception, comprehension and primary memorization of knowledge and methods of action, connections and relationships in the object of study, organization of active independent actions of students. | |
| Teacher activities | Student activities |
| Organizes a reminder on the topic "Triangles". Worksheet Task 1 ( in Worksheet) - Look at the shapes, find the triangles and color them. Check. | Perform individually task 1 in the Worksheet. |
| How many figures have been painted over? | Answer the teacher's questions. |
| Task 2 (in Worksheet) Each student receives a set of triangles. (see appendix 1) - Consider triangles. Think about how many groups and on what basis can triangles be distributed? - Divide by side lengths and stick on Worksheet. | Consider a set of triangles. |
| Organize work in pairs. - Discuss and write the name of each group. | Divide into groups, choosing a base. Explain the choice of base. Glued in three groups according to the lengths of the sides. Discuss in pairs what the triangles of each group have in common, generalize, give a name to the group. |
| Check . Organizes discussion, asks clarifying questions. | Joint check. |
| Organizes independent work Task 3 | Consider triangles, perform tasks. 1 option |
| Verification - mutual verification according to the sample on the slide | Pair check. Check the sample. |
| I organize work on solving problems on new material. (Slide 5) Task 4 Verification | You can use a document camera. |
| What type of triangle is not named? | Answer: isosceles. |
| Organizes front work. Task (Slide 6) . The perimeter of an isosceles triangle is 24 cm, the sum of the lengths of two identical sides is equal to the length of the third side. Find the lengths of the sides of the triangle. | Read the task. |
| Performs task analysis. - Read the problem. - What figure is given? - What else is known in the problem? | Answer the teacher's questions. Answer: an isosceles triangle. Answer: data on the sides - two sides are the same in length, and the length of the third is the sum of two identical lengths. |
| Offers to make a short note in the form of a diagram that will help solve the problem.
| Draw a diagram. |
| Offers to solve the problem independently. | Solve the problem on their own. |
| Check (Slide 7) 1 variant 2 variant | Explain the solution. |
| Organizes work with data of different types of triangles. | The task is performed in pairs. |
| Task 5 (see Worksheet)
- Color in blue the plate with the lengths of the sides of an equilateral triangle. | Discuss progress, paint over. |
| Verification | Frontal check. One tells, the rest control with a signal card. |
| Task 6 (see Worksheet) | Read the assignment and do it yourself. One student shows work through a document camera. The rest are controlled by a signal card. |
| Initial learning test | |
| Task: establishing the correctness and awareness of the assimilation of new knowledge at the reproductive level, identifying gaps, misconceptions, typical mistakes and correcting them. | |
| Teacher activities | Student activities |
Organizes the initial check. Marks are set only "5" and "4". Annex 2 | Do it yourself |
| Verification frontal | Check |
| Total on a reflexive basis . min. | |
| Task: to provide an analysis, an assessment of each student's own activities, taking into account the goals set at the beginning of the lesson, students to receive information about real educational results, determine the personal comfort of students in the lesson, express the degree of satisfaction with individual and joint educational activities and motivation for its further continuation. | |
| Teacher activities | Student activities |
| Offers to analyze whether the objectives of lesson have been achieved. (Slide 8) | Choose a phrase, add your comment. |
3rd class 
What is the name of such a triangle? Find its perimeter. 
These are equal sides, and we find the length of the third side by adding two lengths. 
Draw a segment in it so that you get equilateral and scalene triangles. 
The educational project “Triangles in our life”
GOU RK “C (K) S (K) SH No. 45” Ukhta
Training project
in mathematics
9000 9000 work performed 9000 9000
5b grade student
Zhakheev Gavril
Manager
Polyakova T.
A.
Ukhta
2016
Introduction
From childhood we are familiar with such figures as triangle, circle, square. And it would seem that there is nothing surprising in them. But in fact, this is a misconception.
In the 5th grade at the lessons of geometry, we got acquainted in detail with a geometric figure - a triangle. It turned out that we did not know much.
In order to learn more about this figure, we decided to do a little research. Our parents and school teachers helped us in this.
SLIDE 2
We have set ourselves questions that we would like to receive answers to:
1) what triangular objects surround us
2) what properties of a triangle are used in everyday life
3) what riddles are associated with triangles
The most important question - can a triangle be called an important figure?
We asked the teachers and students of the school to answer it.
SLIDE 3
11 students and 8 teachers were interviewed
Questioner: Do you consider the triangle an important figure?
As you can see, the majority of respondents do not consider the triangle an important figure. We will try to prove otherwise.
Question 1. What is a triangle?
SLIDE 4
The concept of "triangle" has different meanings:
- in mathematics it is a geometric figure bounded by three mutually intersecting straight lines forming three internal angles
- in drawing - a ruler in the form of such a figure, serving for drafting
- in music - a percussion musical instrument made of a steel bar, which is struck with a metal stick
- in everyday life - a type of relationship between three people
In our work we take only the first meaning - mathematical.
Question 2. How did triangles come about?
SLIDE 5
It is not known exactly how the triangles appeared. But geometry was discovered by the Egyptians, and arose when measuring the Earth. The floods of the Nile River washed away the boundaries of the plots, and people had to re-mark the fertile sections of its banks, from which the water had gone. The simplest was a plot in the shape of a triangle.
Question 3.
Where do triangles occur in our lives?
SLIDE 6
In our life, the triangle is found everywhere, you just have to look closely. Here are the answers of teachers and students to our second question: where does the triangle meet in your life?
Roof of the house bicycle spruce
On clothing sails road signs
House Lessons Baking
There are other triangular items.
Front triangle
SLIDE 7
White flocks of letters flew to Rus'.
They read them with excitement, knew them by heart.
These letters are still not lost, they are not burned.
How they protect their sons as a great shrine.
These lines are dedicated to the front-line letter.
Since there were not enough envelopes during the Great Patriotic War, soldiers wrote letters on a plain sheet of paper folded into a triangle.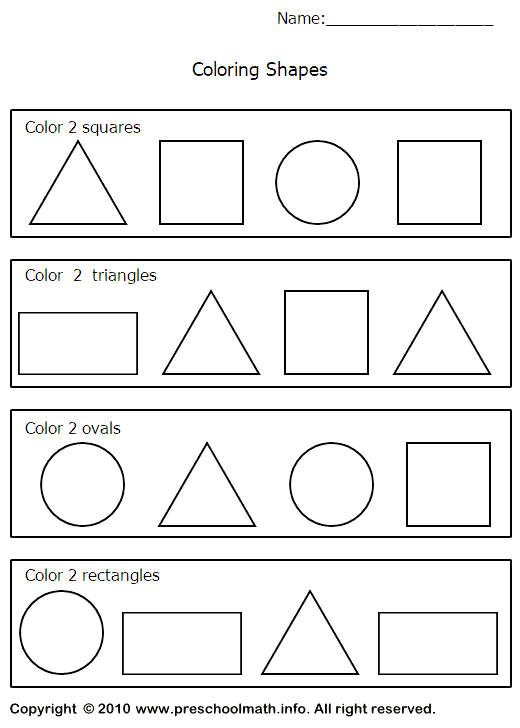
Send these emails for free.
Relatives were looking forward to triangular news from the front. The letter about the death of a soldier came in an envelope.
In memory of the heroic warriors - defenders in the Battle of Stalingrad, a memorial complex "Soldier's Field" was built near the road Volgograd - Moscow. An urn with the ashes of fallen soldiers was buried in a mass grave. Nearby is a sculpture of a girl with a flower in her hand. Nearby is a marble triangle of a soldier's letter.
On this memorable triangle is a letter from Major D. Petrakov to his daughter, written on September 18, 1942 after the battle: "My black-eyed Mila! I am sending you a cornflower. Imagine: there is a battle, enemy shells are exploding around, craters are all around and here a flower grows. And suddenly another explosion - the cornflower is plucked. I picked it up and put it in the pocket of my tunic.
The flower grew, reached for the sun, but it was torn off by an explosive wave, and if I had not picked it up, they would have trampled it. This is how the Nazis act in the occupied settlements, where they kill children. Mila! Papa Dima will fight the fascists to the last breath, so that the fascists do not treat you the same way as with this flower..."
Road signs
SLIDE 8
Many of us would like to become drivers in the future.
In this case, we will definitely need to know the road signs. There is a group of road signs in the shape of a triangle. What do they mean?
A triangle-shaped road sign on a white background with a red border - something warns about. They are installed in a city or village for 50-100 m, outside the village for 150 - 300 meters to some site.
Based on these forms, you can make various crafts:
Modular origami is especially beautiful.
These products have their own peculiarity - they are assembled from a large number of small origami blocks.
Parts are placed in each other, which is well fixed and the product does not fall apart.
Question 4. What riddles are associated with triangles?
SLIDE 10
The Bermuda Triangle is an amazing area of the western Atlantic Ocean. This place is also called the anomalous zone. Place of the mysterious disappearance of ships and planes.
At the lesson of natural history, the teacher Troshina N.M. she told us about this phenomenon and showed us on the map where this mysterious place is located.
This region is not easy - there are many shoals, a huge number of fast water and air currents, cyclones often arise and hurricanes rage.
Another reason for the huge number of accidents, experts call the ability of the ocean to produce infrasound signals that cause panic among the crew, because of which people can even throw themselves overboard.
Despite the hype raised around the Bermuda Triangle, scientists say that in reality this area is no different, and a large number of accidents are mainly due to difficult environmental conditions.
Question 5. Why is the triangle used in construction?
SLIDE 11
In the process of studying the topic, we learned that a triangle has such a property as rigidity. Consider models of two figures - a triangle and a quadrilateral.
Under the action of a small force, the quadrilateral changed its shape, but the triangle did not. This is because the triangle is a rigid figure.
This property of the triangle is used in construction.
| The rafters of the buildings are triangular. This gives them strength and stability | When making a garden gate, be sure to nail the bar to get a triangle. This gives the gate strength, otherwise it will warp |
| During the construction of any bridges, lifting cranes in their designs, triangles |
Conclusions
In the course of our research, we came to the conclusion that the triangle is indeed an interesting and important figure.
