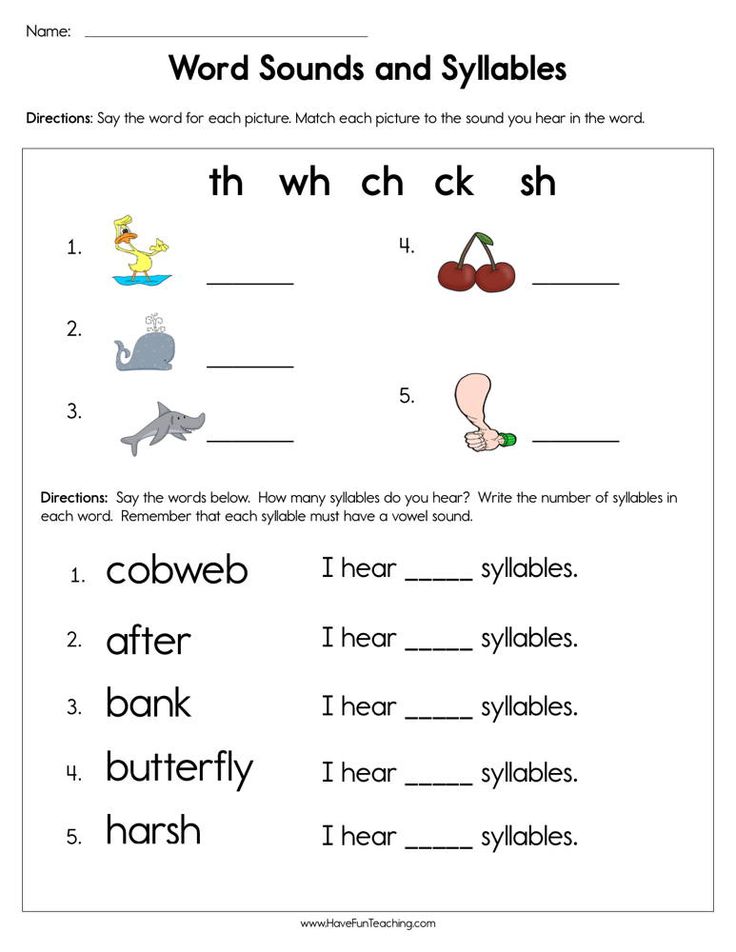
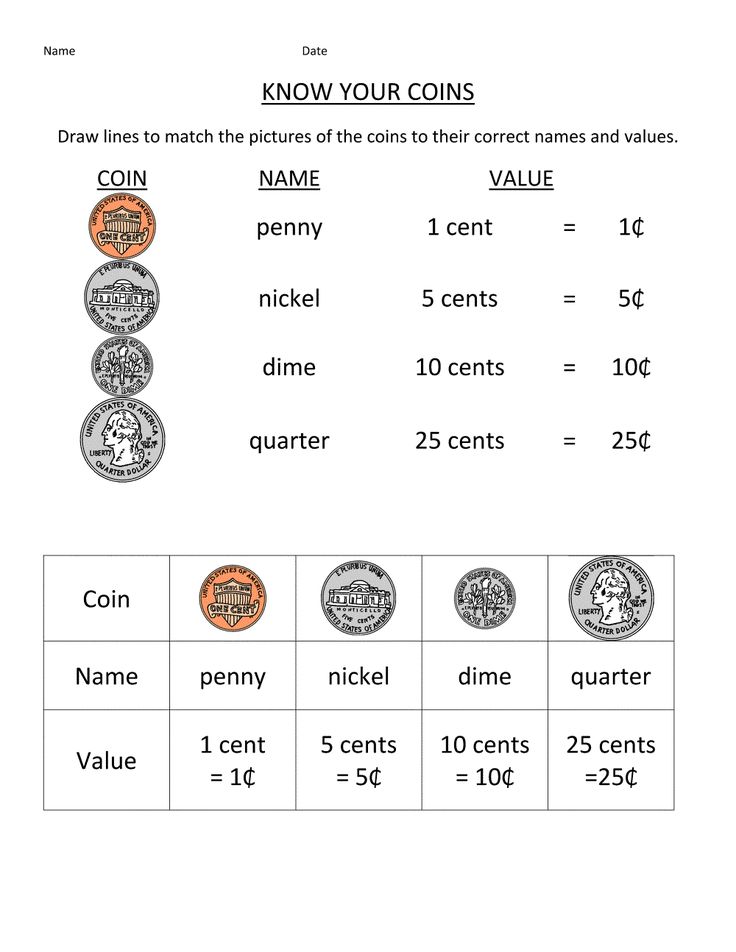
1St grade math concepts
1st Grade Math Skills, What Your Child Will Learn, Komodo Math
- Math Tips
- Education
- 1st
Your child is heading to first grade! After the year in kindergarten, your first grader will be ready for some amazing growth. For many children, first grade is the year that they bloom as readers and mathematicians. Get ready to support your child’s mathematical growth by learning about first grade math skills.
In first grade, you can expect your child to learn about:
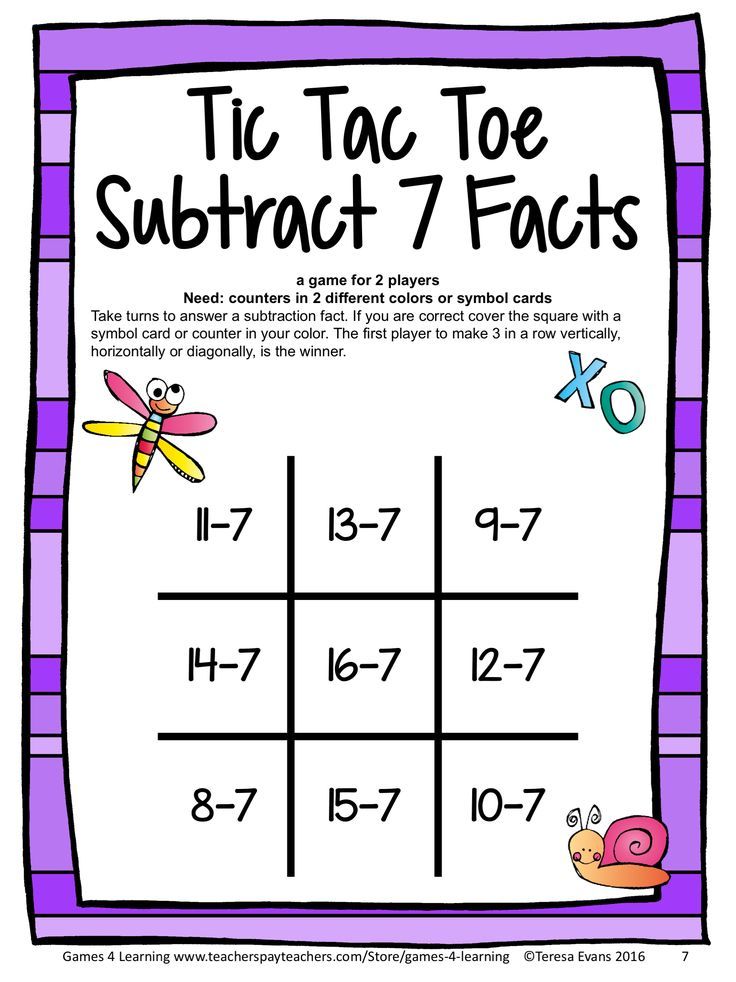
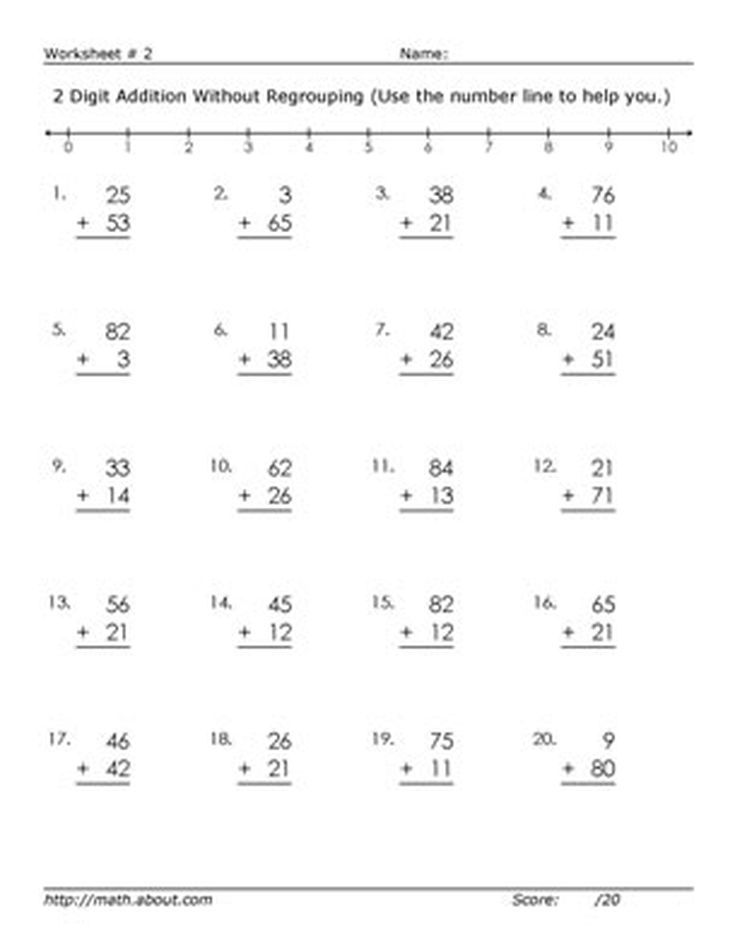
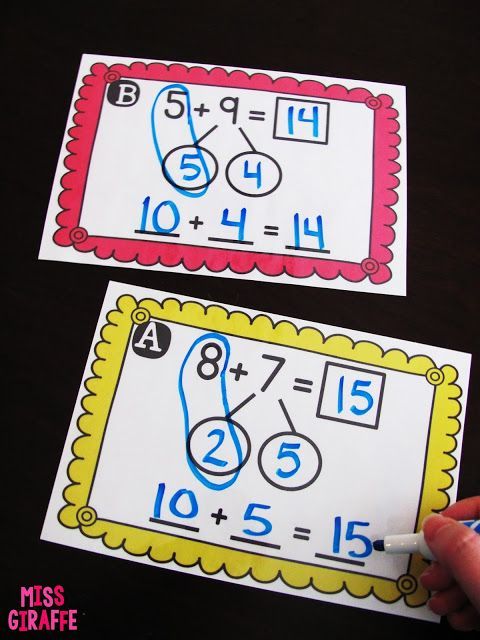
1. Addition and subtraction facts to 20
Now that your child has mastered the idea of adding and subtracting, they’re ready to practice math facts. This means getting faster when answering addition and subtraction problems to 20.
Help your child develop fluency by asking basic addition and subtraction problems - we find that using treats can help keep kids interested! If your first grader needs support, encourage the use of physical objects or fingers as problem-solving tools.
2. Addition and subtraction as inverse operations
Your child probably understands the concept of addition as “putting together” and subtraction as “taking apart.” In first grade, children are encouraged to see the connections between addition and subtraction. Your child will learn how addition and subtraction are inverse operations, or that one is the opposite of the other, and create “fact families” of related addition and subtraction problems.
When working with addition and subtraction, ask your child to see connections. For example, if your child has four dolls and three cars, ask how many toys there are in all. Then ask how many toys there would be if the four dolls are taken away.
3. Count and write within 120
Your child has probably mastered counting to 20. But in first grade kids will learn to count all the way up to 120! That’s not all. Kids will be expected to not only count, but write, the numbers. This is great practice for understanding multi-digit numbers.
This is great practice for understanding multi-digit numbers.
At home: Encourage your child to write numbers whenever possible. Talk about how two-digit numbers are made up of tens and ones and how three-digit numbers are made up of hundreds, tens, and ones. Just looking closely at multi-digit numbers together can be a great learning opportunity.
4. Add within 100
Now that your child has an understanding of numbers past 100 as well as basic addition and subtraction facts, it’s time to practice adding within 100. Children will practice adding one-digit numbers to two-digit numbers using strategies like counting on and number charts. Children can practice adding larger numbers with the help of a 1-100 chart.
First graders are also ready to practice adding and subtracting 10s to and from two digit numbers.
At home: Help your child see patterns when adding and subtracting 10s. For example, after solving a problem like 59 - 10 = 49, point out to your child that 49 has one less 10 than 59. This is another great way to learn about place value.
This is another great way to learn about place value.
5. Measure objects
In first grade, kids learn how to measure using rulers and more unusual things like paper clips. After taking measurements, children compare and order objects by length.
At home: Kids love measuring things around the house, so keep a couple of rulers handy. Pay attention to how your child is using a ruler and taking measurements. Sometimes kids don’t quite measure from end to end, so they might need a bit of help...
6. Tell time to hour and half hour
One of the trickiest concepts first graders will learn is to tell time. Using analog clocks is confusing, especially when kids are more used to seeing digital clocks. In first grade, your child will learn about the big and little hands of a clock and will practice telling time to the hour and half hour.
At home: Get hold of an analog clock for your home (either a real one or one made just for learning). Talk with your child about the time and how the hands move around the clock. Remember to just focus on telling time to the hour and half hour to start!
7. Understand basic fractions
First graders also get an introduction to fractions as equal shares. They will learn how to divide into equal groups and learn basic fractions like ½, ⅓, and ¼. First graders usually have a good understanding of fairness, so practicing making equal shares should be a relatively easy task for them!
At home: Help your child to divide pizzas, pies, and sandwiches into equal shares. As you do, talk about the fractions of the whole that you created.
First graders are ready to dive deep into mathematical concepts. Find time to connect with your child about classroom learning and get ready to have some fun!
Found this useful? Check out our grade by grade math guides from Kindergarten to 5th grade
Written by Lily Jones, Lily loves all things learning. She has been a kindergarten & first grade teacher, instructional coach, curriculum developer, and teacher trainer. She loves to look at the world with curiosity and inspire people of all ages to love learning. She lives in California with her husband, two kids, and a little dog.
She has been a kindergarten & first grade teacher, instructional coach, curriculum developer, and teacher trainer. She loves to look at the world with curiosity and inspire people of all ages to love learning. She lives in California with her husband, two kids, and a little dog.
About Komodo – Komodo is a fun and effective way to boost K-5 math skills. Designed for 5 to 11-year-olds to use in the home, Komodo uses a little and often approach to learning math (15 minutes, three to five times per week) that fits into the busy family routine. Komodo helps users develop fluency and confidence in math – without keeping them at the screen for long.
Find out more about Komodo and how it helps thousands of children each year do better at maths – you can even try Komodo for free.
Back to School - 5 Tips to Help you Ease Back into the Routine
Here are some steps you can take to ease children back from full vacation mode so that the first week of school doesn't knock you sideways.
Mindset - The Path to Mastery
People who have a growth mindset believe that they always have the potential to learn and improve. They are more motivated to persevere with difficult tasks, to take risks and to learn from failure.
The Most Important Math Concepts Kids Learn In 1st Grade
Your child has progressed from kindergarten to first grade. That’s exciting news! There is so much learning to come their way, especially from their first grade math class.
Math skills and concepts build on each other from grade to grade, which is why children need to get a firm foundation so they can handle the more complex challenges as they progress in school.
As a concerned parent, you might be wondering what some of these mathematical concepts will be and, more importantly, how you can help your child master them. You don’t have to figure it out on your own.
Here, we will give you a breakdown of what to expect from your child’s math class. We’ll also add a few tips on how to help your young learner thrive through it all.
We’ll also add a few tips on how to help your young learner thrive through it all.
Let’s get started!
Why Is Math Important?
Math is taught in the classroom, but that doesn’t mean that’s the only place it’s relevant. We use it every day!
From the hexagonal bee combs to the circles, semi-circles, and crescents of the phases of our moon, mathematics is an essential part of the world we live in, and learning it helps us make sense of everything around us.
Did you know that math skills can also be linked to music? Children who play musical instruments use the same part of the brain when doing math. This is why studies have shown that music students do better in mathematics than their non-musical peers.
Sports and mathematics also have an interesting connection. Just think about all the coordination involved in performing well in certain sports. Research has shown that these skills can also be used to learn math.
In addition, mathematics helps us be stronger logical thinkers. Since most young kids tend to enjoy math time, it’s essential to foster this natural love for the subject just as much as we want to encourage children’s love for reading.
Since most young kids tend to enjoy math time, it’s essential to foster this natural love for the subject just as much as we want to encourage children’s love for reading.
Helping children develop a love for mathematics generally works well when approached actively as a problem-solving skill rather than a rote memory task. Math helps children thrive in various aspects of their lives.
So, how do we get there? It all starts with the foundation.
Below are the key first grade math concepts your child will soon learn and some tips on how you can support them on their journey.
8 Important First Grade Math Concepts
1) Numbers And Counting
At first grade level (and for the next few years in school), learning different numbers and counting will form a significant part of your child’s mathematics lessons.
By the end of the first grade, your child will have learned to:
- Count and write numbers from 1 to 100
- Count by 1s, 2s, 5s, and 10s
- Count backward
- Count onward from any number
- Count backward from any number
There are different ways to help your child grasp numbers and counting at home, and hands-on activities work best.
An effective strategy is to help your child visualize what all these numbers mean. For example, instead of just memorizing the numbers, they can count bears, large dried beans, or even craft sticks.
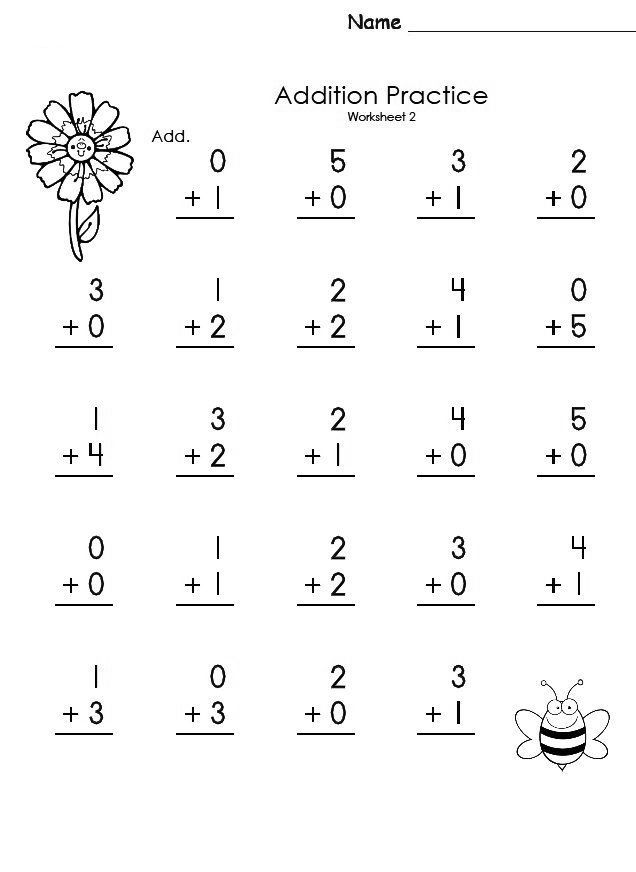
2) Addition And Subtraction
In first grade math, your young learner will start adding and subtracting numbers up to 30. They will also solve basic word problems with the help of drawings, objects, and equations.
By the end of the first grade, your child will have been shown how to:
- Add three one-digit numbers
- Write and show an understanding of the mathematical symbols (+, -, =)
- Solve problems involving one and two-digit numbers
- Solve problems involving an unknown. For example, 1 + _ = 4
Addition and subtraction are two math skills that can be demonstrated in everyday life situations. This makes it relatively easy to practice at home!
For instance, you might ask, “If you have two teddy bears and granny buys you three more, how many teddy bears will you have in total?” Or, “There were six strawberries in the fridge. Daddy ate some strawberries. There are now four left. How many did daddy eat?”
Daddy ate some strawberries. There are now four left. How many did daddy eat?”
3) 2-D Shapes
During pre-k, children get introduced to different shapes. In first grade, they will continue to extend their understanding of them.
By the end of the first grade, your child may be able to:
- Examine the attributes of different shapes (number of sides, faces, etc.)
- Name the 2-D shapes
To help your child grasp these shapes at home, continue to point out and name the 2-D shapes in the world around you (circles, triangles, pentagons, etc.).
When doing so, remember to always highlight the attributes (e.g., this book has four equal sides, so it’s a square).
4) Sorting And Patterns
Understanding and sorting patterns also forms a part of first grade math.
Your first grader will learn to:
- Sort different objects by attributes such as color, shape, and function. For example, sorting a mixed group of blocks so that the red, blue, green, and yellow blocks are separated.
- In addition, if these blocks are placed in a pattern (e.g., green, yellow, green, yellow, etc.), your child should be able to both predict which color will come next and create their own identical pattern. This skill will help develop your child’s logical thinking.
Continue to allow your young learner to play with fun building blocks and create their own patterns to help them master this skill.
5) Fractions
Montessori material. Children’s hands. The study of mathematics School and kindergarten. Whole and part. FractionsAs a first-grader, your child will be introduced to fractions as equal shares and basic fractions such as ½, ⅓, and ¼. For children to fully grasp these concepts, it’s essential to keep things intuitive.
For example, you can start by helping them understand that a half is two equal parts, a third is three equal parts, and so forth. They also need to understand that although three is bigger than two, ⅓ is smaller than ½.
Fractions can be tricky for kids to learn, which is why it’s important to use practical and everyday items.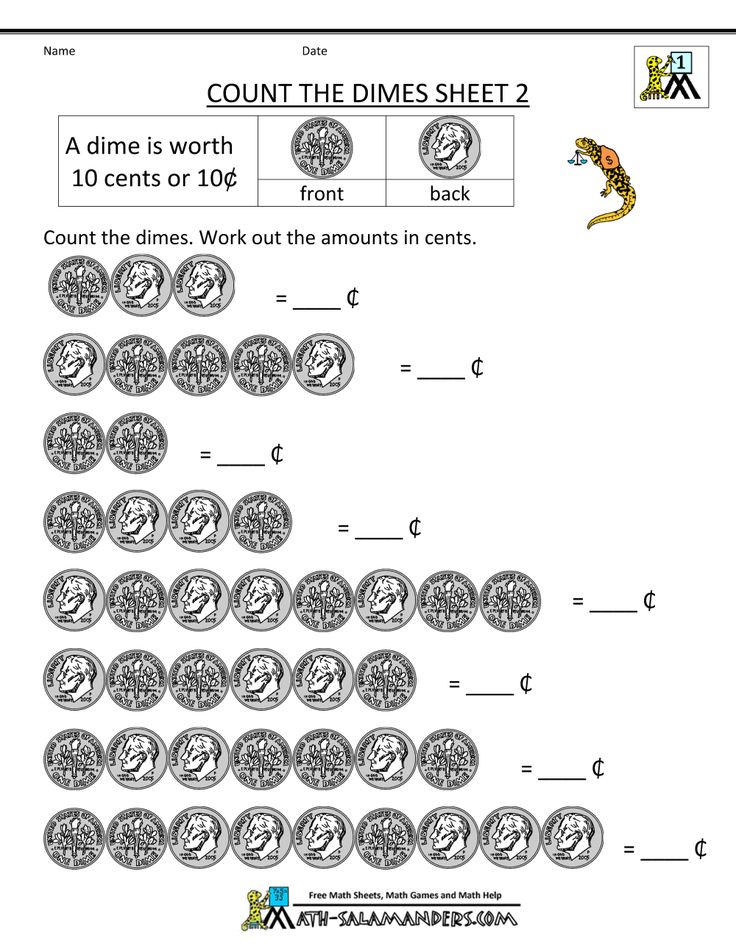
For example, you can help your young learner examine the fractions of a full pizza. Then, as you divide the pizza into different slices, talk about the parts that you’ve created from the whole.
The concept of equal shares can also be demonstrated from one object and a group. For instance, you can have ½ of a single item (e.g., ½ of a cookie), or you can have ½ of a group of objects (e.g., ½ of four cookies is two cookies).
6) Number Place Values
With all the counting in first grade math, your child will naturally be introduced to the concept of place values. For instance, understanding that in the number 288, the 2 is worth 2 “hundreds” (or 200).
There are various activities you can do at home to help your young learner with this concept, including:
- Using number lines
- Base ten blocks
For more ideas to help with number place values and other 1st grade math concepts, take a look at the book Games for Math: Playful Ways to Help Your Child Learn Math, From Kindergarten to Third Grade by HOMER’s very own Peggy Kaye.
7) Time
Telling time (both digital and analog) is an important life skill that kids learn from first grade. The concept of elapsed time will also be introduced at this stage.
In first grade math, your child will learn to:
- Tell time to the nearest hour, half-hour, and quarter-hour (sometimes even to five minutes)
- Make the connection between time and events (e.g., shorter, longer, after, before)
Understanding the analog clock can be tricky for a child who’s only exposed to digital clocks. So help your young learner by buying one (or making one for learning) to hang up at home.
You can then speak to your child about what it means when the hands move. To make things easier, start by helping them tell time to an hour and half-hour before progressing to quarter-hours.
8) Measurements And Comparisons
First grade math also involves some measuring and unit comparisons.
Your child will learn how to measure using a ruler and, after taking measurements, compare and order objects by length. First-graders will also learn how to compare the weights and volumes of different objects.
First-graders will also learn how to compare the weights and volumes of different objects.
To help your young learner at home, keep rulers nearby and take measurements together of some of the objects they love (e.g., stuffed toys, cookies, etc.).
Bonus tip: If you’re a regular baker, why not help them see how you use measuring tools to create their favorite treats? Yum!
Helping Your Child With First Grade Math
We’ve already mentioned a few ways in which you can help your first grader with math at home. In addition to the above, playing math games is a fun and easy way to practice math at home!
Here are some examples of more math activities your young learner will enjoy at home:
- Fill in a number grid puzzle
- Build objects with legos and measure
- Number Hunt, Hopscotch, Is It A Number, and Find A Number
Math Is All Around Us
Helping your child grasp first grade math concepts at home is easier when you focus on the fact that mathematics is a part of our everyday lives.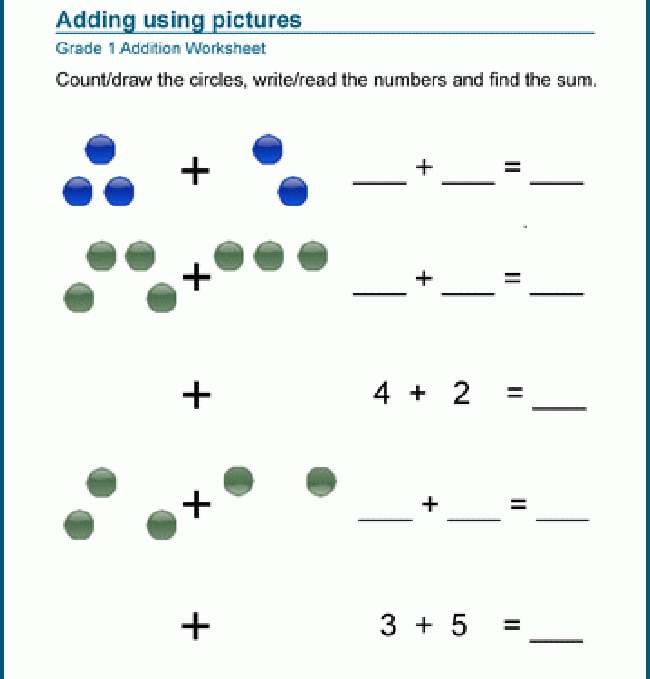 It is in the shape of road signs, the parts of sliced pizza, and even the watches on our wrists!
It is in the shape of road signs, the parts of sliced pizza, and even the watches on our wrists!
Sometimes kids (and parents) forget that math can be lots of fun. So whenever you can, incorporate games and activities to bring a little excitement to all the learning.
Will this help your child become our next best mathematician? Only time will tell. But one thing is for sure — all of the great mathematicians started somewhere. Even Isaac Newton had to master first grade math!
For more ideas and inspiration, visit the HOMER Learn & Grow app.
Author
| Mathematical definitions and concepts | |||||||||||||
| | | Geometric figures. Point and straight line are basic geometric figures. The simplest geometric shapes are a segment, a ray, a broken line. Geometric figures are flat and voluminous. Flat geometric figures are a quadrilateral, triangle, polygon, square, rectangle, circle, oval, rhombus, trapezoid, etc. other | |||||||||||
| | Point. Lines. A point is the smallest geometric figure. Lines are straight, curved, broken. A straight line is a geometric figure that has neither beginning nor end, it is endless. |
A segment is a part of a straight line that has a beginning and an end. Length can be measured. | 3 cm
| ||||||||||
| |
A ray is a part of a straight line that has a beginning but no end. |
| |||||||||||
| | Angle is a geometric figure formed by two rays from one point. |
| |||||||||||
| | Rectangle. A rectangle is a quadrilateral in which all angles are right and opposite sides are equal. The larger side is called the length, the smaller side is called the width. |
| |||||||||||
| | square. A square is a quadrilateral with all sides and angles equal. 9Expressions. 0007 |
| |||||||||||
| Expressions are records of numbers and letters connected by arithmetic signs. Expressions are literal - they contain letters (a + 3), numeric - are composed of numbers (5 - 2). | |||||||||||||
| | Statements. Propositions are true and false sentences about which the question makes sense: is it true or false. | ||||||||||||
| | Equality. Inequality. Equality are statements written with the sign =. An inequality is two numbers or expressions connected by one of their signs: >, To put one of these signs between numbers or expressions means to compare them. | ||||||||||||
| | Increase and decrease by.
| ||||||||||||
| | Components when added. 1 term 2 term Sum 4 4 2 2 6
sum | To find the unknown term, subtract the known term from the sum. | |||||||||||
| | Subtraction components. reduced deductible difference 8- 3 = 5 difference | To find an unknown subtract, it is necessary to subtract the difference 9 from a reduced deduction0007 To find the unknown minuend, add the subtrahend and the difference. | |||||||||||
| | Equation. An equation is an equality with a variable whose value is to be found. To solve an equation means to find all its roots. Algorithm: 1. Select components. 2. Apply the rule. 3. Find the root of the equation. 4. Check. | 1C 2C with x + 2 = 6 x = 6 - 2 x = 4 4 + 2 = 6 6 = 6 | |||||||||||
| | .
| To solve problems, you can use - drawing - diagram - short note Tanya has 4 mushrooms, and Sasha has 2 mushrooms. How many mushrooms do Tanya and Sasha have?
| |||||||||||
| | Whole and parts. ?
Looking for the whole. To find the whole, the parts must be added together. |
Looking for a part. | |||||||||||
| | Comparison task. Tanya has 4 mushrooms, and Sasha has 2 mushrooms. How many more mushrooms does Tanya have than Sasha? | ||||||||||||
| Scheme
T. 4 gr. 2 gr.
> | ||||||||||||
|
| |||||||||||||
| |
| ||||||||||||
Formation of mathematical concepts in primary school
Formation of mathematical concepts in primary school.
The study of mathematics is associated with the assimilation of a certain system of concepts. In order to master this system and then successfully apply the acquired knowledge and skills, teaching younger students and solving the problem of their development by means of mathematics, you must first understand what are the features of mathematical concepts, how their definitions, sentences expressing the properties of concepts are arranged. This knowledge is necessary for a primary school teacher because he is the first to introduce children into the world of mathematical knowledge, and the attitude of the child in the future depends on how competently and successfully he does this.
In order to master this system and then successfully apply the acquired knowledge and skills, teaching younger students and solving the problem of their development by means of mathematics, you must first understand what are the features of mathematical concepts, how their definitions, sentences expressing the properties of concepts are arranged. This knowledge is necessary for a primary school teacher because he is the first to introduce children into the world of mathematical knowledge, and the attitude of the child in the future depends on how competently and successfully he does this.
The appearance in mathematics of new concepts, and hence new terms denoting these concepts, presupposes their definition.
« Definition is a logical operation that reveals the content of the concept. The methods of definition are different: For example, perimeter - is the sum of the sides of some geometric figure.
Perimeter Sum
A = B, and is in
A B
I have no form of coincidence of two concepts.
In elementary school, implicit definitions are mainly found : ostensive and contextual.
In contextual definitions the content of a new concept is revealed through a passage of text, through an analysis of a particular situation. For example: 3 + x = 9
x - unknown number to be found. Which of the numbers 2, 3, 6, 7 should be put instead of x for the equality to be true? This number is 6.
Ostensive definitions are used to introduce terms by showing the objects that these terms denote. Therefore, these definitions are called another definitions by display. For example, in this way equalities 9 are determined in elementary school 0488 and inequalities:
2 ∙ 7 > 2 ∙ 6 9 ∙ 3 = 27
78 – 9
37 + 6 > 37 17 – 5 = 89 + 4
combining context and display.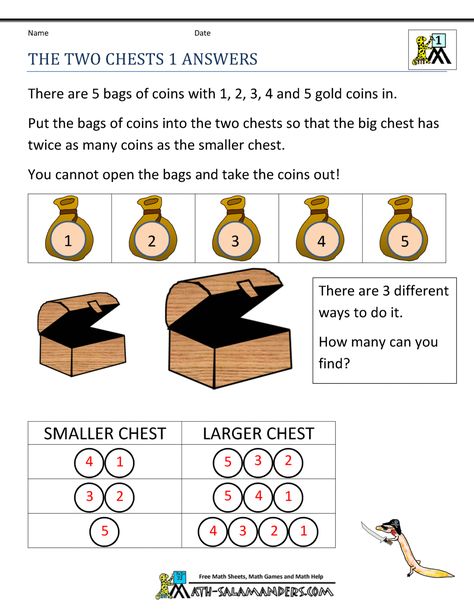
At the next stages of learning, explicit definitions appear, in which two concepts are identified. One of them is called defined by concept, the other is called defining . The content of the defined concept is revealed through the defining concept. The structure of such definitions can be represented by a figure.
Determined concept
Special difference of
Credular concept
+
The defining concept
for example: rectangle - is four -leggel , for all the angles. rectangle
all angles are right
quadrilateral
= , +
[2]
, then it is impossible to say about the definition whether it is true or false; it is neither proven nor disproved. But when formulating definitions, they adhere to a number of rules:
1. The definition must be proportionate.
The definition must be proportionate.
2. There must be no vicious circle in the definition (or their system).
3. The definition must be clear.
4. One and the same concept through genus and species difference can be defined in different ways.
Different definitions of the same concept are possible because of the large number of properties included in the content of the concept, only a few are included in the definition. And when one of the possible definitions is chosen, they proceed from which of them is simpler and more expedient for further construction of the theory.
If the same concept is given two different definitions, then it is necessary to prove their equivalence, i.e. make sure that properties included in one definition imply properties included in another, and vice versa.
An important task of the teacher is that students learn to recognize objects by belonging to a particular class or, in another language, be able to bring an object under a concept.
Mathematical concepts - the most important integral part of the science and the subject of mathematics. At the initial stage of education, students become familiar with most mathematical concepts visually, by contemplating specific examples or by using them in practice, for example, when counting them. In this case, the teacher draws on the life experience of the students.
Stages of concept formation:
1. Organization of observations of single objects (sensory-concrete perception).
2. Enrichment of observation.
3. Identification of common, essential features of the objects under study.
4. Definition of the concept.
5. Refinement and consolidation in memory of the essential features of the concept.
6. Establishing a connection between this concept and others.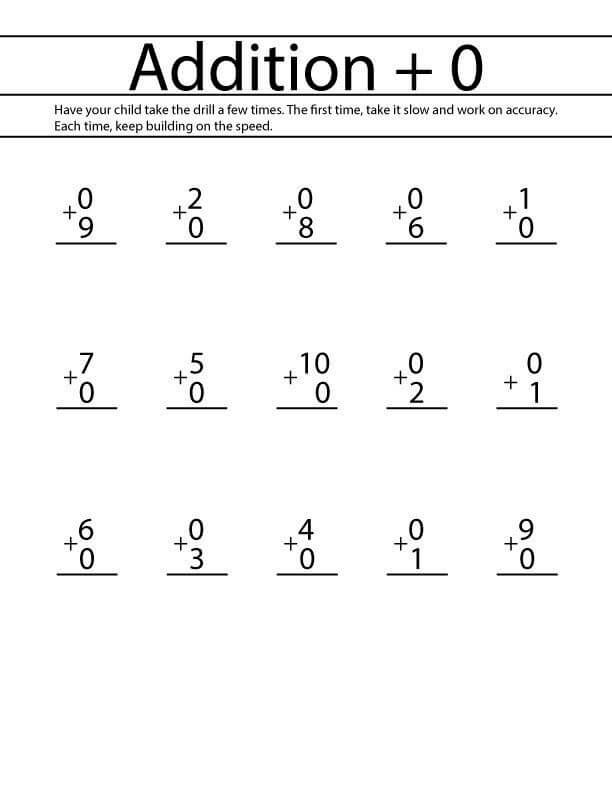
7. Application of the concept in solving elementary educational problems.
8. Classification of concepts - drawing up classification schemes.
9. Exercises to determine the relationship of genus and species.
10. Application of concepts in solving problems of a creative nature.
11. Enrichment of the concept.
12. Secondary more complete definition of the concept.
13. Relying on a given concept when assimilating a new concept.
14. New enrichment of the concept.
15. Establishing links and relationships of the new concept with other concepts .
Conditions for the successful formation of concepts:
We call successful assimilation in which students fully master the content, scope of the concept, knowledge of its connections and relationships with other concepts, as well as the ability to operate with the concept in solving educational and practical problems.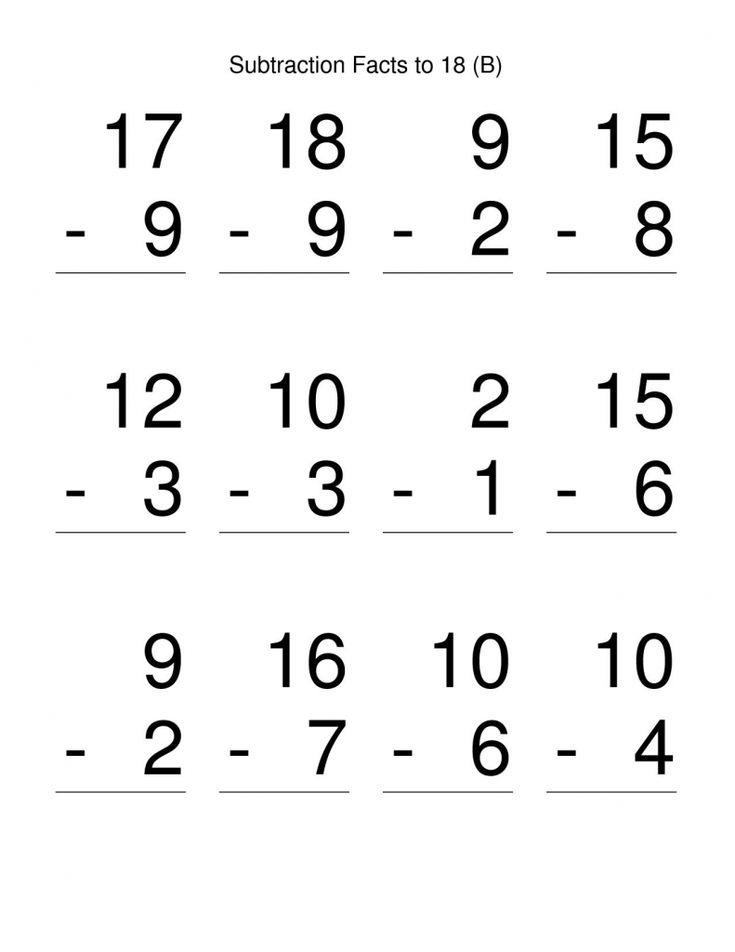
For the successful formation of scientific concepts in students, it is necessary for the teacher to comply with a whole number of conditions :
2. Knowledge of possible sources of concept formation and their influence on the quality of assimilation of formed concepts.
3. Compliance with the stages of concept formation.
4. Organization of active cognitive activity of students at all stages of concept formation.
5. Operational control over the quality of mastering the concept, taking into account the fact that the earlier an error in mastering the concept is detected, the easier it is to overcome it.
6. Motivated introduction of each concept, disclosure to students of its meaning and place in the system of scientific concepts and in practice.
Concept levels:
1. the student learns the concepts;
the student learns the concepts;
2. knows the wording of the definition;
3. understands the meaning of each word, each constituent part of the definition, separates essential properties from non-essential ones;
4. can give his own examples of objects that fit the definition;
5. can prove why one object fits the definition and another does not;
6. can use concepts in explicit situations when solving problems;
7. Can use concepts to solve non-standard problems.
The listed levels are specific didactic goals for studying concepts. Exploring the process of concept formation, L.S. Vygotsky established the following pattern: “The development of processes that subsequently lead to the formation of concepts has its roots deep in childhood, but only in adolescence do those intellectual functions mature and take shape that, in a peculiar combination, form the psychological basis for the formation of concepts. ”
”
Direct teaching of concepts is always practically impossible and pedagogically fruitless. Scientific concepts are not assimilated and not memorized by a child, are not taken up by memory, but arise and add up with the help of the greatest exertion of all the activity of his own thought. The power of scientific concepts is found in that area, which is entirely determined by the highest properties of concepts - awareness and arbitrariness.
Formation of concepts is a long and complex process, which should be given sufficient attention. Important in the formation of the concept is assimilation of its essential features. The verbal definition of the concept should be the result of work on the assimilation of essential features. However, it often happens like this: a verbal definition of a concept is given, and it is immediately used in further work, despite the fact that not all students have mastered it well enough. Excessive exaggeration of the role of verbal definition is one of the reasons for gaps in students' knowledge.
A big drawback is the tradition to illustrate the definition of a concept with one or two particular examples, instead of considering all the essential features of the concept. Such inattention leads to the fact that students mainly pay attention to non-essential signs. Better assimilation of the essential features of the concept contributes to variation of non-essential features .
The focus should not be on memorizing definitions, but on the ability to define concepts . It is important to bring to the consciousness of students that scientific concepts are changeable: the definition of a concept is only one of the initial stages of its formation, and then there is a process of development of the concept - a gradual refinement and assimilation of the content and scope of the concept, its connections and relationships with other concepts.
Each concept must be correctly understood, consciously and clearly assimilated by all students during the lesson.


 Corners
Corners 
 To find a part, you must subtract the other part from the whole.
To find a part, you must subtract the other part from the whole.