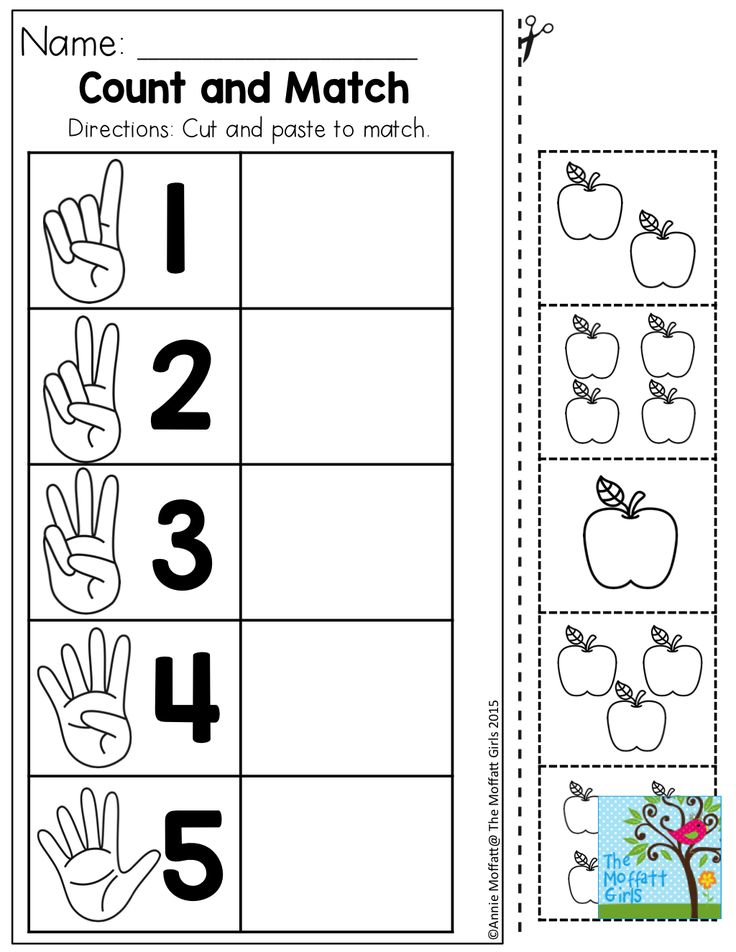
Number matching activities

7 Number Matching Games Your Child Will Want To Play

Whether your child is just starting to learn preschool math or they are becoming a pre-k math whiz, number matching games can be an incredibly helpful, fun way to develop your child’s skills with number recognition.

When talking about number recognition, we mean that your child is able to consistently recognize numbers by both name and written number. This process can take some time.

Just as your child must learn how to read letters, they must also learn to “read” numbers. And that can be a lot like learning a new language! Don’t worry; we’re here to help.

About Number Matching

Number matching is an essential skill for young children and can be done in a few different ways:

Identifying the number name after being given a number of objects
Matching the amount of objects in a picture with their respective number
Matching the name of a number with its corresponding number symbol

As we’ve mentioned, this process won’t come naturally. It may take some patience and clear instruction for your child to understand how number names, objects, and number symbols all correspond to one another.

Thankfully, there are easy — and enjoyable! — methods for helping your child learn.

Below, we’ve compiled 7 number matching games to transform this learning experience into a fun activity your child will look forward to.

7 Number Matching Games For Fun Learning

1) How Many Items?

What You’ll Need

40 or so small items
- Paper clips and dried kidney beans work well
10 pieces of paper
- Each paper should be labeled with a number 1 – 10

What To Do

This game is a great way to master the basics of matching a specific amount of objects with their corresponding number — the perfect activity for preschool math learners!

To play, lay out all of the sheets with numbers. Your child will close their eyes, swirl their arm around, and place their finger on a random number.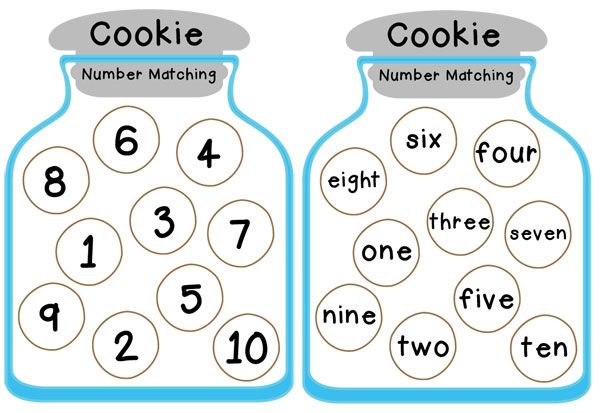

They’ll open their eyes and look at the number they chose. For our example, let’s use the number 7. Once your child picks a number, they’ll count out 7 beans (or whatever small item you’re using).

Repeat the process until all 10 numbers are filled.

This activity is great for working on your child’s understanding of one-to-one correspondence (the idea that each object counted corresponds to one and only one number). This activity can also help build a child’s sense of number, which will help when they engage in mental math.

2) Splash Zone!

What You’ll Need

Colorful chalk
A spray bottle full of water (or an outdoor water hose)

What To Do

To start, you’ll use the chalk to write out the numbers 1 – 10 in large, bold print on pavement. If your child is a bit younger, feel free to scale back and begin with numbers 1 – 5.

You will then call out a number, and your child will race to spray that specific number with the water bottle.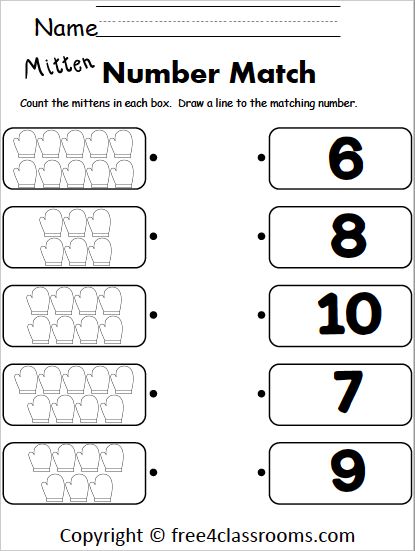 The water will likely erase portions of the number. This is actually a good thing!

When your child is just starting out with number matching, they may become easily confused and mix up numbers. By marking numbers with water, they will know that that number is “unavailable” for the next round.

Make sure you let them know each number can only be sprayed and used one time. You can scale the level of this activity by adding or subtracting the amount of numbers your child is working with.

You can also increase the difficulty by clapping with your hands instead of calling out a number. Your child will have to listen carefully to count how many times you clapped, then spray the correct number.

3) Number Scavenger Hunt

What You’ll Need

Good weather
Paper
Pen

What To Do

You can play this game indoors or outdoors. It’s a great activity for passing the time during a walk around the neighborhood!

Write out a “checklist” of numbers 1 – 10 on the sheet of paper. Make sure the numbers are big, bold, and legible because your child will use the checklist as a reference during your scavenger hunt.

Then, off you go! You will work together to match the numbers on the checklist with the numbers they see in real life.

Mailboxes, clocks, menus at outdoor cafes — numbers could be anywhere! Feel free to play up the detective aspect of the game. It’s part of the fun!

4) Hungry, Hungry Giraffe

What You’ll Need

A stuffed giraffe (or any animal)
Green construction paper cut into leaf shapes
- You’ll need 10 leaf shapes
- Each leaf should be labeled with a number 1 – 10

What To Do

For this game, your child will play the zookeeper of a very hungry giraffe. But this giraffe is special because he’s a picky eater — he only eats certain numbers!

You will play the puppeteer and talk on behalf of the giraffe. When the giraffe grumbles about being hungry, he will complain about craving a specific number. For example, he may say, “Wow, I’m starving! If only I had a leaf with the number 7 on it to eat right now…”

Your child will then locate the leaf marked with a 7 and feed it to the picky giraffe.

If they feed the giraffe the wrong number, the giraffe will spit it out. “Yuck! That doesn’t taste like 7! That was the [number your child gave] leaf! I’m still hungry!”

Your child will probably laugh at the giraffe’s funny reaction. Tell them to try again until they get the right number.

This game is very silly and fun and gives your child multiple opportunities to find the correctly labeled leaf without demotivating them in the process.

5) Fill The Cup

What You’ll Need

A small cup (you can also cut off the top portion of a larger, plastic cup)
Large dried beans, pebbles, Cheerios, or other objects that are easy to count
A pair of dice

What To Do

You and your child will roll your dice at the same time. Depending on what number you roll, you will add that many beans to your plastic cup.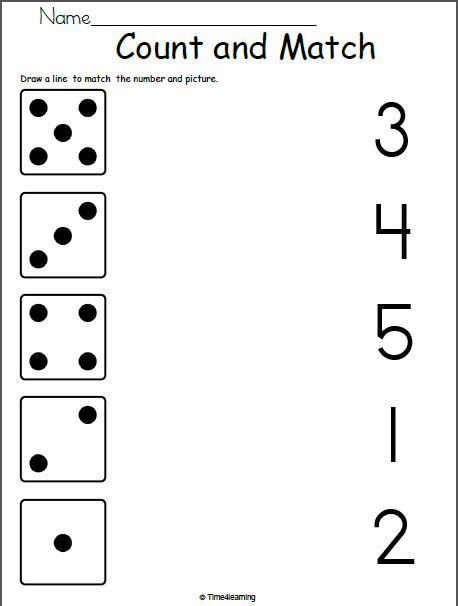

The goal is to finish filling up your cup before the other person. Nothing is more fun than a friendly competition! Your child will excitedly roll their dice and squeal as they count out their items.

Your child must match the dots on the dice to the number of beans they add to play fair, so feel free to help them get used to the process before diving in!

If you notice they’re scooping up too many just to rush and fill their cup, feel free to change the rules of the game. Instead of rolling their own dice, you can roll for one another.

That means if they roll a 6, you count 6 beans into their cup and vice versa. That way they are counting with you — which doubles their practice — and you keep each other accountable!

6) Sticky Ball Match-Up

What You’ll Need

10 sheets of paper, each labeled sequentially with a number between 1 and 10
Tape
A balled-up piece of paper covered in tape

What To Do

After labeling the sheets of paper, tape them to a wall or flat against the ground.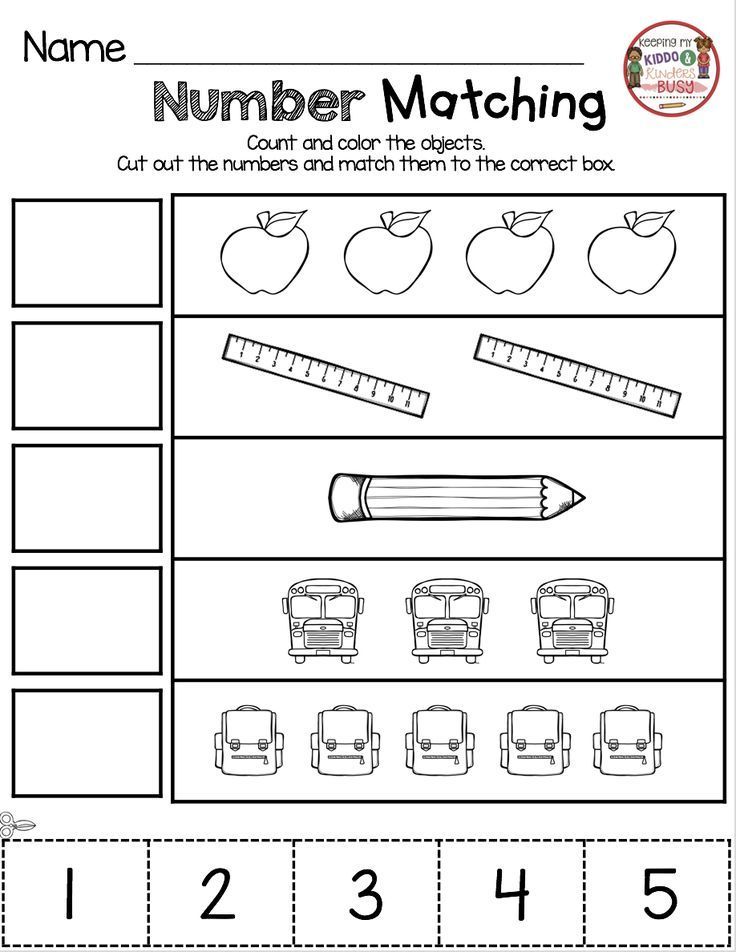

Then, give your child the sticky ball. You will call out a random number and their goal is to throw the ball on the right sheet of paper and make it stick. They can try as many times as necessary to “score” and hit the right sheet of paper.

This game is simple but effective and easily scaled. One way to increase the difficulty, for example, is by representing the numbers on the paper with dots instead of number symbols.

Do what’s most appropriate for your child. Number matching games should be challenging but attainable for young learners!

7) Numbered Chocolate Chip Cookies

What You’ll Need

Cookie dough (without add-ins)
Chocolate chips
Dice

What To Do

Number matching games are fun, but they can taste good, too! With this game, the reward is built-in, as you’ll be making cookies together.

Pick your favorite chocolate chip cookie recipe. Before adding chocolate chips to the batter, portion out enough batter to form the cookies.

Each cookie dough ball will get its own dice roll. This will determine how many chocolate chips can go into each cookie. You can play with a pair of dice (instead of one) if you like your cookies extra chocolatey!

Since everyone loves chocolate chips in their cookies, your child’s goal is obviously to get the highest score possible! The goal is to reach 6 (or 12 for a pair of dice) chips per cookie.

If you sneak in a couple of extra chocolate chips, we won’t tell (as long as your child counts them!).

Number Matching Games For Everyone!

We hope these activities sparked your sense of imagination and got you thinking about how exciting number matching games can be for your child.

But here at HOMER, we know that busy families like yours may not have time for a number matching game every day of the week. Some days, it’s time to take a bath and hit the hay before you know it!

For the times when you need a helping hand, we recommend checking out our Learn & Grow app or our Explore Numbers Kit.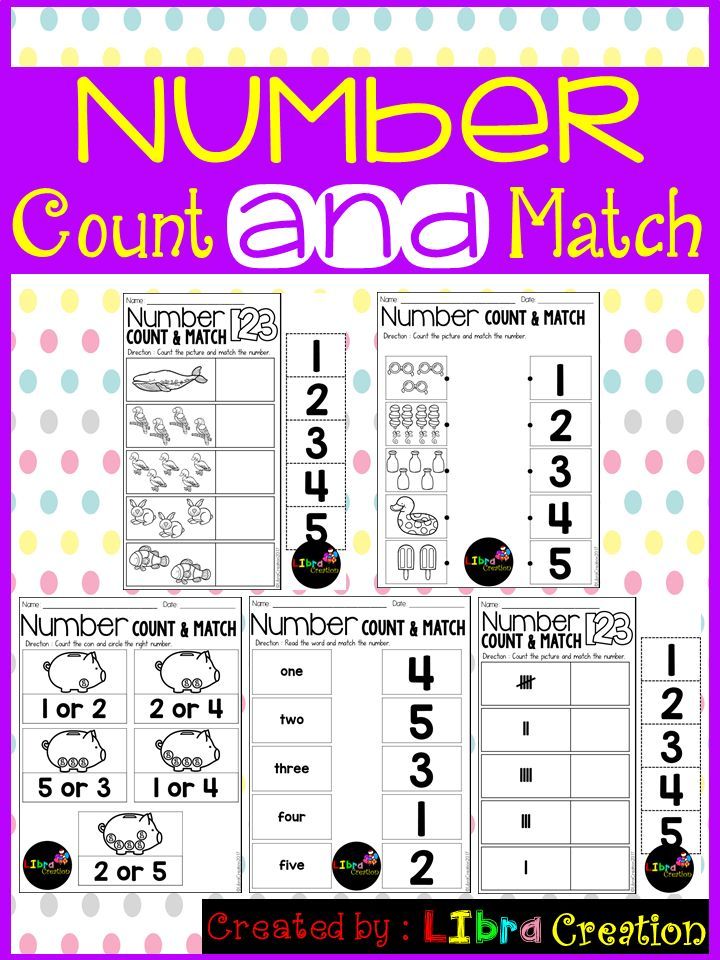 Our personalized products are perfect for squeezing in reading and math practice for emergent learners without sacrificing a morsel of fun!

Author

40 Awesome Number Activities for Preschoolers

You are here: Home / Activities / Learning / Math & 123s / 40+ Awesome Number Activities for Preschoolers

27 Apr

Math & 123s
PopularPreschoolersCounting
Numbers
Resources59 Comments

Number activities for preschoolers don't have to be boring and just worksheets, make them fun so your preschooler will love math!

I love math. I’m a nerd, yes. But I love anything to do with numbers.

I really do hope I can pass this along to my kids by making learning activities about numbers and counting fun for them and not a chore.

As I’m typing this, Henry’s shouting excitedly as he’s counting how many things on his sprayer…though I’m not really sure what he’s counting exactly.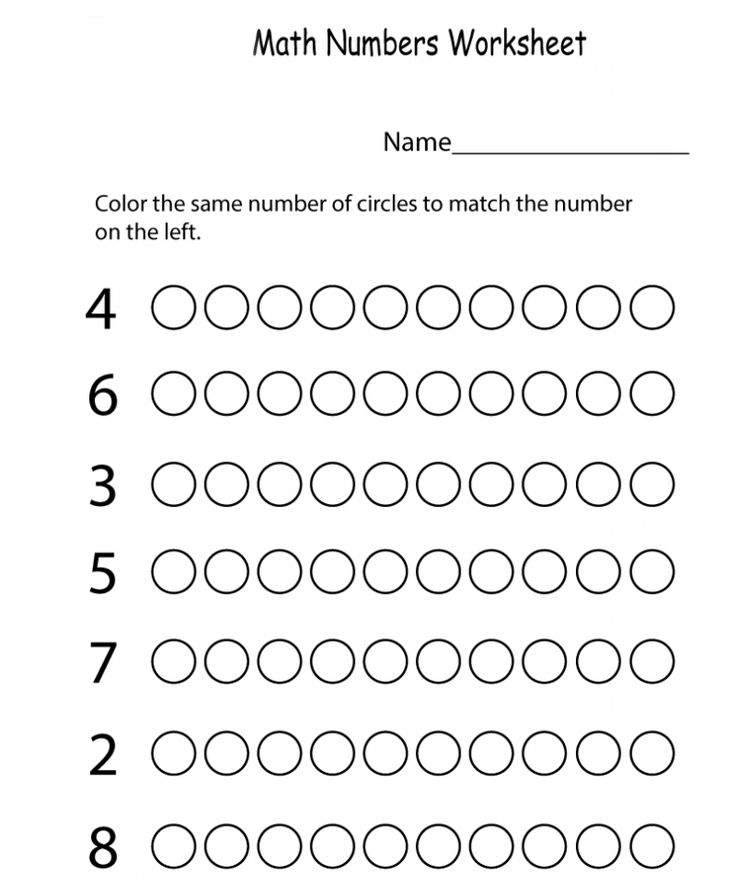 He made it to 39 though!

Because I’m a huge math nerd I searched for easy and fun number activities for preschoolers.

My Favorite 40+ Number Activities for Preschoolers!

Try these activities to help preschoolers learn their ABCs!

Recognizing Numbers Activities for Preschoolers

Identifying numbers can be a learning experience for preschoolers (and younger!). Many of the ABC recognition activities can also be adjusted for numbers.

My kids love these 12 number activities for preschoolers to recognize numbers.

Turn a number into sensory art – perfect for the 100th day of school!
Go on a hunt for numbers and match it with the same number!
Follow a number from start to finish in a maze.
Trace numbers, really big!
Little Family Fun created a parking lot with numbers.
Pop! Find the number and pop it!
Find and match playing cards.
Have target practice with water balloons and numbers, like Motherhood on a Dime
Make art! Do this paint by number canvas art.
Use stickers and printable number cards to play hide and seek, from Teach Mama.
Draw and paint over numbers with q-tips, like Toddler at Play!
Clip and match with giant numbers from You’ve Got This Math!

Activities for Number Recognition

Counting Activities for Preschoolers

Learning there’s a sequence to the numbers and what comes next. Try these 18 number activities to help preschoolers learn to count.

Connect the dots! A simple past-time activity that reinforces the order of numbers.
Upcycle a box into a puzzle of numbers!
Create a maze of numbers to drive through. Can they count their way to the end?
Stacking up boxes and counting how high you can go!
Make a craft together with multiple pieces. Have your child do the counting!
Count cars of a particular color while on the road, or semis, or vans, whatever suits their interest!
Create a learning game with your ABC mat and number blocks. Choose a number block and pound the corresponding times with a hammer on the corresponding number mat!
Let your child run an experiment and measure how much something holds! How many cups fit?
Make puzzles with a picture. Label sections of the puzzle in numerical order. Cut apart and have your child put it back together again, from Growing in PreK.
Use friendly animal crackers to practice counting and number recognition, like I Can Teach My Child.
Measure objects (or yourself!) and count how big they are, from The Imagination Tree
Count objects around the house. No Time For Flash Cards wants to know “How many doors do you have?”
Play any board game, or make your own! Little Family Fun creates a fish race game!
Count with LEGO! Do Play Learn likes to label a paper with numbers and count out the LEGO next to it.
Snack time! Use dice and fruit snacks and play until you eat them all up, like Kids Activities Blog.
Have a bean bag toss on the stairs, number them!
new
new

Counting Activities for Preschoolers

One to One Correspondence Activities for Preschoolers

Knowing your numbers is one thing and knowing how to count is another. But being able to put them into context that they each have meaning is done with one to one correspondence.

Get ready for preschool with 35 name activities!

I love these 12 number activities that help a preschool practice one to one correspondence.

Head out into nature and explore flowers. Count and compare flower petals!
Go on a hunt for a number and match it to its corresponding dots.
Build towers of blocks on a number mat, with the correct number of blocks high!
Spark your child’s interest with their interests! A farming approach of loading grain bins with the corresponding number of pieces of “grain.”
Have a newspaper throwing game and count up the newspaper balls, or how many you make, or miss!
Make something in the kitchen together, or do an experiment and let your child measure out and do the counting.
Sort through objects by color, and count how many you have of each!
Fill a dump truck with objects (like Dominoes!) and count how many you can fit in there, like Inspiration Laboratories.
Estimate first for some fun and then count item, from Teach Preschool.
Have a counting race in the front yard and pick dandelions in the process!
Roll a die (or two!) and practice one to one correspondence as kids build towers with blocks.
Use a dice and small objects (like pom poms or corn kernels) and count them and fill up a tray.

One to One Number Activities for Preschoolers

Counting and number books that will get preschoolers excited about numbers:

The Baker’s Dozen: A Counting Book
I Spy Numbers
One Big Building: A Counting Book About Construction (Know Your Numbers)

Do you actively work on numbers and counting with your child? Share your favorite activities!

About Jamie Reimer

Jamie learned to be a hands on mom by creating activities, crafts and art projects for her three boys to do. Jamie needed the creative outlet that activities provided to get through the early years of parenting with a smile! Follow Jamie on Pinterest and Instagram!

Reader Interactions

Activities that Hands-on Parents Absolutely Love

How to work with floating point numbers in Python / Sudo Null IT News

By the start of the Fullstack development course in Python, we share solutions to the classic problem of inaccurate floating point numbers. In the material you will find examples of working with functions and classes designed specifically for solving problems with floating point numbers.

Floating point numbers are a fast and efficient way to store and work with numbers. But it is associated with a number of difficulties for beginners and experienced programmers! Here is a classic example:

 >>> 0.1 + 0.2 == 0.3 False

Seeing this for the first time can be confusing. This behavior is correct! Let's talk about why floating point errors are so common, why they occur, and how to deal with them in Python.

The computer is fooling you

You've seen that 0.1 + 0.2 is not 0.3, but the madness doesn't stop there. Here are a couple more confusing examples:

 >>> 0.2 + 0.2 + 0.2 == 0.6 False >>> 1.3 + 2.0 == 3.3 False >>> 1.2 + 2.4 + 3.6 == 7.2 False

The problem also concerns the comparison:

 >>> 0.1 + 0.2 <= 0.3 False >>> 10.4 + 20.8 > 31.2 True >>> 0.8 - 0. 1 > 0.7 True

What's going on? When you enter the number 0.1 into the Python interpreter, it is stored in memory as a floating point number and converted. 0.1 is a base 10 decimal number, but floating point numbers are stored in binary notation. That is, base 0.1 is converted from 10 to 2.

The resulting binary number may not accurately represent the original number in base 10. 0.1 is one example. The binary representation would be 0.0(0011). That is, 0.1 is an infinitely repeating decimal number written with base 2. The same thing happens when ⅓ is written as a decimal number with base 10. It turns out an infinitely repeating decimal number 0.3 (3).

Computer memory is finite, so the infinitely repeating representation of the binary fraction 0.1 is rounded up to the final fraction. Its value depends on the architecture of the computer (32-bit or 64-bit).

You can see the floating point value stored for 0.1 using the .as_integer_ratio() method. The floating point representation consists of a numerator and a denominator:

 >>> numerator, denominator = (0. 1).as_integer_ratio() >>> f"0.1 ≈ {numerator} / {denominator}" '0.1 ≈ 3602879701896397 / 36028797018963968'

To display a fraction to 55 decimal places, use format():

 >>> format(numerator / denominator, ".55f") '0.1000000000000000055511151231257827021181583404541015625'

So 0.1 is rounded up to a number slightly larger than its true value.

Learn more about number methods like .as_integer_ratio() in my article 3 Things You Might Not Know About Numbers in Python.

This float representation error is more common than you might think.

Number representation error is very common

There are three reasons why a number is rounded when represented as a floating point number:

There are more significant digits in the number than floating point allows.
This is an irrational number.
The number is rational, but without a finite binary representation.

64-bit floating point numbers have 16 or 17 significant digits. Any number with more significant digits is rounded up. Irrational numbers such as π and e cannot be represented as a finite fraction with an integer base. And, again, irrational numbers are rounded up anyway when stored as floating point numbers.

These two situations create an infinite set of numbers that cannot be exactly represented as a floating point number. But you're not likely to run into these problems unless you're a chemist dealing with tiny numbers, or a physicist dealing with astronomically large numbers.

What about infinite rational numbers, like 0.1 with base 2? This is where you'll run into most of the trouble with floating point, and thanks to the math—which allows you to determine whether it's a finite fraction or not—you'll run into representational errors more often than you think.

A fraction with base 10 can be represented as a finite fraction if its denominator is the product of powers of prime factors 10. The two prime factors of 10 are 2 and 5, so the fractions ½, ¼, ⅕, ⅛ and ⅒ are finite, and ⅓, ⅐ and ⅑ - no. Base 2 has only one prime factor, 2.

The only finite fractions here are those whose denominator is a power of 2. As a result, the fractions ⅓, ⅕, ⅙, ⅐, ⅑, and ⅒ are infinite when represented in binary notation.

Our first example should now be clearer. 0.1, 0.2 and 0.3 are rounded up when converted to floating point numbers:

 >>> # -----------vvvv Display with 17 significant digits >>> format(0.1, ".17g") '0.10000000000000001' >>> format(0.2, ".17g") '0.20000000000000001' >>> format(0.3, ".17g") '0.29999999999999999'

Adding 0.1 and 0.2 results in a number slightly larger than 0.3:

 >>> 0.1 + 0.2 0.30000000000000004

And since 0.1 + 0.2 is slightly greater than 0.3, and 0.3 is a number slightly less than 0.3, the expression 0.1 + 0.2 == 0.3 turns out to be False.

Every programmer in any language should know about the floating point representation error and be able to deal with it. It is not unique to Python. The output of 0. 1 + 0.2 in different languages can be seen on the site with the appropriate name 0.30000000000000004.com.

How to compare floating point numbers in Python

So how do you deal with floating point representation errors when comparing floating point numbers in Python? The trick is to avoid checking for equality. Instead of ==, >=, or <=, always use math.isclose() with floating point numbers:

 >>> import math >>> math.isclose(0.1 + 0.2, 0.3) True

math.isclose() checks to see if the first argument is close enough to the second. That is, the distance between the two arguments is checked. It is equal to the absolute value of the difference between both values:

 >>> a = 0.1 + 0.2 >>> b = 0.3 >>> abs(a - b) 5.551115123125783e-17

If abs(a - b) is less than a percentage of the greater value of a or b, then a is said to be close enough to b to be considered "equal" to b. This percentage is called the relative error and is specified by the rel_tol named argument to math. isclose(), which defaults to 1e-9.

That is, if abs(a - b) is less than 0.00000001 * max(abs(a), abs(b)), then a and b are considered "close" to each other. This ensures that a and b will be approximately nine decimal places.

You can change the relative error if needed:

 >>> math.isclose(0.1 + 0.2, 0.3, rel_tol=1e-20) False

Of course, the relative error depends on the constraints of the problem, but for most everyday applications the standard relative error should be sufficient. The problem occurs if a or b is zero and rel_tol is less than one. Then, no matter how close the non-zero value is to zero, the relative error guarantees that the proximity test will fail. As a fallback, absolute error is applied here:

 >>> # Relative check fails! >>> # ---------------vvvv Relative tolerance >>> # ----------------------vvvvv max(0, 1e-10) >>> abs(0 - 1e-10) < 1e-9 * 1e-10 False >>> # Absolute check works! >>> # ---------------vvvv Absolute tolerance >>> abs(0 - 1e-10) < 1e-9 True

In math. isclose() this check is done automatically. The absolute error is defined using the named argument abs_tol. But abs_tol is 0.0 by default, so you have to set it manually if you want to check if the value is close to zero.

As a result, the math.isclose() function returns the result of the following comparison - with relative and absolute checks in one expression:

 abs(a - b) <= max(rel_tol * max(abs(a), abs(b) ), abs_tol)

math.isclose() was introduced in PEP 485 and is available since Python 3.5.

When should you use math.isclose()?

In general, math.isclose() should be used when comparing floating point values. Replace == with math.isclose():

 >>> # Don't do this: >>> 0.1 + 0.2 == 0.3 False >>> # Do this instead: >>> math.isclose(0.1 + 0.2, 0.3) True

You have to be careful with >= and <= comparisons. We handle the equality separately using math.isclose() and then check for a strict comparison:

 >>> a, b, c = 0. 1, 0.2, 0.3 >>> # Don't do this: >>> a + b <= c False >>> # Do this instead: >>> math.isclose(a + b, c) or (a + b < c) True

There are alternatives to math.isclose(). If you are working with NumPy, you can use numpy.allclose() and numpy.isclose():

 >>> import numpy as np >>> # Use numpy.allclose() to check if two arrays are equal >>> # to each other within a tolerance. >>> np.allclose([1e10, 1e-7], [1.00001e10, 1e-8]) False >>> np.allclose([1e10, 1e-8], [1.00001e10, 1e-9]) True >>> # Use numpy.isclose() to check if the elements of two arrays >>> # are equal to each other within a tolerance >>> np.isclose([1e10, 1e-7], [1.00001e10, 1e-8]) array([True, False]) >>> np.isclose([1e10, 1e-8], [1.00001e10, 1e-9]) array([ True, True])

Note that the standard relative and absolute errors are not the same as math.isclose(). The standard relative error for numpy.allclose() and numpy.isclose() is 1e-05 and the standard absolute error is 1e-08.

math.isclose() is especially handy for unit tests, although there are alternatives. Python's built-in unittest module has the unittest.TestCase.assertAlmostEqual() method.

But it uses only the absolute difference test. And this is also an assertion, that is, if it fails, an AssertionError occurs, due to which it is unsuitable for comparisons in business logic.

An excellent alternative to math.isclose() for unit testing is the pytest.approx() function from pytest pytest. As with math.isclose(), it takes two arguments and returns whether they are equal or not, within some margin of error:

 >>> import pytest >>> 0.1 + 0.2 == pytest.approx(0.3) True

Like math.isclose(), pytest.approx() has named arguments rel_tol and abs_tol to specify relative and absolute errors. But the standard values are different. rel_tol has 1e-6 and abs_tol has 1e-12.

If the first two arguments passed to pytest.approx() are array-like (that is, it's a Python iterable object, like a list or tuple, or even a NumPy array), then pytest.approx() behaves like numpy. allclose() and returns whether the two arrays are equal or not within the margin of error:

 >>> import numpy as np >>> np.array([0.1, 0.2]) + np.array([0.2, 0.4]) == pytest.approx(np.array([0.3, 0.6])) True

Even dictionary values will do for pytest.approx():

 >>> {'a': 0.1 + 0.2, 'b': 0.2 + 0.4} == pytest.approx({'a': 0.3, ' b': 0.6}) True

Floating point numbers are great for working with numbers where absolute precision is not required. They are fast and efficient in terms of memory consumption. But, if precision is needed, there are a number of alternatives to floats to consider.

Exact alternatives to floating point numbers

Python has two built-in numeric types that provide full precision in situations where floating point numbers are inappropriate: Decimal and Fraction.

The Decimal type

The Decimal type can store decimal values with exactly the precision you need. By default, it stores 28 significant digits (this number can be changed according to the specific task being solved):

 >>> # Import the Decimal type from the decimal module >>> from decimal import Decimal >>> # Values are represented exactly so no rounding error occurs >>> Decimal("0. 1") + Decimal("0.2") == Decimal("0.3") True >>> # By default 28 significant figures are preserved >>> Decimal(1) / Decimal(7) Decimal('0.1428571428571428571428571429') >>> # You can change the significant figures if needed >>> from decimal import getcontext >>> getcontext().prec = 6 # Use 6 significant figures >>> Decimal(1) / Decimal(7) Decimal('0.142857')

To learn more about the Decimal type, see the Python documentation.

The Fraction type

An alternative to floating point numbers is the Fraction type. It can exactly store rational numbers. This fixes problems with representation errors that occur in floating point numbers:

 >>> # import the Fraction type from the fractions module >>> from fractions import Fraction >>> # Instantiate a Fraction with a numerator and denominator >>> Fraction(1, 10) Fraction(1, 10) >>> # Values are represented exactly so no rounding error occurs >>> Fraction(1, 10) + Fraction(2, 10) == Fraction(3, 10) True

The Fraction and Decimal types have many advantages over standard floating point values. But there are also disadvantages: lower speed and increased memory consumption.

If you don't need absolute precision, it's best to stick with floating point numbers. But in the case of financial and mission-critical applications, these disadvantages of the Fraction and Decimal types can be acceptable.

Conclusion

Floating point values are both a boon and a curse. They provide fast arithmetic operations and efficient memory consumption at the expense of imprecise representation. In this article, you learned:

Why floating point numbers are inexact.
Why floating point representation error is common.
How to correctly compare floating point values.
How to accurately represent numbers using the Fraction and Decimal types.

Learn more about numbers in Python. For example, did you know that int is not the only integer type in Python? Find out what else there is, as well as other little-known facts about numbers in my article.

And we will help you improve your skills or master a profession that is in demand at any time from the very beginning:

Course "Algorithms and data structures"

Profession C ++ Developer

Ethical hacker

as well as

techniques for the formation of elementary mathematical representations among preschoolers "- JV "Kindergarten No. 2 GBOU secondary school No. 30 Syzran"

Message on the topic:

"Methods and techniques for the formation of elementary mathematical concepts in preschoolers"

Mathematical knowledge of a preschooler is a directly important basis in the mental development of a child. Thanks to mathematical knowledge, children learn to: analyze, compare, synthesize, perform computational operations, think logically, distinguish between geometric shapes, name their features, navigate in space. Preschool children develop memory, attention, thinking. The knowledge gained in kindergarten, children apply in everyday life. Therefore, the task of the teacher: to arouse children's interest in educational activities, to give the necessary elementary mathematical knowledge, to lead children to independent answers, search for solutions. The teacher must find an approach to each child and give this knowledge to all children.

Methods and techniques for the formation of mathematical representations in preschoolers.

In the process of forming elementary mathematical concepts in preschool children, the teacher uses a variety of teaching methods:

practical,
visual,
verbal,
gaming.

When choosing a method, a number of factors are taken into account:

program tasks to be solved at this stage;

age and individual characteristics of children;

availability of the necessary teaching aids, etc. ;

The teacher's constant attention to a reasonable choice of methods and techniques, their rational use in each specific case ensures:

— successful formation of elementary mathematical concepts and their reflection in speech;

- the ability to perceive and highlight relations of equality and inequality (by number, size, shape), sequential dependence (decrease or increase in size, number), highlight quantity, shape, size as a common feature of the analyzed objects, determine relationships and dependencies;

- orientation of children to the application of mastered methods of practical actions (for example, comparison by comparison, counting, measurement) in new conditions and an independent search for practical ways to identify, detect signs, properties, and connections that are significant in a given situation. For example, in the conditions of the game, to identify the sequence, the pattern of alternation of features, the commonality of properties.

In the formation of elementary mathematical representations, the leading method is a practical method.

Its essence lies in the organization of children's practical activities aimed at mastering strictly defined methods of action with objects or their substitutes (images, graphic drawings, models, etc.).

Characteristic features of the practical method in the formation of elementary mathematical concepts:

— performance of various practical actions;

— wide use of didactic material;

- the emergence of ideas as a result of practical actions with didactic material:

- development of counting skills, measurement and calculation in the most elementary form;

- wide use of formed ideas and mastered actions in everyday life, play, work, i.e. in various types of activity.

This method involves the organization of special exercises that can be offered in the form of a task, organized as actions with demonstration material, or proceed in the form of independent work with handout didactic material.

Exercises can be collective - they are performed by all children at the same time, and individual - they are carried out by an individual child at the teacher's board or table. Collective exercises, in addition to mastering and consolidating knowledge, can be used for control.

Individual, performing the same functions, also serve as a model for which children are guided in collective activities.

Game elements are included in the exercises in all age groups: in the younger ones - in the form of a surprise moment, imitation movements, a fairy-tale character, etc.; in seniors, they acquire the character of a search, a competition.

From the point of view of children's manifestation of activity, independence, creativity in the process of performance, reproductive (imitative) and productive exercises can be distinguished.

The game as a method of teaching and the formation of elementary mathematical representations involves the use in the classroom of individual elements of different types of games (plot, mobile, etc.), game techniques (surprise moment, competition, search, etc. Currently a system of so-called learning games has been developed. 0003

All didactic games on the formation of elementary mathematical representations are divided into several groups:

Games with numbers and numbers
Time travel games
Orientation games in space
Games with geometric shapes
Logic games

Visual and verbal methods in the formation of "elementary" mathematical representations are not independent, they accompany practical and game methods.

Techniques for the formation of mathematical representations.

Kindergarten widely uses methods related to visual, verbal and practical methods and applied in close unity with each other: This is the main method of teaching, it has a visual-practical-effective nature, is performed using a variety of didactic means, makes it possible to form skills and abilities in children. It has the following requirements:

- clarity, dissection of the display of methods of action;

- coordination of actions with verbal explanations;

- accuracy, brevity and expressiveness of speech accompanying the show:

- activation of children's perception, thinking and speech.

Manual for self-exercises. This technique is connected with showing the educator methods of action and follows from it. The instruction reflects what and how to do in order to get the desired result. In the older groups, the instruction is given in full before the start of the task, in the younger ones it precedes each new action.
Explanations, explanations, instructions. These verbal devices are used by the educator when demonstrating a method of action or in the course of performing a task by children in order to prevent errors, overcome difficulties, etc. They should be specific, short and figurative.

Demonstration is appropriate in all age groups when getting acquainted with new actions (application, measurement), but it requires activation of mental activity, excluding direct imitation. During the development of a new action, the formation of the ability to count, measure, it is desirable to avoid repeated display.

Mastering the action and improving it is carried out under the influence of verbal techniques: explanations, instructions, questions. At the same time, the speech expression of the mode of action is being mastered.

Questions for children.

Questions activate the perception, memory, thinking, speech of children, provide comprehension and assimilation of the material. When forming elementary mathematical representations, a series of questions is most significant: from simpler ones aimed at describing specific features, properties of an object, the results of practical actions, that is, ascertaining, to more complex ones that require establishing connections, relationships, dependencies, their justification and explanation, use the simplest proofs.

Most often, such questions are asked after the educator demonstrates the sample or the children complete the exercises. For example, after the children have divided the paper rectangle into two equal parts, the teacher asks: “What did you do? What are these parts called? Why can each of these two parts be called a half? What shape are the parts? How to prove that the squares are obtained? What should be done to divide the rectangle into four equal parts?

Basic requirements for questions as a methodological device:

- accuracy, concreteness, conciseness:

- logical sequence;

— variety of formulations, i. e. the same question should be asked in different ways

— the optimal ratio of reproductive and productive questions depending on the age of the children and the material being studied;

— give children time to think;

- the number of questions should be small, but sufficient to achieve the didactic goal;

- prompting questions should be avoided.

The teacher usually asks the whole group a question, and the called child answers it. In some cases, choral responses are possible, especially in younger groups. Children need to be given the opportunity to think about the answer.

Children's answers should be:

- short or complete, depending on the nature of the question;

- independent, conscious;

- accurate, clear, loud enough;

- grammatically correct (observance of the order of words, the rules for their agreement, the use of special terminology).

In working with preschoolers, an adult often has to resort to the method of reformulating the answer, giving it the correct sample and offering to repeat it. For example: “There are four mushrooms on the shelf,” says the baby. “There are four mushrooms on the shelf,” the teacher clarifies.

During the formation of elementary mathematical concepts in preschoolers comparison, analysis, synthesis, generalization are not only

as cognitive processes (operations), but also as methodological techniques that determine the path along which the child's thought moves in the process of learning.

Comparison is based on establishing similarities and differences between objects. Children compare objects according to quantity, shape, size, spatial arrangement, time intervals - according to duration, etc.

Analysis and synthesis as methodological devices act in unity. An example of their use is the formation in children of ideas about "many" and "one", which arise under the influence of observation and practical actions with objects.

Modeling is a visual-practical technique that includes the creation of models and their use in order to form elementary mathematical concepts in children. The reception is extremely promising due to the following factors:

- the use of models and modeling puts the child in an active position, stimulates his cognitive activity;

- the preschooler has some psychological prerequisites for the introduction of individual models and elements of modeling: the development of visual-effective and visual-figurative thinking.

Models can play different roles: some reproduce external connections, help the child to see those that he does not notice on his own, others reproduce the sought-for but hidden connections, the properties of things that are not directly perceived.

Models are widely used in the formation of

time representations: model of parts of the day, week, year, calendar;
quantitative; numerical ladder, numerical figure, etc. ), spatial: (models of geometric shapes), etc.
when forming elementary mathematical representations, subject, subject-schematic, graphic models are used.

Experimentation is a method of mental education that provides the child with self-identification through trial and error of connections and dependencies hidden from direct observation. For example, experimentation in measurement (size, measurement, volume).
Monitoring and evaluation .

These techniques are interrelated. Control is exercised through monitoring the process of children performing tasks, the results of their actions, and responses. These techniques are combined with instructions, explanations, explanations, demonstration of methods of action for adults as a model, direct assistance, and include the correction of errors.

Methods and results of actions, behavior of children are subject to evaluation. The assessment of an adult who teaches to be guided by a model begins to be combined with the assessment of comrades and self-esteem.