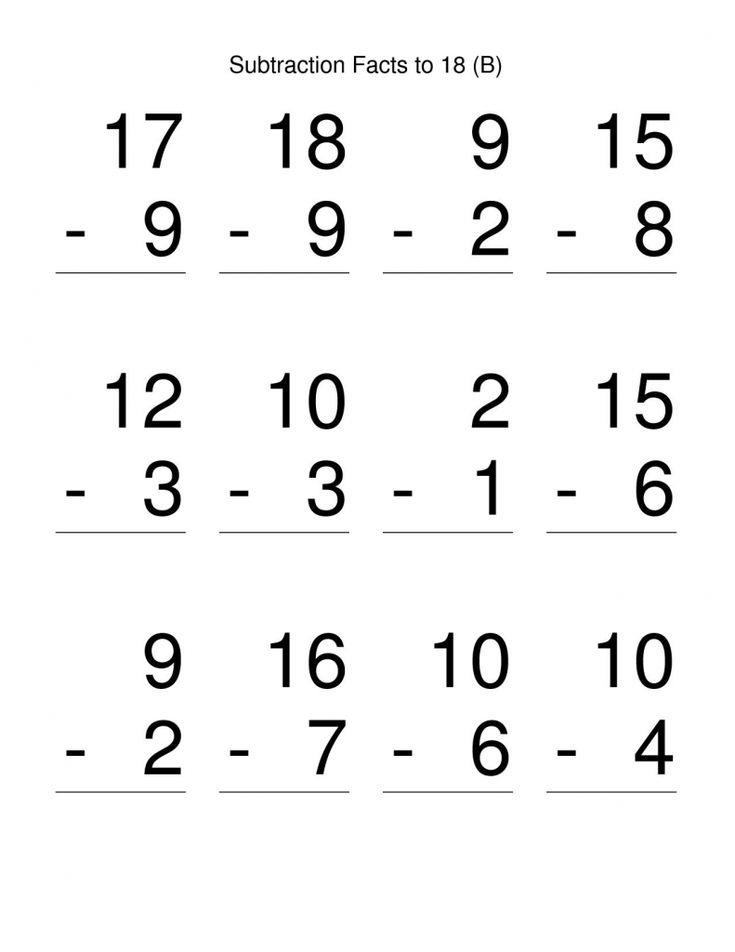
Home » Misc » What are math facts 1st grade

What are math facts 1st grade


Why are Math Facts so Important for Children to Learn

Amina Reshma

3 Min Read

Even though it might seem old school, knowing your math facts fluently and thoroughly is still a crucial skill. So, before delving into the importance of math facts, let’s first define the term.

What are Math Facts?

Basic number combinations for addition, subtraction, multiplication, and division are known as math facts. Children should be able to recall these problems within a few seconds. They are addition, subtraction, multiplication, or even division problems. Children can learn math facts, which are simple calculations, to help them complete math problems more quickly. Math facts can be memorized and remembered easily, freeing up mental energy for higher-order mathematical operations.

Math facts fluency refers to “The ability to recall the basic facts in all four operations accurately, quickly and effortlessly.   When students achieve automaticity with these facts, they have attained a level of mastery that enables them to retrieve them for long-term memory without conscious effort or attention.”1 

Why Should Children Learn Math Facts?

Many people may wonder why children still need to memorize their basic addition, subtraction, multiplication, and division operations in this day and age when we have calculators and computers. 

  • Number sense is developed by math fact fluency.2 Children who have a strong sense of numbers will have a better understanding of how numbers relate to one another. They can use and comprehend ideas and information more effectively. It is an important concept that helps children understand the meaning of numbers as well as their grasp of math and ability to apply math in the real world. A key factor that distinguishes between a cursory understanding and in-depth mastery of a subject is number sense.
  • Complex mathematical concepts are built on math facts foundation. A child will come across a number of concepts fairly early in their learning process, including long multiplication and division, measurement, time telling, counting money, and adding and subtracting larger numbers. Children who spend a lot of time working on the fundamentals are more likely to struggle with the procedures and become lost in their calculations.1 
  • Higher-order mathematical learning is facilitated by math fact fluency. This has been proven by research.1 By automating tasks, children can use their working memory to solve problems and learn new concepts and abilities. Simply put, a child’s inability to recall basic math facts fluently significantly impedes their ability to move forward with problem solving, algebra, and higher-order math concepts.2 Therefore, fluency in math facts aids students in completing more challenging math problems more quickly. If they have mastered their math facts, understanding other concepts will be much simpler, and they will be better prepared to solve them more quickly.  

In fact, cognitive scientists and educators both concur that for students to master higher-order math concepts, they must be able to recall fundamental math facts with ease. At the federal Math Summit’s opening, Grover Whitehurst, the director of the Institute for Educational Sciences (IES), mentioned this study: “Cognitive psychologists have discovered that humans have fixed limits on the attention and memory that can be used to solve problems. One way around these limits is to have certain components of a task become so routine and over-learned that they become automatic.”3

  • Math anxiety is reduced by having solid math fact fluency. Mastering math requires some amount of rote learning. When children struggle with math and fall behind, they tend to develop math anxiety. Early elementary school students should concentrate on learning the foundational math skills needed for later years, including learning math facts, to prevent these anxieties. 1
  • Brain activity is promoted by fluency in math facts. Independent research demonstrates the necessity and significance of students mastering their math facts. They found that learning math facts caused actual changes in brain patterns! “Recent research in cognitive science, using MRI’s, has revealed the actual shift in brain activation patterns as untrained math facts are learned” 4

There is disagreement over whether or not children should  memorize their foundational math facts or be subjected to timed math fact fluency tests. Although math fact fluency is supported by research, many schools have shifted their emphasis away from fact practice and toward more advanced math concepts.5 Regardless of popular opinion, it’s critical to strike a balance between developing skills and applying them if you want to give children the best chance for long-term success.

If you enjoyed the article, you can find more intriguing and educational posts on BYJU’S FutureSchool Blog.

References:

  1. Why do Kids Need to Learn Math Facts? | K5 Learning. (n.d.). Retrieved June 30, 2022, from https://www.k5learning.com/blog/why-do-kids-need-learn-math-facts 
  2. Why Are Math Facts Important? (n.d.). Retrieved June 30, 2022, from http://www.parent-homework-help.com/2010/05/06/why-are-math-facts-important/ 
  3. Math – Elm Street School. (n.d.). Retrieved June 30, 2022, from https://walpoleess.ss5.sharpschool.com/staff_directory/miss_spiniello_s_second_grade/math 
  4. Are Math Facts Important | Important Math Facts | Research. (n.d.). Retrieved June 30, 2022, from https://www.gigglelearn.com/math-facts-research/ 
  5. The truth about math fact fluency (..you’ll be surprised). (n.d.). Retrieved June 30, 2022, from https://www.differentiatedteaching.com/math-fact-fluency-matters/ 

Learn with Math, Math

You might also like

1st Grade Math Skills, What Your Child Will Learn, Komodo Math

  • Math Tips
  • Education
  • 1st

Your child is heading to first grade! After the year in kindergarten, your first grader will be ready for some amazing growth.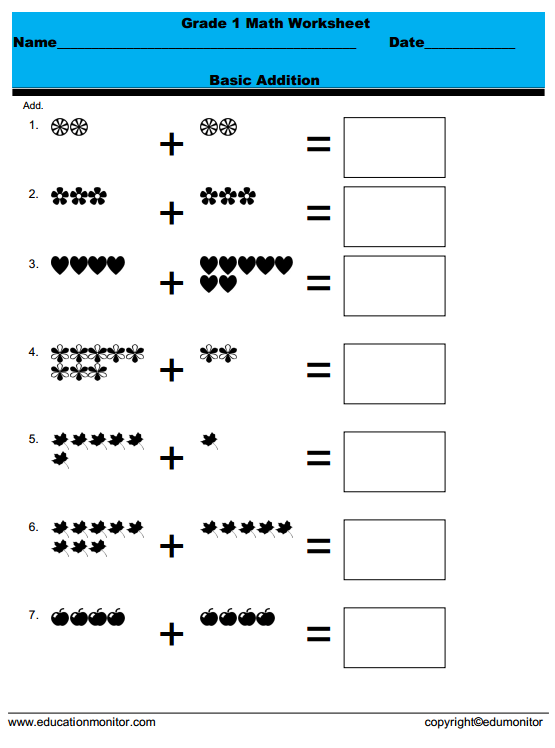 For many children, first grade is the year that they bloom as readers and mathematicians. Get ready to support your child’s mathematical growth by learning about first grade math skills. 

In first grade, you can expect your child to learn about: 

1. Addition and subtraction facts to 20 

Now that your child has mastered the idea of adding and subtracting, they’re ready to practice math facts. This means getting faster when answering addition and subtraction problems to 20. 

Help your child develop fluency by asking basic addition and subtraction problems - we find that using treats can help keep kids interested! If your first grader needs support, encourage the use of physical objects or fingers as problem-solving tools. 

2. Addition and subtraction as inverse operations 

Your child probably understands the concept of addition as “putting together” and subtraction as “taking apart.” In first grade, children are encouraged to see the connections between addition and subtraction. Your child will learn how addition and subtraction are inverse operations, or that one is the opposite of the other, and create “fact families” of related addition and subtraction problems. 

When working with addition and subtraction, ask your child to see connections. For example, if your child has four dolls and three cars, ask how many toys there are in all. Then ask how many toys there would be if the four dolls are taken away. 

3. Count and write within 120 

Your child has probably mastered counting to 20. But in first grade kids will learn to count all the way up to 120! That’s not all. Kids will be expected to not only count, but write, the numbers. This is great practice for understanding multi-digit numbers. 

At home: Encourage your child to write numbers whenever possible. Talk about how two-digit numbers are made up of tens and ones and how three-digit numbers are made up of hundreds, tens, and ones. Just looking closely at multi-digit numbers together can be a great learning opportunity.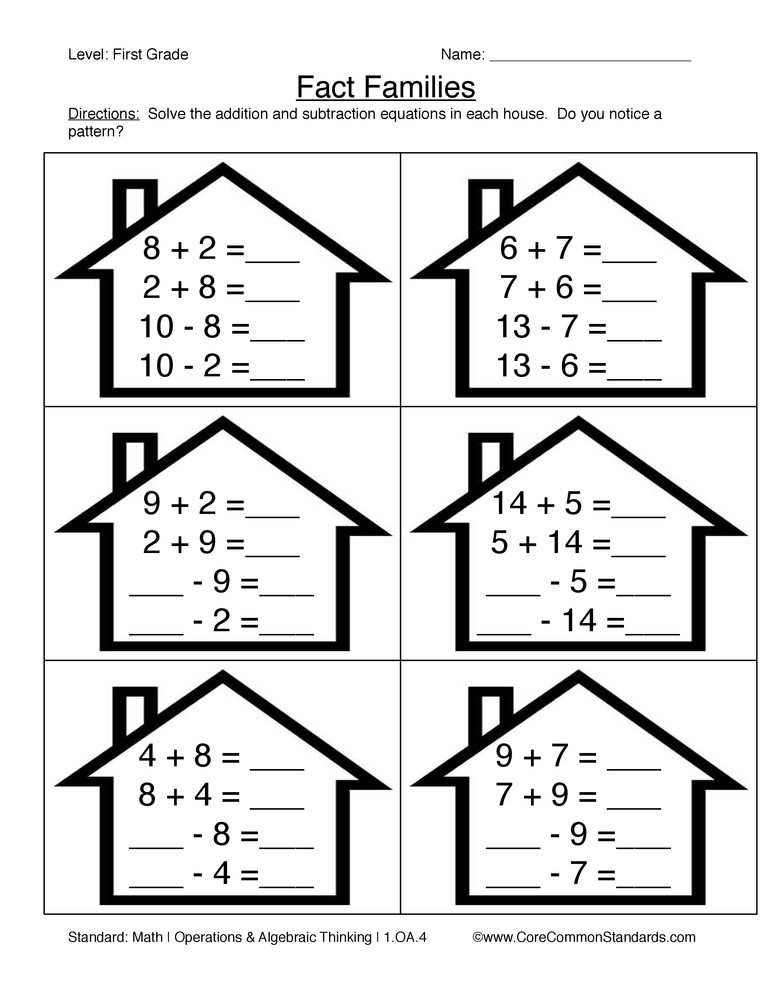  

4. Add within 100 

Now that your child has an understanding of numbers past 100 as well as basic addition and subtraction facts, it’s time to practice adding within 100. Children will practice adding one-digit numbers to two-digit numbers using strategies like counting on and number charts. Children can practice adding larger numbers with the help of a 1-100 chart. 

First graders are also ready to practice adding and subtracting 10s to and from two digit numbers. 

At home: Help your child see patterns when adding and subtracting 10s. For example, after solving a problem like 59 - 10 = 49, point out to your child that 49 has one less 10 than 59. This is another great way to learn about place value. 

5. Measure objects

In first grade, kids learn how to measure using rulers and more unusual things like paper clips. After taking measurements, children compare and order objects by length.

At home: Kids love measuring things around the house, so keep a couple of rulers handy. Pay attention to how your child is using a ruler and taking measurements. Sometimes kids don’t quite measure from end to end, so they might need a bit of help...  

6. Tell time to hour and half hour 

One of the trickiest concepts first graders will learn is to tell time. Using analog clocks is confusing, especially when kids are more used to seeing digital clocks. In first grade, your child will learn about the big and little hands of a clock and will practice telling time to the hour and half hour. 

At home: Get hold of an analog clock for your home (either a real one or one made just for learning). Talk with your child about the time and how the hands move around the clock. Remember to just focus on telling time to the hour and half hour to start! 

7.

Understand basic fractions

First graders also get an introduction to fractions as equal shares. They will learn how to divide into equal groups and learn basic fractions like ½, ⅓, and ¼. First graders usually have a good understanding of fairness,  so practicing making equal shares should be a relatively easy task for them!

At home: Help your child to divide pizzas, pies, and sandwiches into equal shares. As you do, talk about the fractions of the whole that you created. 

First graders are ready to dive deep into mathematical concepts. Find time to connect with your child about classroom learning and get ready to have some fun! 

Found this useful? Check out our grade by grade math guides from Kindergarten to 5th grade

Written by Lily Jones, Lily loves all things learning. She has been a kindergarten & first grade teacher, instructional coach, curriculum developer, and teacher trainer. She loves to look at the world with curiosity and inspire people of all ages to love learning. She lives in California with her husband, two kids, and a little dog.

About Komodo – Komodo is a fun and effective way to boost K-5 math skills. Designed for 5 to 11-year-olds to use in the home, Komodo uses a little and often approach to learning math (15 minutes, three to five times per week) that fits into the busy family routine. Komodo helps users develop fluency and confidence in math – without keeping them at the screen for long.

Find out more about Komodo and how it helps thousands of children each year do better at maths – you can even try Komodo for free. 

Back to School - 5 Tips to Help you Ease Back into the Routine

Here are some steps you can take to ease children back from full vacation mode so that the first week of school doesn't knock you sideways.

Mindset - The Path to Mastery

People who have a growth mindset believe that they always have the potential to learn and improve.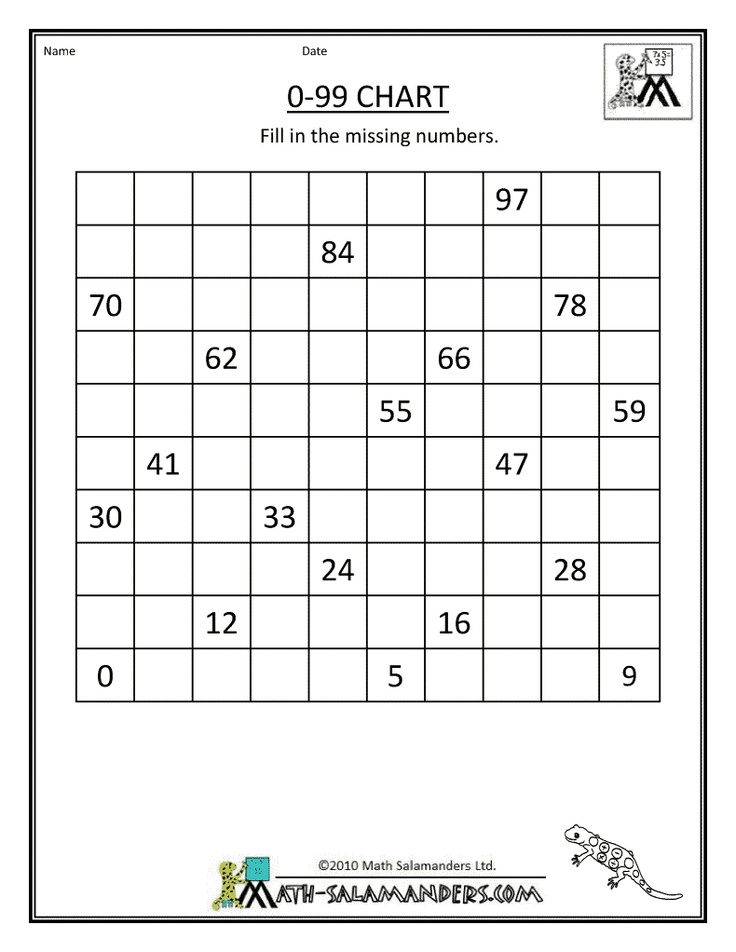 They are more motivated to persevere with difficult tasks, to take risks and to learn from failure.

Interesting facts about mathematics for kids – centro.press

Text: Centropress

11982 fourteen

How to get a child interested in mathematics?

Mathematics is a wonderful science that surrounds us everywhere. It not only develops analytical thinking, but often helps in life situations. In practice, the ancient Egyptians understood this. No wonder a responsible parent wants to see their child love math. The science of numbers is considered to be one of the most ancient teachings. The need for an account arose when there was no writing yet, and people wore loincloths. Here is the first interesting fact about mathematics for elementary school children. Parents, take note!

How to captivate a child with mathematics?

How to captivate a child with mathematics?

Primary school teachers often simply do not have enough time to tell something other than the program. The school tries to captivate the child in its own way, but you, dad or mom, can give the kid the opportunity to really fall in love with the science of numbers by talking about the most unusual and exciting in the history of mathematics. If you are wondering how to interest a child and reveal to him all the beauty of numbers and signs, then our selection of interesting facts about mathematics for elementary school children will become an indispensable and affordable tool. Let's go to surf the arithmetic spaces together.

Elementary School Math Fun Facts

Elementary School Math Fun Facts

  1. The first fact on our list is that the centillion is considered the largest number in the world that has a name.
  2. Italian scientists have found that fish have mathematical abilities. Previously, these creatures were known to be able to distinguish large schools from small ones, but there was evidence that they could even count the number of fish swimming around. True, so far only up to 4.
  3. Some animals can also count, such as dolphins and monkeys.
  4. Mathematics did not know the "=" sign until the 16th century. It was first applied by the British mathematician Robert Record.
  5. An interesting fact about famous mathematicians is that most of them misbehaved at school. Although it is better not to tell the child.
  6. World Mathematics Day and April Fool's Day are celebrated on April 1st.
  7. The magic of numbers is reflected not only in the beauty of equations, but also in the attitude of different peoples towards them. All over the world there are many superstitions on this subject. The most interesting of them: the number "7" is considered the happiest among people from different countries; in many Asian countries, the number "4" is not used, since it is consonant with the word "death"; in the West, the number "13" is credited with an unfortunate influence, so many buildings do not have a 13th floor.
  8. By the way, the belief about the unlucky number "13" came from the Bible. All because of the Last Supper, which was attended by 12 people, including Jesus Christ. The 13th was Judas, who turned out to be a traitor.
  9. There is a concept of "Euclidean geometry". This ancient scientist formulated his ideas about mathematics in the book "Beginnings". Euclid's conclusions were considered absolute truth for almost 2,000 years, until Lobachevsky proved in the 19th century that Euclid's axioms were not so universal. From that moment began a revolution in science.
  10. Mathematics is definitely an exact science. However, history shows that there is a place in it for fateful accidents. There is a story about a student who was late for class. He saw several problems on the board and thought that they should be solved at home. The equations caused him difficulty, but he solved them anyway. This is how easily and simply an ordinary student was able to find answers to problems that the greatest minds of the world had been struggling with for several decades. The name of this student was George Dantzig, who later became one of the founders of linear programming.
  11. The word "algebra" in all languages ​​sounds the same - a fact!
  12. Abraham de Moivre - English mathematician - invented a formula by which he was able to predict the date of his own death. Or did the scientist believe in his prediction so much that he could not justify it?
  13. An interesting fact is that chewing gum improves math skills. American scientists set up an experiment, during which it turned out that those students who chewed gum, passed the exam in mathematics better.
  14. Mathematicians are not just armchair old men or “nerds” with glasses. One of the great mathematicians of antiquity was a four-time Olympic champion. He even received a laurel wreath for winning a fistfight. The name of this scientist is Pythagoras of Samos.
  15. A little earlier we talked about folk superstitions related to numbers and highlighted the most common of them. But if we talk about unusual and confusing beliefs, then the Italians are in the lead. In Italy, the number "17" is considered unkind. And all because in ancient Rome on the tombstones they often wrote "VIXI", in the translation "I lived." The vast majority of the population was illiterate, so the inscription and the Roman numeral (XVII) were often confused. As a result, the number "17" became associated with death. Italians are not only afraid of Friday the 13th, but also Friday the 17th.

Future Albert Einstein

According to the editors of the news portal "Centropress" , the facts listed in the article, of course, are far from all that mathematics can offer. Over its centuries-old history, science has acquired many interesting stories and legends. How many discoveries and turning points that turned the world upside down! Mathematics is truly the queen of sciences. Pass on to your child the love of this exciting knowledge, interest and encourage him. Who knows, maybe the future Einstein is growing up in your family?

Interesting facts about mathematics presentation, report

Slide 1
Slide text:

METHEMATICS, ONE OF THE MOST IMPORTANT SUBJECTS IN THE WORLD!

Slide 2
Slide text:

Interesting facts about mathematics.

Slide 3
Slide text:

Mathematics……..

Mathematics (from other Greek. μάθημα - study, science) - the science of structures, order and relationships, which historically developed on the basis of operations of counting, measuring and describing the forms of real objects [1] (from other Greek μάθημα - study, science) - the science of structures , order and relationships, which historically developed on the basis of operations of counting, measuring and describing the forms of real objects.1 Mathematical objects are created by idealization (from other Greek μάθημα - study, science) - the science of structures, order and relationships, which historically developed on the basis of operations of counting, measuring and describing the shapes of real objects[1]. Mathematical objects are created by idealizing the properties of real or other mathematical objects and writing these properties in a formal language. Mathematics is not a natural science ( from other Greek. μάθημα - study, science) - the science of structures, order and relationships, which historically developed on the basis of operations of counting, measuring and describing the forms of real objects [1]. Mathematical objects are created by idealizing the properties of real or other mathematical objects and writing these properties in a formal language. Mathematics does not belong to the natural sciences, but is widely used in them both for the precise formulation of their content and for obtaining new results [2] (from other Greek μάθημα - study, science) - the science of structures, order and relationships, which historically developed on the basis of operations of counting, measuring and describing the shapes of real objects[1]. Mathematical objects are created by idealizing the properties of real or other mathematical objects and writing these properties in a formal language.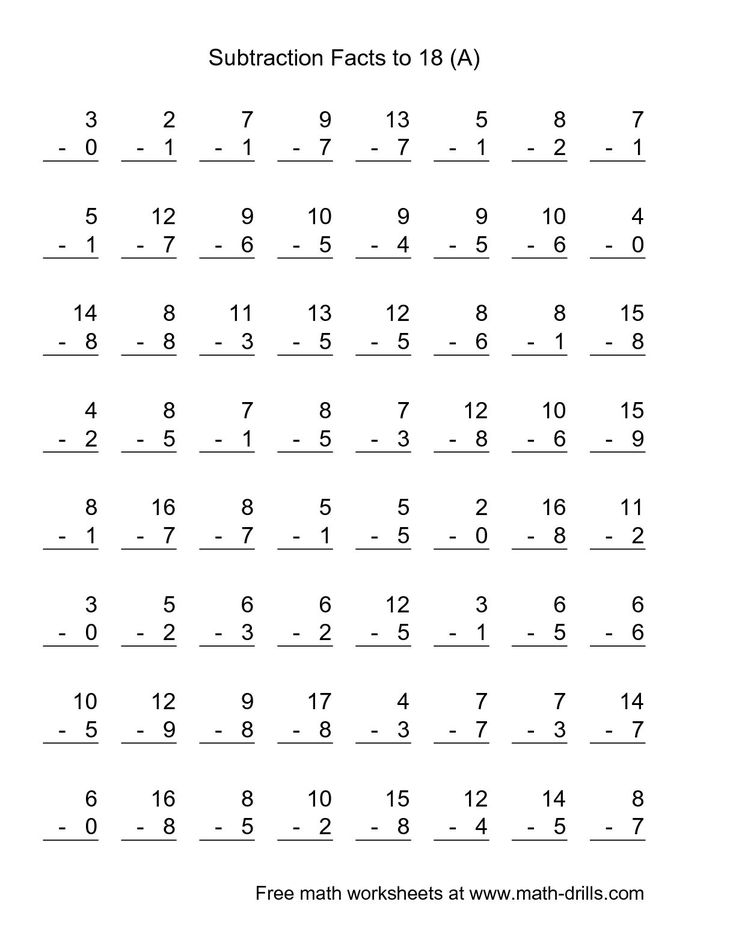 Mathematics does not belong to the natural sciences, but is widely used in them both for the exact formulation of their content and for obtaining new results [2]. Mathematics is a fundamental science that provides (common) linguistic means to other sciences; thus, it reveals their structural relationship and contributes to finding the most general laws of nature[3].

Slide 4
Slide text:

Mathematicians.

French mathematician, physicist, philosopher, writer. He was born into the family of a lawyer involved in mathematics. Early showed mathematical abilities. Has a treatise "Experience on conic sections". Designed a summing machine. Has works on number theory, arithmetic, probability theory. Found a general algorithm for finding signs of divisibility of numbers. Has a treatise on the Arithmetic Triangle. Established the principle of action of liquids and gases. He wrote Letters to a Provincial, a masterpiece of French satirical prose.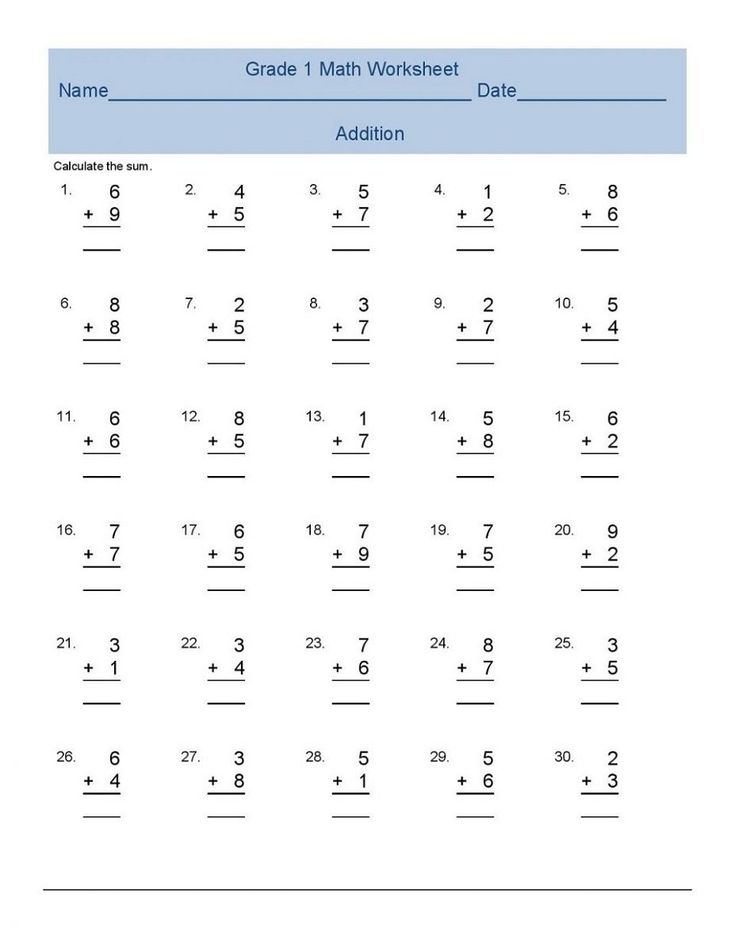

Slide 5
Slide text:

Sofia Vasilievna Kovalevskaya

There was not enough wallpaper to cover the rooms, and the walls of the room were covered with sheets of lithographed lectures by M. V. Ostrogradsky on mathematical analysis. Subsequently, she became the first woman mathematician, Ph.D. She owns the novel The Nihilist.

Slide 6
Slide text:

Carl Gauss (1777-1855)

His mathematical talent manifested itself already in childhood. They say that at the age of 3 he surprised others by correcting his father's calculations with masons. One day at school (Gauss was 10 at the time), the teacher asked the class to add up all the numbers from one to one hundred. While he was dictating the task, Gauss already had an answer ready. On his slate it was written: 101*50=5050

Slide 7
Slide text:

Riddles for children in mathematics.

Slide 8
Slide text:

☺…………………. ☺

Petya and Kolya each bought a box of chocolates. Each box contains 12 candies. Petya ate a few sweets from his box, and Kolya ate as many sweets from his box as Petya had left in his box. How many sweets did Petya and Kolya have left for two?

12 candies.

A hot air balloon is carried by a continuous wind in a southerly direction. In which direction do the flags on his gondola fly?

A ball carried away by an air current is at rest relative to the surrounding air; therefore, the flags will not develop in the wind in any direction, but will hang down, as if in a calm.

Slide 9
Slide text:

Logic tasks

Five diggers dig 5 m of a ditch in 5 hours. How many diggers will it take to dig a 100m ditch in 100 hours?

It will take the same five diggers, no more. In fact, five diggers dig 5 m of a ditch in 5 hours; this means that five diggers would dig 1 m of a ditch in 1 hour, and 100 m in 100 hours.

Slide 10
Slide text:

((((((Poems. ))))))

It is a lie that there is no poetry in science.
In the reflections of the great world
The poet will catch hundreds of colors from the sounds
And the magic lyre will repeat.
Beyond the halls of formulas, forgetting about spring,
Wandering in the world of numbers like a lunatic,
Suddenly a string gives harmony of conclusions,
A mathematician, clinging to a sonorous violin.
A real scientist, he is also a poet,
Eternally eager to know and foresee.
Who said that there is no poetry in science?
You just need to understand and see.

Slide 11
Slide text:

(((…………)))

It is believed that there is a good reason why bird eggs have one end dumber than the other. What is this reason?

Spherical and oval eggs would roll in a straight line. Asymmetric eggs, in which one end is blunter and the other sharper, tend to roll in a circle when rolling.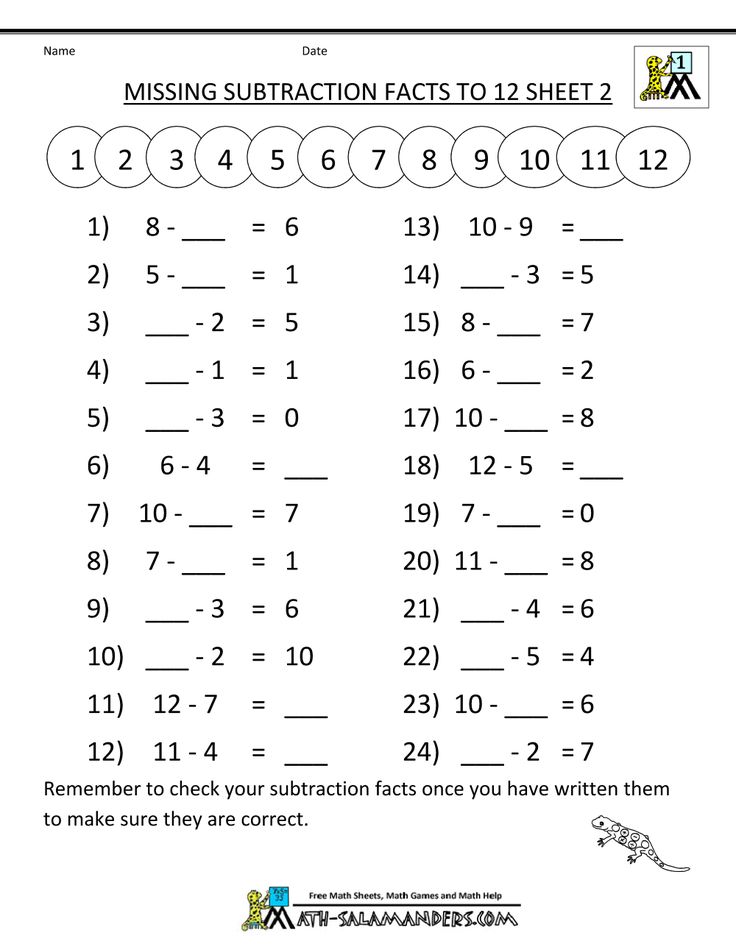 If the egg is lying on the edge of a cliff or in some other unreliable place, the desire to roll in a circle rather than in a straight line is a great advantage.

There are 9 kg of cereals and a pan balance with weights of 50 g and 200 g. Try to weigh 2 kg of this cereal in three steps

You need to hang the cereal into two equal parts of 4.5 kg; then hang one of these parts in half again, that is, 2.25 kg each, and subtract 250 g from one of these parts using the two available weights. Thus, you will get a weight of 2 kg.

Slide 12
Slide text:

RIDDLES AND PUZZLES

How to write the number 1000 with fifteen digits 4?

Has 4 teeth. Every day he appears at the table, but does not eat anything. What's this?

I stand on four legs, but I can't walk at all.

Can it rain for two consecutive days?

Slide 13
Slide text:

Isaac Newton

Great English scientist.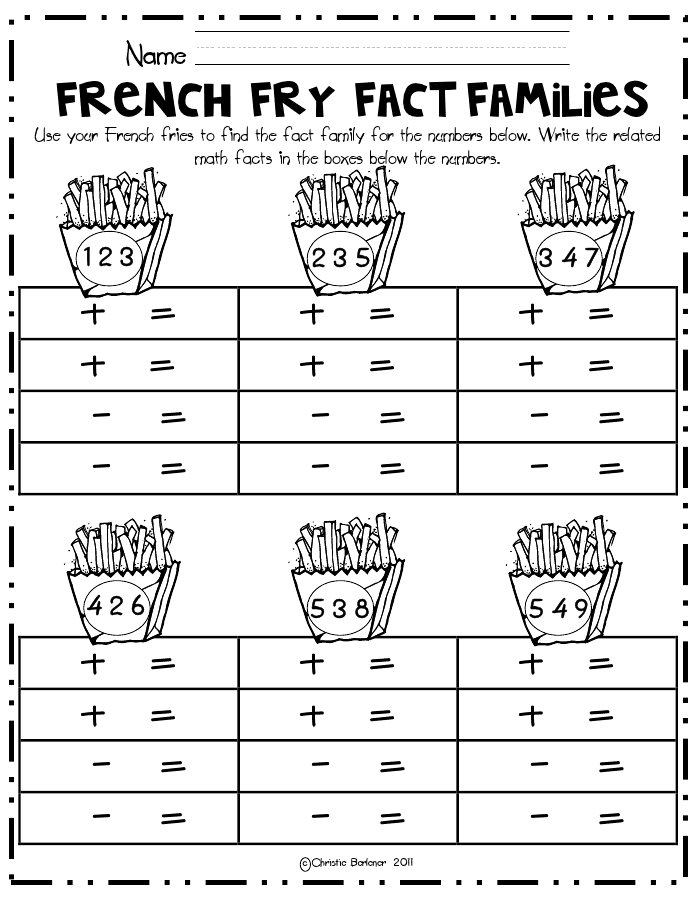 Simultaneously with Leibniz, he developed the foundations of mathematical analysis. Creator of classical mechanics. Newton owns outstanding discoveries in optics, other branches of physics and mathematics. His main work - "The Mathematical Principles of Natural Philosophy" - had a tremendous impact on the development of natural science.

Slide 14
Slide text:

Pafnuty Lvovich Chebyshev (1821-1894)


One of the greatest mathematicians. Studies in number theory put the young Russian mathematician among the first scientists in Europe. He was considered the founder of the Russian school of probability theory. He had works devoted to drawing geographical maps; rational cutting of clothes; he made a cover, tightly fitting the ball; created mechanisms that move along certain curves: a rowing machine that repeats the movement of oars, a scooter chair.

Slide 15
Slide text:

Math examples.

Learn more